Elementary Statistics: Picturing the World (7th Edition)
7th Edition
ISBN: 9780134683416
Author: Ron Larson, Betsy Farber
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 8.3, Problem 14E
Testing the Difference Between Two Means In Exercises 9–20, (a) identify the claim and state H0 and Ha, (b) find the critical value(s) and identify the rejection region(s), (c) calculate
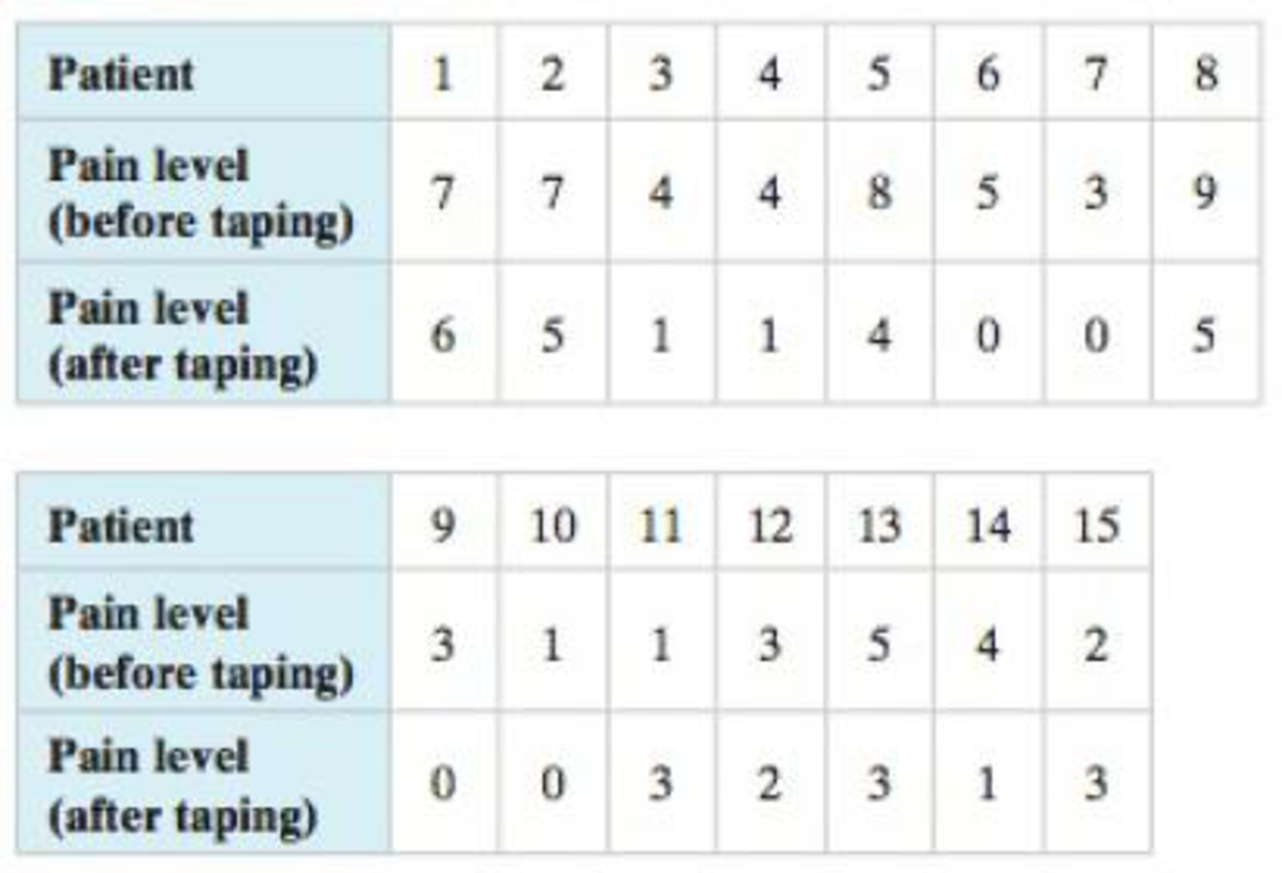
14. Therapeutic Taping A physical therapist claims that the use of a specific type of therapeutic tape reduces pain in patients with chronic tennis elbow. The table shows the pain levels on a scale of 0 to 10, where 0 is no pain and 10 is the worst pain possible, for 15 patients with chronic tennis elbow when holding a 1 kilogram weight. At α = 0.05, is there enough evidence to support the therapist’s claim? (Adapted from BioMed Central, Ltd.)
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
P-values In Exercises 17–20, do the following:
a. Identify the hypothesis test as being two-tailed, left-tailed, or right-tailed.
b. Find the P- value. (See Figure 8-3 on page 364.)
c. Using a significance level of α = 0.05, should we reject H0 or should we fail to reject H0?
The test statistic of z = 1.00 is obtained when testing the claim that p > 0.3.
Applying the Concepts and SkillsIn Exercises, perform a Kruskal–Wallis test by using either the critical-value approach or the P-value approach.TV-Commercial Placement. In the article “Violence and Sex Impair Memory for Television Ads” (Journal of Applied Psychology, Vol. 87, No. 3, pp. 557–564), B. Bushman and A. Bonacci studied whether the type of television program in which commercials are placed affects a viewer’s ability to remember the product. Participants watched a violent, sexually explicit, or neutral TV program that contained nine advertisements. The next day, participants were asked to recall the advertised brands. The number of brands the participants were able to recall are presented in the following table.
Violent
Sexually explict
Neutral
0
0
4
0
2
1.000
2
2
2
1
2
3
1
0
2
0
1
7
At the 5% significance level, do the data provide sufficient evidence to conclude that a difference exists in the median number of advertised brands a viewer is able to recall…
Perform the hypothesis test at 5% significance level using the p-value approach
x=33, s=10, n=24, H0: u=26, Ha: u>26
What is the test statistic, p value, and conclusion for the hypothesis
Chapter 8 Solutions
Elementary Statistics: Picturing the World (7th Edition)
Ch. 8.1 - Classify each pair of samples as independent or...Ch. 8.1 - A survey indicates that the mean annual wages for...Ch. 8.1 - A travel agency claims that the average daily cost...Ch. 8.1 - What is the difference between two samples that...Ch. 8.1 - Explain how to perform a two-sample z-test for the...Ch. 8.1 - Describe another way you can perform a hypothesis...Ch. 8.1 - What conditions are necessary in order to use the...Ch. 8.1 - Prob. 5ECh. 8.1 - Prob. 6ECh. 8.1 - Independent and Dependent Samples In Exercises 58,...
Ch. 8.1 - Prob. 8ECh. 8.1 - In Exercises 9 and 10, use the TI-H4 Plus display...Ch. 8.1 - Prob. 10ECh. 8.1 - Prob. 11ECh. 8.1 - In Exercises 1114, test the claim about the...Ch. 8.1 - In Exercises 1114, test the claim about the...Ch. 8.1 - Prob. 14ECh. 8.1 - Testing the Difference Between Two Means In...Ch. 8.1 - Testing the Difference Between Two Means In...Ch. 8.1 - Prob. 17ECh. 8.1 - Prob. 18ECh. 8.1 - Prob. 19ECh. 8.1 - Testing the Difference Between Two Means In...Ch. 8.1 - Testing the Difference Between Two Means In...Ch. 8.1 - Prob. 22ECh. 8.1 - Prob. 23ECh. 8.1 - Prob. 24ECh. 8.1 - Prob. 25ECh. 8.1 - Getting at the Concept Explain why the null...Ch. 8.1 - Testing a Difference Other Than Zero Sometimes a...Ch. 8.1 - Testing a Difference Other Than Zero Sometimes a...Ch. 8.1 - Prob. 29ECh. 8.1 - Architect Salaries Construct a 99% confidence...Ch. 8.2 - The annual earnings of 25 people with a high...Ch. 8.2 - A manufacturer claims that the mean driving cost...Ch. 8.2 - What conditions are necessary in order to use the...Ch. 8.2 - Explain how to perform a two-sample t-test for the...Ch. 8.2 - Prob. 3ECh. 8.2 - Prob. 4ECh. 8.2 - Prob. 5ECh. 8.2 - Prob. 6ECh. 8.2 - In Exercises 38, use Table 5 in Appendix B to find...Ch. 8.2 - Prob. 8ECh. 8.2 - In Exercises 912, test the claim about the...Ch. 8.2 - Prob. 10ECh. 8.2 - Prob. 11ECh. 8.2 - In Exercises 912, test the claim about the...Ch. 8.2 - Testing the Difference Between Two Means in...Ch. 8.2 - Testing the Difference Between Two Means in...Ch. 8.2 - Testing the Difference Between Two Means in...Ch. 8.2 - Testing the Difference Between Two Means in...Ch. 8.2 - Prob. 17ECh. 8.2 - Prob. 18ECh. 8.2 - Prob. 19ECh. 8.2 - Testing the Difference Between Two Means in...Ch. 8.2 - Testing the Difference Between Two Means in...Ch. 8.2 - Testing the Difference Between Two Means in...Ch. 8.2 - Constructing Confidence Intervals for 1 2 When...Ch. 8.2 - Constructing Confidence Intervals for 1 2 When...Ch. 8.2 - Constructing Confidence Intervals for 1 2 When...Ch. 8.2 - Prob. 26ECh. 8.2 - How Protein Affects Weight Gain in Overeaters In a...Ch. 8.2 - Prob. 2CSCh. 8.2 - How Protein Affects Weight Gain in Overeaters In a...Ch. 8.2 - Prob. 4CSCh. 8.2 - Prob. 5CSCh. 8.3 - A shoe manufacturer claims that athletes can...Ch. 8.3 - Prob. 2TYCh. 8.3 - Prob. 1ECh. 8.3 - Prob. 2ECh. 8.3 - Prob. 3ECh. 8.3 - Prob. 4ECh. 8.3 - Prob. 5ECh. 8.3 - Prob. 6ECh. 8.3 - Prob. 7ECh. 8.3 - Prob. 8ECh. 8.3 - Prob. 9ECh. 8.3 - Testing the Difference Between Two Means In...Ch. 8.3 - Prob. 11ECh. 8.3 - Prob. 12ECh. 8.3 - Prob. 13ECh. 8.3 - Testing the Difference Between Two Means In...Ch. 8.3 - Prob. 15ECh. 8.3 - Prob. 16ECh. 8.3 - Testing the Difference Between Two Means In...Ch. 8.3 - Testing the Difference Between Two Means In...Ch. 8.3 - Testing the Difference Between Two Means In...Ch. 8.3 - Prob. 20ECh. 8.3 - Prob. 21ECh. 8.3 - Prob. 22ECh. 8.3 - Prob. 23ECh. 8.3 - Prob. 24ECh. 8.4 - Consider the results of the study discussed on...Ch. 8.4 - Prob. 2TYCh. 8.4 - What conditions are necessary in order to use the...Ch. 8.4 - Explain how to perform a two-sample z-test for the...Ch. 8.4 - In Exercises 36, determine whether a normal...Ch. 8.4 - Prob. 4ECh. 8.4 - Prob. 5ECh. 8.4 - In Exercises 36, determine whether a normal...Ch. 8.4 - Prob. 7ECh. 8.4 - Testing the Difference Between Two Proportions In...Ch. 8.4 - Prob. 9ECh. 8.4 - Prob. 10ECh. 8.4 - Prob. 11ECh. 8.4 - Testing the Difference Between Two Proportions In...Ch. 8.4 - Prob. 13ECh. 8.4 - Prob. 14ECh. 8.4 - Intermarriages In Exercises 1318, use the figure,...Ch. 8.4 - Prob. 16ECh. 8.4 - Prob. 17ECh. 8.4 - Intermarriages In Exercises 1318, use the figure,...Ch. 8.4 - Prob. 19ECh. 8.4 - Prob. 20ECh. 8.4 - Prob. 21ECh. 8.4 - U.S. Workforce In Exercises 1922, use the figure...Ch. 8.4 - Prob. 23ECh. 8.4 - Prob. 24ECh. 8.4 - Prob. 25ECh. 8.4 - Prob. 26ECh. 8 - Uses Hypothesis Testing with Two Samples...Ch. 8 - Medical research often involves blind and...Ch. 8 - Prob. 8.1.1RECh. 8 - Prob. 8.1.2RECh. 8 - Sample 1: The fuel efficiencies of 20 sports...Ch. 8 - Prob. 8.1.4RECh. 8 - Prob. 8.1.5RECh. 8 - In Exercises 58, test the claim about the...Ch. 8 - Prob. 8.1.7RECh. 8 - In Exercises 58, test the claim about the...Ch. 8 - In Exercises 9 and 10, (a) identify the claim and...Ch. 8 - Prob. 8.1.10RECh. 8 - Prob. 8.2.11RECh. 8 - Prob. 8.2.12RECh. 8 - Prob. 8.2.13RECh. 8 - Prob. 8.2.14RECh. 8 - Prob. 8.2.15RECh. 8 - Prob. 8.2.16RECh. 8 - Prob. 8.2.17RECh. 8 - Prob. 8.2.18RECh. 8 - Prob. 8.3.19RECh. 8 - In Exercises 1922, test the claim about the mean...Ch. 8 - Prob. 8.3.21RECh. 8 - Prob. 8.3.22RECh. 8 - Prob. 8.3.23RECh. 8 - In Exercises 23 and 24, (a) identify the claim and...Ch. 8 - Prob. 8.4.25RECh. 8 - Prob. 8.4.26RECh. 8 - Prob. 8.4.27RECh. 8 - Prob. 8.4.28RECh. 8 - Prob. 8.4.29RECh. 8 - Prob. 8.4.30RECh. 8 - Prob. 1CQCh. 8 - Prob. 2CQCh. 8 - Prob. 3CQCh. 8 - Prob. 4CQCh. 8 - Take this test as you would take a test in class....Ch. 8 - Prob. 2CTCh. 8 - A physical therapist suggests that soft tissue...Ch. 8 - Take this test as you would take a test in class....Ch. 8 - The U.S. Department of Health Human Services...Ch. 8 - Prob. 2RSRDCh. 8 - Prob. 3RSRDCh. 8 - Prob. 4RSRDCh. 8 - Prob. 1TCh. 8 - Prob. 2TCh. 8 - Prob. 3TCh. 8 - Prob. 4TCh. 8 - Prob. 5TCh. 8 - Prob. 1CRCh. 8 - Prob. 2CRCh. 8 - Prob. 3CRCh. 8 - Prob. 4CRCh. 8 - In Exercises 36, construct the indicated...Ch. 8 - In Exercises 36, construct the indicated...Ch. 8 - In Exercises 710, the statement represents a...Ch. 8 - In Exercises 710, the statement represents a...Ch. 8 - In Exercises 710, the statement represents a...Ch. 8 - In Exercises 710, the statement represents a...Ch. 8 - Prob. 11CRCh. 8 - Prob. 12CRCh. 8 - Prob. 13CRCh. 8 - Prob. 14CRCh. 8 - Prob. 15CRCh. 8 - Prob. 16CRCh. 8 - A researcher claims that 5% of people who wear...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.Similar questions
- Test the claim that the proportion of people who own cats is larger than 20% at the 0.005 significance level. The null and alternative hypothesis would be: H2:p = 0.2 Họ:p 2 0.2 H9:p 0.2 H9:p = 0.2 Hg: u 0.2 H1: u 0.2 The test is: left-tailed two-tailed right-tailed Based on a sample of 300 people, 24% owned cats The test statistic is: (to 2 decimals) The p-value is: (to 2 decimals) Based on this we: O Reject the null hypothesis O Fail to reject the null hypothesisarrow_forwardHypothesis testing will consist of four hypothesis tests which you need to complete. While you will not need to show your work or upload any files, each test will require the following: Type of Test: Null Hypothesis: Alternate Hypothesis: SE: Test Statistic: P-value: Decision: Sentence:arrow_forwardWork Conduct the hypothesis test and provide the test statistic and the critical value, and state the conclusion. A person drilled a hole in a die and filled it with a lead weight, then proceeded to roll it 200 times. Here are the observed frequencies for the outcomes of 1, 2, 3, 4, 5, and 6, respectively: 27, 29, 50, 41, 29, 24. Use a 0.10 significance level to test the claim that the outcomes are not equally likely. Does it appear that the loaded die behaves differently than a fair die? Click here to view the chi-square distribution table. The test statistic is. (Round to three decimal places as needed.) The critical value is (Round to three decimal places as needed.) State the conclusion. Ho- There sufficient evidence to support the claim that the outcomes are not equally likely. The outcomes to be equally likely, so the loaded die to behave differently from a fair die.arrow_forward
- Data set presents a sample of the number of defective flash drives produced by a small manufacturing company over the last 30 weeks. The company's operations manager believes that the number of defects produced by the process is less than seven defective flash drives per week. Construct a hypothesis test to verify the operations manager's claim. Hypothesis test should include a t test statistic value, a p value, a decision, and a conclusion. This is the null hypotheses (see attachment): Data: Mean 7.0300 SD 1.3700 SEM 0.2501 N 30arrow_forwardA standardized exam consists of three parts: math, writing, and critical reading. Sample data showing the math and writing scores for a sample of 12 students who took the exam follow. Student Math Writing 1 540 474 2 432 380 3 528 463 4 574 612 5 448 420 6 502 526 7 480 424 8 499 459 9 610 615 10 572 541 11 390 329 12 593 613 (a) Use a 0.05 level of significance and test for a difference between the population mean for the math scores and the population mean for the writing scores. (Use math score − writing score.) Formulate the hypotheses. 1. H0: μd > 0 Ha: μd ≤ 0 2. H0: μd ≤ 0 Ha: μd = 0 3. H0: μd ≠ 0 Ha: μd = 0 4. H0: μd = 0 Ha: μd ≠ 0 5. H0: μd ≤ 0 Ha: μd > 0 Calculate the test statistic. (Round your answer to three decimal places.) Calculate the p-value. (Round your answer to four decimal places.) p-value = What is your conclusion? 1.Do not reject H0. We cannot conclude that there is a significant difference…arrow_forwardA standardized exam consists of three parts: math, writing, and critical reading. Sample data showing the math and writing scores for a sample of 12 students who took the exam follow. Student Math Writing 1 540 474 2 432 380 3 528 457 4 574 606 5 448 420 6 502 526 7 480 430 8 499 459 9 610 615 10 572 541 11 390 335 12 593 613 (a) Use a 0.05 level of significance and test for a difference between the population mean for the math scores and the population mean for the writing scores. (Use math score − writing score.) Formulate the hypotheses. a) H0: ?d = 0 Ha: ?d ≠ 0 b) H0: ?d ≤ 0 Ha: ?d > 0 c)H0: ?d ≤ 0 Ha: ?d = 0 d) H0: ?d > 0 Ha: ?d ≤ 0 e) H0: ?d ≠ 0 Ha: ?d = 0 Calculate the test statistic. (Round your answer to three decimal places.) answer: _____ Calculate the p-value. (Round your answer to four decimal places.) p-value = answer: ______ What is your conclusion? a) Do not reject H0. We can conclude that there is a significant difference…arrow_forward
- Passive and Active Smoke Data Set 12 “Passive and Active Smoke” in Appendix B lists measured cotinine levels from a sample of subjects who smoke, another sample of subjects who do not smoke but are exposed to environmental tobacco smoke, and a third sample of subjects who do not smoke and are not exposed to environmental tobacco smoke. Cotinine is produced when the body absorbs nicotine. Use a 0.01 significance level to test the claim that the three samples are from populations with the same median. What do the results suggest about a smoker who argues that he absorbs as much nicotine as people who don’t smoke?arrow_forwardHypothesis testing is a systematic procedure for determining whether the results of an experiment are significant. List the 5 steps of hypothesis testing:1.2.3.4.5.arrow_forwardTest the claim that the mean GPA of night students is larger than 3.1 at the 0.01 significance level. The null and alternative hypothesis would be: Но: р — 0.775 Но:р> 0.775 Но: и — 3.1 Но:р 3.1 Н:р+ 0.775 Hi:р 3.1 H]:p > 0.775 Hi:р < 3.1 The test is: right-tailed two-tailed left-tailed Based on a sample of 80 people, the sample mean GPA was 3.14 with a standard deviation of 0.07 The test statistic is: (to 2 decimals) The p-value is: (to 2 decimals) Based on this we: O Reject the null hypothesis O Fail to reject the null hypothesisarrow_forward
- Test the claim that the proportion of men who own cats is smaller than the proportion of women who own cats at the .025 significance level. The null and alternative hypothesis would be: Ho MMF Ho: PM = PF H₁: MMF O H₁: PM > PF O The test is: two-tailed right-tailed O The test statistic is: Based on a sample of 60 men, 25% owned cats Based on a sample of 40 women, 40% owned cats The p-value is: Ho: M = μF H₁: UMarrow_forwardHypothesis testing is a statistical method that uses data to evaluate a hypothesis about a population (Gravetter, Wallnau & Forzano, 2018). Outline the four steps that allow us to use sample data to answer questions about an unknown population and include your own examples following each step.arrow_forwardHypothesis testing will consist of four hypothesis tests which you need to complete. While you will not need to show your work or upload any files, each test will require the following: Type of Test: Null Hypothesis: Alternate Hypothesis: SE: Test Statistic: P-value: Decision: Sentence:arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
Recommended textbooks for you
MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
MATLAB: An Introduction with Applications
Statistics
ISBN:9781119256830
Author:Amos Gilat
Publisher:John Wiley & Sons Inc
Probability and Statistics for Engineering and th...
Statistics
ISBN:9781305251809
Author:Jay L. Devore
Publisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...
Statistics
ISBN:9781305504912
Author:Frederick J Gravetter, Larry B. Wallnau
Publisher:Cengage Learning
Elementary Statistics: Picturing the World (7th E...
Statistics
ISBN:9780134683416
Author:Ron Larson, Betsy Farber
Publisher:PEARSON
The Basic Practice of Statistics
Statistics
ISBN:9781319042578
Author:David S. Moore, William I. Notz, Michael A. Fligner
Publisher:W. H. Freeman
Introduction to the Practice of Statistics
Statistics
ISBN:9781319013387
Author:David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:W. H. Freeman
F- Test or F- statistic (F- Test of Equality of Variance); Author: Prof. Arvind Kumar Sing;https://www.youtube.com/watch?v=PdUt7InTyc8;License: Standard Youtube License
Statistics 101: F-ratio Test for Two Equal Variances; Author: Brandon Foltz;https://www.youtube.com/watch?v=UWQO4gX7-lE;License: Standard YouTube License, CC-BY
Hypothesis Testing and Confidence Intervals (FRM Part 1 – Book 2 – Chapter 5); Author: Analystprep;https://www.youtube.com/watch?v=vth3yZIUlGQ;License: Standard YouTube License, CC-BY
Understanding the Levene's Test for Equality of Variances in SPSS; Author: Dr. Todd Grande;https://www.youtube.com/watch?v=udJr8V2P8Xo;License: Standard Youtube License