Chapter 7, Problem 7.7.17P

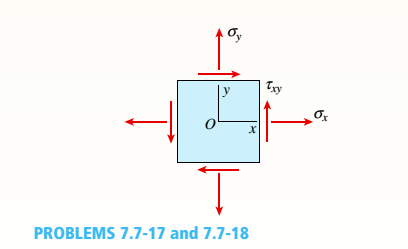
An element in plane stress is subjected to stresses ?? = -8400 psi, ??y = 1100 psi, and

(see figure). The material is aluminum with modulus of elasticity E = 10,000 ksi and Poisson’s ratio v = 0.33.

Determine the following quantities: (a) the strains for an element oriented at an angle 0 = 30°, (b) the principal strains, and (C) the maximum shear strains. Show the results on sketches of properly oriented elements.

  

To determine

The strains for an element oriented at $\theta =30°$.

Answer to Problem 7.7.17P

The normal strain in x direction is $-7.56\times {10}^{-4}$.

The normal strain along y direction is $2.671\times {10}^{-4}$.

The shear strain is $8.68\times {10}^{-4}$in x-y plane.

Explanation of Solution

Given:

Stress along x direction is $-8400\text{psi}$, the stress along y direction is $1100\text{psi}$and the shear stress along x-y plane is $-1700\text{psi}$.

Write the Equation for strain along x axis

${\epsilon }_x=\frac{1}{E}\left({\sigma }_x-\nu {\sigma }_y\right)$(I)

Here, the normal strain along x direction is ${\epsilon }_x$, direct stress along x direction is ${\sigma }_x$and direct stress along y direction is ${\sigma }_y$Poissons ratio is $\nu$and Young modulus is $E$.

Write the Equation for strain along y axis

${\epsilon }_y=\frac{1}{E}\left({\sigma }_y-\nu {\sigma }_x\right)$(II)

Here, the normal strain along the y direction is ${\epsilon }_y$.

Write the expression for the shear strain ix-y plane

${\gamma }_{xy}=\frac{2{\tau }_{xy}\left(1+\nu \right)}{E}$(III)

Here, modulus of rigidity is $G$and the shear strain along x-y plane is ${\gamma }_{xy}$.

Write the expression for strain along x direction

${\epsilon }_{x_1}=\frac{{\epsilon }_x+{\epsilon }_y}{2}+\frac{{\epsilon }_x-{\epsilon }_y}{2}\cos 2\theta +\frac{{\gamma }_{xy}}{2}\sin 2\theta$(IV)

Write the expression for strain along y direction

${\epsilon }_{y_1}={\epsilon }_x+{\epsilon }_y-{\epsilon }_{x_1}$(V)

Write the expression for shear strain

$\frac{{\gamma }_{x_1{y}_1}}{2}=-\frac{{\epsilon }_x-{\epsilon }_y}{2}\sin 2\theta +\frac{{\gamma }_{xy}}{2}\cos 2\theta$(VI)

Here, the shear strain along x-y plane is ${\gamma }_{xy}$.

Calculation:

Substitute $-8400\text{psi}$for ${\sigma }_x$, $1100\text{psi}$for ${\sigma }_y$and $0.33$for $\nu$and $10\times {10}^7\text{psi}$for $E$in Equation (I).

$\begin{array}{c}{\epsilon }_x=\frac{-8400\text{psi}-\left(\left(0.33\right)\times 1100\text{psi}\right)}{10000\text{ksi}}\\ =\frac{1}{10000\text{ksi}\left(\frac{1000\text{psi}}{1\text{ksi}}\right)}\left(-8400\text{psi}-\left(\left(0.33\right)\times 1100\text{psi}\right)\right)\\ =\frac{-8763\text{psi}}{{10}^7\text{psi}}\\ =-8.763\times {10}^{-4}\end{array}$

Substitute $-8400\text{psi}$for ${\sigma }_x$, $1100\text{psi}$for ${\sigma }_y$and $0.33$for $\nu$and $10\times {10}^7\text{psi}$for $E$in Equation (II).

$\begin{array}{c}{\epsilon }_y=\frac{1100\text{psi}-\left(\left(0.33\right)\times -8400\text{psi}\right)}{10000\text{ksi}}\\ =\frac{1}{10000\text{ksi}\left(\frac{1000\text{psi}}{1\text{ksi}}\right)}\left(1100\text{psi}-\left(\left(0.33\right)\times -8400\text{psi}\right)\right)\\ =\frac{3972\text{psi}}{{10}^7\text{psi}}\\ =3.872\times {10}^{-4}\end{array}$

Substitute $-1700\text{psi}$for ${\tau }_{xy}$, $0.33$for $\nu$and $10\times {10}^7\text{psi}$for $E$in Equation (III).

$\begin{array}{c}{\gamma }_{xy}=\frac{2\left(-1700\text{psi}\right)\left(1+0.33\right)}{10000\text{ksi}}\\ =\frac{2\left(-1700\text{psi}\right)\left(1+0.33\right)}{10000\text{ksi}\left(\frac{1000\text{psi}}{1\text{ksi}}\right)}\\ =\frac{-4522\text{psi}}{{10}^7\text{psi}}\\ =-4.522\times {10}^{-4}\end{array}$

Substitute $-8.763\times {10}^{-4}$for ${\epsilon }_x$, $3.872\times {10}^{-4}$for ${\epsilon }_y$and $-4.522\times {10}^{-4}$for ${\gamma }_{xy}$in Equation (IV).

$\begin{array}{c}{\epsilon }_{x_1}=\left(\begin{array}{l}\frac{\left(-8.763\times {10}^{-4}+3.872\times {10}^{-4}\right)}{2}\\ +\frac{\left(-8.763\times {10}^{-4}-3.872\times {10}^{-4}\right)}{2}\cos \left(2\times \left(30°\right)\right)\\ +\frac{\left(-4.522\times {10}^{-4}\right)}{2}\sin \left(2\times \left(30°\right)\right)\end{array}\right)\\ {\epsilon }_{x_1}=\left(-2.445\times {10}^{-4}+3.159\times {10}^{-4}-1.958\times {10}^{-4}\right)\\ {\epsilon }_{x_1}=-7.56\times {10}^{-4}\end{array}$

Substitute $-8.763\times {10}^{-4}$for ${\epsilon }_x$, $3.872\times {10}^{-4}$for ${\epsilon }_y$and $-7.56\times {10}^{-4}$for ${\epsilon }_{x_1}$in Equation (V).

$\begin{array}{c}{\epsilon }_{y_1}=-8.763\times {10}^{-4}+3.872\times {10}^{-4}-\left(-7.56\times {10}^{-4}\right)\\ =-8.763\times {10}^{-4}+11.43\times {10}^{-4}\\ =2.671\times {10}^{-4}\end{array}$

Substitute $-8.763\times {10}^{-4}$for ${\epsilon }_x$, $3.872\times {10}^{-4}$for ${\epsilon }_y$and $-4.522\times {10}^{-4}$for ${\gamma }_{xy}$in Equation (VI).

$\begin{array}{l}\frac{{\gamma }_{x_1{y}_1}}{2}=-\left(\frac{\left(-8.763\times {10}^{-4}-3.872\times {10}^{-4}\right)}{2}\sin \left(2\times 30°\right)\right)+\left(\frac{-4.522\times {10}^{-4}}{2}\cos \left(2\times 30°\right)\right)\\ \frac{{\gamma }_{x_1{y}_1}}{2}=4.34\times {10}^{-4}\\ {\gamma }_{x_1{y}_1}=8.68\times {10}^{-4}\end{array}$In below figure the normal strains are shown.

    

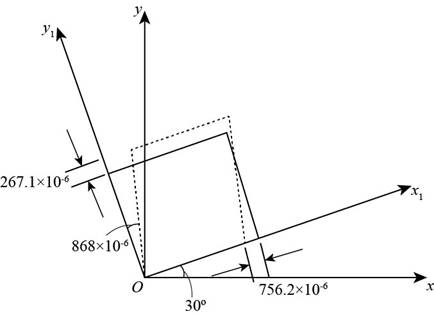
    Figure (1)

Conclusion:

The normal strain along x direction is $-7.56\times {10}^{-4}$.

The normal strain along y direction is $2.671\times {10}^{-4}$.

The shear strain along x-y plane is $8.68\times {10}^{-4}$.

To determine

The principal strains.

Answer to Problem 7.7.17P

The maximum principal strain is $-2.9886\times {10}^{-4}$, and the minimum principal strain is $-9.6464\times {10}^{-4}$.

Explanation of Solution

Write expression for the principal strains.

${\epsilon }_{1,2}=\left(\frac{{\epsilon }_x+{\epsilon }_y}{2}\right)+\sqrt{{\left(\frac{{\epsilon }_x-{\epsilon }_y}{2}\right)}^2+{\left(\frac{{\gamma }_{xy}}{2}\right)}^2}$(VII)

Here, the maximum principal strain is ${\epsilon }_1$and the minimum principal strain is ${\epsilon }_2$.

Write the expression for the principal angle.

$\tan 2{\theta }_p=\frac{{\gamma }_{xy}}{{\epsilon }_x-{\epsilon }_y}$(VIII)

Here, ${\theta }_p$is the principal angle.

Calculation:

Substitute $-8.763\times {10}^{-4}$for ${\epsilon }_x$, $3.872\times {10}^{-4}$for ${\epsilon }_y$and $-4.522\times {10}^{-4}$for ${\gamma }_{xy}$in Equation (VII).

$\begin{array}{c}{\epsilon }_{1,2}=\left(\frac{\left(-8.763\times {10}^{-4}+3.872\times {10}^{-4}\right)}{2}\right)\pm \\ \sqrt{{\left(\frac{\left(-8.763\times {10}^{-4}-3.8762\times {10}^{-4}\right)}{2}\right)}^2+{\left(\frac{-4.522\times {10}^{-4}}{2}\right)}^2}\\ =-2.445\times {10}^{-4}+\sqrt{39.937\times {10}^{-8}+5.112\times {10}^{-8}}\\ =-2.445\times {10}^{-4}\pm 6.709\times {10}^{-4}\end{array}$

While taking positive sign you get maximum principal stress.

$\begin{array}{c}{\epsilon }_1=-2.445\times {10}^{-4}+670.9\times {10}^{-4}\\ =4.264\times {10}^{-4}\end{array}$

While taking negative sign you get minimum principal stress.

$\begin{array}{c}{\epsilon }_2=-2.445\times {10}^{-4}-6.709\times {10}^{-4}\\ =-9.154\times {10}^{-4}\end{array}$

Substitute $-8.763\times {10}^{-4}$for ${\epsilon }_x$, $3.872\times {10}^{-4}$for ${\epsilon }_y$and $-4.522\times {10}^{-4}$for ${\gamma }_{xy}$in Equation (VIII).

$\begin{array}{c}\tan 2{\theta }_p=\frac{-4.522\times {10}^{-4}}{\left(-8.763\times {10}^{-4}-3.872\times {10}^{-4}\right)}\\ \tan 2{\theta }_p=0.35789\\ 2{\theta }_p=19.69°\\ {\theta }_p=9.85°\end{array}$

In below figure principal strains and principal angle are shown.

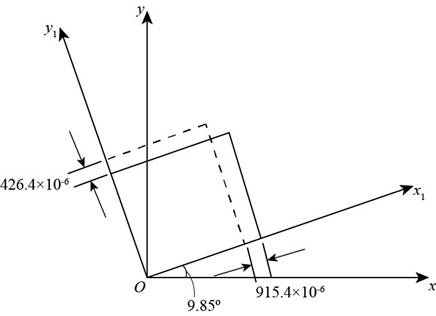    --> Figure (2)

Conclusion:

The maximum principal strain is $-2.9886\times {10}^{-4}$and minimum principal strain is $-9.6464\times {10}^{-4}$.

To determine

The maximum shear strain.

Answer to Problem 7.7.17P

The maximum shear strain is $13.42\times {10}^{-4}$.

Explanation of Solution

Write the expression for maximum shear strain,

$\frac{{\gamma }_{\max }}{2}=\sqrt{{\left(\frac{{\epsilon }_x-{\epsilon }_y}{2}\right)}^2+{\left(\frac{{\gamma }_{xy}}{2}\right)}^2}$(IX)

Here, ${\gamma }_{\max }$is the maximum shear strain.

Write expression for shear angle

${\theta }_{s_1}={\theta }_{p_1}-45°$ (X)

Here, ${\theta }_{s_1}$is the shear angle.

Write the Equation for average strain

${\epsilon }_{avg}=\frac{{\epsilon }_x+{\epsilon }_y}{2}$(XI)

Write expression for maximum shear strain.

${\gamma }_{\min }=-{\gamma }_{\max }$ (XII)

Calculation:

Substitute $-8.763\times {10}^{-4}$for ${\epsilon }_x$, $3.872\times {10}^{-4}$for ${\epsilon }_y$and $-4.522\times {10}^{-4}$for ${\gamma }_{xy}$in Equation (IX).

$\begin{array}{c}\frac{{\gamma }_{\max }}{2}=\sqrt{{\left(\frac{\left(-8.763\times {10}^{-4}-3.872\times {10}^{-4}\right)}{2}\right)}^2+{\left(\frac{-4.522\times {10}^{-4}}{2}\right)}^2}\\ \frac{{\gamma }_{\max }}{2}=\sqrt{{\left(-6.3175\times {10}^{-4}\right)}^2+{\left(-2.261\times {10}^{-4}\right)}^2}\\ \frac{{\gamma }_{\max }}{2}=\sqrt{45.023\times {10}^{-8}}\\ \frac{{\gamma }_{\max }}{2}=6.71\times {10}^{-4}\end{array}$

${\gamma }_{\max }=13.42\times {10}^{-4}$

Substitute $9.84°$for ${\theta }_{p_1}$in Equation (X).

$\begin{array}{c}{\theta }_{s_1}=9.84°-45°\\ =-35.16°\end{array}$

Substitute $-8.763\times {10}^{-4}$for ${\epsilon }_x$, $3.872\times {10}^{-4}$for ${\epsilon }_y$in Equation (XI).

$\begin{array}{c}{\epsilon }_{avg}=\frac{-8.763\times {10}^{-4}+3.872\times {10}^{-4}}{2}\\ =-2.44\times {10}^{-4}\end{array}$

Substitute $13.42\times {10}^{-4}$for ${\gamma }_{\max }$.in Equation (XII).

${\gamma }_{\min }=-{\gamma }_{\max }=-13.42\times {10}^{-4}$

In below figure average strain and minimum strain are shown.

    

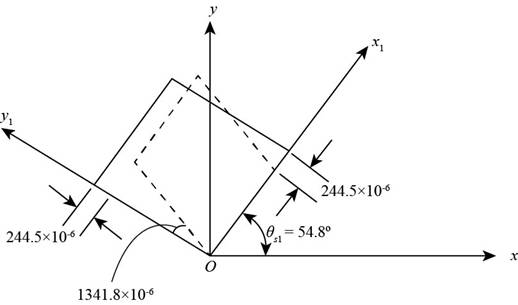
    Figure-(3)

Conclusion:

The maximum shear strain is $13.42\times {10}^{-4}$.