High School Math 2015 Common Core Algebra 1 Student Edition Grade 8/9
15th Edition
ISBN: 9780133281149
Author: Prentice Hall
Publisher: Prentice Hall
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Question
Chapter 5.1, Problem 1MP
a.
To determine
To solve: the rate of change in the water height so the number of marbles.
a.
Expert Solution
Answer to Problem 1MP
Rate of change is 0.3 cm in water level per marble addition
Explanation of Solution
Given Information
A table has been given, representing number of marbles & its corresponding height of rise in water level.
We have to find out the respective rate of change in the water height so the number of marbles, water level rises for each marble and also type of relation in terms of function between number of marbles and water height.
Calculation
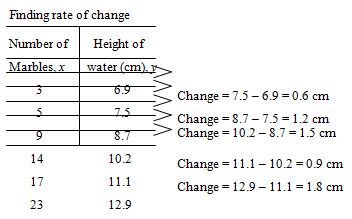
We can clearly observe that, when there were 3 marbles, water level was at 6.9 cm.
On subsequent addition of 2 more marbles, water level rose by 0.6 cm and so on.
Conclusion
We notice that, it is a case of linear relation function and a discrete rise in level is observed on subsequent addition of marbles.
b.
To determine
To solve: the water level rise for each marble
b.
Expert Solution
Answer to Problem 1MP
0.3 cm.
Explanation of Solution
Given Information
A table has been given, representing number of marbles & its corresponding height of rise in water level.
We have to find out the respective rate of change in the water height so the number of marbles, water level rises for each marble and also type of relation in terms of function between number of marbles and water height.
Calculation From the table we clearly notice that,
(i)Addition of further 2 marbles, defined a change in height of 0.6 cm
(ii)Addition of further 4 marbles, a change of 1.2 cm is observed.
Similarly, for 5 marbles ( 1.5
for 3 marbles ( 0.9
for 6 marbles ( 1.8
So, in each case rise of water level per marble will be
Conclusion Therefore, for each subsequent marble, rise in water level is by 0.3 cm.
c.
To determine
To solve: the type of relation in terms of function between number of marbles and water height.
c.
Expert Solution
Answer to Problem 1MP
Linear relationship
Explanation of Solution
Given Information
A table has been given, representing number of marbles & its corresponding height of rise in water level.
We have to find out the respective rate of change in the water height so the number of marbles, water level rises for each marble and also type of relation in terms of function between number of marbles and water height.
Calculation
Establishing the relationship between number of marbles and water height
where,
y = 6 + 0. 3x
y = height of water (in cm)
x = number of marbles
So, for, x = 0; y = 6 cm which signifies that, initially with no marble, the height of water was 6 cm.
For, x = 3
y = 6 (0.3 × 3)
y = 6 + 0.9 = 6.9 cm
which matches the given table.
Conclusion
Hence, our relation, y = 6 + 0. 3x is verified, and it clearly shows a linear relationship.
Chapter 5 Solutions
High School Math 2015 Common Core Algebra 1 Student Edition Grade 8/9
Ch. 5.1 - Prob. 1PCh. 5.1 - Prob. 2PCh. 5.1 - Prob. 3PCh. 5.1 - Prob. 4PCh. 5.1 - Prob. 1LCCh. 5.1 - Prob. 2LCCh. 5.1 - Prob. 3LCCh. 5.1 - Prob. 4LCCh. 5.1 - Prob. 5LCCh. 5.1 - Prob. 6LC
Ch. 5.1 - Prob. 7LCCh. 5.1 - Prob. 8PPECh. 5.1 - Prob. 9PPECh. 5.1 - Prob. 10PPECh. 5.1 - Prob. 11PPECh. 5.1 - Prob. 12PPECh. 5.1 - Prob. 13PPECh. 5.1 - Prob. 14PPECh. 5.1 - Prob. 15PPECh. 5.1 - Prob. 16PPECh. 5.1 - Prob. 17PPECh. 5.1 - Prob. 18PPECh. 5.1 - Prob. 19PPECh. 5.1 - Prob. 20PPECh. 5.1 - Prob. 21PPECh. 5.1 - Prob. 22PPECh. 5.1 - Prob. 23PPECh. 5.1 - Prob. 24PPECh. 5.1 - Prob. 25PPECh. 5.1 - Prob. 26PPECh. 5.1 - Prob. 27PPECh. 5.1 - Prob. 28PPECh. 5.1 - Prob. 29PPECh. 5.1 - Prob. 30PPECh. 5.1 - Prob. 31PPECh. 5.1 - Prob. 32PPECh. 5.1 - Prob. 33PPECh. 5.1 - Prob. 34PPECh. 5.1 - Prob. 35PPECh. 5.1 - Prob. 36PPECh. 5.1 - Prob. 37PPECh. 5.1 - Prob. 38PPECh. 5.1 - Prob. 39PPECh. 5.1 - Prob. 40PPECh. 5.1 - Prob. 41PPECh. 5.1 - Prob. 42PPECh. 5.1 - Prob. 43PPECh. 5.1 - Prob. 44PPECh. 5.1 - Prob. 45PPECh. 5.1 - Prob. 46PPECh. 5.1 - Prob. 47PPECh. 5.1 - Prob. 48PPECh. 5.1 - Prob. 49PPECh. 5.1 - Prob. 50PPECh. 5.1 - Prob. 51PPECh. 5.1 - Prob. 52PPECh. 5.1 - Prob. 53PPECh. 5.1 - Prob. 54PPECh. 5.1 - Prob. 55PPECh. 5.1 - Prob. 56PPECh. 5.1 - Prob. 57PPECh. 5.1 - Prob. 58PPECh. 5.1 - Prob. 1MPCh. 5.2 - Prob. 1PCh. 5.2 - Prob. 2PCh. 5.2 - Prob. 3PCh. 5.2 - Prob. 4PCh. 5.2 - Prob. 1LCCh. 5.2 - Prob. 2LCCh. 5.2 - Prob. 3LCCh. 5.2 - Prob. 4LCCh. 5.2 - Prob. 5LCCh. 5.2 - Prob. 6LCCh. 5.2 - Prob. 7LCCh. 5.2 - Prob. 8LCCh. 5.2 - Prob. 9PPECh. 5.2 - Prob. 10PPECh. 5.2 - Prob. 11PPECh. 5.2 - Prob. 12PPECh. 5.2 - Prob. 13PPECh. 5.2 - Prob. 14PPECh. 5.2 - Prob. 15PPECh. 5.2 - Prob. 16PPECh. 5.2 - Prob. 17PPECh. 5.2 - Prob. 18PPECh. 5.2 - Prob. 19PPECh. 5.2 - Prob. 20PPECh. 5.2 - Prob. 21PPECh. 5.2 - Prob. 22PPECh. 5.2 - Prob. 23PPECh. 5.2 - Prob. 24PPECh. 5.2 - Prob. 25PPECh. 5.2 - Prob. 26PPECh. 5.2 - Prob. 27PPECh. 5.2 - Prob. 28PPECh. 5.2 - Prob. 29PPECh. 5.2 - Prob. 30PPECh. 5.2 - Prob. 31PPECh. 5.2 - Prob. 32PPECh. 5.2 - Prob. 33PPECh. 5.2 - Prob. 34PPECh. 5.2 - Prob. 35PPECh. 5.2 - Prob. 36PPECh. 5.2 - Prob. 37PPECh. 5.2 - Prob. 38PPECh. 5.2 - Prob. 39PPECh. 5.2 - Prob. 40PPECh. 5.2 - Prob. 41PPECh. 5.2 - Prob. 42PPECh. 5.2 - Prob. 43PPECh. 5.2 - Prob. 44PPECh. 5.2 - Prob. 45PPECh. 5.2 - Prob. 46PPECh. 5.2 - Prob. 47PPECh. 5.2 - Prob. 48STPCh. 5.2 - Prob. 49STPCh. 5.2 - Prob. 50STPCh. 5.2 - Prob. 51STPCh. 5.2 - Prob. 52MRCh. 5.2 - Prob. 53MRCh. 5.2 - Prob. 54MRCh. 5.2 - Prob. 55MRCh. 5.2 - Prob. 56MRCh. 5.2 - Prob. 57MRCh. 5.2 - Prob. 58MRCh. 5.2 - Prob. 59MRCh. 5.3 - Prob. 1CBCh. 5.3 - Prob. 2CBCh. 5.3 - Prob. 3CBCh. 5.3 - Prob. 4CBCh. 5.3 - Prob. 5CBCh. 5.3 - Prob. 6CBCh. 5.3 - Prob. 7CBCh. 5.3 - Prob. 1PCh. 5.3 - Prob. 2PCh. 5.3 - Prob. 3PCh. 5.3 - Prob. 4PCh. 5.3 - Prob. 5PCh. 5.3 - Prob. 6PCh. 5.3 - Prob. 1LCCh. 5.3 - Prob. 2LCCh. 5.3 - Prob. 3LCCh. 5.3 - Prob. 4LCCh. 5.3 - Prob. 5LCCh. 5.3 - Prob. 6LCCh. 5.3 - Prob. 7PPECh. 5.3 - Prob. 8PPECh. 5.3 - Prob. 9PPECh. 5.3 - Prob. 10PPECh. 5.3 - Prob. 11PPECh. 5.3 - Prob. 12PPECh. 5.3 - Prob. 13PPECh. 5.3 - Prob. 14PPECh. 5.3 - Prob. 15PPECh. 5.3 - Prob. 16PPECh. 5.3 - Prob. 17PPECh. 5.3 - Prob. 18PPECh. 5.3 - Prob. 19PPECh. 5.3 - Prob. 20PPECh. 5.3 - Prob. 21PPECh. 5.3 - Prob. 22PPECh. 5.3 - Prob. 23PPECh. 5.3 - Prob. 24PPECh. 5.3 - Prob. 25PPECh. 5.3 - Prob. 26PPECh. 5.3 - Prob. 27PPECh. 5.3 - Prob. 28PPECh. 5.3 - Prob. 29PPECh. 5.3 - Prob. 30PPECh. 5.3 - Prob. 31PPECh. 5.3 - Prob. 32PPECh. 5.3 - Prob. 33PPECh. 5.3 - Prob. 34PPECh. 5.3 - Prob. 35PPECh. 5.3 - Prob. 36PPECh. 5.3 - Prob. 37PPECh. 5.3 - Prob. 38PPECh. 5.3 - Prob. 39PPECh. 5.3 - Prob. 40PPECh. 5.3 - Prob. 41PPECh. 5.3 - Prob. 42PPECh. 5.3 - Prob. 43PPECh. 5.3 - Prob. 44PPECh. 5.3 - Prob. 45PPECh. 5.3 - Prob. 46PPECh. 5.3 - Prob. 47PPECh. 5.3 - Prob. 48PPECh. 5.3 - Prob. 49PPECh. 5.3 - Prob. 50PPECh. 5.3 - Prob. 51PPECh. 5.3 - Prob. 52PPECh. 5.3 - Prob. 53PPECh. 5.3 - Prob. 54PPECh. 5.3 - Prob. 55PPECh. 5.3 - Prob. 56PPECh. 5.3 - Prob. 57PPECh. 5.3 - Prob. 58PPECh. 5.3 - Prob. 59PPECh. 5.3 - Prob. 60PPECh. 5.3 - Prob. 61PPECh. 5.3 - Prob. 62PPECh. 5.3 - Prob. 63STPCh. 5.3 - Prob. 64STPCh. 5.3 - Prob. 65STPCh. 5.3 - Prob. 66STPCh. 5.3 - Prob. 67STPCh. 5.3 - Prob. 68MRCh. 5.3 - Prob. 69MRCh. 5.3 - Prob. 70MRCh. 5.3 - Prob. 71MRCh. 5.3 - Prob. 72MRCh. 5.3 - Prob. 73MRCh. 5.3 - Prob. 74MRCh. 5.3 - Prob. 75MRCh. 5.3 - Prob. 76MRCh. 5.3 - Prob. 77MRCh. 5.4 - Prob. 1PCh. 5.4 - Prob. 2PCh. 5.4 - Prob. 3PCh. 5.4 - Prob. 4PCh. 5.4 - Prob. 1LCCh. 5.4 - Prob. 2LCCh. 5.4 - Prob. 3LCCh. 5.4 - Prob. 4LCCh. 5.4 - Prob. 5LCCh. 5.4 - Prob. 6LCCh. 5.4 - Prob. 7LCCh. 5.4 - Prob. 8PPECh. 5.4 - Prob. 9PPECh. 5.4 - Prob. 10PPECh. 5.4 - Prob. 11PPECh. 5.4 - Prob. 12PPECh. 5.4 - Prob. 13PPECh. 5.4 - Prob. 14PPECh. 5.4 - Prob. 15PPECh. 5.4 - Prob. 16PPECh. 5.4 - Prob. 17PPECh. 5.4 - Prob. 18PPECh. 5.4 - Prob. 19PPECh. 5.4 - Prob. 20PPECh. 5.4 - Prob. 21PPECh. 5.4 - Prob. 22PPECh. 5.4 - Prob. 23PPECh. 5.4 - Prob. 24PPECh. 5.4 - Prob. 25PPECh. 5.4 - Prob. 26PPECh. 5.4 - Prob. 27PPECh. 5.4 - Prob. 28PPECh. 5.4 - Prob. 29PPECh. 5.4 - Prob. 30PPECh. 5.4 - Prob. 31PPECh. 5.4 - Prob. 32PPECh. 5.4 - Prob. 1MPCh. 5.4 - Prob. 1MCQCh. 5.4 - Prob. 2MCQCh. 5.4 - Prob. 3MCQCh. 5.4 - Prob. 4MCQCh. 5.4 - Prob. 5MCQCh. 5.4 - Prob. 6MCQCh. 5.4 - Prob. 7MCQCh. 5.4 - Prob. 8MCQCh. 5.4 - Prob. 9MCQCh. 5.4 - Prob. 10MCQCh. 5.4 - Prob. 11MCQCh. 5.4 - Prob. 12MCQCh. 5.4 - Prob. 13MCQCh. 5.4 - Prob. 14MCQCh. 5.4 - Prob. 15MCQCh. 5.4 - Prob. 16MCQCh. 5.4 - Prob. 17MCQCh. 5.4 - Prob. 18MCQCh. 5.4 - Prob. 19MCQCh. 5.4 - Prob. 20MCQCh. 5.4 - Prob. 21MCQCh. 5.4 - Prob. 22MCQCh. 5.4 - Prob. 23MCQCh. 5.4 - Prob. 24MCQCh. 5.4 - Prob. 25MCQCh. 5.4 - Prob. 26MCQCh. 5.5 - Prob. 1PCh. 5.5 - Prob. 2PCh. 5.5 - Prob. 3PCh. 5.5 - Prob. 4PCh. 5.5 - Prob. 5PCh. 5.5 - Prob. 1LCCh. 5.5 - Prob. 2LCCh. 5.5 - Prob. 3LCCh. 5.5 - Prob. 4LCCh. 5.5 - Prob. 5LCCh. 5.5 - Prob. 6LCCh. 5.5 - Prob. 7LCCh. 5.5 - Prob. 8PPECh. 5.5 - Prob. 9PPECh. 5.5 - Prob. 10PPECh. 5.5 - Prob. 11PPECh. 5.5 - Prob. 12PPECh. 5.5 - Prob. 13PPECh. 5.5 - Prob. 14PPECh. 5.5 - Prob. 15PPECh. 5.5 - Prob. 16PPECh. 5.5 - Prob. 17PPECh. 5.5 - Prob. 18PPECh. 5.5 - Prob. 19PPECh. 5.5 - Prob. 20PPECh. 5.5 - Prob. 21PPECh. 5.5 - Prob. 22PPECh. 5.5 - Prob. 23PPECh. 5.5 - Prob. 24PPECh. 5.5 - Prob. 25PPECh. 5.5 - Prob. 26PPECh. 5.5 - Prob. 27PPECh. 5.5 - Prob. 28PPECh. 5.5 - Prob. 29PPECh. 5.5 - Prob. 30PPECh. 5.5 - Prob. 31PPECh. 5.5 - Prob. 32PPECh. 5.5 - Prob. 33PPECh. 5.5 - Prob. 34PPECh. 5.5 - Prob. 35PPECh. 5.5 - Prob. 36PPECh. 5.5 - Prob. 37PPECh. 5.5 - Prob. 38PPECh. 5.5 - Prob. 39PPECh. 5.5 - Prob. 40PPECh. 5.5 - Prob. 41PPECh. 5.5 - Prob. 42PPECh. 5.5 - Prob. 43PPECh. 5.5 - Prob. 44PPECh. 5.5 - Prob. 45PPECh. 5.5 - Prob. 46PPECh. 5.5 - Prob. 47PPECh. 5.5 - Prob. 48PPECh. 5.5 - Prob. 49PPECh. 5.5 - Prob. 50PPECh. 5.5 - Prob. 51PPECh. 5.5 - Prob. 52PPECh. 5.5 - Prob. 53PPECh. 5.5 - Prob. 54PPECh. 5.5 - Prob. 55PPECh. 5.5 - Prob. 56PPECh. 5.5 - Prob. 57PPECh. 5.5 - Prob. 58PPECh. 5.5 - Prob. 59PPECh. 5.5 - Prob. 60PPECh. 5.5 - Prob. 61PPECh. 5.5 - Prob. 62PPECh. 5.5 - Prob. 63PPECh. 5.5 - Prob. 64STPCh. 5.5 - Prob. 65STPCh. 5.5 - Prob. 66STPCh. 5.5 - Prob. 67STPCh. 5.5 - Prob. 68STPCh. 5.5 - Prob. 69MRCh. 5.5 - Prob. 70MRCh. 5.5 - Prob. 71MRCh. 5.5 - Prob. 72MRCh. 5.5 - Prob. 73MRCh. 5.5 - Prob. 74MRCh. 5.5 - Prob. 75MRCh. 5.5 - Prob. 76MRCh. 5.5 - Prob. 77MRCh. 5.5 - Prob. 1CBCh. 5.5 - Prob. 2CBCh. 5.5 - Prob. 3CBCh. 5.5 - Prob. 4CBCh. 5.5 - Prob. 5CBCh. 5.5 - Prob. 6CBCh. 5.5 - Prob. 7CBCh. 5.5 - Prob. 8CBCh. 5.5 - Prob. 9CBCh. 5.5 - Prob. 10CBCh. 5.5 - Prob. 11CBCh. 5.5 - Prob. 12CBCh. 5.5 - Prob. 13CBCh. 5.5 - Prob. 14CBCh. 5.5 - Prob. 15CBCh. 5.5 - Prob. 16CBCh. 5.5 - Prob. 17CBCh. 5.5 - Prob. 18CBCh. 5.5 - Prob. 19CBCh. 5.6 - Prob. 1PCh. 5.6 - Prob. 2PCh. 5.6 - Prob. 3PCh. 5.6 - Prob. 4PCh. 5.6 - Prob. 1LCCh. 5.6 - Prob. 2LCCh. 5.6 - Prob. 3LCCh. 5.6 - Prob. 4LCCh. 5.6 - Prob. 5LCCh. 5.6 - Prob. 6LCCh. 5.6 - Prob. 7PPECh. 5.6 - Prob. 8PPECh. 5.6 - Prob. 9PPECh. 5.6 - Prob. 10PPECh. 5.6 - Prob. 11PPECh. 5.6 - Prob. 12PPECh. 5.6 - Prob. 13PPECh. 5.6 - Prob. 14PPECh. 5.6 - Prob. 15PPECh. 5.6 - Prob. 16PPECh. 5.6 - Prob. 17PPECh. 5.6 - Prob. 18PPECh. 5.6 - Prob. 19PPECh. 5.6 - Prob. 20PPECh. 5.6 - Prob. 21PPECh. 5.6 - Prob. 22PPECh. 5.6 - Prob. 23PPECh. 5.6 - Prob. 24PPECh. 5.6 - Prob. 25PPECh. 5.6 - Prob. 26PPECh. 5.6 - Prob. 27PPECh. 5.6 - Prob. 28PPECh. 5.6 - Prob. 29PPECh. 5.6 - Prob. 30PPECh. 5.6 - Prob. 31PPECh. 5.6 - Prob. 32PPECh. 5.6 - Prob. 33PPECh. 5.6 - Prob. 34PPECh. 5.6 - Prob. 35PPECh. 5.6 - Prob. 36PPECh. 5.6 - Prob. 37PPECh. 5.6 - Prob. 38STPCh. 5.6 - Prob. 39STPCh. 5.6 - Prob. 40STPCh. 5.6 - Prob. 41MRCh. 5.6 - Prob. 42MRCh. 5.6 - Prob. 43MRCh. 5.6 - Prob. 44MRCh. 5.6 - Prob. 45MRCh. 5.6 - Prob. 46MRCh. 5.6 - Prob. 47MRCh. 5.7 - Prob. 1PCh. 5.7 - Prob. 2PCh. 5.7 - Prob. 3PCh. 5.7 - Prob. 4PCh. 5.7 - Prob. 1LCCh. 5.7 - Prob. 2LCCh. 5.7 - Prob. 3LCCh. 5.7 - Prob. 4LCCh. 5.7 - Prob. 5LCCh. 5.7 - Prob. 6LCCh. 5.7 - Prob. 7PPECh. 5.7 - Prob. 8PPECh. 5.7 - Prob. 9PPECh. 5.7 - Prob. 10PPECh. 5.7 - Prob. 11PPECh. 5.7 - Prob. 12PPECh. 5.7 - Prob. 13PPECh. 5.7 - Prob. 14PPECh. 5.7 - Prob. 15PPECh. 5.7 - Prob. 16PPECh. 5.7 - Prob. 17PPECh. 5.7 - Prob. 18PPECh. 5.7 - Prob. 19PPECh. 5.7 - Prob. 20PPECh. 5.7 - Prob. 21PPECh. 5.7 - Prob. 22PPECh. 5.7 - Prob. 23STPCh. 5.7 - Prob. 24STPCh. 5.7 - Prob. 25STPCh. 5.7 - Prob. 26STPCh. 5.7 - Prob. 27MRCh. 5.7 - Prob. 28MRCh. 5.7 - Prob. 29MRCh. 5.7 - Prob. 30MRCh. 5.7 - Prob. 31MRCh. 5.7 - Prob. 32MRCh. 5.7 - Prob. 33MRCh. 5.7 - Prob. 5CBCh. 5.7 - Prob. 6CBCh. 5.8 - Prob. 1PCh. 5.8 - Prob. 2PCh. 5.8 - Prob. 3PCh. 5.8 - Prob. 4PCh. 5.8 - Prob. 1LCCh. 5.8 - Prob. 2LCCh. 5.8 - Prob. 3LCCh. 5.8 - Prob. 4LCCh. 5.8 - Prob. 5LCCh. 5.8 - Prob. 6PPECh. 5.8 - Prob. 7PPECh. 5.8 - Prob. 8PPECh. 5.8 - Prob. 9PPECh. 5.8 - Prob. 10PPECh. 5.8 - Prob. 11PPECh. 5.8 - Prob. 12PPECh. 5.8 - Prob. 13PPECh. 5.8 - Prob. 14PPECh. 5.8 - Prob. 15PPECh. 5.8 - Prob. 16PPECh. 5.8 - Prob. 17PPECh. 5.8 - Prob. 18PPECh. 5.8 - Prob. 19PPECh. 5.8 - Prob. 20PPECh. 5.8 - Prob. 21PPECh. 5.8 - Prob. 22PPECh. 5.8 - Prob. 23PPECh. 5.8 - Prob. 24PPECh. 5.8 - Prob. 25PPECh. 5.8 - Prob. 26PPECh. 5.8 - Prob. 27PPECh. 5.8 - Prob. 28PPECh. 5.8 - Prob. 29PPECh. 5.8 - Prob. 30PPECh. 5.8 - Prob. 31PPECh. 5.8 - Prob. 32PPECh. 5.8 - Prob. 33PPECh. 5.8 - Prob. 34PPECh. 5.8 - Prob. 35PPECh. 5.8 - Prob. 36PPECh. 5.8 - Prob. 37PPECh. 5.8 - Prob. 38STPCh. 5.8 - Prob. 39STPCh. 5.8 - Prob. 40STPCh. 5.8 - Prob. 41STPCh. 5.8 - Prob. 42MRCh. 5.8 - Prob. 43MRCh. 5.8 - Prob. 44MRCh. 5.8 - Prob. 45MRCh. 5.8 - Prob. 46MRCh. 5.8 - Prob. 47MRCh. 5.8 - Prob. 1CBCh. 5.8 - Prob. 2CBCh. 5 - Prob. 1GRCh. 5 - Prob. 2GRCh. 5 - Prob. 3GRCh. 5 - Prob. 4GRCh. 5 - Prob. 5GRCh. 5 - Prob. 6GRCh. 5 - Prob. 7GRCh. 5 - Prob. 8GRCh. 5 - Prob. 9GRCh. 5 - Prob. 10GRCh. 5 - Prob. 11GRCh. 5 - Prob. 12GRCh. 5 - Prob. 13GRCh. 5 - Prob. 14GRCh. 5 - Prob. 15GRCh. 5 - Prob. 16GRCh. 5 - Prob. 17GRCh. 5 - Prob. 1CRCh. 5 - Prob. 2CRCh. 5 - Prob. 3CRCh. 5 - Prob. 4CRCh. 5 - Prob. 5CRCh. 5 - Prob. 6CRCh. 5 - Prob. 7CRCh. 5 - Prob. 8CRCh. 5 - Prob. 9CRCh. 5 - Prob. 10CRCh. 5 - Prob. 11CRCh. 5 - Prob. 12CRCh. 5 - Prob. 13CRCh. 5 - Prob. 14CRCh. 5 - Prob. 15CRCh. 5 - Prob. 16CRCh. 5 - Prob. 17CRCh. 5 - Prob. 18CRCh. 5 - Prob. 19CRCh. 5 - Prob. 20CRCh. 5 - Prob. 21CRCh. 5 - Prob. 22CRCh. 5 - Prob. 23CRCh. 5 - Prob. 24CRCh. 5 - Prob. 25CRCh. 5 - Prob. 26CRCh. 5 - Prob. 27CRCh. 5 - Prob. 28CRCh. 5 - Prob. 29CRCh. 5 - Prob. 30CRCh. 5 - Prob. 31CRCh. 5 - Prob. 32CRCh. 5 - Prob. 33CRCh. 5 - Prob. 34CRCh. 5 - Prob. 35CRCh. 5 - Prob. 36CRCh. 5 - Prob. 37CRCh. 5 - Prob. 38CRCh. 5 - Prob. 39CRCh. 5 - Prob. 40CRCh. 5 - Prob. 1CTCh. 5 - Prob. 2CTCh. 5 - Prob. 3CTCh. 5 - Prob. 4CTCh. 5 - Prob. 5CTCh. 5 - Prob. 6CTCh. 5 - Prob. 7CTCh. 5 - Prob. 8CTCh. 5 - Prob. 9CTCh. 5 - Prob. 10CTCh. 5 - Prob. 11CTCh. 5 - Prob. 12CTCh. 5 - Prob. 13CTCh. 5 - Prob. 14CTCh. 5 - Prob. 15CTCh. 5 - Prob. 16CTCh. 5 - Prob. 17CTCh. 5 - Prob. 18CTCh. 5 - Prob. 19CTCh. 5 - Prob. 20CTCh. 5 - Prob. 21CTCh. 5 - Prob. 22CTCh. 5 - Prob. 23CTCh. 5 - Prob. 1CCSRCh. 5 - Prob. 2CCSRCh. 5 - Prob. 3CCSRCh. 5 - Prob. 4CCSRCh. 5 - Prob. 5CCSRCh. 5 - Prob. 6CCSRCh. 5 - Prob. 7CCSRCh. 5 - Prob. 8CCSRCh. 5 - Prob. 9CCSRCh. 5 - Prob. 10CCSRCh. 5 - Prob. 11CCSRCh. 5 - Prob. 12CCSRCh. 5 - Prob. 13CCSRCh. 5 - Prob. 14CCSRCh. 5 - Prob. 15CCSRCh. 5 - Prob. 16CCSRCh. 5 - Prob. 17CCSRCh. 5 - Prob. 18CCSRCh. 5 - Prob. 19CCSRCh. 5 - Prob. 20CCSRCh. 5 - Prob. 21CCSRCh. 5 - Prob. 22CCSRCh. 5 - Prob. 23CCSRCh. 5 - Prob. 24CCSRCh. 5 - Prob. 25CCSRCh. 5 - Prob. 26CCSRCh. 5 - Prob. 27CCSRCh. 5 - Prob. 28CCSRCh. 5 - Prob. 29CCSRCh. 5 - Prob. 30CCSRCh. 5 - Prob. 31CCSRCh. 5 - Prob. 32CCSR
Additional Math Textbook Solutions
Find more solutions based on key concepts
z Scores. In Exercises 5-8, express all z scores with two decimal places.
8. Plastic Waste Data Set 31 “Garbage...
Elementary Statistics (13th Edition)
The following set of data is from sample of n=5: a. Compute the mean, median, and mode. b. Compute the range, v...
Basic Business Statistics, Student Value Edition
Fill in each blank so that the resulting statement is true. An equation that expresses a relationship between t...
Algebra and Trigonometry (6th Edition)
Assessment 1-1A In a big red box, there are 7 smaller blue boxes. In each of the blue boxes, there are 7 black ...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
The four flaws in the given survey.
Elementary Statistics
To simplify the expression −3(a+2)−a
Pre-Algebra Student Edition
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- (6) ≤ a) Determine the following groups: Homz(Q, Z), Homz(Q, Q), Homz(Q/Z, Z) for n E N. Homz(Z/nZ, Q) b) Show for ME MR: HomR (R, M) = M.arrow_forward1. If f(x² + 1) = x + 5x² + 3, what is f(x² - 1)?arrow_forward2. What is the total length of the shortest path that goes from (0,4) to a point on the x-axis, then to a point on the line y = 6, then to (18.4)?arrow_forward
- موضوع الدرس Prove that Determine the following groups Homz(QZ) Hom = (Q13,Z) Homz(Q), Hom/z/nZ, Qt for neN- (2) Every factor group of adivisible group is divisble. • If R is a Skew ficald (aring with identity and each non Zero element is invertible then every R-module is free.arrow_forwardI have ai answers but incorrectarrow_forwardwhat is the slope of the linear equation-5x+2y-10=0arrow_forward
- ************* ********************************* Q.1) Classify the following statements as a true or false statements: a. If M is a module, then every proper submodule of M is contained in a maximal submodule of M. b. The sum of a finite family of small submodules of a module M is small in M. c. Zz is directly indecomposable. d. An epimorphism a: M→ N is called solit iff Ker(a) is a direct summand in M. e. The Z-module has two composition series. Z 6Z f. Zz does not have a composition series. g. Any finitely generated module is a free module. h. If O→A MW→ 0 is short exact sequence then f is epimorphism. i. If f is a homomorphism then f-1 is also a homomorphism. Maximal C≤A if and only if is simple. Sup Q.4) Give an example and explain your claim in each case: Monomorphism not split. b) A finite free module. c) Semisimple module. d) A small submodule A of a module N and a homomorphism op: MN, but (A) is not small in M.arrow_forwardI need diagram with solutionsarrow_forwardT. Determine the least common denominator and the domain for the 2x-3 10 problem: + x²+6x+8 x²+x-12 3 2x 2. Add: + Simplify and 5x+10 x²-2x-8 state the domain. 7 3. Add/Subtract: x+2 1 + x+6 2x+2 4 Simplify and state the domain. x+1 4 4. Subtract: - Simplify 3x-3 x²-3x+2 and state the domain. 1 15 3x-5 5. Add/Subtract: + 2 2x-14 x²-7x Simplify and state the domain.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON
Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON
Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education
Differential Equation | MIT 18.01SC Single Variable Calculus, Fall 2010; Author: MIT OpenCourseWare;https://www.youtube.com/watch?v=HaOHUfymsuk;License: Standard YouTube License, CC-BY