Fox and McDonald's Introduction to Fluid Mechanics
9th Edition
ISBN: 9781118912652
Author: Philip J. Pritchard, John W. Mitchell
Publisher: WILEY
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 5, Problem 48P
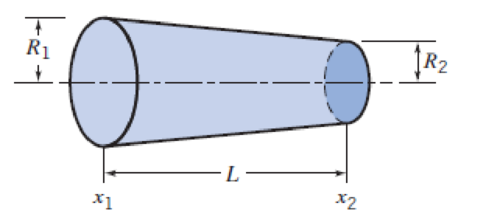
Consider the one-dimensional, incompressible flow through the circular channel shown. The velocity at section ① is given by U = U0 + U1 sin ωt, where U0 = 20 m/s, U1 = 2 m/s, and ω = 0.3 rad/s. The channel dimensions are L = 1 m, R1 = 0.2 m, and R2 = 0.1 m. Determine the particle acceleration at the channel exit. Plot the results as a function of time over a complete cycle. On the same plot, show the acceleration at the channel exit if the channel is constant area, rather than convergent, and explain the difference between the curves.
P5.48
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Flow through a converging nozzle can be approximated by a one-dimensional velocity
distribution u=vo (1+2). For the nozzle shown below, assume that the velocity varies
linearly from u = vo at the entrance to u = 3v, at the exit. Compute the acceleration at the
entrance and exit if vo=10m/s and L = 1m.
x=0
X
u=
:326
x=L
B/ Two components
of velocity in an incompressible fluid flow are given by
v=z³y³ determine the third component.
u= x² - y³
and
Do the velocity field U = 5xi + (3y + ty2)j represent physically possible flow?
1. For a flow in the xy-plane, the y-component of velocity is given by v = y2 −2x+ 2y. Find a possible x-component for steady, incompressible flow. Is it also valid for unsteady, incompressible flow? Why? 2. The x-component of velocity in a steady, incompressible flow field in the xy-plane is u = A/x. Find the simplest y-component of velocity for this flow field.
Chapter 5 Solutions
Fox and McDonald's Introduction to Fluid Mechanics
Ch. 5 - Which of the following sets of equations represent...Ch. 5 - Which of the following sets of equations represent...Ch. 5 - In an incompressible three-dimensional flow field,...Ch. 5 - In a two-dimensional incompressible flow field,...Ch. 5 - The three components of velocity in a velocity...Ch. 5 - The x component of velocity in a steady,...Ch. 5 - The y component of velocity in a steady...Ch. 5 - The velocity components for an incompressible...Ch. 5 - The radial component of velocity in an...Ch. 5 - A crude approximation for the x component of...
Ch. 5 - A useful approximation for the x component of...Ch. 5 - A useful approximation for the x component of...Ch. 5 - For a flow in the xy plane, the x component of...Ch. 5 - Consider a water stream from a jet of an...Ch. 5 - Which of the following sets of equations represent...Ch. 5 - For an incompressible flow in the r plane, the r...Ch. 5 - A viscous liquid is sheared between two parallel...Ch. 5 - A velocity field in cylindrical coordinates is...Ch. 5 - Determine the family of stream functions that...Ch. 5 - The stream function for a certain incompressible...Ch. 5 - Determine the stream functions for the following...Ch. 5 - Determine the stream function for the steady...Ch. 5 - Prob. 23PCh. 5 - A parabolic velocity profile was used to model...Ch. 5 - A flow field is characterized by the stream...Ch. 5 - A flow field is characterized by the stream...Ch. 5 - Prob. 27PCh. 5 - A flow field is characterized by the stream...Ch. 5 - In a parallel one-dimensional flow in the positive...Ch. 5 - Consider the flow field given by V=xy2i13y3j+xyk....Ch. 5 - Prob. 31PCh. 5 - The velocity field within a laminar boundary layer...Ch. 5 - A velocity field is given by V=10ti10t3j. Show...Ch. 5 - The y component of velocity in a two-dimensional,...Ch. 5 - A 4 m diameter tank is filled with water and then...Ch. 5 - An incompressible liquid with negligible viscosity...Ch. 5 - Sketch the following flow fields and derive...Ch. 5 - Consider the low-speed flow of air between...Ch. 5 - As part of a pollution study, a model...Ch. 5 - As an aircraft flies through a cold front, an...Ch. 5 - Wave flow of an incompressible fluid into a solid...Ch. 5 - A steady, two-dimensional velocity field is given...Ch. 5 - A velocity field is represented by the expression...Ch. 5 - A parabolic approximate velocity profile was used...Ch. 5 - A cubic approximate velocity profile was used in...Ch. 5 - The velocity field for steady inviscid flow from...Ch. 5 - Consider the incompressible flow of a fluid...Ch. 5 - Consider the one-dimensional, incompressible flow...Ch. 5 - Expand (V)V in cylindrical coordinates by direct...Ch. 5 - Determine the velocity potential for (a) a flow...Ch. 5 - Determine whether the following flow fields are...Ch. 5 - The velocity profile for steady flow between...Ch. 5 - Consider the velocity field for flow in a...Ch. 5 - Consider the two-dimensional flow field in which u...Ch. 5 - Consider a flow field represented by the stream...Ch. 5 - Fluid passes through the set of thin, closely...Ch. 5 - A two-dimensional flow field is characterized as u...Ch. 5 - A flow field is represented by the stream function...Ch. 5 - Consider the flow field represented by the stream...Ch. 5 - Consider the flow field represented by the stream...Ch. 5 - Consider the velocity field given by V=Ax2i+Bxyj,...Ch. 5 - Consider again the viscometric flow of Example...Ch. 5 - The velocity field near the core of a tornado can...Ch. 5 - A velocity field is given by V=2i4xjm/s. Determine...Ch. 5 - Consider the pressure-driven flow between...Ch. 5 - Consider a steady, laminar, fully developed,...Ch. 5 - Assume the liquid film in Example 5.9 is not...Ch. 5 - Consider a steady, laminar, fully developed...Ch. 5 - Consider a steady, laminar, fully developed...Ch. 5 - A linear velocity profile was used to model flow...Ch. 5 - A cylinder of radius ri rotates at a speed ...Ch. 5 - The velocity profile for fully developed laminar...Ch. 5 - Assume the liquid film in Example 5.9 is...Ch. 5 - The common thermal polymerase chain reaction (PCR)...Ch. 5 - A tank contains water (20C) at an initial depth y0...Ch. 5 - For a small spherical particle of styrofoam...Ch. 5 - Use Excel to generate the progression to an...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- The velocity of the mercury at T_∞=250 o C is U_∞=0.033m/sec, and it flows over the pipe bundle of D= 1.25 cm diameter and L=1.2m length. The ratio of the vertical and diagonal spacing distances for the pipes in the shifted plate arrangement is ST /D=SD /D= 1.405. It consists of a matrix with the number of pipe rows in the horizontal direction NL=60 and the number of pipe rows in the vertical direction NT=30. Pipe wall (surface) temperatures are kept at a uniform temperature of T_w=160 o C. Accordingly, find the average heat transfer coefficient, the total heat transfer coefficient from the mercury to the pipe bundle. (Variables: ST /D=SD /D= 0.75-1.975, U=0.02-0.07m/s, L=0.5-1.4m)arrow_forwardA B A gas enters a piping section AB from the left at density 1.15 kg/m³ and with speed 4 meters/sec. The pipe section has a constant diameter of 10 centimeters. While passing through AB the gas density changes to 0.95 kg/m³. Determine the speed with which the gas exits section AB in meters/sec. Assume steady flow and use the continuity equation. Your answer should include at least 2 digits after the decimal place.arrow_forwardThe stream function for an incompressible flow field is given by the equation ψ=3x^2y+y^3 where the stream function has the units of with x and y in meters. (a) Sketch the streamline passing through the origin. (b) Determine the rate of flow across the straight path.arrow_forward
- 4. The velocity vectors of three flow fileds are given as V, = axĩ + bx(1+1)}+ tk , V, = axyi + bx(1+t)j , and V3 = axyi – bzy(1+t)k where coefficients a and b have constant values. Is it correct to say that flow field 1 is one-, flow filed 2 is two-, and flow filed 3 is three-dimensional? Are these flow fields steady or unsteady?arrow_forwardA two-dimensional flow (x-y plane) is given by u =x +yt and v = - y+xtwhere u and y are the components of the velocity in the x and ydirections respectively, and t is time.A. Is this flow incompressible? Show how you reached yourconclusion.B. Determine the time rate of change of u an v for the fluid particlewhich is located at (x,y) = (2,2) at t = 2 seconds.arrow_forwardy = 2r'sin20 (2) 0.5 m A 20 inviscid incompressible In addition, if Y=1, draw a streamline. Also plot some polar coordinates (r, 0) passing through the streamline, and indicate the direction of the velocity fluid flow around a corner is described by the stream function as shown. The fluid density is 1000kg/m3, and the plane is horizontal, determine the velocity potential. If the pressure at (1) is 30 kPa, what the pressure at (2)? vector.arrow_forward
- 1-Consider the flow of a fluid in rectangular coordinates VX=XY2, VY=-X2Y, VZ=0 and ρ=xy. Make sure the velocity and density represent a physically possible flow. Remarks: For resolution, use the continuity equation. Furthermore, it is important to look in the literature for what flow is possible from the continuity equation together with the substitution of rectangular coordinate values.arrow_forwardPlease Answer Both!!!arrow_forwardIn three-dimensional fluid flow, the velocity component an u = * + y z, v = - (xy + yz + zx). Determine the %3D satisfy the continuity equation.arrow_forward
- 3. A circular cylinder of radius a is fitted with two pressure sensors to measure pressure at 0 = 180° and at 150°. The intent is to use this cylinder as a stream velocimeter, i.e. a device to determine the velocity of a stream by measuring the pressures at the two taps. The fluid is incompressible with a density of p. Figure for Part (a) U Figure for Part (b) 30 a) Using potential flow approximation, derive a formula for calculating U from the measured pressure difference at the two pressure taps. Note that for accurate measurement, the velocimeter must be aligned to have one of the taps exactly facing the stream as shown in the figure. (Ans: 2|Aptaps|/p ) b) Suppose the velocimeter has been misaligned by ổ degrees so that the two pressure taps are now at 180° + 8 and 150° + 8. Derive an expression for the percent error in stream velocity measurement. Then, calculate the error for 8 = 5°,10° and –10°. (Ans: [2/(sin2(150 + 8) – sin²(180 + 8) )– 1] × 100 )arrow_forwardA flat plate of width L= 1 m is pushed down with a velocity wall resulting in the drainage of the fluid between the plate and the wall as shown in the figure. Assume two-dimensional incompressible flow and that the plate remains parallel to the wall. The average velocity, Uvg of the fluid (in m/s) draining out at the U = 0.01 m/s towards a instant shows in the figure is (correct to three decimal places). Plate d = 0.1 m Uavg Wallarrow_forwardThe velocity field of a flow is given by V= axyi + by^2j where a = 1 m^-1s^-1 and b = - 0.5 m^-1s^-1. The coordinates are in meters. Determine whether the flow field is three-, two-, or one-dimensional. Find the equations of the streamlines and sketch several streamlines in the upper half planearrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY
Introduction to Kinematics; Author: LearnChemE;https://www.youtube.com/watch?v=bV0XPz-mg2s;License: Standard youtube license