Chapter 4.4, Problem 48AYU

In Problems 45-56, find the vertical, horizontal, and oblique asymptotes, if any, of each rational function.

$G\left(x\right)\text{ = }\frac{x^3\text{ + 1}}{x^2\text{ - 5}x\text{ - 14}}$

To determine

To find: Vertical, horizontal, or oblique asymptotes.

Answer to Problem 48AYU

Vertical asymptote are $x=-2$, 7, oblique asymptote is $y=x+5$.

Explanation of Solution

Given:

$G\left( x\right)=\frac{ x^3 +1}{ x^2 -5x-14}$

$G\left( x\right)=\frac{ x^3 +1}{ x^2 -5x-14}$

$G$ is in lowest terms, and the zero of the denominator is $x^2-5x-14=0$. The line $x=7$ and $x=-2$ are the vertical asymptote of the graph of $G$.

Therefore vertical asymptote is $x=-2$, 7.

Since the degree of the numerator, 3, is greater than the degree of the denominator, 3, the degree of the numerator is one more than the degree of the denominator, the line $y=ax+b$ is an oblique asymptote, which is the quotient found using long division.

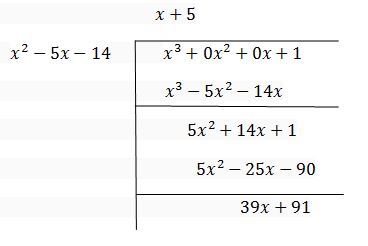
Therefore oblique asymptote is $y=x+5$.

Horizontal asymptotes: None.