Chapter 4, Problem 34RE

To determine

To analyze: The graph of Rational function $H(x)=\frac{x+2}{x(x-2)}$ .

Answer to Problem 34RE

The domain is $\left\{x|x\ne 0,2\right\}$ .

The vertical asymptote is $x=0,2$ .

The horizontal asymptote is $y=0$.

Explanation of Solution

Given information:

The rational function $H(x)=\frac{x+2}{x(x-2)}$ .

Formula used:

Vertical Asymptote: A rational function $R(x)=\frac{p(x)}{q(x)}$ , in lowest terms, will have a

asymptote $x=r$ if $r$ is a real zero of the denominator q .

Horizontal Asymptote: If a rational function is proper , the line $y=0$ is a asymptote of the graph.

Oblique Asymptote : it occur when the degree of the denominator of a rational

function is one less than the degree of the numerator. The asymptote will be along line

  $y=mx+b$ where $m\ne 0$

Calculation:

Consider ,

  $H(x)=\frac{x+2}{x(x-2)}$

Step 1.

The domain of H is the set of all real numbers except $x=0,2$ .

Step 2.

Now, H is in the lowest terms.

Step 3.

Now, the intercepts of the graph H are as follows:

x -intercept of the graph H

  $\begin{array}{l}=x+2=0\\ =x=-2\end{array}$

y -intercept:- There is no , y -intercept as x cannot equal to 0.

Step 4.

Denominator:-

  $=x(x-2)=0$

  $=x=0$ or $\begin{array}{l}=x-2=0\\ =x=2\end{array}$

The real zeros of the denominator H is $x=0,2$ . So, the graph of the H has one vertical asymptote $x=0,2$ .

Step 5.

Since the degree of the numerator ,1,is less than the degree of denominator 2.

Thus, the line $y=0$ is a horizontal asymptote of the graph H .

Step 6.

  

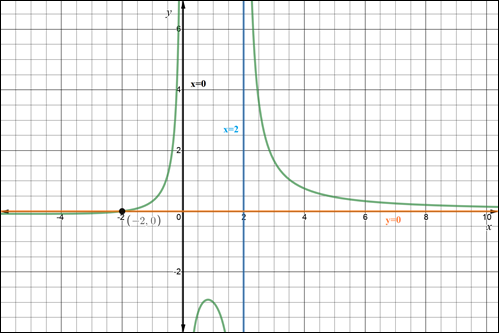
Figure 1.

Step 7.

Using the information gathered from Step 1 − Step 5 the graph of H has been drawn. The green color shows the graph H(x). The graph has the vertical asymptote at $x=0,2$ and horizontal asymptote at $y=0$ . The graph intersects the x -intercept at point $\left(-2,0\right)$ .