Chapter 4.1, Problem 88AYU

To determine

To analyze: The graph of a polynomial function.

Answer to Problem 88AYU

$\text{The graph of a polynomial function }f\left(x\right)={\left(x-2\right)}^2\left(x+2\right)\left(x+4\right)\text{ is analyzed}$.

Explanation of Solution

Given:

$f\left(x\right)={\left(x-2\right)}^2\left(x+2\right)\left(x+4\right)$

Step 1: Determine the end behavior of the graph of the function.

$f\left(x\right)={\left(x-2\right)}^2\left(x+2\right)\left(x+4\right)$

Square the binomial term.

$f\left(x\right)=\left(x^2-4x+4\right)\left(x+2\right)\left(x+4\right)$

Multiply

$f\left(x\right)=x^4+2x^3-12x^2-8x+32$

The polynomial function $f$ is of degree 4. The graph of $f$ behaves like $y=x^4$ for large values of $\left|x\right|$.

Step 2: Find $x$ and $y\text{-intercepts}$ of the graph of the function.

The $y\text{-intercept }=f\left(0\right)=32$.

The $x\text{-intercept }=f\left(x\right)=0$.

$f\left(x\right)={\left(x-2\right)}^2\left(x+2\right)\left(x+4\right)$

$x=2\text{ or }x=-2\text{ or }x=-4$

The $x\text{-intercept }=x=2,-2,-4$.

Step 3: Determine the zeros of the function and their multiplicity. Using this information determine whether the graph crosses or touches the $X\text{-axis}$ at each $x\text{-intercept}$.

The zeros of $f=2,-2,-4$.

The zero $2$ has even multiplicity therefore the graph of $f$ touches the $X\text{-axis}$.

The zero $-2$ has odd multiplicity therefore the graph of $f$ crosses the $X\text{-axis}$.

The zero $-4$ has odd multiplicity therefore the graph of $f$ crosses the $X\text{-axis}$.

Step 4: Using the graphing utility to graph the function.

$y_1=x^2\left(x+3\right)\left(x+1\right)$

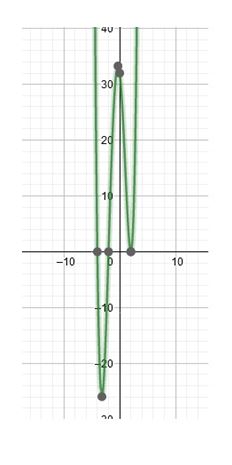
Step 5: Approximate the turning point of the graph.

From the graph of $f$, we see $f$ has 3 turning points.

Using the graph maximum turning point $=\left(0,32\right)$.

Using the graph minimum turning point $=\left(2,-25\right)$.

Step 6: Redraw the graph.

The graph passes through $\left(2,0\right)$ and $\left(-4,0\right)$.

Step 7: Find the domain and range of the function.

The domain and range is the set of all real numbers

Step 8: Use the graph to determine where the function is increasing or where it is decreasing.

Based on the graph, $f$ is increasing $\left[-2,0\right]$ and $\left[2,\infty \right)$.

Based on the graph, $f$ is decreasing $\left(\infty ,-2\right]\left[0,2\right]$.