7th Edition

ISBN: 9780321733627

Author: Douglas C. Giancoli

Publisher: PEARSON

Concept explainers

Newton’s laws of motion describe the relationship between the motion of an object and forces acting on it. Newton’s law of motion has two types as follows:

Videos

Textbook Question

Chapter 4, Problem 50P

A person pushes a 14.0-kg lawn mower at constant speed with a force of F = 88.0 N directed along the handle, which is at an angle of 45.0° to the horizontal (Fig. 4-58) (a) Draw the free-body diagram showing all forces acting on the mower. Calculate (b) the horizontal friction force on the mower, then (c) the normal force exerted vertically upward on the mower by the ground (d) What force must the person exert on the lawn mower to accelerate it from rest to 1.5 m/s in 2.5 seconds, assuming the same friction force?

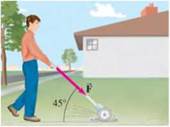
Chapter 4, Problem 50P, A person pushes a 14.0-kg lawn mower at constant speed with a force of F = 88.0 N directed along the

Figure 4-58 Problem 50.

Expert Solution & Answer

Want to see the full answer?

Check out a sample textbook solution

See solution

Chapter 4, Problem 49P

Chapter 4, Problem 51P

Students have asked these similar questions

I. How many significant figures are in the following: 1. 493 = 3 2. .0005 = | 3. 1,000,101 4. 5.00 5. 2.1 × 106 6. 1,000 7. 52.098 8. 0.00008550 9. 21 10.1nx=8.817

please solve and answer the question correctly please. Thank you!! (Hint in second photo)

please solve and answer the question correctly please. Thank you!!

Chapter 4 Solutions

Physics

Ch. 4 - A 150-kg football player collides head-on with a...Ch. 4 - A line by the poet T. S. Eliot (from Murder in the...Ch. 4 - Why does a child in a wagon seem to fall backward...Ch. 4 - A box rests on the (frictionless) bed of a truck....Ch. 4 - Prob. 3Q Ch. 4 - If the acceleration of an object is zero, are no...Ch. 4 - Prob. 5Q Ch. 4 - Prob. 6Q Ch. 4 - Prob. 7Q Ch. 4 - (a) Why do you push down harder on the pedals of a...

Ch. 4 - A stone hangs by a fine thread from the ceiling,...Ch. 4 - Prob. 10Q Ch. 4 - Prob. 11Q Ch. 4 - Prob. 12Q Ch. 4 - Prob. 13Q Ch. 4 - Prob. 14Q Ch. 4 - Prob. 15Q Ch. 4 - Prob. 16Q Ch. 4 - Prob. 17Q Ch. 4 - Prob. 18Q Ch. 4 - Prob. 19Q Ch. 4 - A block is given a brief push so that it slides up...Ch. 4 - Prob. 21Q Ch. 4 - Prob. 22Q Ch. 4 - A truck is traveling horizontally to the right...Ch. 4 - You are trying to push your stalled car. Although...Ch. 4 - Matt, in the foreground of Fig. 4-39, is able to...Ch. 4 - A bear sling, Fig. 4-40, is used in some national...Ch. 4 - What causes the boat in Fig. 4-41 to move forward?...Ch. 4 - A person stands on a scale in an elevator. His...Ch. 4 - When a skier skis down a hill, the normal force...Ch. 4 - A golf ball is hit with a golf club. While the...Ch. 4 - Suppose an object is accelerated by a force of 100...Ch. 4 - You are pushing a heavy box across a rough floor....Ch. 4 - Prob. 11MCQ Ch. 4 - The normal force on an extreme skier descending a...Ch. 4 - To pull an old stump out of the ground, you and a...Ch. 4 - What force is needed to accelerate a sled (mass =...Ch. 4 - Prob. 2P Ch. 4 - How much tension must a rope withstand if it is...Ch. 4 - According to a simplified model of a mammalian...Ch. 4 - Superman must stop a 120-km/h train in 150 m to...Ch. 4 - A person has a reasonable chance of surviving an...Ch. 4 - What average force is required to stop a 950-kg...Ch. 4 - Prob. 8P Ch. 4 - Prob. 9P Ch. 4 - Prob. 10P Ch. 4 - Prob. 11P Ch. 4 - Prob. 12P Ch. 4 - Prob. 13P Ch. 4 - Prob. 14P Ch. 4 - Prob. 15P Ch. 4 - Prob. 16P Ch. 4 - Prob. 17P Ch. 4 - Prob. 18P Ch. 4 - Prob. 19P Ch. 4 - A box weighing 77.0 N rests on a table. A rope...Ch. 4 - Figure 4-46 Problem 21. 21. (I) Draw the free-body...Ch. 4 - Prob. 22P Ch. 4 - Arlene is to walk across a “high wire" strung...Ch. 4 - A window washer pulls herself upward using the...Ch. 4 - One 3.2-kg paint bucket is hanging by a massless...Ch. 4 - Prob. 26P Ch. 4 - A train locomotive is pulling two cars of the same...Ch. 4 - Prob. 28P Ch. 4 - At the instant a race began, a 65-kg sprinter...Ch. 4 - A 27-kg chandelier hangs from a ceiling on a...Ch. 4 - Prob. 31P Ch. 4 - Figure 4-53 [shows a block (mass mA) on a smooth...Ch. 4 - Prob. 33P Ch. 4 - Prob. 34P Ch. 4 - 35. (Ill) Suppose the pulley in Fig. 4-55 is...Ch. 4 - Prob. 36P Ch. 4 - A force of 35.0 N is required to start a 6.0-kg...Ch. 4 - Prob. 38P Ch. 4 - Prob. 39P Ch. 4 - Prob. 40P Ch. 4 - Prob. 41P Ch. 4 - A box is given a push so that it slides across the...Ch. 4 - Prob. 43P Ch. 4 - Prob. 44P Ch. 4 - Prob. 45P Ch. 4 - 46. (II) For the system of Fig. 4-32 (Example...Ch. 4 - Prob. 47P Ch. 4 - Prob. 48P Ch. 4 - Prob. 49P Ch. 4 - A person pushes a 14.0-kg lawn mower at constant...Ch. 4 - Prob. 51P Ch. 4 - (a) A box sits at rest on a rough 33° inclined...Ch. 4 - Prob. 53P Ch. 4 - Prob. 54P Ch. 4 - Prob. 55P Ch. 4 - A 25.0-kg box is released on a 27° incline and...Ch. 4 - Prob. 57P Ch. 4 - Prob. 58P Ch. 4 - The crate shown in Fig. 4-60 lies on a plane...Ch. 4 - A crate is given an initial speed of 3.0 m/s up...Ch. 4 - Prob. 61P Ch. 4 - Prob. 62P Ch. 4 - The coefficient of kinetic friction for a 22-kg...Ch. 4 - On an icy day, you worry about parking your car in...Ch. 4 - Two masses mA= 2.0 kg and mB= 5.0 kg are on...Ch. 4 - Prob. 66P Ch. 4 - Prob. 67P Ch. 4 - A 2.0-kg purse is dropped from the top of the...Ch. 4 - Prob. 69GP Ch. 4 - 70. A 75.0-kg person stands on a scale in an...Ch. 4 - Prob. 71GP Ch. 4 - Prob. 72GP Ch. 4 - Prob. 73GP Ch. 4 - Prob. 74GP Ch. 4 - Prob. 75GP Ch. 4 - (a) What minimum force F is needed to lift the...Ch. 4 - Prob. 77GP Ch. 4 - A jet aircraft is accelerating at 3.8 m/s2 as it...Ch. 4 - Prob. 79GP Ch. 4 - Prob. 80GP Ch. 4 - Prob. 81GP Ch. 4 - Prob. 82GP Ch. 4 - Prob. 83GP Ch. 4 - Prob. 84GP Ch. 4 - Prob. 85GP Ch. 4 - Prob. 86GP Ch. 4 - Prob. 87GP Ch. 4 - Prob. 88GP Ch. 4 - Prob. 89GP Ch. 4 - Prob. 90GP Ch. 4 - A 72-kg water skier is being accelerated by a ski...Ch. 4 - Prob. 92GP Ch. 4 - Prob. 93GP Ch. 4 - Prob. 94GP Ch. 4 - Prob. 95GP Ch. 4 - Prob. 96GP Ch. 4 - Prob. 97GP

Additional Science Textbook Solutions

Find more solutions based on key concepts

Explain all answers clearly, with complete sentences and proper essay structure if needed. An asterisk (*) desi...

Cosmic Perspective Fundamentals

1. Which is a function of the skeletal system? (a) support, (b) hematopoietic site, (c) storage, (d) providing ...

Anatomy & Physiology (6th Edition)

53. This reaction was monitored as a function of time: A plot of In[A] versus time yields a straight ...

Chemistry: Structure and Properties (2nd Edition)

Using the pKa values listed in Table 15.1, predict the products of the following reactions:

Organic Chemistry (8th Edition)

Modified True/False 6. __________ Halophiles inhabit extremely saline habitats, such as the Great Salt Lake.

Microbiology with Diseases by Body System (5th Edition)

10.71 Identify each of the following as an acid or a base: (10.1) H2SO4 RbOH Ca(OH)2 HI ...

Chemistry: An Introduction to General, Organic, and Biological Chemistry (13th Edition)

Knowledge Booster

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.