Fundamentals of Physics Extended
10th Edition
ISBN: 9781118230725
Author: David Halliday, Robert Resnick, Jearl Walker
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 30, Problem 23P
SSM Figure 30-47 shows two parallel loops of wire having a common axis. The smaller loop (radius r) is above the larger loop (radius R) by a distance x ⪢ R. Consequently, the magnetic field due to the counterclockwise current i in the larger loop is nearly uniform throughout the smaller loop. Suppose that x is increasing at the constant rate dx/dt = v. (a) Find an expression for the magnetic flux through the area of the smaller loop as a function of x. (Hint: See Eq. 29-27.) In the smaller loop, find (b) an expression for the induced emf and (c) the direction of the induced current.
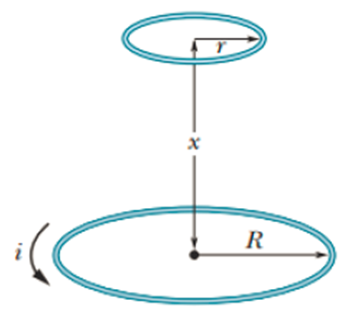
Figure 30-47 Problem 23.
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
A cylindrical region of radius R=3 cm contains a uniform
magnetic field parallel to its axis. If the electric field induced at a
point 1=6 cm from the cylinder axis is 4.5×10³ V/m, the
magnitude of magnetic field must be changing (in T/s) at the rate:
R
r
E
X X
X X
x xB
E
A loop of wire is at the edge of a region of space containing a uniform magnetic field B⃗. The plane of the loop is perpendicular to the magnetic field. Now the loop is pulled out of this region in such a way that the area Aof the coil inside the magnetic field region is decreasing at the constant rate ccc. That is, dA/dt=−c, with c>0. For the case of a square loop of side length L being pulled out of the magnetic field with constant speed v (see the figure), what is the rate of change of area c=−dA/dt?
Problem 7: A square loop of side length a =3.6 cm is placed a distance b = 0.65 cm from
a long wire carrying a current that varies with time at a constant rate, i.e.
I(t) = Qt,
a
where Q = 3.4 A/s is a constant.
Part (a) Find an expression for the magnetic field due to the wire as a function of timet at a distance r from the wire, in
terms of a, b, Q, t, r, and fundamental constants.
Expression :
B(t) =
Select from the variables below to write your expression. Note that all variables may not be required.
B, y, Ho, T, 0, d, g, h, j, m, n, P, Q, r, t
Part (b) What is the magnitude of the flux through the loop? Select the correct expression.
SchematicChoice :
HoQat
In
27
HoQa
In
HOQat
1
1
In
(b+a)2
h2
HoQa
In
1
1
HoQat
HOQat
In
In
6+ a
Ф—
(b+ a)²
(b+a)2
12
2т
h2
Part (c) If the loop has a resistance of 3.5 Q, how much induced current flows in the loop?
Numeric : A numeric value is expected and not an expression.
I =
Part (d) In what direction does this current flow?
MultipleChoice :
1)…
Chapter 30 Solutions
Fundamentals of Physics Extended
Ch. 30 - If the circular conductor in Fig. 30-21 undergoes...Ch. 30 - Prob. 2QCh. 30 - Prob. 3QCh. 30 - Prob. 4QCh. 30 - Prob. 5QCh. 30 - Prob. 6QCh. 30 - Prob. 7QCh. 30 - Prob. 8QCh. 30 - Prob. 9QCh. 30 - Prob. 10Q
Ch. 30 - Figure 30-31 shows three situations in which a...Ch. 30 - Figure 30-32 gives four situations in which we...Ch. 30 - Prob. 1PCh. 30 - A certain elastic conducting material is stretched...Ch. 30 - Prob. 3PCh. 30 - A wire loop of radius 12 cm and resistance 8.5 is...Ch. 30 - Prob. 5PCh. 30 - Figure 30-37a shows a circuit consisting of an...Ch. 30 - In Fig. 30-38, the magnetic flux through the loop...Ch. 30 - Prob. 8PCh. 30 - Prob. 9PCh. 30 - Prob. 10PCh. 30 - A rectangular coil of N turns and of length a and...Ch. 30 - Prob. 12PCh. 30 - Prob. 13PCh. 30 - GO In Fig. 30-42a, a uniform magnetic field B...Ch. 30 - GO A square wire loop with 2.00 m sides is...Ch. 30 - GO Figure 30-44a shows a wire that forms a...Ch. 30 - A small circular loop of area 2.00 cm2 is placed...Ch. 30 - Prob. 18PCh. 30 - ILW An electric generator contains a coil of 100...Ch. 30 - At a certain place, Earths magnetic field has...Ch. 30 - Prob. 21PCh. 30 - A rectangular loop area = 0.15 m2 turns in a...Ch. 30 - SSM Figure 30-47 shows two parallel loops of wire...Ch. 30 - Prob. 24PCh. 30 - GO Two long, parallel copper wires of diameter 2.5...Ch. 30 - GO For the wire arrangement in Fig. 30-49, a =...Ch. 30 - ILW As seen in Fig. 30-50, a square loop of wire...Ch. 30 - Prob. 28PCh. 30 - Prob. 29PCh. 30 - Prob. 30PCh. 30 - Prob. 31PCh. 30 - A loop antenna of area 2.00 cm2 and resistance...Ch. 30 - GO Figure 30-54 shows a rod of length L = 10.0 cm...Ch. 30 - Prob. 34PCh. 30 - Prob. 35PCh. 30 - Prob. 36PCh. 30 - Prob. 37PCh. 30 - Prob. 38PCh. 30 - Prob. 39PCh. 30 - Prob. 40PCh. 30 - A circular coil has a 10.0 cm radius and consists...Ch. 30 - Prob. 42PCh. 30 - Prob. 43PCh. 30 - Prob. 44PCh. 30 - Prob. 45PCh. 30 - Prob. 46PCh. 30 - Inductors in series. Two inductors L1 and L2 are...Ch. 30 - Prob. 48PCh. 30 - Prob. 49PCh. 30 - Prob. 50PCh. 30 - ILW The current in an RL circuit drops from 1.0 A...Ch. 30 - Prob. 52PCh. 30 - Prob. 53PCh. 30 - Prob. 54PCh. 30 - Prob. 55PCh. 30 - Prob. 56PCh. 30 - In Fig. 30-65, R = 15 , L = 5.0 H, the ideal...Ch. 30 - Prob. 58PCh. 30 - Prob. 59PCh. 30 - Prob. 60PCh. 30 - Prob. 61PCh. 30 - A coil with an inductance of 2.0 H and a...Ch. 30 - Prob. 63PCh. 30 - Prob. 64PCh. 30 - Prob. 65PCh. 30 - A circular loop of wire 50 mm in radius carries a...Ch. 30 - Prob. 67PCh. 30 - Prob. 68PCh. 30 - ILW What must be the magnitude of a uniform...Ch. 30 - Prob. 70PCh. 30 - Prob. 71PCh. 30 - Prob. 72PCh. 30 - Prob. 73PCh. 30 - Prob. 74PCh. 30 - Prob. 75PCh. 30 - Prob. 76PCh. 30 - Prob. 77PCh. 30 - Prob. 78PCh. 30 - SSM In Fig. 30-71, the battery is ideal and = 10...Ch. 30 - Prob. 80PCh. 30 - Prob. 81PCh. 30 - A uniform magnetic field B is perpendicular to the...Ch. 30 - Prob. 83PCh. 30 - Prob. 84PCh. 30 - Prob. 85PCh. 30 - Prob. 86PCh. 30 - Prob. 87PCh. 30 - Prob. 88PCh. 30 - A coil with an inductance of 2.0 H and a...Ch. 30 - Prob. 90PCh. 30 - Prob. 91PCh. 30 - Prob. 92PCh. 30 - Prob. 93PCh. 30 - A long cylindrical solenoid with 100 turns/cm has...Ch. 30 - Prob. 95PCh. 30 - A square loop of wire is held in a uniform 0.24 T...Ch. 30 - Prob. 97PCh. 30 - The inductance of a closely wound coil is such...Ch. 30 - The magnetic field in the interstellar space of...Ch. 30 - Prob. 100PCh. 30 - A toroid has a 5.00 cm square cross section, an...
Additional Science Textbook Solutions
Find more solutions based on key concepts
Modified True/False 3. __________ Aquatic microorganisms are more prevalent near the surface than at the bottom...
Microbiology with Diseases by Body System (5th Edition)
A 1500 kg car traveling at 10 m/s suddenly runs out of gas while approaching the valley shown in FIGURE EX10.1...
Physics for Scientists and Engineers: A Strategic Approach, Vol. 1 (Chs 1-21) (4th Edition)
89. Classify each chemical reaction as a synthesis, decomposition, single-displacement, or double-displacement ...
Introductory Chemistry (6th Edition)
All of the following terms can appropriately describe humans except: a. primary consumer b. autotroph c. hetero...
Human Biology: Concepts and Current Issues (8th Edition)
The mammalian trachea and esophagus both connect to the (A) pharynx. (B) stomach. (C) large intestine. (D) rect...
Campbell Biology (11th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- A long, solid, cylindrical conductor of radius 3.0 cm carries a current of 50 A distributed uniformly over its cross-section. Plot the magnetic field as a function of the radial distance r from the center of the conductor.arrow_forwardA long, straight, cylindrical conductor contains a cylindrical cavity whose axis is displaced by n from the axis of the conductor, as shown in the accompanying figure. The current density in the conductor is given by J=J0k, where J0 is a constant and k is along the axis of the conductor. Calculate the magnetic field at an arbitrary point P in the cavity by superimposing the field of a solid cylindrical conductor with radius R1and current density Jonto the field of a solid cylindrical conductor with radius R2and current density J . Then use the fact that the appropriate azimuthal unit vectors can be expressed as 1=kr1and 2=kr2 to show that everywhere inside the cavity the magnetic field is given by the constant B=120J0ka , where a=r1r2 and r1=r1r1 is the position of P relative to the center of the conductor and r2=r2r2 is the position of P relative to the center of the cavity.arrow_forwardA magnetic field directed into the page changes with time according to B = 0.030 0t2 + 1.40, where B is in teslas and t is in seconds. The field has a circular cross section of radius R = 2.50 cm (see Fig. P23.28). When t = 3.00 s and r2 = 0.020 0 m, what are (a) the magnitude and (b) the direction of the electric field at point P2?arrow_forward
- A toroid has a major radius R and a minor radius r and is tightly wound with N turns of wire on a hollow cardboard torus. Figure P31.6 shows half of this toroid, allowing us to see its cross section. If R r, the magnetic field in the region enclosed by the wire is essentially the same as the magnetic field of a solenoid that has been bent into a large circle of radius R. Modeling the field as the uniform field of a long solenoid, show that the inductance of such a toroid is approximately L=120N2r2R Figure P31.6arrow_forwardThe magnetic flux through a metal ring varies with time t according to (B = at3 bt2, where (B is in webers, a = 6.00 s3, b = 18.0 s2, and t is in seconds. The resistance of the ring is 3.00 . For the interval from t = 0 to t = 2.00 s, determine the maximum current induced in the ring.arrow_forwardTwo frictionless conducting rails separated by l = 55.0 cm are connected through a 2.00- resistor, and the circuit is completed by a bar that is free to slide on the rails (Fig. P32.71). A uniform magnetic field of 5.00 T directed out of the page permeates the region, a. What is the magnitude of the force Fp that must be applied so that the bar moves with a constant speed of 1.25 m/s to the right? b. What is the rate at which energy is dissipated through the 2.00- resistor in the circuit?arrow_forward
- Assume the region to the right of a certain plane contains a uniform magnetic field of magnitude 1.00 mT and the field is zero in the region to the left of the plane as shown in Figure P22.71. An electron, originally traveling perpendicular to the boundary plane, passes into the region of the field. (a) Determine the time interval required for the electron to leave the field-filled region, noting that the electrons path is a semicircle. (b) Assuming the maximum depth of penetration into the field is 2.00 cm, find the kinetic energy of the electron.arrow_forwardA square loop with side length L, mass M, and resistance R lies in the xy plane. A magnetic field B = B0(y/L) k is present in the region of the space near the loop. Determine the magnitude and direction of the induced current in the loop as the loop starts moving at velocity v = B0(y/L) j.arrow_forwardA wire is bent in the form of a square loop with sides of length L (Fig. P30.24). If a steady current I flows in the loop, determine the magnitude of the magnetic field at point P in the center of the square. FIGURE P30.24arrow_forward
- The accompanying figure shows a cross-section of a long, hollow, cylindrical conductor of inner radius r1= 3.0 cm and outer radius r2= 5.0 cm. A 50-A current distributed uniformly over the cross-section flows into the page. Calculate the magnetic field at r = 2.0 cm. r = 4.0 cm. and r = 6.0 cm.arrow_forwardSketch a plot of the magnitude of the magnetic field as a function of position r for a coax (Fig. P31.27).arrow_forwardTwo infinitely long current-carrying wires run parallel in the xy plane and are each a distance d = 11.0 cm from the y axis (Fig. P30.83). The current in both wires is I = 5.00 A in the negative y direction. a. Draw a sketch of the magnetic field pattern in the xz plane due to the two wires. What is the magnitude of the magnetic field due to the two wires b. at the origin and c. as a function of z along the z axis, at x = y = 0? FIGURE P30.83arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...
Physics
ISBN:9781337553292
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...
Physics
ISBN:9781305116399
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers
Physics
ISBN:9781337553278
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Magnets and Magnetic Fields; Author: Professor Dave explains;https://www.youtube.com/watch?v=IgtIdttfGVw;License: Standard YouTube License, CC-BY