
Concept explainers
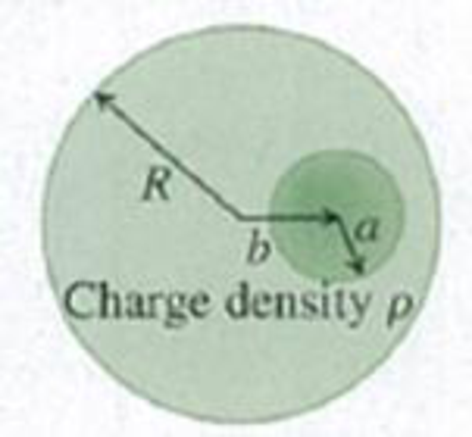
(a) An insulating sphere with radius a has a uniform charge density ρ. The sphere is not centered at the origin but at
Figure P22.57
Want to see the full answer?
Check out a sample textbook solution
Chapter 22 Solutions
University Physics (14th Edition)
Additional Science Textbook Solutions
Introduction to Electrodynamics
Physics: Principles with Applications
Applied Physics (11th Edition)
College Physics: A Strategic Approach (4th Edition)
The Cosmic Perspective Fundamentals (2nd Edition)
Physics for Scientists and Engineers: A Strategic Approach with Modern Physics (4th Edition)
- A solid conducting sphere of radius 2.00 cm has a charge 8.00 μC. A conducting spherical shell of inner radius 4.00 cm and outer radius 5.00 cm is concentric with the solid sphere and has a total charge −4.00 μC. Find the electric field at (a) r = 1.00 cm, (b) r = 3.00 cm, (c) r = 4.50 cm, and (d) r = 7.00 cm from the center of this charge configuration.arrow_forwardTwo solid spheres, both of radius 5 cm, carry identical total charges of 2 C. Sphere A is a good conductor. Sphere B is an insulator, and its charge is distributed uniformly throughout its volume. (i) How do the magnitudes of the electric fields they separately create at a radial distance of 6 cm compare? (a) EA EB = 0 (b) EA EB 0 (c) EA = EB 0 (d) 0 EA EB (e) 0 = EA EB (ii) How do the magnitudes of the electric fields they separately create at radius 4 cm compare? Choose from the same possibilities as in part (i).arrow_forwardThe nonuniform charge density of a solid insulating sphere of radius R is given by = cr2 (r R), where c is a positive constant and r is the radial distance from the center of the sphere. For a spherical shell of radius r and thickness dr, the volume element dV = 4r2dr. a. What is the magnitude of the electric field outside the sphere (r R)? b. What is the magnitude of the electric field inside the sphere (r R)?arrow_forward
- Recall that in the example of a uniform charged sphere, p0=Q/(43R3). Rewrite the answers in terms of the total charge Q on the sphere.arrow_forwardConsider a thin, spherical shell of radius 14.0 cm with a total charge of 32.0 C distributed uniformly on its surface. Find the electric field (a) 10.0 cm and (b) 20.0 cm from the center of the charge distribution.arrow_forwardTwo infinite, nonconducting sheets of charge are parallel to each other as shown in Figure P19.73. The sheet on the left has a uniform surface charge density , and the one on the right hits a uniform charge density . Calculate the electric field at points (a) to the left of, (b) in between, and (c) to the right of the two sheets. (d) What If? Find the electric fields in all three regions if both sheets have positive uniform surface charge densities of value .arrow_forward
- A solid, insulating sphere of radius a has a uniform charge density throughout its volume and a total charge Q. Concentric with this sphere is an uncharged, conducting, hollow sphere whose inner and outer radii are b and c as shown in Figure P19.75. We wish to understand completely the charges and electric fields at all locations. (a) Find the charge contained within a sphere of radius r a. (b) From this value, find the magnitude of the electric field for r a. (c) What charge is contained within a sphere of radius r when a r b? (d) From this value, find the magnitude of the electric field for r when a r b. (e) Now consider r when b r c. What is the magnitude of the electric field for this range of values of r? (f) From this value, what must be the charge on the inner surface of the hollow sphere? (g) From part (f), what must be the charge on the outer surface of the hollow sphere? (h) Consider the three spherical surfaces of radii a, b, and c. Which of these surfaces has the largest magnitude of surface charge density?arrow_forwardA continuous line of charge lies along the x axis, extending from x = +x0 to positive infinity. The line carries positive charge with a uniform linear charge density ?0. (a) What is the magnitude of the electric field at the origin?arrow_forwardCharge of a uniform density (11 pC/m?) is distributed over the entire xy plane. A charge of uniform density (6 pC/m2) is distributed over the parallel plane defined by z = 2.0 m. Determine the magnitude of the electric field for any point with z = 3.0 m.arrow_forward
- A solid insulating sphere of radius 5 cm carries electric charge uniformly distributed throughout its volume. Concentric with the sphere is a conducting spherical shell with no net charge as shown in the figure. The inner radius of the shell is 10 cm, and the outer radius is 15 cm. No other charges are nearby. Rank the magnitude of the electric field at points A (at radius 4 cm), B (radius 8 cm), C (radius 12 cm), and D (radius 16 cm) from largest to smallest. Display any cases of equality in your ranking. 1. A>B>D>C 2. B D A>C 3. D>B D>A ABC 4. C>B>A=D 5. None of these 01 03arrow_forwardAn insulating solid sphere of radius a has a uniform bulk density ρ and a total positive charge Q. Calculate the magnitude of the electric field at a point outside the sphere.arrow_forwardConsider an infinitely long, hollow cylinder, with inner radius R1 and outer radius Ra. the material of the cylinder is uniformly charged so that the cylinder has a charge per length. Finally, consider a solid cylinder of radius R, with a uniform charge density. What is the magnitude of the electric field inside the cylinder at a distance r from the center (r<R)?arrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning


