Fundamentals of Physics Extended
10th Edition
ISBN: 9781118230725
Author: David Halliday, Robert Resnick, Jearl Walker
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 17, Problem 20P
Figure 17-36 shows four isotropic point sources of sound that are uniformly spaced on an x axis. The sources emit sound at the same wavelength λ and same amplitude sm, and they emit in phase. A point P is shown on the x axis. Assume that as the sound waves travel to P the decrease in their amplitude is negligible. What multiple of sm is the amplitude of the net wave at P if distance d in the figure is (a) λ/4, (b) λ/2, and (c) λ?
Figure 17-36 Problem 20.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
suggest a reason ultrasound cleaning is better than cleaning by hand?
Checkpoint 4
The figure shows four orientations of an electric di-
pole in an external electric field. Rank the orienta-
tions according to (a) the magnitude of the torque
on the dipole and (b) the potential energy of the di-
pole, greatest first.
(1)
(2)
E
(4)
What is integrated science.
What is fractional distillation
What is simple distillation
Chapter 17 Solutions
Fundamentals of Physics Extended
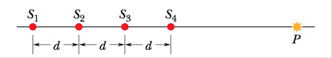
Ch. 17 - In a first experiment, a sinusoidal sound wave is...Ch. 17 - In Fig. 17-25, two point sources S1, and S2, which...Ch. 17 - In Fig. 17-26, three long tubes A,B, and C are...Ch. 17 - Prob. 4QCh. 17 - In Fig. 17-27, pipe A is made to oscillate in its...Ch. 17 - Prob. 6QCh. 17 - Figure 17-28 shows a moving sound source S that...Ch. 17 - Prob. 8QCh. 17 - For a particular tube, here are four of the six...Ch. 17 - Prob. 10Q
Ch. 17 - You are given four tuning forks. The fork with the...Ch. 17 - Two spectators at a soccer game see, and a moment...Ch. 17 - What is the bulk modulus of oxygen if 32.0 g of...Ch. 17 - Prob. 3PCh. 17 - A column of soldiers, marching at 120 paces per...Ch. 17 - Prob. 5PCh. 17 - A man strikes one end of a thin rod with a hammer....Ch. 17 - SSM WWW A stone is dropped into a well. The splash...Ch. 17 - GO Hot chocolate effect. Tap a metal spoon inside...Ch. 17 - If the form of a sound wave traveling through air...Ch. 17 - Prob. 10PCh. 17 - SSM Diagnostic ultrasound of frequency 4.50 MHz is...Ch. 17 - The pressure in a traveling sound wave is given by...Ch. 17 - A sound wave of the form s = sm coskx t travels...Ch. 17 - Figure 17-32 shows the output from a pressure...Ch. 17 - GO A handclap on stage in an amphitheater sends...Ch. 17 - Two sound waves, from two different sources with...Ch. 17 - Prob. 17PCh. 17 - Prob. 18PCh. 17 - GO Figure 17-35 shows two isotropic point sources...Ch. 17 - Figure 17-36 shows four isotropic point sources of...Ch. 17 - SSM In Fig. 17-37, two speakers separated by...Ch. 17 - In Fig. 17-38, sound with a 40.0 cm wavelength...Ch. 17 - GO Figure 17-39 shows two point sources S1 and S2...Ch. 17 - Suppose that the sound level of a conversation is...Ch. 17 - A sound wave of frequency 300Hz has an intensity...Ch. 17 - Prob. 26PCh. 17 - SSM WWW A certain sound source is increased in...Ch. 17 - Two sounds differ in sound level by 1.00 dB. What...Ch. 17 - Prob. 29PCh. 17 - The source of a sound wave has a power of 1.00 W....Ch. 17 - GO When you crack a knuckle, you suddenly widen...Ch. 17 - Approximately a third of people with normal...Ch. 17 - Male Rana catesbeiana bullfrogs arc known for...Ch. 17 - GO Two atmospheric sound sources A and B emit...Ch. 17 - A point source emits 30.0 W of sound...Ch. 17 - Party hearing. As the number of people at a party...Ch. 17 - Prob. 37PCh. 17 - The water level in a vertical glass tube 1.00 m...Ch. 17 - Prob. 39PCh. 17 - Organ pipe A, with both ends open, has a...Ch. 17 - A violin siring 15.0 cm long and fixed at both...Ch. 17 - A sound wave in a fluid medium is reflected at a...Ch. 17 - SSM In Fig. 17-41, S is a small loudspeaker driven...Ch. 17 - The crest of a Parasaurolophus dinosaur skull is...Ch. 17 - In pipe A, the ratio of a particular harmonic...Ch. 17 - GO Pipe A. which is 1.20 m long and open at both...Ch. 17 - A well with vertical sides and water at the bottom...Ch. 17 - One of the harmonic frequencies of tube A with two...Ch. 17 - SSM A violin string 30.0 cm long with linear...Ch. 17 - Prob. 50PCh. 17 - The A string of a violin is a little too tightly...Ch. 17 - A tuning fork of unknown frequency makes 3.00...Ch. 17 - SSM Two identical piano wires have a fundamental...Ch. 17 - You have five tuning forks that oscillate at close...Ch. 17 - Prob. 55PCh. 17 - An ambulance with a siren emitting a whine at 1600...Ch. 17 - A state trooper chases a speeder along a straight...Ch. 17 - Prob. 58PCh. 17 - GO In Fig. 17-42, a French submarine and a U.S....Ch. 17 - A stationary motion detector sends sound waves of...Ch. 17 - GO A bat is flitting about in a cave, navigating...Ch. 17 - Figure 17-43 shows four tubes with lengths 1.0 m...Ch. 17 - ILWAn acoustic burglar alarm consists of a source...Ch. 17 - A stationary detector measures the frequency of a...Ch. 17 - GO A 2000 Hz siren and a civil defense official...Ch. 17 - GO Two trains are traveling toward each other at...Ch. 17 - SSM WWWA girl is sitting near the open window of a...Ch. 17 - Prob. 68PCh. 17 - SSMA jet plane passes over you at a height of 5000...Ch. 17 - A plane flies at 1.25 times the speed of sound....Ch. 17 - At a distance of 10 km, a 100 Hz horn, assumed to...Ch. 17 - A bullet is fired with a speed of 685 m/s. Find...Ch. 17 - Prob. 73PCh. 17 - The average density of Earths crust 10 km beneath...Ch. 17 - A certain loudspeaker system emits sound...Ch. 17 - Find the ratios greater to smaller of the a...Ch. 17 - Prob. 77PCh. 17 - A trumpet player on a moving railroad flatcar...Ch. 17 - GO In Fig. 17-46, sound of wavelength 0.850 m is...Ch. 17 - GO A detector initially moves at constant velocity...Ch. 17 - SSMa If two sound waves, one in air and one in...Ch. 17 - A continuous sinusoidal longitudinal wave is sent...Ch. 17 - SSMUltrasound, which consists of sound waves with...Ch. 17 - The speed of sound in a certain metal is vm. One...Ch. 17 - An avalanche of sand along some rare desert sand...Ch. 17 - A sound source moves along an x axis, between...Ch. 17 - SSMA siren emitting a sound of frequency 1000 Hz...Ch. 17 - Prob. 88PCh. 17 - Prob. 89PCh. 17 - Prob. 90PCh. 17 - Prob. 91PCh. 17 - You can estimate your distance from a lightning...Ch. 17 - SSMFigure 17-48 shows an air-filled, acoustic...Ch. 17 - Prob. 94PCh. 17 - SSMThe sound intensity is 0.0080 W/m2 at a...Ch. 17 - Four sound waves are to be sent through the same...Ch. 17 - Prob. 97PCh. 17 - A point source that is stationary on an x axis...Ch. 17 - You are standing at a distance D from an isotropic...Ch. 17 - Pipe A has only one open end; pipe B is four times...Ch. 17 - A pipe 0.60 m long and closed at one end is filled...Ch. 17 - A sound wave travels out uniformly in all...Ch. 17 - A police car is chasing a speeding Porsche 911....Ch. 17 - Suppose a spherical loudspeaker emits sound...Ch. 17 - In Fig. 17-35. S1 and S2 are two isotropic point...Ch. 17 - Prob. 106PCh. 17 - Kundts method for measuring the speed of sound. In...Ch. 17 - Prob. 108PCh. 17 - In Fig. 17-53, a point source S of sound waves...Ch. 17 - A person on a railroad car blows a trumpet note at...Ch. 17 - A listener at rest with respect to the air and the...
Additional Science Textbook Solutions
Find more solutions based on key concepts
Name the components (including muscles) of the thoracic cage. List the contents of the thorax.
Human Physiology: An Integrated Approach (8th Edition)
The similarity and difference in ionic and covalent bond should be determined. Concept Introduction: The intera...
Living By Chemistry: First Edition Textbook
Could an organism be a fermenter and also be both MR and V—P negative? Explain.
Laboratory Experiments in Microbiology (12th Edition) (What's New in Microbiology)
Choose the best answer to each of the following. Explain your reasoning. Which describes our understanding of f...
Cosmic Perspective Fundamentals
6.1 State the number of electrons that be must be lost by atoms of each of the following to achieve a stable el...
Chemistry: An Introduction to General, Organic, and Biological Chemistry (13th Edition)
Q1. Which wavelength of light has the highest frequency?
a) 10 nm
b) 10 mm
c) 1 nm
d) 1 mm
Chemistry: A Molecular Approach (4th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- 19:39 · C Chegg 1 69% ✓ The compound beam is fixed at Ę and supported by rollers at A and B. There are pins at C and D. Take F=1700 lb. (Figure 1) Figure 800 lb ||-5- F 600 lb بتا D E C BO 10 ft 5 ft 4 ft-—— 6 ft — 5 ft- Solved Part A The compound beam is fixed at E and... Hình ảnh có thể có bản quyền. Tìm hiểu thêm Problem A-12 % Chia sẻ kip 800 lb Truy cập ) D Lưu of C 600 lb |-sa+ 10ft 5ft 4ft6ft D E 5 ft- Trying Cheaa Những kết quả này có hữu ích không? There are pins at C and D To F-1200 Egue!) Chegg Solved The compound b... Có Không ☑ ||| Chegg 10 וחarrow_forwardNo chatgpt pls will upvotearrow_forwardNo chatgpt pls will upvotearrow_forward
- No chatgpt pls will upvotearrow_forwardair is pushed steadily though a forced air pipe at a steady speed of 4.0 m/s. the pipe measures 56 cm by 22 cm. how fast will air move though a narrower portion of the pipe that is also rectangular and measures 32 cm by 22 cmarrow_forwardNo chatgpt pls will upvotearrow_forward
- 13.87 ... Interplanetary Navigation. The most efficient way to send a spacecraft from the earth to another planet is by using a Hohmann transfer orbit (Fig. P13.87). If the orbits of the departure and destination planets are circular, the Hohmann transfer orbit is an elliptical orbit whose perihelion and aphelion are tangent to the orbits of the two planets. The rockets are fired briefly at the depar- ture planet to put the spacecraft into the transfer orbit; the spacecraft then coasts until it reaches the destination planet. The rockets are then fired again to put the spacecraft into the same orbit about the sun as the destination planet. (a) For a flight from earth to Mars, in what direction must the rockets be fired at the earth and at Mars: in the direction of motion, or opposite the direction of motion? What about for a flight from Mars to the earth? (b) How long does a one- way trip from the the earth to Mars take, between the firings of the rockets? (c) To reach Mars from the…arrow_forwardNo chatgpt pls will upvotearrow_forwarda cubic foot of argon at 20 degrees celsius is isentropically compressed from 1 atm to 425 KPa. What is the new temperature and density?arrow_forward
- Calculate the variance of the calculated accelerations. The free fall height was 1753 mm. The measured release and catch times were: 222.22 800.00 61.11 641.67 0.00 588.89 11.11 588.89 8.33 588.89 11.11 588.89 5.56 586.11 2.78 583.33 Give in the answer window the calculated repeated experiment variance in m/s2.arrow_forwardNo chatgpt pls will upvotearrow_forwardCan you help me solve the questions pleasearrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1
Physics
ISBN:9781938168277
Author:William Moebs, Samuel J. Ling, Jeff Sanny
Publisher:OpenStax - Rice University
What Are Sound Wave Properties? | Physics in Motion; Author: GPB Education;https://www.youtube.com/watch?v=GW6_U553sK8;License: Standard YouTube License, CC-BY