Chapter 1.3, Problem 28E

Solution

To Find: The suitable functions from twelve basic functions based on the given description.

The two functions whose graphs are identical except for a horizontal shift are:

  $\begin{array}{c}y=\sin x\\ y=\cos x\end{array}$

Given information:

The two functions whose graphs are identical except for a horizontal shift.

Given functions are:

  $\begin{array}{l}1.f\left(x\right)=x2.f\left(x\right)=x^2\\ 3.f\left(x\right)=x^{3}4.f\left(x\right)=\frac{1}{x}\\ 5.f\left(x\right)=\sqrt{x}6.f\left(x\right)=e^x\\ 7.f\left(x\right)=\ln x8.f\left(x\right)=\sin x\\ 9.f\left(x\right)=\cos x10.f\left(x\right)=\left|x\right|\\ 11.f\left(x\right)=\mathrm{int}x12.f\left(x\right)=\frac{1}{1+e^{-x}}\end{array}$

Calculation:

Find the two functions whose graphs are identical except for a horizontal shift:

In trigonometry, the sine and cosine functions are separated by a horizontal shift.

For example:

  $\sin \left(x+\frac{\pi }{2}\right)=\cos x$

Proof by sine angle sum identity:

  $\begin{array}{c}\sin \left(x+\frac{\pi }{2}\right)=\sin x\cos \frac{\pi }{2}+\sin \frac{\pi }{2}\cos x\\ \sin \left(x+\frac{\pi }{2}\right)=\sin x\cdot 0+1\cdot \cos x\\ \sin \left(x+\frac{\pi }{2}\right)=\cos x\end{array}$

The graphical representation of $y=\sin x$ is:

  

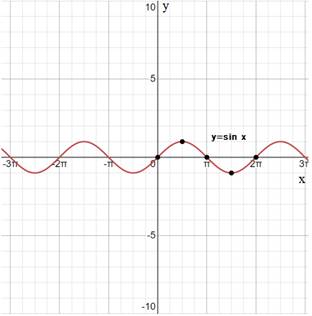
The graphical representation of $y=\cos x$ is:

  

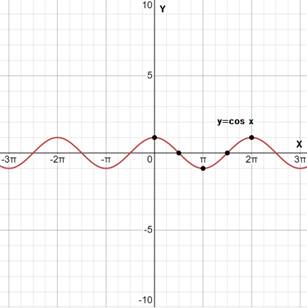
Thus, the two functions whose graphs are identical except for a horizontal shift are:

  $\begin{array}{c}y=\sin x\\ y=\cos x\end{array}$