Chapter 10, Problem 10.4.40P

A thin steel beam AB used in conjunction with an electromagnet in a high-energy physics experiment is securely bolted to rigid supports (see figure), A magnetic field produced by coils C results in a force acting on the beam. The force is trapezoidally distributed with maximum intensity q₀= 18 kN/m. The length of the beam between supports is L = 200 mm, and the dimension c of the trapezoidal load is 50 mm. The beam has a rectangular cross section with width b = 60 and height h = 20 mm.

Determine the maximum bending stress ${\sigma }_{\max }$ and the maximum deflection for the beam. (Disregard any effects of axial deformations and consider only the effects of bending. Use E = 200 GPa.)

  

To determine

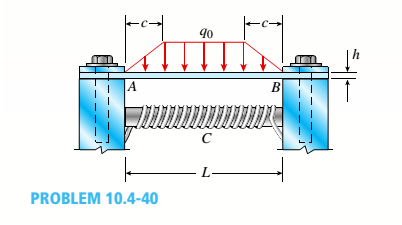
The maximum bending stress and the maximum deflection.

Answer to Problem 10.4.40P

The maximum bending stress is $13360000\text{N}/{\text{m}}^2$ .

The maximum deflection in the beam is $8.91\times {10}^{-6}\text{m}$ .

Explanation of Solution

Given information:

Width of the rectangular cross-section is $60\text{mm}$ , height is $20\text{mm}$ , maximum intensity is $18\text{kN}/\text{m}$ , length of the beam between supports is $200\text{mm}$ , dimension $c$ of the trapezoidal load is $50\text{mm,}$ and the modulus of elasticity is $200\text{GPa}$ .

The below figure shows the schematic diagram of the beam with parameters.

  

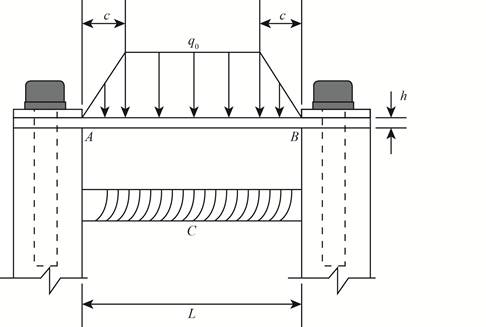
  Figure-(1)

Write the expression for the equilibrium in vertical direction.

  $\begin{array}{c}{R}_A+R_B=\frac{q_0}{2}\left(\frac{L}{4}\right)+\frac{q_0}{2}\left(\frac{L}{4}\right)+q_0\left(L-\frac{L}{2}\right)\\ =\frac{q_{0}L}{8}+\frac{q_{0}L}{8}+\frac{q_{0}L}{2}\\ =\frac{6q_{0}L}{8}\\ =\frac{3q_{0}L}{4}\end{array}$   ......(I)

Substitute $R_A$ for $R_B$ in Equation (I).

  $R_B=\frac{3q_{0}L}{8}$

Here, the reaction force at point A is $R_A$ , the reaction force at point B is $R_B$ , intensity of the load is $q_0,$ and length of the beam is $L$ .

There is symmetry in the beam therefore the reaction forces at A and B will be same.

There is symmetry in the beam therefore the moment at A will be equal to moment at B .

Write the expression for the relation between the reaction forces at A and B .

  $R_A=R_B$   ......(II)

Write the expression for the relation between the moment about A and B .

  $M_A=M_B$   ......(III)

Here, the moment about A is $M_A$ and the moment about B is $M_B$ .

The below figure shows the deflection in the beam.

  

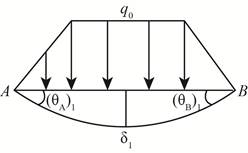
  Figure-(2)

Write the expression for the compatibility.

  ${\left({\theta }_A\right)}_1={\left({\theta }_B\right)}_1$   ......(IV)

Here, the rotation about point A is ${\left({\theta }_A\right)}_1$ and the rotation about point B is ${\left({\theta }_B\right)}_1$ .

The below figure shows the deflection slope.

  

  Figure-(3)

Write the expression for the slope from figure-(3).

  ${\theta }_0=\frac{Px\left(L-x\right)}{2EI}$   ......(V)

Here, the load is $P$ , distance is $x$ from point A , modulus of elasticity is $E$ and the moment of inertia is $I$ .

Write the expression for the deflection for figure-(3).

  ${\delta }_0=\frac{Px}{24EI}\left(3L^2-4x^2\right)$   ......(VI)

Write the expression for load.

  $P=qdx$

Write the expression for load from $0$ to $\frac{L}{4}$ .

  $q=\frac{4q_{0}x}{L}$   ......(VII)

Write the expression for load from $\frac{L}{4}$ to $\frac{L}{2}$ .

  $q=q_{0}x$   ......(VIII)

Write the expression for rotation about A .

  ${\left({\theta }_A\right)}_1={\displaystyle \underset{0}{\overset{\frac{L}{4}}{\int }}\frac{Px\left(L-x\right)}{2EI}}+{\displaystyle \underset{\frac{L}{4}}{\overset{\frac{L}{2}}{\int }}\frac{Px\left(L-x\right)}{2EI}}$   ......(IX)

Write the expression for the deflection.

  ${\left(\delta \right)}_1={\displaystyle \underset{0}{\overset{\frac{L}{4}}{\int }}\frac{Px}{24EI}\left(3L^2-4x^2\right)}+{\displaystyle \underset{\frac{L}{4}}{\overset{\frac{L}{2}}{\int }}\frac{Px}{24EI}\left(3L^2-4x^2\right)}$   ......(X)

The below figure shows the moments at the ends of the beam.

  

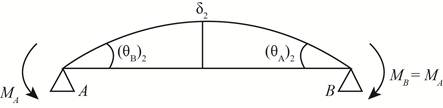
  Figure-(4)

Write the expression for the compatibility.

  ${\left({\theta }_A\right)}_2={\left({\theta }_B\right)}_2$   ......(XI)

Write the expression for slope for figure-(4).

  ${\left({\theta }_A\right)}_2=\frac{M_{A}L}{2EI}$   ......(XII)

Write the expression for the deflection for figure-(4).

  ${\delta }_2=\frac{M_A{L}^2}{8EI}$   ......(XIII)

Write the expression for maximum deflection.

  ${\delta }_{\max }={\delta }_1-{\delta }_2$   ......(XIV)

Substitute $\frac{361q_0{L}^4}{30720EI}$ for ${\delta }_1$ and $\frac{M_A{L}^2}{8EI}$ for ${\delta }_2$ in Equation (XIV).

  ${\delta }_{\max }=\frac{361q_0{L}^4}{30720EI}-\frac{M_A{L}^2}{8EI}$   ......(XV)

Write the expression for the moment about C .

  $M_C=R_A\left(\frac{L}{2}\right)-M_A-\frac{q_0{L}^2}{24}-\frac{q_0{L}^2}{32}$   ......(XVI)

Write the expression for the maximum moment.

  $\begin{array}{c}{M}_{\max }=M_A\\ M_{\max }=\frac{19q_0{L}^2}{256}\end{array}$   ......(XVII)

Write the expression for moment of inertia.

  $I=\frac{b{h}^3}{12}$   ......(XVIII)

Here, moment of inertia is $I$ and width is $b$ .

Write the expression for the section modulus.

  $S=\frac{b{h}^2}{6}$   ......(XIX)

Write the expression for the normal stress.

  $\sigma =\frac{M_{\max }}{S}$   ......(XX)

Calculation:

Substitute $\frac{4q_{0}x}{L}$ for $q$ from $0$ to $\frac{L}{4}$ and $q_{0}x$ for $\frac{L}{4}$ to $\frac{L}{2}$ in Equation (IX).

  $\begin{array}{c}{\left({\theta }_A\right)}_1={\displaystyle \underset{0}{\overset{\frac{L}{4}}{\int }}\frac{\left(\frac{4q_{0}x}{L}\right)x\left(L-x\right)}{2EI}}+{\displaystyle \underset{\frac{L}{4}}{\overset{\frac{L}{2}}{\int }}\frac{\left(q_{0}x\right)x\left(L-x\right)}{2EI}}\\ =\frac{4q_0}{2EIL}{\displaystyle \underset{0}{\overset{\frac{L}{4}}{\int }}{x}^2\left(L-x\right)}+\frac{q_0}{2EI}{\displaystyle \underset{\frac{L}{4}}{\overset{\frac{L}{2}}{\int }}{x}^2\left(L-x\right)}\\ =\frac{4q_0}{2EIL}{\left(\frac{L{x}^3}{3}-\frac{x^4}{4}\right)}_0^{\frac{L}{4}}+\frac{q_0}{2EI}{\left(\frac{L{x}^3}{3}-\frac{x^4}{4}\right)}_{\frac{L}{4}}^{\frac{L}{2}}\\ =\frac{4q_0}{2EIL}\left(\frac{L{\left(\frac{L}{4}\right)}^3}{3}-\frac{{\left(\frac{L}{4}\right)}^4}{4}\right)+\frac{q_0}{2EI}\left(\frac{L{\left(\frac{L}{2}\right)}^3}{3}-\frac{{\left(\frac{L}{2}\right)}^4}{4}-\frac{L{\left(\frac{L}{4}\right)}^3}{3}+\frac{{\left(\frac{L}{4}\right)}^4}{4}\right)\end{array}$

  $\begin{array}{c}{\left({\theta }_A\right)}_1=\frac{13q_0{L}^3}{1536EI}+\frac{11q_0{L}^3}{384EI}\\ =\frac{19q_0{L}^3}{512EI}\end{array}$

Substitute $\frac{4q_{0}x}{L}$ for $q$ from $0$ to $\frac{L}{4}$ and $q_{0}x$ for $\frac{L}{4}$ to $\frac{L}{2}$ in Equation (X).

  ${\left(\delta \right)}_1={\displaystyle \underset{0}{\overset{\frac{L}{4}}{\int }}\frac{\left(\frac{4q_{0}x}{L}\right)x}{24EI}\left(3L^2-4x^2\right)}+{\displaystyle \underset{\frac{L}{4}}{\overset{\frac{L}{2}}{\int }}\frac{\left(q_{0}x\right)x}{24EI}\left(3L^2-4x^2\right)}$

  $=\frac{1}{24EI}\left[\frac{4q_0}{L}{\left(\frac{L^2{x}^3}{3}-\frac{4x^5}{5}\right)}_0^{\frac{L}{4}}+{\left(3q_0{L}^2\frac{x^2}{2}-\frac{4q_0{x}^4}{4}\right)}_{\frac{L}{4}}^{\frac{L}{2}}\right]$

  $=\frac{1}{24EI}\left[\begin{array}{l}\frac{4q_0}{L}{\left(\frac{L^2{\left(\frac{L}{4}\right)}^3}{3}-\frac{4{\left(\frac{L}{4}\right)}^5}{5}\right)}_0^{\frac{L}{4}}\\ +{\left(3q_0{L}^2\frac{{\left(\frac{L}{2}\right)}^2}{2}-\frac{4q_0{\left(\frac{L}{2}\right)}^4}{4}-3q_0{L}^2\frac{{\left(\frac{L}{4}\right)}^2}{2}+\frac{4q_0{\left(\frac{L}{4}\right)}^4}{4}\right)}_{\frac{L}{4}}^{\frac{L}{2}}\end{array}\right]$

  $=\frac{19q_0{L}^4}{7680EI}+\frac{199q_0{L}^4}{2048EI}$

  ${\left(\delta \right)}_1=\frac{361q_0{L}^4}{30720EI}$

Substitute $\frac{19q_0{L}^2}{256}$ for $M_A$ in Equation (XV).

  $\begin{array}{c}{\delta }_{\max }=\frac{361q_0{L}^4}{30720EI}-\frac{\left(\frac{19q_0{L}^2}{256}\right)L^2}{8EI}\\ =\frac{361q_0{L}^4}{30720EI}-\left(\frac{19q_0{L}^2}{256}\right)\frac{L^2}{8EI}\\ {\delta }_{\max }=\frac{19q_0{L}^4}{7680EI}\end{array}$   ......(XXI)

Substitute $\frac{3q_{0}L}{8}$ for $R_A$ and $\frac{19q_0{L}^2}{256}$ for $M_A$ in Equation (XVI).

  $\begin{array}{c}{M}_C=\left(\frac{3q_{0}L}{8}\right)\left(\frac{L}{2}\right)-\frac{19q_0{L}^2}{256}-\frac{q_0{L}^2}{24}-\frac{q_0{L}^2}{32}\\ =\frac{3q_0{L}^2}{16}-\frac{19q_0{L}^2}{256}-\frac{q_0{L}^2}{24}-\frac{q_0{L}^2}{32}\\ =\frac{31q_0{L}^2}{768}\end{array}$

Substitute $60\text{mm}$ for $b$ and $20\text{mm}$ for $h$ in Equation (XVIII).

  $\begin{array}{c}I=\frac{\left(60\text{mm}\right){\left(20\text{mm}\right)}^3}{12}\\ =\frac{\left(60\text{mm}\left(\frac{{10}^{-3}\text{m}}{1\text{mm}}\right)\right){\left(20\text{mm}\left(\frac{{10}^{-3}\text{m}}{1\text{mm}}\right)\right)}^3}{12}\\ =\frac{\left(0.060\text{m}\right)\left({20}^3\times {10}^{-9}{\text{m}}^3\right)}{12}\\ =40\times {10}^{-9}{\text{m}}^4\end{array}$

Substitute $60\text{mm}$ for $b$ and $20\text{mm}$ for $h$ in Equation (XIX).

  $\begin{array}{c}S=\frac{\left(60\text{mm}\right){\left(20\text{mm}\right)}^2}{6}\\ =\frac{\left(60\text{mm}\left(\frac{{10}^{-3}\text{m}}{1\text{mm}}\right)\right){\left(20\text{mm}\left(\frac{{10}^{-3}\text{m}}{1\text{mm}}\right)\right)}^2}{6}\\ =\frac{0.060\text{m}\times {20}^2\times {10}^{-6}{\text{m}}^2}{6}\\ =4\times {10}^{-6}{\text{m}}^3\end{array}$

Substitute $18\text{kN}/\text{m}$ for $q_0$ and $200\text{mm}$ for $L$ in Equation (XVII).

  $\begin{array}{c}{M}_{\max }=\frac{19\left(18\text{kN}/\text{m}\right){\left(200\text{mm}\right)}^2}{256}\\ =\frac{19\left(18\text{kN}/\text{m}\left(\frac{1000\text{N}}{1\text{kN}}\right)\right){\left(200\text{mm}\left(\frac{{10}^{-3}\text{m}}{1\text{mm}}\right)\right)}^2}{256}\\ =53.44\text{N}\cdot \text{m}\end{array}$

Substitute $53.44\text{N}\cdot \text{m}$ for $M_{\max }$ and $4\times {10}^{-6}{\text{m}}^3$ for $S$ in Equation (XX).

  $\begin{array}{c}\sigma =\frac{53.44\text{N}\cdot \text{m}}{4\times {10}^{-6}{\text{m}}^3}\\ =\frac{53.44\text{N}\cdot \text{m}}{0.000004{\text{m}}^3}\\ =13360000\text{N}/{\text{m}}^2\end{array}$

Substitute $18\text{kN}/\text{m}$ for $q_0$ , $200\text{GPa}$ for $E$ , $40\times {10}^{-9}{\text{m}}^4$ for $I$ and $200\text{mm}$ for $L$ in Equation (XXI).

  $\begin{array}{c}{\delta }_{\max }=\frac{19\left(18\text{kN}/\text{m}\right){\left(200\text{mm}\right)}^4}{7680\left(200\text{GPa}\right)\left(40\times {10}^{-9}{\text{m}}^4\right)}\\ =\frac{19\left(18\text{kN}/\text{m}\left(\frac{1000\text{N}}{1\text{kN}}\right)\right){\left(200\text{mm}\left(\frac{{10}^{-3}\text{m}}{1\text{mm}}\right)\right)}^4}{7680\left(200\text{GPa}\left(\frac{{10}^9\text{Pa}}{1\text{GPa}}\right)\right)\left(40\times {10}^{-9}{\text{m}}^4\right)}\\ =\frac{19\left(18000\text{N}/\text{m}\right){\left(0.200\text{m}\right)}^4}{7680\left(2\times {10}^{11}\text{Pa}\left(\frac{1\text{N}/{\text{m}}^2}{1\text{Pa}}\right)\right)\left(40\times {10}^{-9}{\text{m}}^4\right)}\\ =8.91\times {10}^{-6}\text{m}\end{array}$

Conclusion:

The maximum bending stress is $13360000\text{N}/{\text{m}}^2$ .

The maximum deflection in the beam is $8.91\times {10}^{-6}\text{m}$ .