13th Edition

ISBN: 9780134462455

Author: Mario F. Triola

Publisher: PEARSON

See similar textbooks

Videos

Textbook Question

Chapter 9.2, Problem 16BSC

In Exercises 5–20, assume that the two samples are independent simple random samples selected from normally distributed populations, and do not assume that the population standard deviations are equal. (Note: Answers in Appendix D include technology answers based on Formula 9-1 along with “Table” answers based on Table A-3 with df equal to the smaller of n₁ − 1 and n₂ − 1.)

16. Bad Stuff in Children’s Movies Data Set 11 “Alcohol and Tobacco in Movies” in Appendix B includes lengths of times (seconds) of tobacco use shown in animated children’s movies. For the Disney movies, n = 33, x ¯ = 61.6 sec, s = 118.8 sec. For the other movies, n = 17, x ¯ = 49.3 sec, s = 69.3 sec. The sorted times for the non-Disney movies are listed below.

a. Use a 0.05 significance level to test the claim that Disney animated children’s movies and other animated children’s movies have the same mean time showing tobacco use.

b. Construct a confidence interval appropriate for the hypothesis test in part (a).

c. Conduct a quick visual inspection of the listed times for the non-Disney movies and comment on the normality requirement. How does the normality of the 17 non-Disney times affect the results?

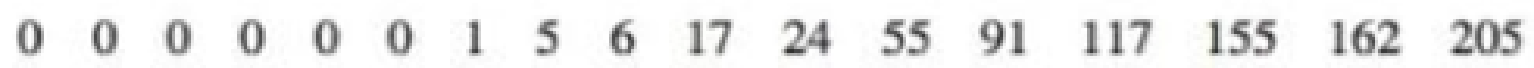
Chapter 9.2, Problem 16BSC, In Exercises 520, assume that the two samples are independent simple random samples selected from

Features Features Normal distribution is characterized by two parameters, mean (µ) and standard deviation (σ). When graphed, the mean represents the center of the bell curve and the graph is perfectly symmetric about the center. The mean, median, and mode are all equal for a normal distribution. The standard deviation measures the data's spread from the center. The higher the standard deviation, the more the data is spread out and the flatter the bell curve looks. Variance is another commonly used measure of the spread of the distribution and is equal to the square of the standard deviation.

Expert Solution & Answer

Want to see the full answer?

Check out a sample textbook solution

See solution

Chapter 9.2, Problem 15BSC

Chapter 9.2, Problem 17BSC

Students have asked these similar questions

Throughout, A, B, (An, n≥ 1), and (Bn, n≥ 1) are subsets of 2. 1. Show that AAB (ANB) U (BA) = (AUB) (AB), Α' Δ Β = Α Δ Β, {A₁ U A2} A {B₁ U B2) C (A1 A B₁}U{A2 A B2).

16. Show that, if X and Y are independent random variables, such that E|X|< ∞, and B is an arbitrary Borel set, then EXI{Y B} = EX P(YE B).

Proposition 1.1 Suppose that X1, X2,... are random variables. The following quantities are random variables: (a) max{X1, X2) and min(X1, X2); (b) sup, Xn and inf, Xn; (c) lim sup∞ X and lim inf∞ Xn- (d) If Xn(w) converges for (almost) every w as n→ ∞, then lim- random variable. → Xn is a

Chapter 9 Solutions

Elementary Statistics (13th Edition)

Ch. 9.1 - Verifying Requirements In the largest clinical...Ch. 9.1 - Verifying Requirements In the largest clinical...Ch. 9.1 - Hypotheses and Conclusions Refer to the hypothesis...Ch. 9.1 - Using Confidence Intervals a. Assume that we want...Ch. 9.1 - Interpreting Displays. In Exercises 5 and 6, use...Ch. 9.1 - Treating Carpal Tunnel Syndrome Carpal tunnel...Ch. 9.1 - Testing Claims About Proportions. In Exercises...Ch. 9.1 - Accuracy of Fast Food Drive-Through Orders In a...Ch. 9.1 - Testing Claims About Proportions. In Exercises...Ch. 9.1 - Testing Claims About Proportions. In Exercises...

Knowledge Booster

$Statistics$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.

Solution Summary: The author concludes that there is no sufficient evidence for rejection of the claims that Disney animated children's movies have the same mean time showing the use of tobacco.

Videos

Want to see the full answer?

Chapter 9 Solutions