Chapter 9.1, Problem 6GP

a.

To determine

To graph the given equation.

Explanation of Solution

Given information :

Given function is $y=-16x^2+64x+6$ .

Graph :

  

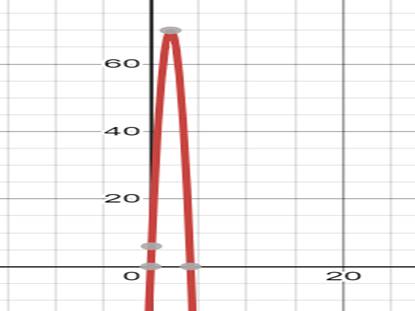
Interpretation :

The graph of a quadratic equation ( $y=a{x}^2+bx+c$ ) is a parabola. The parabola is symmetric in nature and the point of symmetry is known as the vertex. This vertex lies on the line of symmetry and is symmetric about this line.

This vertex point shall be:

Highest point (if $a<0$ ) and be called the maximum. Here, the graph opens downwards.

Or, lowest point (if $a>0$ ) and can be called the minimum. Here the graph opens upwards.

In this case, ‘a’ is lesser than 0 hence the graph will have a maximum and will open downwards.

A parabola always points to infinity, either negative or positive.

To graph a quadratic function, compute the axis of symmetry, vertex and y-intercept, post which, plot the same on a graph.

The axis of symmetry bisects the parabola into two equal parts. Hence each point on the parabola would have an equal point on the other side of the axis of symmetry. Plot these points on the graph with a smooth curve.

Formula to compute equation of the axis of symmetry $x=-\frac{b}{2\times a}$ . Here, $a=-16$ and $b=64$ . ‘a’ and ‘b’ are coefficients $x^2$ and $x$ respectively.

Axis of symmetry for the given function is

  $x=-\frac{b}{2\times a}$

Formula for axis of symmetry.

  $x=-\frac{64}{2\times \left(-16\right)}$

Putting the values of ‘a’ and ‘b’ .

  $=\frac{64}{32}$

Simplifying this

  $x=2$ is the equation of axis of symmetry.

Vertex can be found out by putting the value of x computed in the axis of symmetry in the original function. This will give a value of y . These two coordinates of x and y would be the point where the vertex is.

  $=-16{\left(2\right)}^2+64\left(2\right)+6$

Putting the value of $x=2$ in the original function.

  $=-64+128+6$

Simplifying the expression.

  $=70$

Thus the vertex is $\left(2,70\right)$ .

y-intercept is computed by substituting the value of x in the equation by 0.

  $=-16{\left(0\right)}^2+64\left(0\right)+6$

Simplifying

  $=6$

Hence the point of y-intercept is $\left(0,6\right)$ .

Now, plot these points along with their reflecting symmetric points, starting from the vertex.

b.

To determine

To calculate the height from which the javelin is thrown.

Answer to Problem 6GP

The height from with the javelin is thrown is 6 feet .

Explanation of Solution

Given information :

The function provided is $y=-16x^2+64x+6$ .

Formula used :

The javelin is thrown from a certain height. This can be calculated by finding the y-intercept of the equation.

For finding the y-intercept, substitute the value of x in the function with 0 and compute the value of y . Y -intercept is always given by the notion (0,y) .

Calculation :

y-intercept is computed by substituting the value of x in the equation by 0.

  $=-16{\left(0\right)}^2+64\left(0\right)+6$

Simplifying

  $=6$

Hence the point of y-intercept is $\left(0,6\right)$ .

Thus it can be said that the javelin was initially at the height of 6 feet . This is the y coordinate of the point of the y-intercept.

c.

To determine

To calculate the maximum height that the javelin reaches.

Answer to Problem 6GP

The height from with the javelin is thrown is 70 feet .

Explanation of Solution

Given information :

The function provided is $y=-16x^2+16x+5$ .

Formula used :

The maximum height can be calculated using the vertex formula. Here, find the y-coordinate of the point of the vertex. This value will be the maximum height attained by the javelin.

  $x=-\frac{b}{2\times a}$ . Here, $a=-16$ and $b=64$ . ‘a’ and ‘b’ are coefficients $x^2$ and $x$ respectively. The corresponding value of ‘y’ for the vertex would be the maximum or minimum.

Calculation :

Time at which the javelin reaches maximum height.

  $x=-\frac{b}{2\times a}$

  $x=-\frac{64}{2\times \left(-16\right)}$

Putting the values of ‘a’ and ‘b’ .

  $=\frac{64}{32}$

Simplifying

  $x=2$ seconds

Vertex can be found out by putting the value of x computed in the axis of symmetry in the original function. This will give a value of y , that will be the maximum height in feet.

  $=-16{\left(2\right)}^2+64\left(2\right)+6$

Putting the value of $x=2$ in the original function.

  $=-64+128+6$

Simplifying the expression.

  $=70$ feet.

Thus, the maximum height is at 70 feet .