Glencoe Algebra 1, Student Edition, 9780079039897, 0079039898, 2018
18th Edition
ISBN: 9780079039897
Author: Carter
Publisher: McGraw Hill
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Question
Chapter 9.2, Problem 26PPS
(a)
To determine
The sprinkler that send the water at the farthest.
(a)
Expert Solution
Answer to Problem 26PPS
The parabola for Sprinkler C is the most compressed vertically, so it will send water the farthest.
Explanation of Solution
Given information:
The path of water from a sprinkler can be modelled by quadratic functions. The following functions model paths for three different sprinklers.
Formula used:
The quadratic functions are of the form f (x) = ax2 + c
Calculation:
All three of the quadratic functions are of the form f (x) = ax2 + c. Use a graphing calculator to compare the graphs of the three functions. All three of the quadratic functions are of the form f (x) = ax2+ c. Use a graphing calculator to compare the graphs of the three functions.
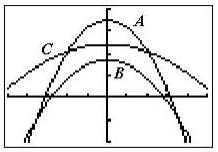
The value of a in the function for Sprinkler C is the smallest of the three. Therefore, the parabola for Sprinkler C is the most compressed vertically, so it will send water the farthest.
Conclusion:
The parabola for Sprinkler C is the most compressed vertically, so it will send water the farthest.
(b)
To determine
The sprinkler that send the water at the highest.
(b)
Expert Solution
Answer to Problem 26PPS
The parabola for Sprinkler A is translated up the most, so it will send water the highest.
Explanation of Solution
Given information:
SPRINKLERS the path of water from a sprinkler can be modelled by quadratic functions. The following functions model paths for three different sprinklers.
Formula used:
The quadratic functions are of the form f (x) = ax2 + c
Calculation:
All three of the quadratic functions are of the form f (x) = ax2 + c. Use a graphing calculator to compare the graphs of the three functions. All three of the quadratic functions are of the form f (x) = ax2+ c. Use a graphing calculator to compare the graphs of the three functions.
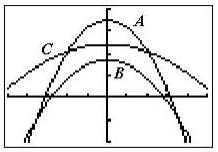
The value of c in the function for Sprinkler A is the largest of the three. Therefore, the parabola for Sprinkler A is translated up the most, so it will send water the highest.
Conclusion:
The parabola for Sprinkler A is translated up the most, so it will send water the highest.
(c)
To determine
The sprinkler that send the water at the narrowest path.
(c)
Expert Solution
Answer to Problem 26PPS
The parabola for Sprinkler A is the most stretched vertically, so it will expand the least, and therefore have the narrowest path.
Explanation of Solution
Given information:
SPRINKLERS the path of water from a sprinkler can be modelled by quadratic functions. The following functions model paths for three different sprinklers.
Formula used:
The quadratic functions are of the form f (x) = ax2 + c
Calculation:
All three of the quadratic functions are of the form f (x) = ax2 + c. Use a graphing calculator to compare the graphs of the three functions. All three of the quadratic functions are of the form f (x) = ax2+ c. Use a graphing calculator to compare the graphs of the three functions.
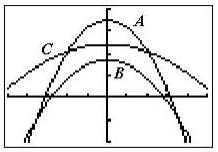
The value of a in the function for Sprinkler A is the largest of the three. Therefore, the parabola for Sprinkler A is the most stretched vertically, so it will expand the least, and therefore have the narrowest path.
Conclusion:
The parabola for Sprinkler A is the most stretched vertically, so it will expand the least, and therefore have the narrowest path.
Chapter 9 Solutions
Glencoe Algebra 1, Student Edition, 9780079039897, 0079039898, 2018
Ch. 9.1 - Prob. 1GPCh. 9.1 - Prob. 2AGPCh. 9.1 - Prob. 2BGPCh. 9.1 - Prob. 3AGPCh. 9.1 - Prob. 3BGPCh. 9.1 - Prob. 4AGPCh. 9.1 - Prob. 4BGPCh. 9.1 - Prob. 4CGPCh. 9.1 - Prob. 5AGPCh. 9.1 - Prob. 5BGP
Ch. 9.1 - Prob. 6GPCh. 9.1 - Prob. 1CYUCh. 9.1 - Prob. 2CYUCh. 9.1 - Prob. 3CYUCh. 9.1 - Prob. 4CYUCh. 9.1 - Prob. 5CYUCh. 9.1 - Prob. 6CYUCh. 9.1 - Prob. 7CYUCh. 9.1 - Prob. 8CYUCh. 9.1 - Prob. 9CYUCh. 9.1 - Prob. 10CYUCh. 9.1 - Prob. 11CYUCh. 9.1 - Prob. 12CYUCh. 9.1 - Prob. 13CYUCh. 9.1 - Prob. 14CYUCh. 9.1 - Prob. 15CYUCh. 9.1 - Prob. 16CYUCh. 9.1 - Prob. 17CYUCh. 9.1 - Prob. 18CYUCh. 9.1 - Prob. 19CYUCh. 9.1 - Prob. 20CYUCh. 9.1 - Prob. 21CYUCh. 9.1 - Prob. 22PPSCh. 9.1 - Prob. 23PPSCh. 9.1 - Prob. 24PPSCh. 9.1 - Prob. 25PPSCh. 9.1 - Prob. 26PPSCh. 9.1 - Prob. 27PPSCh. 9.1 - Prob. 28PPSCh. 9.1 - Prob. 29PPSCh. 9.1 - Prob. 30PPSCh. 9.1 - Prob. 31PPSCh. 9.1 - Prob. 32PPSCh. 9.1 - Prob. 33PPSCh. 9.1 - Prob. 34PPSCh. 9.1 - Prob. 35PPSCh. 9.1 - Prob. 36PPSCh. 9.1 - Prob. 37PPSCh. 9.1 - Prob. 38PPSCh. 9.1 - Prob. 39PPSCh. 9.1 - Prob. 40PPSCh. 9.1 - Prob. 41PPSCh. 9.1 - Prob. 42PPSCh. 9.1 - Prob. 43PPSCh. 9.1 - Prob. 44PPSCh. 9.1 - Prob. 45PPSCh. 9.1 - Prob. 46PPSCh. 9.1 - Prob. 47PPSCh. 9.1 - Prob. 48PPSCh. 9.1 - Prob. 49PPSCh. 9.1 - Prob. 50PPSCh. 9.1 - Prob. 51PPSCh. 9.1 - Prob. 52PPSCh. 9.1 - Prob. 53PPSCh. 9.1 - Prob. 54PPSCh. 9.1 - Prob. 55PPSCh. 9.1 - Prob. 56PPSCh. 9.1 - Prob. 57PPSCh. 9.1 - Prob. 58PPSCh. 9.1 - Prob. 59PPSCh. 9.1 - Prob. 60PPSCh. 9.1 - Prob. 61PPSCh. 9.1 - Prob. 62PPSCh. 9.1 - Prob. 63PPSCh. 9.1 - Prob. 64PPSCh. 9.1 - Prob. 65PPSCh. 9.1 - Prob. 66PPSCh. 9.1 - Prob. 67PPSCh. 9.1 - Prob. 68HPCh. 9.1 - Prob. 69HPCh. 9.1 - Prob. 70HPCh. 9.1 - Prob. 71HPCh. 9.1 - Prob. 72HPCh. 9.1 - Prob. 73HPCh. 9.1 - Prob. 74HPCh. 9.1 - Prob. 75PFACh. 9.1 - Prob. 76PFACh. 9.1 - Prob. 77PFACh. 9.1 - Prob. 78PFACh. 9.1 - Prob. 79PFACh. 9.1 - Prob. 80PFACh. 9.2 - Prob. 1AGPCh. 9.2 - Prob. 1BGPCh. 9.2 - Prob. 1CGPCh. 9.2 - Prob. 1DGPCh. 9.2 - Prob. 2AGPCh. 9.2 - Prob. 2BGPCh. 9.2 - Prob. 3AGPCh. 9.2 - Prob. 3BGPCh. 9.2 - Prob. 4AGPCh. 9.2 - Prob. 4BGPCh. 9.2 - Prob. 5AGPCh. 9.2 - Prob. 5BGPCh. 9.2 - Prob. 5CGPCh. 9.2 - Prob. 6GPCh. 9.2 - Prob. 7GPCh. 9.2 - Prob. 8AGPCh. 9.2 - Prob. 8BGPCh. 9.2 - Prob. 8CGPCh. 9.2 - Prob. 1CYUCh. 9.2 - Prob. 2CYUCh. 9.2 - Prob. 3CYUCh. 9.2 - Prob. 4CYUCh. 9.2 - Prob. 5CYUCh. 9.2 - Prob. 6CYUCh. 9.2 - Prob. 7CYUCh. 9.2 - Prob. 8CYUCh. 9.2 - Prob. 9CYUCh. 9.2 - Prob. 10PPSCh. 9.2 - Prob. 11PPSCh. 9.2 - Prob. 12PPSCh. 9.2 - Prob. 13PPSCh. 9.2 - Prob. 14PPSCh. 9.2 - Prob. 15PPSCh. 9.2 - Prob. 16PPSCh. 9.2 - Prob. 17PPSCh. 9.2 - Prob. 18PPSCh. 9.2 - Prob. 19PPSCh. 9.2 - Prob. 20PPSCh. 9.2 - Prob. 21PPSCh. 9.2 - Prob. 22PPSCh. 9.2 - Prob. 23PPSCh. 9.2 - Prob. 24PPSCh. 9.2 - Prob. 25PPSCh. 9.2 - Prob. 26PPSCh. 9.2 - Prob. 27PPSCh. 9.2 - Prob. 28PPSCh. 9.2 - Prob. 29PPSCh. 9.2 - Prob. 30PPSCh. 9.2 - Prob. 31PPSCh. 9.2 - Prob. 32PPSCh. 9.2 - Prob. 33PPSCh. 9.2 - Prob. 34PPSCh. 9.2 - Prob. 35PPSCh. 9.2 - Prob. 36PPSCh. 9.2 - Prob. 37PPSCh. 9.2 - Prob. 38PPSCh. 9.2 - Prob. 39PPSCh. 9.2 - Prob. 40PPSCh. 9.2 - Prob. 41PPSCh. 9.2 - Prob. 42HPCh. 9.2 - Prob. 43HPCh. 9.2 - Prob. 44HPCh. 9.2 - Prob. 45HPCh. 9.2 - Prob. 46HPCh. 9.2 - Prob. 47PFACh. 9.2 - Prob. 48PFACh. 9.2 - Prob. 49PFACh. 9.2 - Prob. 50PFACh. 9.2 - Prob. 51PFACh. 9.3 - Prob. 1AGPCh. 9.3 - Prob. 1BGPCh. 9.3 - Prob. 2AGPCh. 9.3 - Prob. 2BGPCh. 9.3 - Prob. 3AGPCh. 9.3 - Prob. 3BGPCh. 9.3 - Prob. 5GPCh. 9.3 - Prob. 6GPCh. 9.3 - Prob. 1CYUCh. 9.3 - Prob. 2CYUCh. 9.3 - Prob. 3CYUCh. 9.3 - Prob. 4CYUCh. 9.3 - Prob. 5CYUCh. 9.3 - Prob. 6CYUCh. 9.3 - Prob. 7CYUCh. 9.3 - Prob. 8CYUCh. 9.3 - Prob. 9CYUCh. 9.3 - Prob. 10PPSCh. 9.3 - Prob. 11PPSCh. 9.3 - Prob. 12PPSCh. 9.3 - Prob. 13PPSCh. 9.3 - Prob. 14PPSCh. 9.3 - Prob. 15PPSCh. 9.3 - Prob. 16PPSCh. 9.3 - Prob. 17PPSCh. 9.3 - Prob. 18PPSCh. 9.3 - Prob. 19PPSCh. 9.3 - Prob. 20PPSCh. 9.3 - Prob. 21PPSCh. 9.3 - Prob. 22PPSCh. 9.3 - Prob. 23PPSCh. 9.3 - Prob. 24PPSCh. 9.3 - Prob. 25PPSCh. 9.3 - Prob. 26PPSCh. 9.3 - Prob. 27PPSCh. 9.3 - Prob. 28PPSCh. 9.3 - Prob. 29PPSCh. 9.3 - Prob. 30PPSCh. 9.3 - Prob. 31PPSCh. 9.3 - Prob. 32PPSCh. 9.3 - Prob. 33PPSCh. 9.3 - Prob. 34PPSCh. 9.3 - Prob. 35PPSCh. 9.3 - Prob. 36PPSCh. 9.3 - Prob. 37PPSCh. 9.3 - Prob. 38PPSCh. 9.3 - Prob. 39PPSCh. 9.3 - Prob. 40PPSCh. 9.3 - Prob. 41HPCh. 9.3 - Prob. 42HPCh. 9.3 - Prob. 43HPCh. 9.3 - Prob. 44HPCh. 9.3 - Prob. 45PFACh. 9.3 - Prob. 46PFACh. 9.3 - Prob. 47PFACh. 9.3 - Prob. 48PFACh. 9.3 - Prob. 49PFACh. 9.3 - Prob. 50PFACh. 9.4 - Prob. 1AGPCh. 9.4 - Prob. 1BGPCh. 9.4 - Prob. 1CGPCh. 9.4 - Prob. 2GPCh. 9.4 - Prob. 3AGPCh. 9.4 - Prob. 3BGPCh. 9.4 - Prob. 3CGPCh. 9.4 - Prob. 4AGPCh. 9.4 - Prob. 4BGPCh. 9.4 - Prob. 4CGPCh. 9.4 - Prob. 5AGPCh. 9.4 - Prob. 5BGPCh. 9.4 - Prob. 5CGPCh. 9.4 - Prob. 1CYUCh. 9.4 - Prob. 2CYUCh. 9.4 - Prob. 3CYUCh. 9.4 - Prob. 4CYUCh. 9.4 - Prob. 5CYUCh. 9.4 - Prob. 6CYUCh. 9.4 - Prob. 7CYUCh. 9.4 - Prob. 8CYUCh. 9.4 - Prob. 9CYUCh. 9.4 - Prob. 10CYUCh. 9.4 - Prob. 11CYUCh. 9.4 - Prob. 12CYUCh. 9.4 - Prob. 13CYUCh. 9.4 - Prob. 14CYUCh. 9.4 - Prob. 15CYUCh. 9.4 - Prob. 16CYUCh. 9.4 - Prob. 17PPSCh. 9.4 - Prob. 18PPSCh. 9.4 - Prob. 19PPSCh. 9.4 - Prob. 20PPSCh. 9.4 - Prob. 21PPSCh. 9.4 - Prob. 22PPSCh. 9.4 - Prob. 23PPSCh. 9.4 - Prob. 24PPSCh. 9.4 - Prob. 25PPSCh. 9.4 - Prob. 26PPSCh. 9.4 - Prob. 27PPSCh. 9.4 - Prob. 28PPSCh. 9.4 - Prob. 29PPSCh. 9.4 - Prob. 30PPSCh. 9.4 - Prob. 31PPSCh. 9.4 - Prob. 32PPSCh. 9.4 - Prob. 33PPSCh. 9.4 - Prob. 34PPSCh. 9.4 - Prob. 35PPSCh. 9.4 - Prob. 36PPSCh. 9.4 - Prob. 37PPSCh. 9.4 - Prob. 38PPSCh. 9.4 - Prob. 39PPSCh. 9.4 - Prob. 40PPSCh. 9.4 - Prob. 41PPSCh. 9.4 - Prob. 42PPSCh. 9.4 - Prob. 43PPSCh. 9.4 - Prob. 44PPSCh. 9.4 - Prob. 45PPSCh. 9.4 - Prob. 46PPSCh. 9.4 - Prob. 47PPSCh. 9.4 - Prob. 48PPSCh. 9.4 - Prob. 49PPSCh. 9.4 - Prob. 50HPCh. 9.4 - Prob. 51HPCh. 9.4 - Prob. 52HPCh. 9.4 - Prob. 53HPCh. 9.4 - Prob. 54HPCh. 9.4 - Prob. 55HPCh. 9.4 - Prob. 56HPCh. 9.4 - Prob. 57HPCh. 9.4 - Prob. 58HPCh. 9.4 - Prob. 59HPCh. 9.4 - Prob. 60HPCh. 9.4 - Prob. 61HPCh. 9.4 - Prob. 62PFACh. 9.4 - Prob. 63PFACh. 9.4 - Prob. 64PFACh. 9.4 - Prob. 65PFACh. 9.4 - Prob. 66PFACh. 9.4 - Prob. 67PFACh. 9.4 - Prob. 68PFACh. 9.5 - Prob. 1GPCh. 9.5 - Prob. 2GPCh. 9.5 - Prob. 3GPCh. 9.5 - Prob. 4GPCh. 9.5 - Prob. 5GPCh. 9.5 - Prob. 1CYUCh. 9.5 - Prob. 2CYUCh. 9.5 - Prob. 3CYUCh. 9.5 - Prob. 4CYUCh. 9.5 - Prob. 5CYUCh. 9.5 - Prob. 6CYUCh. 9.5 - Prob. 7CYUCh. 9.5 - Prob. 8CYUCh. 9.5 - Prob. 9CYUCh. 9.5 - Prob. 10CYUCh. 9.5 - Prob. 11CYUCh. 9.5 - Prob. 12CYUCh. 9.5 - Prob. 13PPSCh. 9.5 - Prob. 14PPSCh. 9.5 - Prob. 15PPSCh. 9.5 - Prob. 16PPSCh. 9.5 - Prob. 17PPSCh. 9.5 - Prob. 18PPSCh. 9.5 - Prob. 19PPSCh. 9.5 - Prob. 20PPSCh. 9.5 - Prob. 21PPSCh. 9.5 - Prob. 22PPSCh. 9.5 - Prob. 23PPSCh. 9.5 - Prob. 24PPSCh. 9.5 - Prob. 25PPSCh. 9.5 - Prob. 26PPSCh. 9.5 - Prob. 27PPSCh. 9.5 - Prob. 28PPSCh. 9.5 - Prob. 29PPSCh. 9.5 - Prob. 30PPSCh. 9.5 - Prob. 31PPSCh. 9.5 - Prob. 32PPSCh. 9.5 - Prob. 33PPSCh. 9.5 - Prob. 34PPSCh. 9.5 - Prob. 35PPSCh. 9.5 - Prob. 36PPSCh. 9.5 - Prob. 37PPSCh. 9.5 - Prob. 38PPSCh. 9.5 - Prob. 39PPSCh. 9.5 - Prob. 40PPSCh. 9.5 - Prob. 41PPSCh. 9.5 - Prob. 42HPCh. 9.5 - Prob. 43HPCh. 9.5 - Prob. 44HPCh. 9.5 - Prob. 45HPCh. 9.5 - Prob. 46PFACh. 9.5 - Prob. 47PFACh. 9.5 - Prob. 48PFACh. 9.5 - Prob. 49PFACh. 9.5 - Prob. 50PFACh. 9.5 - Prob. 51PFACh. 9.5 - Prob. 52PFACh. 9.5 - Prob. 53PFACh. 9.6 - Prob. 1AGPCh. 9.6 - Prob. 1BGPCh. 9.6 - Prob. 1CGPCh. 9.6 - Prob. 1DGPCh. 9.6 - Prob. 2AGPCh. 9.6 - Prob. 2BGPCh. 9.6 - Prob. 3AGPCh. 9.6 - Prob. 3BGPCh. 9.6 - Prob. 4AGPCh. 9.6 - Prob. 4BGPCh. 9.6 - Prob. 1CYUCh. 9.6 - Prob. 2CYUCh. 9.6 - Prob. 3CYUCh. 9.6 - Prob. 4CYUCh. 9.6 - Prob. 5CYUCh. 9.6 - Prob. 6CYUCh. 9.6 - Prob. 7CYUCh. 9.6 - Prob. 8CYUCh. 9.6 - Prob. 9CYUCh. 9.6 - Prob. 10CYUCh. 9.6 - Prob. 11CYUCh. 9.6 - Prob. 12CYUCh. 9.6 - Prob. 13CYUCh. 9.6 - Prob. 14CYUCh. 9.6 - Prob. 15CYUCh. 9.6 - Prob. 16PPSCh. 9.6 - Prob. 17PPSCh. 9.6 - Prob. 18PPSCh. 9.6 - Prob. 19PPSCh. 9.6 - Prob. 20PPSCh. 9.6 - Prob. 21PPSCh. 9.6 - Prob. 22PPSCh. 9.6 - Prob. 23PPSCh. 9.6 - Prob. 24PPSCh. 9.6 - Prob. 25PPSCh. 9.6 - Prob. 26PPSCh. 9.6 - Prob. 27PPSCh. 9.6 - Prob. 28PPSCh. 9.6 - Prob. 29PPSCh. 9.6 - Prob. 30PPSCh. 9.6 - Prob. 31PPSCh. 9.6 - Prob. 32PPSCh. 9.6 - Prob. 33PPSCh. 9.6 - Prob. 34PPSCh. 9.6 - Prob. 35PPSCh. 9.6 - Prob. 36PPSCh. 9.6 - Prob. 37PPSCh. 9.6 - Prob. 38PPSCh. 9.6 - Prob. 39PPSCh. 9.6 - Prob. 40PPSCh. 9.6 - Prob. 41PPSCh. 9.6 - Prob. 42PPSCh. 9.6 - Prob. 43PPSCh. 9.6 - Prob. 44PPSCh. 9.6 - Prob. 45PPSCh. 9.6 - Prob. 46PPSCh. 9.6 - Prob. 47PPSCh. 9.6 - Prob. 48PPSCh. 9.6 - Prob. 49PPSCh. 9.6 - Prob. 50PPSCh. 9.6 - Prob. 51HPCh. 9.6 - Prob. 52HPCh. 9.6 - Prob. 53HPCh. 9.6 - Prob. 54HPCh. 9.6 - Prob. 55HPCh. 9.6 - Prob. 56HPCh. 9.6 - Prob. 57HPCh. 9.6 - Prob. 58HPCh. 9.6 - Prob. 59PFACh. 9.6 - Prob. 60PFACh. 9.6 - Prob. 61PFACh. 9.6 - Prob. 62PFACh. 9.6 - Prob. 63PFACh. 9.6 - Prob. 64PFACh. 9.7 - Prob. 1GPCh. 9.7 - Prob. 2GPCh. 9.7 - Prob. 3GPCh. 9.7 - Prob. 4GPCh. 9.7 - Prob. 1CYUCh. 9.7 - Prob. 2CYUCh. 9.7 - Prob. 3CYUCh. 9.7 - Prob. 4CYUCh. 9.7 - Prob. 5CYUCh. 9.7 - Prob. 6CYUCh. 9.7 - Prob. 7CYUCh. 9.7 - Prob. 8CYUCh. 9.7 - Prob. 9CYUCh. 9.7 - Prob. 10PPSCh. 9.7 - Prob. 11PPSCh. 9.7 - Prob. 12PPSCh. 9.7 - Prob. 13PPSCh. 9.7 - Prob. 14PPSCh. 9.7 - Prob. 15PPSCh. 9.7 - Prob. 16PPSCh. 9.7 - Prob. 17PPSCh. 9.7 - Prob. 18PPSCh. 9.7 - Prob. 19PPSCh. 9.7 - Prob. 20PPSCh. 9.7 - Prob. 21PPSCh. 9.7 - Prob. 22PPSCh. 9.7 - Prob. 23PPSCh. 9.7 - Prob. 24PPSCh. 9.7 - Prob. 25PPSCh. 9.7 - Prob. 26PPSCh. 9.7 - Prob. 27PPSCh. 9.7 - Prob. 28HPCh. 9.7 - Prob. 29HPCh. 9.7 - Prob. 30HPCh. 9.7 - Prob. 31HPCh. 9.7 - Prob. 32HPCh. 9.7 - Prob. 33HPCh. 9.7 - Prob. 34PFACh. 9.7 - Prob. 35PFACh. 9.7 - Prob. 36PFACh. 9.7 - Prob. 37PFACh. 9.7 - Prob. 38PFACh. 9.7 - Prob. 39PFACh. 9.7 - Prob. 40PFACh. 9.8 - Prob. 1AGPCh. 9.8 - Prob. 1BGPCh. 9.8 - Prob. 2AGPCh. 9.8 - Prob. 2BGPCh. 9.8 - Prob. 3AGPCh. 9.8 - Prob. 3BGPCh. 9.8 - Prob. 4GPCh. 9.8 - Prob. 1CYUCh. 9.8 - Prob. 2CYUCh. 9.8 - Prob. 3CYUCh. 9.8 - Prob. 4CYUCh. 9.8 - Prob. 5CYUCh. 9.8 - Prob. 6CYUCh. 9.8 - Prob. 7CYUCh. 9.8 - Prob. 8CYUCh. 9.8 - Prob. 9CYUCh. 9.8 - Prob. 10CYUCh. 9.8 - Prob. 11CYUCh. 9.8 - Prob. 12CYUCh. 9.8 - Prob. 13CYUCh. 9.8 - Prob. 14PPSCh. 9.8 - Prob. 15PPSCh. 9.8 - Prob. 16PPSCh. 9.8 - Prob. 17PPSCh. 9.8 - Prob. 18PPSCh. 9.8 - Prob. 19PPSCh. 9.8 - Prob. 20PPSCh. 9.8 - Prob. 21PPSCh. 9.8 - Prob. 22PPSCh. 9.8 - Prob. 23PPSCh. 9.8 - Prob. 24PPSCh. 9.8 - Prob. 25PPSCh. 9.8 - Prob. 26PPSCh. 9.8 - Prob. 27PPSCh. 9.8 - Prob. 28PPSCh. 9.8 - Prob. 29PPSCh. 9.8 - Prob. 30PPSCh. 9.8 - Prob. 31HPCh. 9.8 - Prob. 32HPCh. 9.8 - Prob. 33HPCh. 9.8 - Prob. 34HPCh. 9.8 - Prob. 35HPCh. 9.8 - Prob. 36PFACh. 9.8 - Prob. 37PFACh. 9.8 - Prob. 38PFACh. 9.8 - Prob. 39PFACh. 9.8 - Prob. 40PFACh. 9.8 - Prob. 41PFACh. 9.9 - Prob. 1AGPCh. 9.9 - Prob. 1BGPCh. 9.9 - Prob. 2AGPCh. 9.9 - Prob. 2BGPCh. 9.9 - Prob. 3AGPCh. 9.9 - Prob. 3BGPCh. 9.9 - Prob. 3CGPCh. 9.9 - Prob. 4AGPCh. 9.9 - Prob. 4BGPCh. 9.9 - Prob. 1CYUCh. 9.9 - Prob. 2CYUCh. 9.9 - Prob. 3CYUCh. 9.9 - Prob. 4CYUCh. 9.9 - Prob. 5CYUCh. 9.9 - Prob. 6CYUCh. 9.9 - Prob. 7CYUCh. 9.9 - Prob. 8CYUCh. 9.9 - Prob. 9CYUCh. 9.9 - Prob. 10CYUCh. 9.9 - Prob. 11CYUCh. 9.9 - Prob. 12PPSCh. 9.9 - Prob. 13PPSCh. 9.9 - Prob. 14PPSCh. 9.9 - Prob. 15PPSCh. 9.9 - Prob. 16PPSCh. 9.9 - Prob. 17PPSCh. 9.9 - Prob. 18PPSCh. 9.9 - Prob. 19PPSCh. 9.9 - Prob. 20PPSCh. 9.9 - Prob. 21PPSCh. 9.9 - Prob. 22PPSCh. 9.9 - Prob. 23PPSCh. 9.9 - Prob. 24PPSCh. 9.9 - Prob. 25PPSCh. 9.9 - Prob. 26PPSCh. 9.9 - Prob. 27PPSCh. 9.9 - Prob. 28PPSCh. 9.9 - Prob. 29PPSCh. 9.9 - Prob. 30PPSCh. 9.9 - Prob. 31PPSCh. 9.9 - Prob. 32PPSCh. 9.9 - Prob. 33PPSCh. 9.9 - Prob. 34PPSCh. 9.9 - Prob. 35PPSCh. 9.9 - Prob. 36PPSCh. 9.9 - Prob. 37PPSCh. 9.9 - Prob. 38PPSCh. 9.9 - Prob. 39PPSCh. 9.9 - Prob. 40PPSCh. 9.9 - Prob. 41PPSCh. 9.9 - Prob. 42PPSCh. 9.9 - Prob. 43PPSCh. 9.9 - Prob. 44PPSCh. 9.9 - Prob. 45PPSCh. 9.9 - Prob. 46PPSCh. 9.9 - Prob. 47PPSCh. 9.9 - Prob. 48HPCh. 9.9 - Prob. 49HPCh. 9.9 - Prob. 50HPCh. 9.9 - Prob. 51HPCh. 9.9 - Prob. 52HPCh. 9.9 - Prob. 53PFACh. 9.9 - Prob. 54PFACh. 9.9 - Prob. 55PFACh. 9.9 - Prob. 56PFACh. 9.9 - Prob. 57PFACh. 9.9 - Prob. 58PFACh. 9.9 - Prob. 59PFACh. 9 - Prob. 1MCQCh. 9 - Prob. 2MCQCh. 9 - Prob. 3MCQCh. 9 - Prob. 4MCQCh. 9 - Prob. 5MCQCh. 9 - Prob. 6MCQCh. 9 - Prob. 7MCQCh. 9 - Prob. 8MCQCh. 9 - Prob. 9MCQCh. 9 - Prob. 10MCQCh. 9 - Prob. 11MCQCh. 9 - Prob. 12MCQCh. 9 - Prob. 13MCQCh. 9 - Prob. 14MCQCh. 9 - Prob. 15MCQCh. 9 - Prob. 16MCQCh. 9 - Prob. 17MCQCh. 9 - Prob. 18MCQCh. 9 - Prob. 19MCQCh. 9 - Prob. 20MCQCh. 9 - Prob. 21MCQCh. 9 - Prob. 22MCQCh. 9 - Prob. 23MCQCh. 9 - Prob. 24MCQCh. 9 - Prob. 25MCQCh. 9 - Prob. 26MCQCh. 9 - Prob. 27MCQCh. 9 - Prob. 1SGRCh. 9 - Prob. 2SGRCh. 9 - Prob. 3SGRCh. 9 - Prob. 4SGRCh. 9 - Prob. 5SGRCh. 9 - Prob. 6SGRCh. 9 - Prob. 7SGRCh. 9 - Prob. 8SGRCh. 9 - Prob. 9SGRCh. 9 - Prob. 10SGRCh. 9 - Prob. 11SGRCh. 9 - Prob. 12SGRCh. 9 - Prob. 13SGRCh. 9 - Prob. 14SGRCh. 9 - Prob. 15SGRCh. 9 - Prob. 16SGRCh. 9 - Prob. 17SGRCh. 9 - Prob. 18SGRCh. 9 - Prob. 19SGRCh. 9 - Prob. 20SGRCh. 9 - Prob. 21SGRCh. 9 - Prob. 22SGRCh. 9 - Prob. 23SGRCh. 9 - Prob. 24SGRCh. 9 - Prob. 25SGRCh. 9 - Prob. 26SGRCh. 9 - Prob. 27SGRCh. 9 - Prob. 28SGRCh. 9 - Prob. 29SGRCh. 9 - Prob. 30SGRCh. 9 - Prob. 31SGRCh. 9 - Prob. 32SGRCh. 9 - Prob. 33SGRCh. 9 - Prob. 34SGRCh. 9 - Prob. 35SGRCh. 9 - Prob. 36SGRCh. 9 - Prob. 37SGRCh. 9 - Prob. 38SGRCh. 9 - Prob. 39SGRCh. 9 - Prob. 40SGRCh. 9 - Prob. 41SGRCh. 9 - Prob. 42SGRCh. 9 - Prob. 43SGRCh. 9 - Prob. 44SGRCh. 9 - Prob. 45SGRCh. 9 - Prob. 46SGRCh. 9 - Prob. 47SGRCh. 9 - Prob. 48SGRCh. 9 - Prob. 49SGRCh. 9 - Prob. 50SGRCh. 9 - Prob. 51SGRCh. 9 - Prob. 52SGRCh. 9 - Prob. 53SGRCh. 9 - Prob. 54SGRCh. 9 - Prob. 55SGRCh. 9 - Prob. 56SGRCh. 9 - Prob. 57SGRCh. 9 - Prob. 58SGRCh. 9 - Prob. 59SGRCh. 9 - Prob. 60SGRCh. 9 - Prob. 61SGRCh. 9 - Prob. 62SGRCh. 9 - Prob. 63SGRCh. 9 - Prob. 64SGRCh. 9 - Prob. 65SGRCh. 9 - Prob. 66SGRCh. 9 - Prob. 67SGRCh. 9 - Prob. 68SGRCh. 9 - Prob. 69SGRCh. 9 - Prob. 70SGRCh. 9 - Prob. 71SGRCh. 9 - Prob. 1PTCh. 9 - Prob. 2PTCh. 9 - Prob. 3PTCh. 9 - Prob. 4PTCh. 9 - Prob. 5PTCh. 9 - Prob. 6PTCh. 9 - Prob. 7PTCh. 9 - Prob. 8PTCh. 9 - Prob. 9PTCh. 9 - Prob. 10PTCh. 9 - Prob. 11PTCh. 9 - Prob. 12PTCh. 9 - Prob. 13PTCh. 9 - Prob. 14PTCh. 9 - Prob. 15PTCh. 9 - Prob. 16PTCh. 9 - Prob. 17PTCh. 9 - Prob. 18PTCh. 9 - Prob. 19PTCh. 9 - Prob. 20PTCh. 9 - Prob. 21PTCh. 9 - Prob. 22PTCh. 9 - Prob. 23PTCh. 9 - Prob. 24PTCh. 9 - Prob. 25PTCh. 9 - Prob. 26PTCh. 9 - Prob. 27PTCh. 9 - Prob. 28PTCh. 9 - Prob. 29PTCh. 9 - Prob. 1PFACh. 9 - Prob. 2PFACh. 9 - Prob. 3PFACh. 9 - Prob. 4PFACh. 9 - Prob. 5PFACh. 9 - Prob. 6PFACh. 9 - Prob. 7PFACh. 9 - Prob. 8PFACh. 9 - Prob. 9PFACh. 9 - Prob. 10PFACh. 9 - Prob. 11PFACh. 9 - Prob. 12PFACh. 9 - Prob. 13PFA
Additional Math Textbook Solutions
Find more solutions based on key concepts
Two dice are thrown. Let E be the event that the sum of the dice is odd, let F be the event that at least one o...
A First Course in Probability (10th Edition)
Fill in each blank so that the resulting statement is true.
1. A combination of numbers, variables, and opera...
College Algebra (7th Edition)
Position, velocity, and acceleration Suppose the position of an object moving horizontally after t seconds is g...
Calculus: Early Transcendentals (2nd Edition)
Explain the meaning of the term “statistically significant difference” in statistics terminology.
Intro Stats, Books a la Carte Edition (5th Edition)
CHECK POINT 1 Find a counterexample to show that the statement The product of two two-digit numbers is a three-...
Thinking Mathematically (6th Edition)
7. Quinela In a horse race, a quinela bet is won if you selected the two horses that finish first and second, a...
Elementary Statistics (13th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- Use the graph of y = f(x) to answer the following. 3- 2 -4 -2 -1 1 2 3 4 -1 2 m -3- + (d) Find all x for which f(x) = -2. If there is more than one value, separate them with commas or write your answer in interval notation, if necessary. Select "None", if applicable. Value(s) of x for which f(x)=-2: | (0,0) (0,0) (0,0) (0,0) 0,0... -00 None (h) Determine the range of f. The range is (0,0) Garrow_forwardWhat is g(f(4))arrow_forward10) Multiply (8m + 3)² A) 8m²+11m+6 B) m² + 48m+9 C) 64m²+48m+9 D) 16m²+11m+6arrow_forward
- Let R be field and X= R³/s Vector space over R M=(a,b,c)labic, e Rra+b= 3- <3 Show that Ms and why with proof. 1) is convexset and affine set of botost ii) is blanced set and symmetirs set of x iii) is hy per space and hyper plane ofx or hot iii) find f:MR st kerf = M 18/103 and finnd fiM→R/{0} st M= {xEX, f(t) = x, texiαER? jiii) show that Mis Maxsubspace or not and Mis a max. affine set or not.arrow_forwardFind The partial fraction decomposition for each The following 2× B) (x+3) a 3 6 X-3x+2x-6arrow_forward1) Find the partial feraction decomposition for each of 5- X 2 2x+x-1 The following: 3 B) 3 X + 3xarrow_forward
- Kate, Luke, Mary and Nancy are sharing a cake. The cake had previously been divided into four slices (s1, s2, s3 and s4). The following table shows the values of the slices in the eyes of each player. What is fair share to nancy? S1 S2 S3 S4 Kate $4.00 $6.00 $6.00 $4.00 Luke $5.30 $5.00 $5.25 $5.45 Mary $4.25 $4.50 $3.50 $3.75 Nancy $6.00 $4.00 $4.00 $6.00arrow_forwardKate, Luke, Mary and Nancy are sharing a cake. The cake had previously been divided into four slices (s1, s2, s3 and s4). The following table shows the values of the slices in the eyes of each player. S1 S2 S3 S4 Kate $4.00 $6.00 $6.00 $4.00 Luke $5.30 $5.00 $5.25 $5.45 Mary $4.25 $4.50 $3.50 $3.75 Nancy $6.00 $4.00 $4.00 $6.00 how much is the cak worth to maryarrow_forwardKate, Luke, Mary and Nancy are sharing a cake. The cake had previously been divided into four slices (s1, s2, s3 and s4). The following table shows the values of the slices in the eyes of each player. What is the threshold of fair share for Luke? S1 S2 S3 S4 Kate $4.00 $6.00 $6.00 $4.00 Luke $5.30 $5.00 $5.25 $5.45 Mary $4.25 $4.50 $3.50 $3.75 Nancy $6.00 $4.00 $4.00 $6.00arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON
Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON
Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education
What is Ellipse?; Author: Don't Memorise;https://www.youtube.com/watch?v=nzwCInIMlU4;License: Standard YouTube License, CC-BY