Chapter 9, Problem 38P

Using Fig. 9.45, design a problem to help other students better understand admittance.

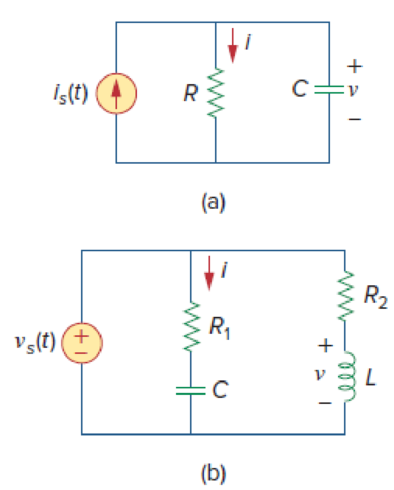
Figure 9.45

To determine

Design a problem to make better understand about the admittance using Figure 9.45.

Explanation of Solution

Problem design:

Determine the value of current $i\left(t\right)$ and the voltage $v\left(t\right)$ in each of the circuits of Figure 9.45.

Formula used:

Write the expression to convert the time domain expression into phasor domain.

$A\cos \left(\omega t+\varphi \right)\iff A\angle \varphi$        (1)

Here,

A is the magnitude,

$\omega$ is the angular frequency,

t is the time, and

$\varphi$ is the phase angle.

Write the expression to calculate the impedance of the passive elements resistor, inductor and capacitor.

$Z_R=R$        (2)

$Z_L=j\omega L$        (3)

$Z_C=\frac{1}{j\omega C}$        (4)

Here,

$R$ is the value of the resistor,

$L$ is the value of the inductor, and

$C$ is the value of the capacitor.

Calculation:

(a)

The Figure 9.45(a) is redrawn as Figure 1 by assuming the values for the respective elements.

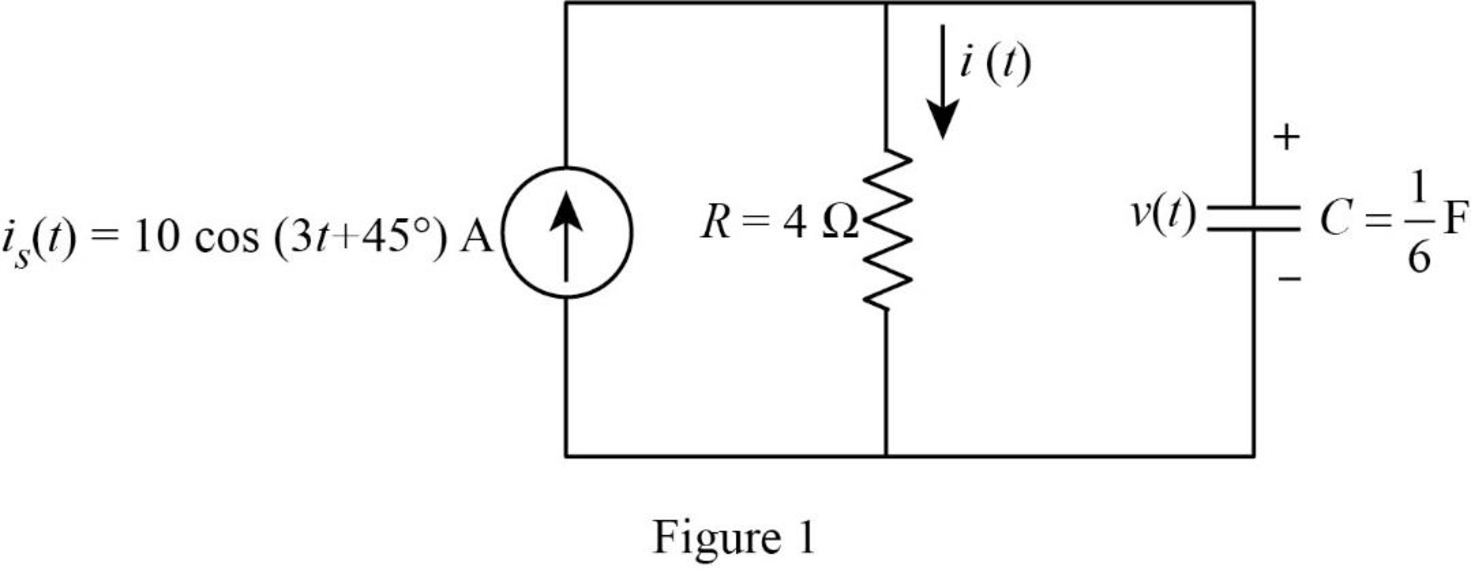
Refer to Figure 1, the current equation is,

$i_s\left(t\right)=10\cos \left(3t+45°\right)\text{A}$

Here, angular frequency $\omega =3\frac{\text{rad}}{\text{s}}$.

Use the equation (1) to express the above equation in phasor form.

$I_s=\left(10\angle 45°\right)\text{A}$

Substitute $4\Omega$ for $R$ in equation (2) to find $Z_R$.

$Z_R=4\Omega$

Substitute $\frac{1}{6}\text{F}$ for $C$ and $3\frac{\text{rad}}{\text{s}}$ for $\omega$ in equation (4) to find $Z_C$.

$\begin{array}{c}{Z}_C=\frac{1}{j\left(3\frac{\text{rad}}{\text{s}}\right)\left(\frac{1}{6}\text{F}\right)}\\ =\frac{1}{j\left(3\right)\left(\frac{1}{6}\right)\frac{\text{rad}}{\text{s}}\cdot \text{F}}\\ =\frac{1}{j\left(\frac{1}{2}\right)\frac{\text{1}}{\text{s}}\cdot \frac{\text{s}}{\Omega }}\left\{\because 1\text{F}=\frac{\text{1}\text{s}}{1\Omega }\right\}\\ =-j2\Omega \end{array}$

The Figure 1 is redrawn as impedance circuit in the following Figure 2.

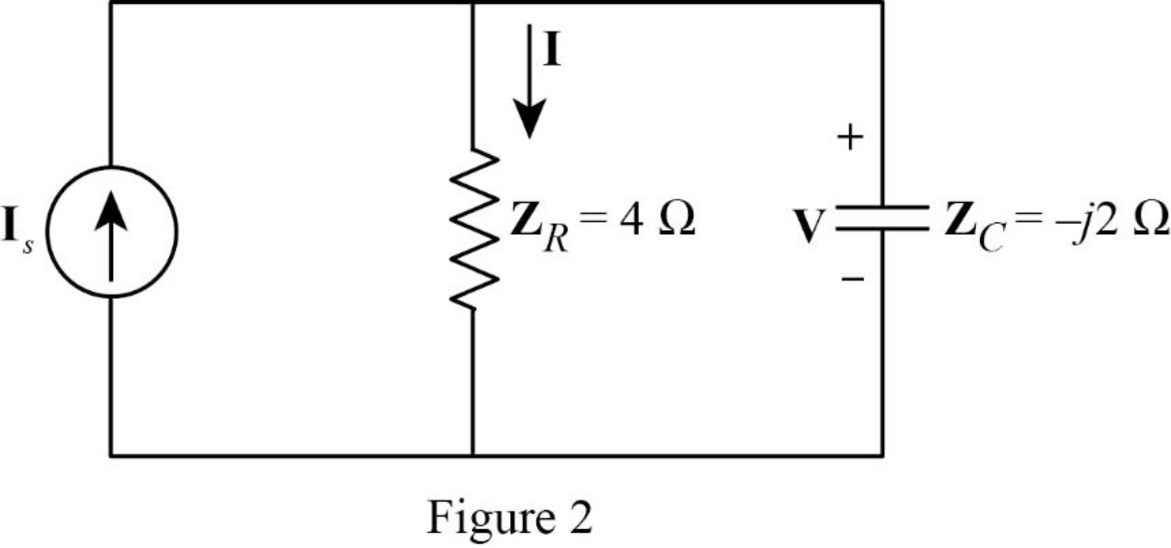
Apply current division rule on Figure 2 to find $I$.

$I=\frac{-j2\Omega }{4\Omega -j2\Omega }\left(I_s\right)$

Substitute $\left(10\angle 45°\right)\text{A}$ for $I_s$ in above equation to find $I$.

$\begin{array}{c}I=\frac{-j2\Omega }{4\Omega -j2\Omega }\left(\left(10\angle 45°\right)\text{A}\right)\\ =\left(0.4472\angle -63.435°\right)\left(\left(10\angle 45°\right)\text{A}\right)\\ =\left(4.472\angle -18.43°\right)\text{A}\end{array}$

Use the equation (1) to express the above equation in time domain form.

$i\left(t\right)=4.472\cos \left(\omega t-18.43°\right)\text{A}$

Substitute $3$ for $\omega$ in above equation to find $i\left(t\right)$.

$\begin{array}{c}i\left(t\right)=4.472\cos \left(\left(3\right)t-18.43°\right)\text{A}\\ =4.472\cos \left(3t-18.43°\right)\text{A}\end{array}$

Refer to Figure 2, the voltage across the impedance $Z_R$ is expressed as,

$V=\left(4\Omega \right)I$

Substitute $\left(4.472\angle -18.43°\right)\text{A}$ for $I$ in above equation to find $V$.

$\begin{array}{c}V=\left(4\Omega \right)\left(4.472\angle -18.43°\right)\text{A}\\ =\left(17.89\angle -18.43°\right)\text{V}\end{array}$

Use the equation (1) to express the above equation in time domain form.

$v\left(t\right)=17.89\cos \left(\omega t-18.43°\right)\text{V}$

Substitute $3$ for $\omega$ in above equation to find $v\left(t\right)$.

$\begin{array}{c}v\left(t\right)=17.89\cos \left(\left(3\right)t-18.43°\right)\text{V}\\ =17.89\cos \left(3t-18.43°\right)\text{V}\end{array}$

Therefore, the value of current $i\left(t\right)$ and the voltage $v\left(t\right)$ in Figure 9.45(a) is $4.472\cos \left(3t-18.43°\right)\text{A}$ and $17.89\cos \left(3t-18.43°\right)\text{V}$ respectively.

(b)

The Figure 9.45(b) is redrawn as Figure 3 by assuming the values for the respective elements.

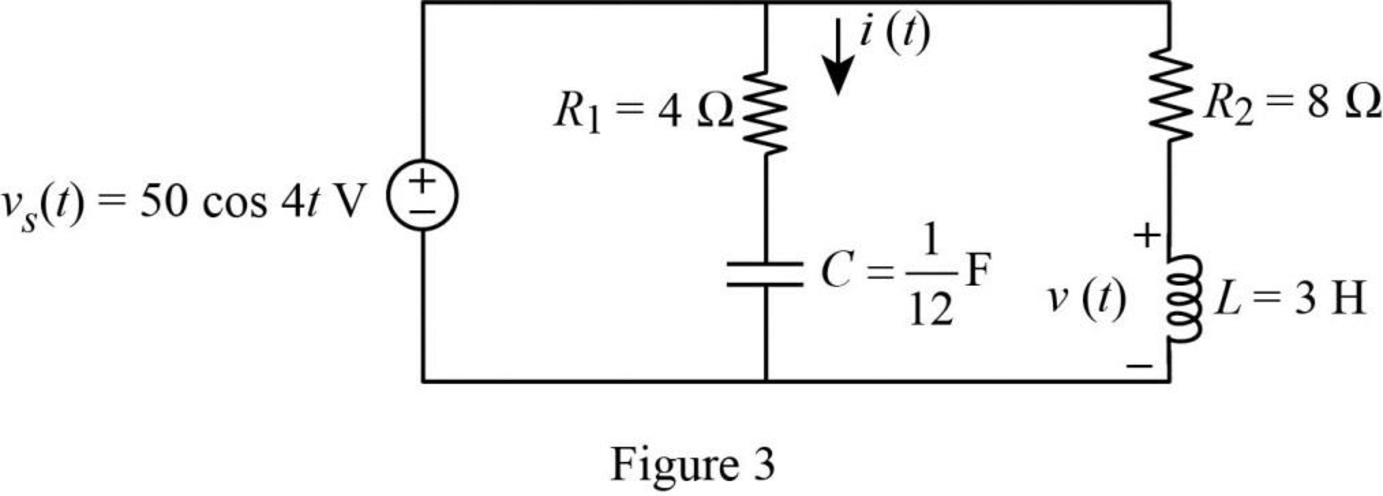
Refer to Figure 3, the voltage equation is,

$v_s\left(t\right)=50\cos 4t\text{V}$

Here, angular frequency $\omega =4\frac{\text{rad}}{\text{s}}$.

Use the equation (1) to express the above equation in phasor form.

$V_s=\left(50\angle 0°\right)\text{V}$

Substitute $4\Omega$ for $R_1$ in equation (2) to find $Z_{R_1}$.

$Z_{R_1}=4\Omega$

Substitute $8\Omega$ for $R_2$ in equation (2) to find $Z_{R_2}$.

$Z_{R_2}=8\Omega$

Substitute $3\text{H}$ for $L$ and $4\frac{\text{rad}}{\text{s}}$ for $\omega$ in equation (3) to find $Z_L$.

$\begin{array}{c}{Z}_L=j\left(4\frac{\text{rad}}{\text{s}}\right)\left(3\text{H}\right)\\ =j\left(4\frac{\text{1}}{\text{s}}\right)\left(3\Omega \cdot \text{s}\right)\left\{\because 1\text{H}=1\Omega \cdot 1\text{s}\right\}\\ =j12\Omega \end{array}$

Substitute $\frac{1}{12}\text{F}$ for $C$ and $4\frac{\text{rad}}{\text{s}}$ for $\omega$ in equation (4) to find $Z_C$.

$\begin{array}{c}{Z}_C=\frac{1}{j\left(4\frac{\text{rad}}{\text{s}}\right)\left(\frac{1}{12}\text{F}\right)}\\ =\frac{1}{j\left(4\right)\left(\frac{1}{12}\right)\frac{\text{rad}}{\text{s}}\cdot \text{F}}\\ =\frac{1}{j\left(\frac{1}{3}\right)\frac{\text{1}}{\text{s}}\cdot \frac{\text{s}}{\Omega }}\left\{\because 1\text{F}=\frac{\text{1}\text{s}}{1\Omega }\right\}\\ =-j3\Omega \end{array}$

The Figure 3 is redrawn as impedance circuit in the following Figure 4.

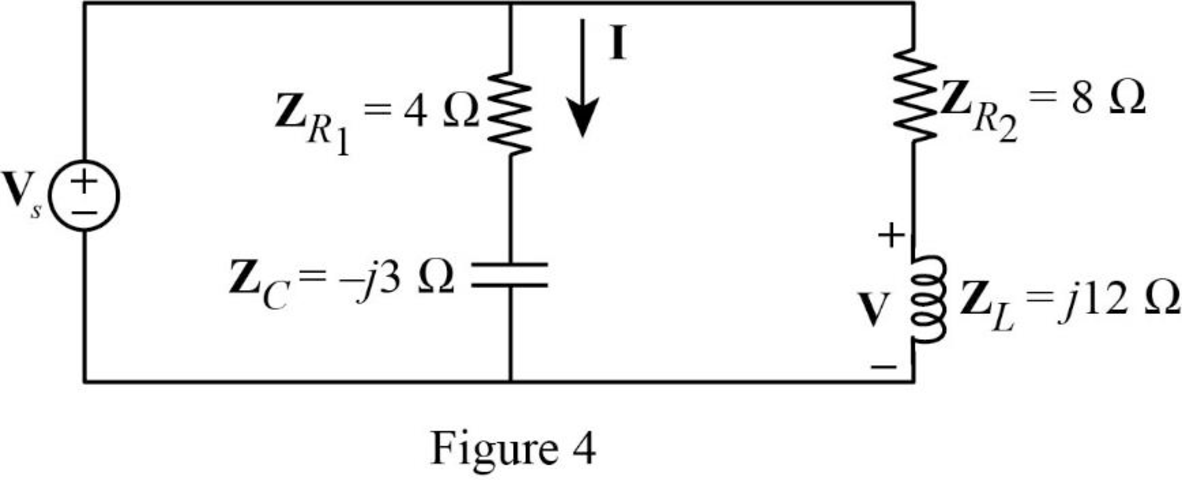
Refer to Figure 4, the impedances $Z_{R_1}$ and $Z_C$ are connected in series form.

Write the expression to calculate the equivalent impedance of the series connected impedances $Z_{R_1}$ and $Z_C$.

$Z_{\text{eq}}=Z_{R_1}+Z_C$

Refer to Figure 4, the source voltage $V_s$ is connected in parallel with the series connections of impedances $Z_{R_1}$ and $Z_C$. For parallel connection, the voltage is same. The current $I$ is passing through the impedance $Z_{\text{eq}}$.

Write the expression to calculate the current $I$.

$I=\frac{V_s}{Z_{\text{eq}}}$

Substitute $Z_{R_1}+Z_C$ for $Z_{\text{eq}}$ in above equation to find $I$.

$I=\frac{V_s}{Z_{R_1}+Z_C}$

Substitute $\left(50\angle 0°\right)\text{V}$ for $V_s$, $4\Omega$ for $Z_{R_1}$ and $-j3\Omega$ for $Z_C$ in above equation to find $I$.

$\begin{array}{c}I=\frac{\left(50\angle 0°\right)\text{V}}{4\Omega -j3\Omega }\\ =\frac{\left(50\angle 0°\right)\text{V}}{\left(4-j3\right)\Omega }\\ =\frac{\left(50\angle 0°\right)\text{V}}{\left(5\angle -36.87°\right)\Omega }\\ =\left(10\angle 36.87°\right)\text{A}\end{array}$

Use the equation (1) to express the above equation in time domain form.

$i\left(t\right)=10\cos \left(\omega t+36.87°\right)\text{A}$

Substitute $4$ for $\omega$ in above equation to find $i\left(t\right)$.

$\begin{array}{c}i\left(t\right)=10\cos \left(\left(4\right)t+36.87°\right)\text{A}\\ =10\cos \left(4t+36.87°\right)\text{A}\end{array}$

Apply voltage division rule on Figure 4 to find $V$.

$\begin{array}{c}V=\frac{j12\Omega }{8\Omega +j12\Omega }{V}_s\\ =\frac{j12\Omega }{\left(8+j12\right)\Omega }{V}_s\\ =\left(0.832\angle 33.69°\right)V_s\end{array}$

Substitute $\left(50\angle 0°\right)\text{V}$ for $V_s$ in above equation to find $V$.

$\begin{array}{c}V=\left(0.832\angle 33.69°\right)\left(\left(50\angle 0°\right)\text{V}\right)\\ =\left(41.6\angle 33.69°\right)\text{V}\end{array}$

Use the equation (1) to express the above equation in time domain form.

$v\left(t\right)=41.6\cos \left(\omega t+33.69°\right)\text{V}$

Substitute $4$ for $\omega$ in above equation to find $v\left(t\right)$.

$\begin{array}{c}v\left(t\right)=41.6\cos \left(\left(4\right)t+33.69°\right)\text{V}\\ =41.6\cos \left(4t+33.69°\right)\text{V}\end{array}$

Therefore, the value of current $i\left(t\right)$ and the voltage $v\left(t\right)$ in Figure 9.45(b) is $10\cos \left(4t+36.87°\right)\text{A}$ and $41.6\cos \left(4t+33.69°\right)\text{V}$ respectively.

Conclusion:

Thus, the problem to make better understand about the admittance using Figure 9.45 is designed.