Chapter 8.5, Problem 20E

To determine

To write and graph:

An equation giving the distancey (in feet) that the robot after x weeks. Use the graph to estimate how quickly the robot could reach the end of the tunnel?

Answer to Problem 20E

Our required equation would be $y=210-10x$ .

Therobot will reach the end of tunnel after 21 minutes.

Explanation of Solution

Given:

In 2002, a robot explored a tunnel 210 feet long inside the Great Pyramid inEgypt. The robot could travel about 10 feet per minute.

Concept used:

We know that slope-intercept form of an equation is $y=mx+b$ , where m represents slope and b represents the y -intercept.

Calculation:

Let x represent number of minutes and y represent distance in feet.

We have been given that robot could travel about 10 feet per minute, so total distance traveled in xminutes would be $10x$ .

The distance inside tunnel left after xminutes would be total length of tunnel minus $10x$ . We can represent our given information in an equation as:

$y=210-10x$

Therefore, our required equation would be $y=210-10x$ .

Upon graphing our given equation, we will get our required graph as shown below:

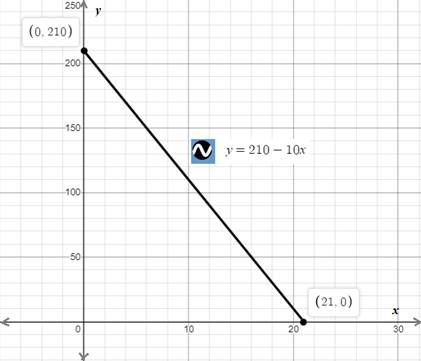
Upon looking at our graph, we can see that at $x=21$ , the distance is 0. Therefore, the robot will reach the end of tunnel after 21 minutes.