Chapter 8, Problem 10RE

Solution

To find: The center, vertices and foci of the identified conic $\frac{{\left(y-3\right)}^2}{9}-\frac{{\left(x-7\right)}^2}{12}=1$ and sketch its graph.

Hyperbola; Center: $\left(7,3\right)$ ;vertices: $\left(7,6\right),\left(7,0\right)$ ; foci: $\left(7,3\pm \sqrt{21}\right)$ .

Given information:

Equation of conic: $\frac{{\left(y-3\right)}^2}{9}-\frac{{\left(x-7\right)}^2}{12}=1$

Formula used:

The standard equation of the hyperbola is $\frac{{\left(y-k\right)}^2}{a^2}-\frac{{\left(x-h\right)}^2}{b^2}=1$

Center is $\left(h,k\right)$

Foci is $\left(h,k\pm c\right)$

Vertices is $\left(h,k\pm a\right)$

Pythagorean relation: $c^2=a^2+b^2$

Calculation:

The equation $\frac{{\left(y-3\right)}^2}{9}-\frac{{\left(x-7\right)}^2}{12}=1$ is of the form of standard equation of the hyperbola $\frac{{\left(y-k\right)}^2}{a^2}-\frac{{\left(x-h\right)}^2}{b^2}=1$

Substitute $7$ for $h$ and $3$ for $k$ in the center formula.

Center is $\left(7,3\right)$ .

Compare the equation with the standard equation of the hyperbola.

  $\begin{array}{l}{a}^2=9\\ a=3\\ b^2=12\end{array}$

Take square root on both sides of the equation.

  $\begin{array}{c}b=\sqrt{12}\\ =\sqrt{4\times 3}\\ =2\sqrt{3}\end{array}$

Substitute $9$ for $a^2$ and $12$ for $b^2$ in the Pythagorean relation.

  $\begin{array}{c}{c}^2=9+12\\ =21\end{array}$

Take square root on both sides of the equation.

  $c=\sqrt{21}$

Substitute $7$ for $h$ , $\sqrt{21}$ for $c$ and $3$ for $k$ in the foci formula.

Therefore, foci is $\left(7,3\pm \sqrt{21}\right)$ .

Substitute $7$ for $h$ , $3$ for $k$ and $3$ for $a$ in the vertices formula.

Therefore, vertices are

  $\begin{array}{l}\left(7,3+3\right)=\left(7,6\right)\\ \left(7,3-3\right)=\left(7,0\right)\end{array}$ .

Draw the graph as follows:

  

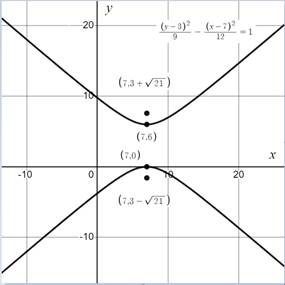
Hence, the given equation of conic is a hyperbola, center is $\left(7,3\right)$ ,vertices are $\left(7,6\right),\left(7,0\right)$ and foci is $\left(7,3\pm \sqrt{21}\right)$ .