
Concept explainers
Two shafts AC and CF, which lie in the vertical xy plane, are connected by a universal joint at C. The bearings at B and D do not exert any axial force. A couple with a magnitude of 500 lb·in. (clockwise when viewed from the positive x axis) is applied to shaft CF at F. At a time when the arm of the crosspiece attached to shaft CF is horizontal, determine (a) the magnitude of the couple that must be applied to shaft AC at A to maintain equilibrium, (b) the reactions at B, D, and E. (Hint: The sum of the couples exerted on the crosspiece must be zero.)
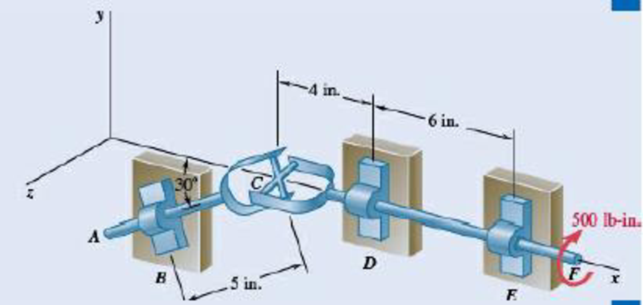
Fig. P6.161
*6.162 Solve Prob. 6.161 assuming that the arm of the crosspiece attached to shaft CF is vertical.
(a)
The magnitude of the couple that must be applied to shaft
Answer to Problem 6.162P
The magnitude of the couple that must be applied to shaft
Explanation of Solution
Take all vectors along the
The free body diagram of the shaft
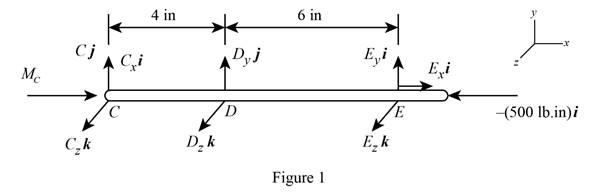
Here,
At equilibrium total moment will be zero.
Write the expression for the equilibrium moment about
Here,
The moment along the
From free body diagram in figure1, write the complete equilibrium expression of moment about
 (II)
(II)
Here,
The free body diagram of the shaft
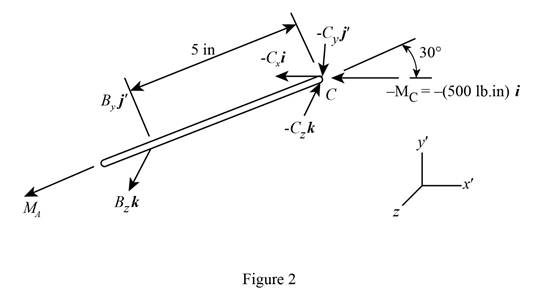
Here,
Write the expression for the equilibrium moment about
Here,
Resolve
From free body diagram in figure2, write the complete equilibrium expression of moment about

Here,
Calculation:
Rearrange equation (II) to get
Equate coefficient of
Therefore, the magnitude of the couple that must be applied to shaft
(b)
The reaction at
Answer to Problem 6.162P
The reaction at
Explanation of Solution
From free body diagram in figure2, write the complete equilibrium expression of moment about

Here,
Since the net force at the shaft
Using free body diagram in figure1, apply the equilibrium condition for moment about
Here,
From figure1, write the complete expression of moment
Since net force at shaft
Calculation:
Equate coefficient of
Equate coefficient of
Therefore,
Substitute
Equate coefficient of
Equate coefficient of
Equate coefficient of
Therefore, net reaction at
Substitute
Equate coefficient of
Therefore, total reaction at
Therefore, the reaction at
Want to see more full solutions like this?
Chapter 6 Solutions
EBK VECTOR MECHANICS FOR ENGINEERS: STA
- Carbon dioxide contained in a piston–cylinder device is compressed from 0.3 to 0.1 m3. During the process, the pressure and volume are related by P = aV–2, where a = 6 kPa·m6. Calculate the work done on carbon dioxide during this process. The work done on carbon dioxide during this process is kJ.arrow_forwardThe volume of 1 kg of helium in a piston–cylinder device is initially 5 m3. Now helium is compressed to 3 m3 while its pressure is maintained constant at 130 kPa. Determine the initial and final temperatures of helium as well as the work required to compress it, in kJ. The gas constant of helium is R = 2.0769 kJ/kg·K. The initial temperature of helium is K. The final temperature of helium is K. The work required to compress helium is kJ.arrow_forwardA piston-cylinder device initially contains 0.4 kg of nitrogen gas at 160 kPa and 140°C. Nitrogen is now expanded isothermally to a pressure of 80 kPa. Determine the boundary work done during this process. The properties of nitrogen are R= 0.2968 kJ/kg-K and k= 1.4. N₂ 160 kPa 140°C The boundary work done during this process is KJ.arrow_forward
- ! Required information An abrasive cutoff wheel has a diameter of 5 in, is 1/16 in thick, and has a 3/4-in bore. The wheel weighs 4.80 oz and runs at 11,700 rev/min. The wheel material is isotropic, with a Poisson's ratio of 0.20, and has an ultimate strength of 12 kpsi. Choose the correct equation from the following options: Multiple Choice о σmax= (314) (4r2 — r²) - о σmax = p² (3+) (4r² + r²) 16 σmax = (314) (4r² + r²) σmax = (314) (4² - r²)arrow_forwardI don't know how to solve thisarrow_forwardI am not able to solve this question. Each part doesn't make sense to me.arrow_forward
- Exercises Find the solution of the following Differential Equations 1) y" + y = 3x² 3) "+2y+3y=27x 5) y"+y=6sin(x) 7) y"+4y+4y = 18 cosh(x) 9) (4)-5y"+4y = 10 cos(x) 11) y"+y=x²+x 13) y"-2y+y=e* 15) y+2y"-y'-2y=1-4x³ 2) y"+2y' + y = x² 4) "+y=-30 sin(4x) 6) y"+4y+3y=sin(x)+2 cos(x) 8) y"-2y+2y= 2e* cos(x) 10) y+y-2y=3e* 12) y"-y=e* 14) y"+y+y=x+4x³ +12x² 16) y"-2y+2y=2e* cos(x)arrow_forwardQu. 15 What are the indices for the Plane 1 drawn in the following sketch? Qu. 16 What are the Miller indices for the Plane shown in the following cubic unit cell? this is material engineering please show all workarrow_forwardI do not understand how to approach this question. I tried to answer it but I kept getting it incorrect.arrow_forward
 International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
International Edition---engineering Mechanics: St...Mechanical EngineeringISBN:9781305501607Author:Andrew Pytel And Jaan KiusalaasPublisher:CENGAGE L
