Calculus Volume 3
1st Edition
ISBN: 9781630182038
Author: Gilbert Strang, Edwin Jed Herman
Publisher: OpenStax College.
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 6.1, Problem 29E
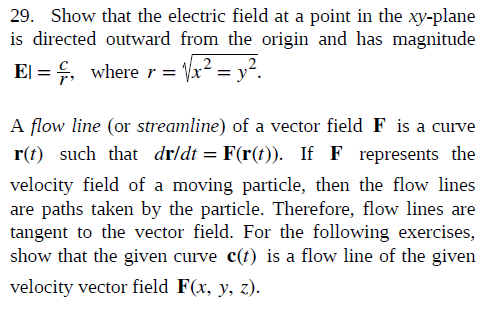
For the following exercises, assume that an electric field in the xy-plane caused by an infinite line of charge along the x-axis is a gradient field with potential function V(x, y) = c In
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
The electric field in the xy-plane due to a point charge at (0,0) is a gradient field with the
where k> 0 is a physical constant. Find the
potential function V(x,y)=
k
√√√x²+x2
components of the electric field where E(x,y)=-VV(x,y)
Find the gradient fields
ƒ(x, y, z) = (x raise to the power 2 + y raise to the power 2 + z raise to the power 2)-1>2
Let A be the vector potential and B the magnetic field of the infinite solenoid of radius R. Then
B(r) = {Bk if R
r
where r = √x² + y² is the distance to the z-axis and B is a constant that depends on the current strength I and the spacing of
the turns of wire.
The vector potential for B is
A(r) = { }B (-3,2,0)
B(-y.x,0)
8
(a) Use Stokes' Theorem to compute the flux of B through a circle in the xy-plane of radius r = 6 R
if r < R
A dr =
(b) Use Stokes' Theorem to compute the circulation of A around the boundary C of a surface lying outside the solenoid.
(Use symbolic notation and fractions where needed.)
C
2
Br
0
Chapter 6 Solutions
Calculus Volume 3
Ch. 6.1 - The domain of vector field F = F(x, y) is a set of...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, determine whether the...Ch. 6.1 - For the following exercises, describe each vector...Ch. 6.1 - For the following exercises, describe each vector...Ch. 6.1 - For the following exercises, describe each vector...Ch. 6.1 - For the following exercises, describe each vector...Ch. 6.1 - For the following exercises, describe each vector...Ch. 6.1 - For the following exercises, describe each vector...
Ch. 6.1 - For the following exercises, describe each vector...Ch. 6.1 - For the following exercises, describe each vector...Ch. 6.1 - For the following exercises, describe each vector...Ch. 6.1 - For the following exercises, describe each vector...Ch. 6.1 - For the following exercises, find the gradient...Ch. 6.1 - For the following exercises, find the gradient...Ch. 6.1 - For the following exercises, find the gradient...Ch. 6.1 - For the following exercises, find the gradient...Ch. 6.1 - For the following exercises, find the gradient...Ch. 6.1 - For the following exercises, find the gradient...Ch. 6.1 - What is vector field F(x, y) with a value at (x,...Ch. 6.1 - For the following exercises, write formulas for...Ch. 6.1 - For the following exercises, write formulas for...Ch. 6.1 - For the following exercises, write formulas for...Ch. 6.1 - Give a formula F(x, y) = M(x, y)i + N(x, y)j for...Ch. 6.1 - Is vector field F(x, y) = (P(x, y), Q(x, y)) =...Ch. 6.1 - Find a formula for vector field F(x, y) = M(x,,y)i...Ch. 6.1 - For the following exercises, assume that an...Ch. 6.1 - For the following exercises, assume that an...Ch. 6.1 - For the following exercises, assume that an...Ch. 6.1 - c(t) = (sin t. cos t, et); F(x,y,z)=y,x,zCh. 6.1 - For the following exercises, let F = xi + yi, G =...Ch. 6.1 - For the following exercises, let F = xi + yi, G =...Ch. 6.1 - For the following exercises, let F = xi + yi, G =...Ch. 6.1 - For the following exercises, let F = xi + yj, G =...Ch. 6.1 - For the following exercises,...Ch. 6.1 - For the following exercises, let...Ch. 6.1 - For the following exercises, let...Ch. 6.2 - True or False? Line integral cf(x,y)dsis equal to...Ch. 6.2 - True or False? Vector functions r1= ti +t2j,...Ch. 6.2 - True or False? c(Pdx+Qdy)=c(PdxQdy)Ch. 6.2 - True or False? A piecewise smooth cuive C consists...Ch. 6.2 - True or False?If C is given by x(t) = t,y(t) = t,0...Ch. 6.2 - For the following exercises, use a computer...Ch. 6.2 - For the following exercises, use a computer...Ch. 6.2 - For the following exercises, use a computer...Ch. 6.2 - For the following exercises, use a computer...Ch. 6.2 - For the following exercises, use a computer...Ch. 6.2 - For the following exercises, find the work done....Ch. 6.2 - For the following exercises, find the work done....Ch. 6.2 - For the following exercises, find the work done....Ch. 6.2 - For the following exercises, find the work done....Ch. 6.2 - For the following exercises, find the work done....Ch. 6.2 - For the following exercises, find the work done....Ch. 6.2 - For the following exercises, evaluate the line...Ch. 6.2 - For the following exercises, evaluate the line...Ch. 6.2 - For the following exercises, evaluate the line...Ch. 6.2 - For the following exercises, evaluate the line...Ch. 6.2 - For the following exercises, evaluate the line...Ch. 6.2 - For the following exercises, evaluate the line...Ch. 6.2 - For the following exercises, evaluate the line...Ch. 6.2 - For the following exercises, evaluate the line...Ch. 6.2 - For the following exercises, evaluate the line...Ch. 6.2 - For the following exercises, evaluate the line...Ch. 6.2 - In the following exercises, find the work done by...Ch. 6.2 - In the following exercises, find the work done by...Ch. 6.2 - In the following exercises, find the work done by...Ch. 6.2 - In the following exercises, find the work done by...Ch. 6.2 - In the following exercises, find the work done by...Ch. 6.2 - In the following exercises, find the work done by...Ch. 6.2 - In the following exercises, find the work done by...Ch. 6.2 - In the following exercises, find the work done by...Ch. 6.2 - In the following exercises, find the work done by...Ch. 6.2 - In the following exercises, find the work done by...Ch. 6.2 - Evaluate the line integral of scalar function xy...Ch. 6.2 - Find yc2dx+(xy x 2)dy along C: y = 3x from C (0,...Ch. 6.2 - Find yc2dx+(xy x 2)dyalong C: y2= 9x from (0, 0)...Ch. 6.2 - For the following exercises, use a CAS to evaluate...Ch. 6.2 - For the following exercises, use a CAS to evaluate...Ch. 6.2 - For the following exercises, use a CAS to evaluate...Ch. 6.2 - For the following exercises, use a CAS to evaluate...Ch. 6.2 - For the following exercises, use a CAS to evaluate...Ch. 6.2 - For the following exercises, use a CAS to evaluate...Ch. 6.2 - For the following exercises, use a CAS to evaluate...Ch. 6.2 - For the following exercises, use a CAS to evaluate...Ch. 6.2 - For the following exercises, use a CAS to evaluate...Ch. 6.2 - For the following exercises, find the flux. 87....Ch. 6.2 - For the following exercises, find the flux. 88....Ch. 6.2 - For the following exercises, find the flux. 89....Ch. 6.2 - For the following exercises, find the flux. 90....Ch. 6.2 - For the following exercises, find the flux. 91....Ch. 6.2 - Find the line integral of k c z 2dx+ydy+2ydz,where...Ch. 6.2 - A spring is made of a thin wire twisted into the...Ch. 6.2 - A thin wire is bent into the shape of a semicircle...Ch. 6.2 - An object moves in force field...Ch. 6.2 - Find the work done when an object moves in force...Ch. 6.2 - If an inverse force field F. is given by F(x, y,...Ch. 6.2 - David and Sandra plan to evaluate line integral...Ch. 6.3 - True or False? If vector field F is conservative...Ch. 6.3 - Trueor False? Function r(t) = a + t(b — a), where...Ch. 6.3 - True or False? Vector field F(x, y,z) = (y sinz)i...Ch. 6.3 - True or False?Vector field F(x,y,z)= yi + (x + z)j...Ch. 6.3 - Justify the Fundamental Theorem of Line Integrals...Ch. 6.3 - [T] Find cF.dr,,] where...Ch. 6.3 - [T] Evaluate line integral cF.dr, where...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, evaluate the line...Ch. 6.3 - For the following exercises, evaluate the line...Ch. 6.3 - For the following exercises, evaluate the line...Ch. 6.3 - For the following exercises, evaluate the line...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, determine whether the...Ch. 6.3 - For the following exercises, evaluate the integral...Ch. 6.3 - For the following exercises, evaluate the integral...Ch. 6.3 - For the following exercises, evaluate the integral...Ch. 6.3 - For the following exercises, evaluate the integral...Ch. 6.3 - For the following exercises, let F(x, y) = 2xy2i +...Ch. 6.3 - For the following exercises, let F(x, y) = 2xy2i +...Ch. 6.3 - For the following exercises, let F(x, y) = 2xy2i +...Ch. 6.3 - For the following exercises, let F(x, y) = 2xy2i +...Ch. 6.3 - [T] Let F(x, y, z) = x2i + zsin(yz)j + y sin(yz)k....Ch. 6.3 - [T] Find line integral cF.dr,of vector field F(x,...Ch. 6.3 - For the following exercises, show that the...Ch. 6.3 - For the following exercises, show that the...Ch. 6.3 - For the following exercises, show that the...Ch. 6.3 - For the following exercises, show that the...Ch. 6.3 - For the following exercises, show that the...Ch. 6.3 - For the following exercises, show that the...Ch. 6.3 - For the following exercises, show that the...Ch. 6.3 - Find the circulation and flux of field F=yi+xj...Ch. 6.3 - Compute ccosxcosydxsinxsinydy, where...Ch. 6.3 - Complete the proof of The Path Independence Test...Ch. 6.4 - Measuring Area from a Boundary: The Planimeter...Ch. 6.4 - Measuring Area from a Boundary: The Planimeter...Ch. 6.4 - easuring Area from a Boundary: The Planimeter...Ch. 6.4 - Measuring Area from a Boundary: The Planimeter...Ch. 6.4 - Measuring Area from a Boundary: The Planimeter...Ch. 6.4 - Measuring Area from a Boundary: The Planimeter...Ch. 6.4 - Measuring Area from a Boundary: The Planimeter...Ch. 6.4 - Measuring Area from a Boundary: The Planimeter...Ch. 6.4 - Measuring Area from a Boundary: The Planimeter...Ch. 6.4 - ]Measuring Area from a Boundary: The Planimeter...Ch. 6.4 - For the following exercises, evaluate the line...Ch. 6.4 - For the following exercises, evaluate the line...Ch. 6.4 - For the following exercises, evaluate the line...Ch. 6.4 - For the following exercises, evaluate the line...Ch. 6.4 - For the following exercises, evaluate the line...Ch. 6.4 - For the following exercises, evaluate the line...Ch. 6.4 - For the following exercises, use Green’s theorem....Ch. 6.4 - For the following exercises, use Green’s theorem....Ch. 6.4 - Find the counterclockwise circulation of field...Ch. 6.4 - Evaluate cy3dxx3y2dy,where C is the positively...Ch. 6.4 - Evaluate cy3dxx3dy,where C includes the two...Ch. 6.4 - Calculate cx2ydx+xy2dy,where C isa circle of...Ch. 6.4 - Calculate integral...Ch. 6.4 - Evaluate integral c( x 2+ y 2)dx+2xydy,where C is...Ch. 6.4 - Evaluate line integralc(ysin( y)cos( y)dx+2x sin...Ch. 6.4 - For the following exercises, use Green’s theorem...Ch. 6.4 - For the following exercises, use Green’s theorem...Ch. 6.4 - For the following exercises, use Green’s theorem...Ch. 6.4 - For the following exercises, use Green’s theorem...Ch. 6.4 - For the following exercises, use Green’s theorem...Ch. 6.4 - For the following exercises, use Green’s theorem...Ch. 6.4 - For the following exercises, use Green’s theorem...Ch. 6.4 - For the following exercises, use Green’s theorem...Ch. 6.4 - For the following exercises, use Green’s theorem...Ch. 6.4 - [T] Evaluate Green’s theorem using a computer...Ch. 6.4 - Evaluate c(x2y2xy+y2)ds,where C is the boundary of...Ch. 6.4 - Evaluate ( y+2)dx+( x1)dyc ( x1 ) 2+ ( y+2 )...Ch. 6.4 - 173. Evaluate xdx+ydy c x 2 + y 2 , . where C is...Ch. 6.4 - For the following exercises, use Green’s theorem...Ch. 6.4 - For the following exercises, use Green’s theorem...Ch. 6.4 - For the following exercises, use Green’s theorem...Ch. 6.4 - A particle starts at point (-2, 0), moves along...Ch. 6.4 - David and Sandra are skating on a frictionless...Ch. 6.4 - Use Green’s theorem to find the work done by force...Ch. 6.4 - Use Green’s theorem to evaluate line integral...Ch. 6.4 - Evaluate line integral c y 2dx+x2dy,where C is...Ch. 6.4 - Use Green’s theorem to evaluate line integral...Ch. 6.4 - Use Green’s theorem to evaluate line integral c1+...Ch. 6.4 - Use Green’s theorem to evaluate line integral...Ch. 6.4 - Use Green’s theorem to evaluate line integral c(3y...Ch. 6.4 - Use Green’s theorem to evaluate line integral...Ch. 6.4 - Let C be a tiiangulai closed curve from (0, 0) to...Ch. 6.4 - Use Green’s theoiem to evaluate line integral...Ch. 6.4 - Use Green’s theorem to evaluate line integral...Ch. 6.4 - Use Green’s theorem to evaluate line integral...Ch. 6.4 - Use Green’s theorem to evaluate cxydx+ x 3 y 3dy,...Ch. 6.4 - Use Green’s theorem to evaluate line integral...Ch. 6.4 - Let F(x,y)=(cos(x5))13y3i+13x3j.Find the...Ch. 6.4 - Use Green’s theorem to evaluate line integral...Ch. 6.4 - Let C be the boundary of square 0x,0y, traversed...Ch. 6.4 - Use Green’s theorem to evaluate line integral,...Ch. 6.4 - Use Green’s Theorem to evaluate integial...Ch. 6.4 - Use Green’s theorem in a plane to evaluate line...Ch. 6.4 - Calculate the outward flux of F = -xi + 2yj over a...Ch. 6.4 - 200. [T] Let C be circle x2+ y2= 4 oriented in the...Ch. 6.4 - Find the flux of field F = -xi + yj across x2+ y2...Ch. 6.4 - Let F = (y2— x2)i + (x2+y2)j, and let C be a...Ch. 6.4 - [T] Let C be unit circle x2+ y2 = 1 traversed once...Ch. 6.4 - [T] Find the outward flux of vector field F = xy2i...Ch. 6.4 - Consider region R bounded by parabolas y= x2and x...Ch. 6.5 - For the following exercises, find the curl of F at...Ch. 6.5 - For the following exercises, determine whether the...Ch. 6.5 - For the following exercises, determine whether the...Ch. 6.5 - For the following exercises, determine whether the...Ch. 6.5 - For the following exercises, determine whether the...Ch. 6.5 - For the following exercises, determine whether the...Ch. 6.5 - For the following exercises, determine whether the...Ch. 6.5 - For the following exercises, find the curl of F....Ch. 6.5 - For the following exercises, find the curl of F....Ch. 6.5 - For the following exercises, find the curl of F....Ch. 6.5 - For the following exercises, find the curl of F....Ch. 6.5 - For the following exercises, find the curl of F....Ch. 6.5 - For the following exercises, find the curl of F....Ch. 6.5 - For the following exercises, find the curl of F....Ch. 6.5 - For the following exercises, find the curl of F....Ch. 6.5 - For the following exercises, find the curl of F....Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - 233.w(x,y,z)=(x2+y2+z2)1/2Ch. 6.5 - 232.u(x,y,z)=ex(cosysiny)...Ch. 6.5 - 234.IfF(x,y,z)=2i+2xj+3ykCh. 6.5 - ...Ch. 6.5 - Find div F, given that F = f, where f(x,y,z)=xy3z2...Ch. 6.5 - 237. Find the divergence of F for vector field...Ch. 6.5 - Find the divergence of F for vector field...Ch. 6.5 - For the following exercises, use r = |r|and r =...Ch. 6.5 - For the following exercises, use r = |r|and r =...Ch. 6.5 - For the following exercises, use r = |r|and r =...Ch. 6.5 - For the following exercises, use r = |r| and r =...Ch. 6.5 - For the following exercises, use a computer...Ch. 6.5 - For the following exercises, use a computer...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the divergence...Ch. 6.5 - For the following exercises, find the curl of F at...Ch. 6.5 - For the following exercises, find the curl of F at...Ch. 6.5 - For the following exercises, find the curl of F at...Ch. 6.5 - For the following exercises, find the curl of F at...Ch. 6.5 - For the following exercises, find the curl of F at...Ch. 6.5 - For the following exercises, find the curl of F at...Ch. 6.5 - For the following exercises, find the curl of F at...Ch. 6.5 - For the following exercises, find the curl of F at...Ch. 6.5 - For the following exercises, find the curl of F at...Ch. 6.5 - For the following exercises, find the curl of F at...Ch. 6.5 - For the following exercises, find the curl of F at...Ch. 6.5 - For the following exercises, consider a rigid body...Ch. 6.5 - For the following exercises, consider a rigid body...Ch. 6.5 - For the following exercises, consider a rigid body...Ch. 6.5 - In the following exercises, suppose that F=0 and...Ch. 6.5 - In the following exercises, suppose that F=0 and...Ch. 6.5 - In the following exercises, suppose a solid object...Ch. 6.5 - In the following exercises, suppose a solid object...Ch. 6.5 - Consider rotational velocity field v=0,10z,-10y....Ch. 6.6 - For the following exercises, determine whether the...Ch. 6.6 - wFor the following exercises, determine whether...Ch. 6.6 - For the following exercises, determine whether the...Ch. 6.6 - For the following exercises, determine whether the...Ch. 6.6 - For the following exercises, find parametric...Ch. 6.6 - For the following exercises, find parametric...Ch. 6.6 - For the following exercises, find parametric...Ch. 6.6 - For the following exercises, find parametric...Ch. 6.6 - For the following exercises, find parametric...Ch. 6.6 - For the following exercises, find parametric...Ch. 6.6 - For the following exercises, use a computer...Ch. 6.6 - For the following exercises, use a computer...Ch. 6.6 - For the following exercises, let S be the...Ch. 6.6 - For the following exercises, let S be the...Ch. 6.6 - For the following exercises, let S be the...Ch. 6.6 - wFor the following exercises, evaluate sFNds for...Ch. 6.6 - For the following exercises, evaluate sFNds for...Ch. 6.6 - For the following exercises, evaluate sFNds for...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - wFor the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, approximate the mass...Ch. 6.6 - For the following exercises, express the surface...Ch. 6.6 - For the following exercises, express the surface...Ch. 6.6 - For the following exercises, express the surface...Ch. 6.6 - For the following exercises, express the surface...Ch. 6.6 - For the following exercises, express the surface...Ch. 6.6 - For the following exercises, express the surface...Ch. 6.6 - For the following exercises, express the surface...Ch. 6.6 - For the following exercises, express the surface...Ch. 6.6 - For the following exercises, express the surface...Ch. 6.6 - For the following exercises, express the surface...Ch. 6.6 - For the following exercises, use geometric...Ch. 6.6 - For the following exercises, use geometric...Ch. 6.6 - For the following exercises, use geometric...Ch. 6.6 - A lamina has the shape of a portion of sphere...Ch. 6.6 - A lamina has the shape of a portion of sphere...Ch. 6.6 - A paper cup has the shape of an inverted right...Ch. 6.6 - For the following exercises, the heat flow vector...Ch. 6.6 - For the following exercises, the heat flow vector...Ch. 6.6 - For the following exercises, consider the radial...Ch. 6.6 - For the following exercises, consider the radial...Ch. 6.7 - For the following exercises, without using Stokes’...Ch. 6.7 - For the following exercises, without using Stokes’...Ch. 6.7 - For the following exercises, without using Stokes’...Ch. 6.7 - For the following exercises, without using Stokes’...Ch. 6.7 - For the following exercises, without using Stokes’...Ch. 6.7 - For the following exercises, without using Stokes’...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following application exercises, the goal...Ch. 6.7 - For the following application exercises, the goal...Ch. 6.7 - For the following application exercises, the goal...Ch. 6.7 - For the following exercises, let S he the disk...Ch. 6.7 - For the following exercises, let S he the disk...Ch. 6.7 - For the following exercises, let S he the disk...Ch. 6.7 - For the following exercises, let S he the disk...Ch. 6.7 - For the following exercises, let S he the disk...Ch. 6.7 - For the following exercises, let S he the disk...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.7 - For the following exercises, use Stokes’ theorem...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a computer...Ch. 6.8 - For the following exercises, use a CAS along with...Ch. 6.8 - For the following exercises, use a CAS along with...Ch. 6.8 - For the following exercises, use a CAS along with...Ch. 6.8 - `For the following exercises, use a CAS along with...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, use a CAS and the...Ch. 6.8 - For the following exercises, Fourier’s law of heat...Ch. 6.8 - For the following exercises, Fourier’s law of heat...Ch. 6.8 - For the following exercises, Fourier’s law of heat...Ch. 6 - True or False? Justify your answer with a proof or...Ch. 6 - True or False? Justify your answer with a proof or...Ch. 6 - True or False? Justify your answer with a proof or...Ch. 6 - True or False? Justify your answer with a proof or...Ch. 6 - Draw the following vector fields. 431....Ch. 6 - Draw the following vector fields. 432....Ch. 6 - Are the following the vector fields conservative?...Ch. 6 - Are the following the vector fields conservative?...Ch. 6 - Are the following the vector fields conservative?...Ch. 6 - Are the following the vector fields conservative?...Ch. 6 - Evaluate the following integrals. 437....Ch. 6 - Evaluate the following integrals. 438. Cydx+xy2dy...Ch. 6 - Evaluate the following integrals. 439. Sxy2dS ,...Ch. 6 - Find the divergence and curl for the following...Ch. 6 - Find the divergence and curl for the following...Ch. 6 - Use Green’s theorem to evaluate the following...Ch. 6 - Use Green’s theorem to evaluate the following...Ch. 6 - Use Stokes’ theorem to evaluate ScurlFdS . 444....Ch. 6 - Use Stokes’ theorem to evaluate ScurlFdS . 445....Ch. 6 - Use the divergence theorem to evaluate SFdS . 446....Ch. 6 - Use the divergence theorem to evaluate SFdS . 447....Ch. 6 - Find the amount of work perfumed by a 50 -kg woman...Ch. 6 - Find the total mass of a thin wire in the shape of...Ch. 6 - Find the total mass of a thin sheet in the shape...Ch. 6 - Use the divergence theorem to compute the value of...
Additional Math Textbook Solutions
Find more solutions based on key concepts
29-36. Total and Annual Returns. Compute the total and annual returns on the following investments.
29. Five ye...
Using and Understanding Mathematics: A Quantitative Reasoning Approach (6th Edition)
Sine substitution Evaluate the following integrals. 11. 01/2x21x2dx
Calculus: Early Transcendentals (2nd Edition)
TRY IT YOURSELF 1
Find the mean of the points scored by the 51 winning teams listed on page 39.
Elementary Statistics: Picturing the World (7th Edition)
log a =
Precalculus
Area Suppose that the radius r and area A = πr2 of a circle are differentiable functions of t. Write an equatio...
University Calculus: Early Transcendentals (4th Edition)
Show that 34=12 using each of the following models. a. Repeated-addition number line b. Rectangular array c. Ar...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- Draw the slope field (direction field) for the following DE: y'=y-tarrow_forwardcalculate the gradient field of the equation attached. But use math convention for the sign of gradient potentialarrow_forward6) Let f(xxx)=3xy²-x² be a potential function of a fluid velocity field. a) Find the magnitude of the greatest rate of change for f(x,y) at (1,3). Please provide a physical interpretation. b) Find the direction angles for this greatest rate of change. Express angles in degrees. Please provide a physical interpretation.arrow_forward
- Calculate the gradient ∇f, where f(x, y) = ln(x2 + y2) (here (x, y) ̸= (0, 0)).arrow_forwardFind the work done for a force F 12 =— x² N from x=2 to x =6 m.arrow_forward(i) An electrostatic field in xy-plane is given by p(x, y) = 3x2y- y³. Find the stream function w such that the complex potential co= + iw is an analytic function.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
Discrete Mathematics and Its Applications ( 8th I...MathISBN:9781259676512Author:Kenneth H RosenPublisher:McGraw-Hill Education
Mathematics for Elementary Teachers with Activiti...MathISBN:9780134392790Author:Beckmann, SybillaPublisher:PEARSON
Thinking Mathematically (7th Edition)MathISBN:9780134683713Author:Robert F. BlitzerPublisher:PEARSON
Discrete Mathematics With ApplicationsMathISBN:9781337694193Author:EPP, Susanna S.Publisher:Cengage Learning,
Pathways To Math Literacy (looseleaf)MathISBN:9781259985607Author:David Sobecki Professor, Brian A. MercerPublisher:McGraw-Hill Education
Discrete Mathematics and Its Applications ( 8th I...
Math
ISBN:9781259676512
Author:Kenneth H Rosen
Publisher:McGraw-Hill Education
Mathematics for Elementary Teachers with Activiti...
Math
ISBN:9780134392790
Author:Beckmann, Sybilla
Publisher:PEARSON
Thinking Mathematically (7th Edition)
Math
ISBN:9780134683713
Author:Robert F. Blitzer
Publisher:PEARSON
Discrete Mathematics With Applications
Math
ISBN:9781337694193
Author:EPP, Susanna S.
Publisher:Cengage Learning,
Pathways To Math Literacy (looseleaf)
Math
ISBN:9781259985607
Author:David Sobecki Professor, Brian A. Mercer
Publisher:McGraw-Hill Education
Basic Differentiation Rules For Derivatives; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=IvLpN1G1Ncg;License: Standard YouTube License, CC-BY