Chapter 6, Problem 6.7P

Parallel flow of atmospheric air over a flat plate of length $L=3\text{m}$ is disrupted by an array of stationary rods placed in the flow path over the plate.

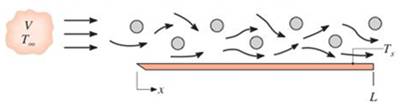
Laboratory measurements of the local convection coefficient at the surface of the plate are made for a prescribed value of V and $T_s>T_{\infty }.$ The results are correlated by an expression of the form $h_x=0.7+13.6x-3.4x^2,$ where $h_x$ has units of ${\text{W/m}}^{\text{2}}\cdot \text{K}$ and x is in meters. Evaluate the average convection coefficient ${\bar{h}}_L$ for the entire plate and the ratio ${\bar{h}}_L/h_L$ at the trailing edge.