Control Systems Engineering
7th Edition
ISBN: 9781118170519
Author: Norman S. Nise
Publisher: WILEY
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 5, Problem 75P
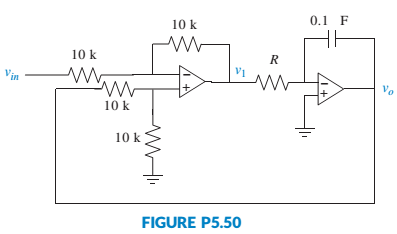
Assume ideal operational amplifiers in the circuit of Figure P5.50.
a. Show that the leftmost operational amplifier works as a subtracting amplifier. Namely,
b. Draw a block diagram of the system, with the subtracting amplifier represented with a summing junction, and the circuit of the rightmost operational amplifier with a transfer function in the forward path. Keep R as a variable.
c. Obtain the system’s closed-loop transfer function.
d. For a unit step input, obtain the value of R that will result in a settling time Ts= 1 msec.
e. Using the value of R calculated in Part d, make a sketch of the resulting unit step response.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Obtain the state space model of the system shown below. Use equations for control theory state space modeling.
26. For the system shown in Figure P4.8, a step torque is
applied at 01 (t). Find
a. The transfer function, G(s) = 02(s)/T(s).
b. The percent overshoot, settling time, and peak
time for 02(t). [Section: 4.6]
T(t) 01(1)
02(1)
ff
1.07 kg-m2
1.53 N-m-s/rad
1.92 N-m/rad
FIGURE P4.8
٢٢
Chapter 5 Solutions
Control Systems Engineering
Ch. 5 - Prob. 1RQCh. 5 - Name three basic forms for interconnecting...Ch. 5 - For each of the forms in Question 2, state...Ch. 5 - Besides knowing the basic forms as discussed in...Ch. 5 - For a simple, second-order feedback control system...Ch. 5 - Prob. 6RQCh. 5 - Prob. 7RQCh. 5 - How are summing junctions shown on a signal-flow...Ch. 5 - If a forward path touched all closed loops, what...Ch. 5 - Name five representations of systems in state...
Ch. 5 - Prob. 11RQCh. 5 - Which form of the state-space representation leads...Ch. 5 - When the system matrix is diagonal, what...Ch. 5 - What terms lie along the diagonal for a system...Ch. 5 - Prob. 15RQCh. 5 - Prob. 16RQCh. 5 - For what kind of system would you use the observer...Ch. 5 - Describe state-vector transformations from the...Ch. 5 - Prob. 19RQCh. 5 - Prob. 20RQCh. 5 - Prob. 21RQCh. 5 - Find the closed-loop transfer function, T(s) =...Ch. 5 - Find the equivalent transfer function, T(s) =...Ch. 5 - Reduce the system shown in Figure P5.4 to a single...Ch. 5 - Reduce the block diagram shown in Figure P5.6 to a...Ch. 5 - Find the unity feedback system that is equivalent...Ch. 5 - 8. Given the block diagram of a system shown in...Ch. 5 - 9. Reduce the block diagram shown in Figure P5.9...Ch. 5 - Reduce the block diagram shown in Figure P5.10 to...Ch. 5 - 11. For the system shown in Figure P5.11, find the...Ch. 5 - 12. For the system shown in Figure P5.12, find the...Ch. 5 - Prob. 13PCh. 5 - For the system of Figure P5.14, find the value of...Ch. 5 - 15. For the system shown in Figure P5.15, find K...Ch. 5 - For the system of Figure P5.16, find the values of...Ch. 5 - Find the following for the system shown in Figure...Ch. 5 - 18. For the system shown in Figure P5.18, find ,...Ch. 5 - Prob. 19PCh. 5 - Prob. 20PCh. 5 - Find the transfer function G(s) = Eo(s)/T(s) for...Ch. 5 - Prob. 22PCh. 5 - Prob. 23PCh. 5 - State Space SS
24. Given the system below, draw a...Ch. 5 - Prob. 25PCh. 5 - Using Mason’s rule, find the transfer function,...Ch. 5 - Using Mason’s rule, find the transfer function,...Ch. 5 - Prob. 28PCh. 5 - Use block diagram reduction to find the transfer...Ch. 5 - State Space SS 30. Represent the following systems...Ch. 5 - Prob. 31PCh. 5 - State Space SS 32. Repeat Problem 31 and represent...Ch. 5 - Prob. 33PCh. 5 - Prob. 34PCh. 5 - Repeat Problem 34 for the system shown in Figure...Ch. 5 - Prob. 37PCh. 5 - State Space SS 38. Consider the rotational...Ch. 5 - Prob. 40PCh. 5 - Prob. 41PCh. 5 - State Space SS
42. Consider the subsystems shown...Ch. 5 - Prob. 43PCh. 5 - Prob. 44PCh. 5 - State Space SS
45. Diagonalize the following...Ch. 5 - Prob. 46PCh. 5 - Prob. 48PCh. 5 - Prob. 51PCh. 5 - Figure P5.33 shows a noninverting operational...Ch. 5 - Figure P5.34 shows the diagram of au inverting...Ch. 5 - Prob. 54PCh. 5 - A car active suspension system adds an active...Ch. 5 - Prob. 58PCh. 5 - Prob. 60PCh. 5 - Some medical procedures require the insertion of a...Ch. 5 - Prob. 62PCh. 5 - Prob. 64PCh. 5 - Prob. 65PCh. 5 - The purpose of an Automatic Voltage Regulator is...Ch. 5 - 68. Integrated circuits are manufactured through a...Ch. 5 - Prob. 69PCh. 5 - Prob. 72PCh. 5 - Prob. 73PCh. 5 - Assume ideal operational amplifiers in the circuit...Ch. 5 - Parabolic trough collector. Effective controller...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- 3. Obtain the transfer function Y(s)/R(s) for the system represented by the block diagram shown in Figure 3. H2 k R(s) G1 G2 Y(s) y Figure 3 Figure 4arrow_forward1 / 1 Problem No. 1 1A. 100% + 1B. Consider the translational mechanical system shown in Figure P4.17. A 1-pound force, f(t), is applied at t = 0. If fy = 1, find K and M such that the response is characterized by a 4-second settling time and a 1-second peak time. Also, what is the resulting percent overshoot? [Section: 4.6] 70) 0000 31/1 10000 K FIGURE P4.17 Given the translational mechanical system of Figure P4.17, where K = 1 and f(1) is a unit step. find the values of M and ƒ, to yield a response with 17% overshoot and a settling time of 10 seconds. [Section: 4.6]arrow_forwardThe ratio of output to input of a system in Laplace domain is known as Transfer function . Select one: True Falsearrow_forward
- A translational mechanical system is shown in Figure Q1. In this system, u(t) is the displacement of the cart and the input to the system. The displacement y(t) of the mass relative to the ground is the output. Q1 (a) Determine the mathematical model of the system. (b) Using the result in Q1(a), determine a transfer function of the system. Massless Cart k m Figure Q1arrow_forwardConsider in Figure 1 = 0. Iff, the translational mechanical system shown P4.17. A 1-pound force, f(t), is applied at 1, find K and M such that the response is characterized by a 4-second settling time and a 1-second peak time. Also, what is the resulting percent overshoot? [Section: 4.6] 1+ 270 Karrow_forwardSimplify the block diagram shown below. Then, obtain the closed-loop transfer function C(s)/R(s). H3 R(s)- G1 G2 G3 G4 > C(s) H +arrow_forward
- 2. Consider the closed-loop system shown below. Determine the range of K for stability. Assume that K > 0. R(s) K S-2 (s + 1)(s² + 6s+25) C(s)arrow_forwardThe state z(t) of a dynamical system is solution of equation ż(t) + aż(t) + 25z(t) = 12, with a = 7.3. Calculate the Peak time of the response.arrow_forwardBriefly explain the terms time constant, damping ratio, undamped natural frequency and damped natural frequency in relation to the transient response of systems. For each of the systems with transfer funtions given below, determine the closed-loop poles and sketch the response c(t) when the input, r(t), is a unit step. Indicate the steady-state value of c(t) in each case. (i) (ii) (iii) C(s) 1 R(s) s+0.5 = C(s) 1 = R(s) s² +5.5s +2.5 C(s) 1 = R(s) s² +s+1 b y(t) m d k₂ wwwx(1) For the system in the figure shown above, x(t) and y(t) are displacements, k, and k2 are spring constants and b is a damping coefficient. Derive the transfer function Y(s) x(s)arrow_forward
- 9. Derive the transfer function X₂ (s)/F(s) for the mechanical system shown in Figure 2. Assume the following values: M₁ = 3 kg, M₂ = 2 kg, K₁ = 5 N/m, K₁= 3 N/m, D₁ = 1 N.s/m, K₁ oooo OH M₁ oooo K₂ Figure 2 M₂ 1(1)arrow_forward(2) A mechanical system is modeled by the system of ODE's. For this system choose [x₂] [y] -0-0 = X3 18 2 consider the output to be y, and do the following: Determine the state-space matrices A, B, and C ● Determine the characteristic equation mÿ+k₁y+k₂(y−z)=F c₂ż−k₂(y-z)=0arrow_forwardExample 2 Obtain the transfer function of C(s)/R(s) of the system whose signal flow graph is shown in Figure -H2 R(s) 1 GI G2 1 C(s) G3 H1arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY
Introduction to Kinematics; Author: LearnChemE;https://www.youtube.com/watch?v=bV0XPz-mg2s;License: Standard youtube license