
Concept explainers
(a)
The smallest outside radius permitted for a bend in the fiber.
(a)
Answer to Problem 49P
The smallest outside radius permitted for a bend in the fiber is
Explanation of Solution
The ray diagram for the optical fiber is shown in figure below.
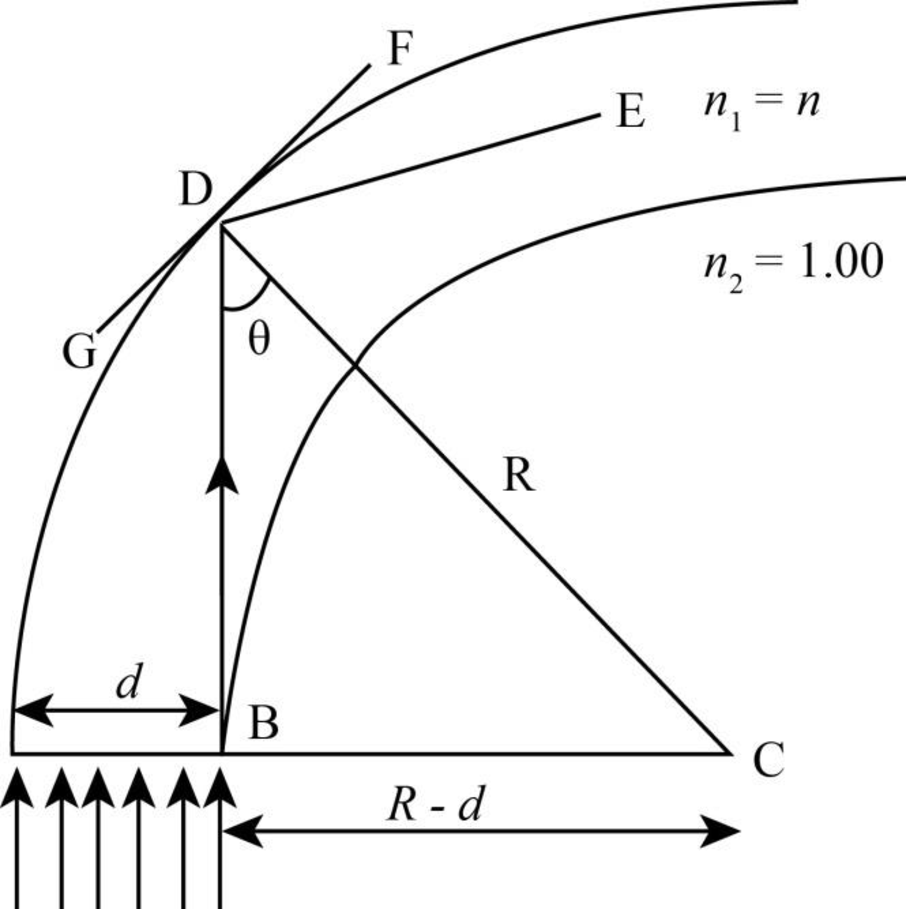
Figure (1)
It is given that,
Here,
Write the expression to calculate the critical angle.
Here,
Re arrange equation (I) to get the expression for
Consider figure (1).
Since the light ray on the left side of point
From figure (1).
Substitute
Here,
Also,
Since,
Consider
Substitute
The condition for total internal reflection to occur is,
Substitute
Conclusion:
Substitute
So the smallest outside radius for no light to escape is,
Therefore, the smallest outside radius permitted for a bend in the fiber is
(b).
The effect on the smallest outer radius when
(b).
Answer to Problem 49P
The effect on the smallest outer radius when
Explanation of Solution
Rearrange the expression for
From equation (IX), it is clearly visible that
So, as
Yes, this behavior is reasonable because for very small diameter, the light strikes the interface at very large angles of incidence.
(c).
The effect on the smallest outer radius when
(c).
Answer to Problem 49P
The value of smallest outer radius
Explanation of Solution
From equation (IX), when
From equation (I), when
Thus, the above result is reasonable because with the increase in the value of
(d).
The effect on the smallest outer radius when
(d).
Answer to Problem 49P
The value of smallest outer radius
Explanation of Solution
From equation (IX), when
From equation (I), when
This result is reasonable because the critical angle becomes close to
(e).
The value of
(e).
Answer to Problem 49P
The value of
Explanation of Solution
Conclusion:
Substitute
Therefore, the value of
Want to see more full solutions like this?
Chapter 35 Solutions
Bundle: Physics for Scientists and Engineers with Modern Physics, Loose-leaf Version, 9th + WebAssign Printed Access Card, Multi-Term
- 3.77 is not the correct answer!arrow_forwardA I squar frame has sides that measure 2.45m when it is at rest. What is the area of the frame when it moves parellel to one of its diagonal with a m² speed of 0.86.c as indicated in the figure? >V.arrow_forwardAn astronent travels to a distant star with a speed of 0.44C relative to Earth. From the austronaut's point of view, the star is 420 ly from Earth. On the return trip, the astronent travels speed of 0.76c relative to Earth. What is the distance covered on the return trip, as measured by the astronant? your answer in light-years. with a Give ly.arrow_forward
- star by spaceship Sixus is about 9.00 ly from Earth. To preach the star in 15.04 (ship time), how fast must you travel? C.arrow_forwardIf light-bulb A is unscrewed, how will the brightness of bulbs B and C change, if at all? How does the current drawn by from the battery change?arrow_forwardCan someone help mearrow_forward
- Can someone help me with this thank youarrow_forward(a) For a spherical capacitor with inner radius a and outer radius b, we have the following for the capacitance. ab C = k₂(b- a) 0.0695 m 0.145 m (8.99 × 10º N · m²/c²)( [0.145 m- 0.0695 m × 10-11 F = PF IIarrow_forwardA pendulum bob A (0.5 kg) is given an initialspeed of vA = 4 m/s when the chord ishorizontal. It then hits a stationary block B (1kg) which then slides to a maximum distanced before it stops. Determine the value of d.The coefficient of static friction between theblock and the plane is μk = 0.2. The coefficientof restitution between A and B is e = 0.8.Ans: d=1.0034 marrow_forward
- Figure 29-43 Problem 12. ••13 In Fig. 29-44, point P₁ is at distance R = 13.1 cm on the perpendicular bisector of a straight wire of length L = 18.0 cm carrying current i = 58.2 mA. (Note that the wire is not long.) What is the magnitude of the magnetic field at P₁ due to i? P2° R R Larrow_forwardCheckpoint 1 The figure shows the current i in a single-loop circuit with a battery B and a resistance R (and wires of neg- ligible resistance). (a) Should the emf arrow at B be drawn pointing leftward or rightward? At points a, B C R b, and c, rank (b) the magnitude of the current, (c) the electric potential, and (d) the electric potential energy of the charge carriers, greatest first.arrow_forwardPls help ASAParrow_forward
 Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





