Algebra 2
1st Edition
ISBN: 9780078884825
Author: McGraw-Hill/Glencoe
Publisher: Glencoe/McGraw-Hill School Pub Co
expand_more
expand_more
format_list_bulleted
Videos
Question
Chapter 3.2, Problem 73STP
a.
To determine
To write: an equation that represents cost of belonging to the gyms.
a.
Expert Solution
Answer to Problem 73STP
Explanation of Solution
Given:
There are two option that is shown below:
| OPTION 1 | OPTION 2 |
| $ 400/year | $150/ year |
| Unlimited visit | 5 visits |
Calculation:
Let x be the number of visits in the gym.
According to the question,
Hence, the system of the equation is
b.
To determine
To graph: the equation and estimate the break-even point for the gym membership.
b.
Expert Solution
Answer to Problem 73STP
Explanation of Solution
Given:
There are two option that is shown below:
| OPTION 1 | OPTION 2 |
| $ 400/year | $150/ year |
| Unlimited visit | 5 visits |
Calculation:
Let x be the number of visits in the gym.
From part (a),
Now, draw the graph of the above equation:
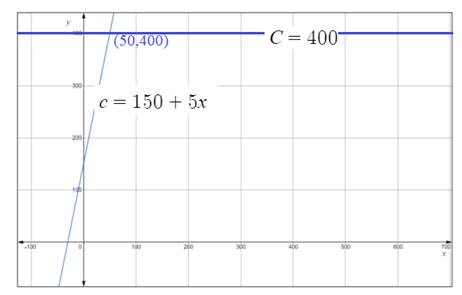
At break-even point:
Hence, the break-even point for the gym membership is
c.
To determine
To explain: the meaning of breakeven point.
c.
Expert Solution
Answer to Problem 73STP
Below 50 option 2 would be preferable.
Otherwise option 1 is preferable.
Explanation of Solution
Given:
There are two option that is shown below:
| OPTION 1 | OPTION 2 |
| $ 400/year | $150/ year |
| Unlimited visit | 5 visits |
Calculation:
Let x be the number of visits in the gym.
From part (a),
Breakeven point of means that if a person visits 50 times in a year then both the option will be equivalent.
Thus, below 50 option 2 would be preferable.
Otherwise option 1 is preferable.
At break-even point:
Hence, the break-even point for the gym membership is
c.
To determine
To explain: the meaning of breakeven point.
c.
Expert Solution
Answer to Problem 73STP
Below 50 option 2 would be preferable.
Otherwise option 1 is preferable.
Explanation of Solution
Given:
There are two option that is shown below:
| OPTION 1 | OPTION 2 |
| $ 400/year | $150/ year |
| Unlimited visit | 5 visits |
Calculation:
Let x be the number of visits in the gym.
From part (a),
Breakeven point of means that if a person visits 50 times in a year then both the option will be equivalent.
Thus, below 50 option 2 would be preferable.
Otherwise option 1 is preferable.
At break-even point:
d.
To determine
To explain: that which of the given option is preferable if he go once in a week.
d.
Expert Solution
Answer to Problem 73STP
Option 1 is preferable.
Explanation of Solution
Given:
There are two option that is shown below:
| OPTION 1 | OPTION 2 |
| $ 400/year | $150/ year |
| Unlimited visit | 5 visits |
Calculation:
If anybody goes gym once in a week, then they go 52 days in 1 year.
From part (c),
Below 50 option 2 would be preferable.
Otherwise option 1 is preferable.
Hence, option 1 is preferable.
Chapter 3 Solutions
Algebra 2
Ch. 3.1 - Prob. 1ACYPCh. 3.1 - Prob. 1BCYPCh. 3.1 - Prob. 2ACYPCh. 3.1 - Prob. 2BCYPCh. 3.1 - Prob. 3CYPCh. 3.1 - Prob. 4ACYPCh. 3.1 - Prob. 4BCYPCh. 3.1 - Prob. 4CCYPCh. 3.1 - Prob. 4DCYPCh. 3.1 - Prob. 1CYU
Ch. 3.1 - Prob. 2CYUCh. 3.1 - Prob. 3CYUCh. 3.1 - Prob. 4CYUCh. 3.1 - Prob. 5CYUCh. 3.1 - Prob. 6CYUCh. 3.1 - Prob. 7CYUCh. 3.1 - Prob. 8CYUCh. 3.1 - Prob. 9CYUCh. 3.1 - Prob. 10CYUCh. 3.1 - Prob. 11CYUCh. 3.1 - Prob. 12CYUCh. 3.1 - Prob. 13PPSCh. 3.1 - Prob. 14PPSCh. 3.1 - Prob. 15PPSCh. 3.1 - Prob. 16PPSCh. 3.1 - Prob. 17PPSCh. 3.1 - Prob. 18PPSCh. 3.1 - Prob. 19PPSCh. 3.1 - Prob. 20PPSCh. 3.1 - Prob. 21PPSCh. 3.1 - Prob. 22PPSCh. 3.1 - Prob. 23PPSCh. 3.1 - Prob. 24PPSCh. 3.1 - Prob. 25PPSCh. 3.1 - Prob. 26PPSCh. 3.1 - Prob. 27PPSCh. 3.1 - Prob. 28PPSCh. 3.1 - Prob. 29PPSCh. 3.1 - Prob. 30PPSCh. 3.1 - Prob. 31PPSCh. 3.1 - Prob. 32PPSCh. 3.1 - Prob. 33PPSCh. 3.1 - Prob. 34PPSCh. 3.1 - Prob. 35PPSCh. 3.1 - Prob. 36PPSCh. 3.1 - Prob. 37PPSCh. 3.1 - Prob. 38PPSCh. 3.1 - Prob. 39PPSCh. 3.1 - Prob. 40PPSCh. 3.1 - Prob. 41PPSCh. 3.1 - Prob. 42HPCh. 3.1 - Prob. 43HPCh. 3.1 - Prob. 44HPCh. 3.1 - Prob. 45HPCh. 3.1 - Prob. 46HPCh. 3.1 - Prob. 47HPCh. 3.1 - Prob. 48HPCh. 3.1 - Prob. 49HPCh. 3.1 - Prob. 50HPCh. 3.1 - Prob. 51STPCh. 3.1 - Prob. 52STPCh. 3.1 - Prob. 53STPCh. 3.1 - Prob. 54STPCh. 3.1 - Prob. 55STPCh. 3.1 - Prob. 56STPCh. 3.1 - Prob. 57STPCh. 3.1 - Prob. 58STPCh. 3.1 - Prob. 59STPCh. 3.1 - Prob. 60STPCh. 3.1 - Prob. 61SCh. 3.1 - Prob. 62SCh. 3.1 - Prob. 63SCh. 3.1 - Prob. 64SCh. 3.1 - Prob. 65SCh. 3.1 - Prob. 66SCh. 3.1 - Prob. 1ECh. 3.1 - Prob. 2ECh. 3.1 - Prob. 3ECh. 3.1 - Prob. 4ECh. 3.1 - Prob. 5ECh. 3.1 - Prob. 6ECh. 3.1 - Prob. 7ECh. 3.1 - Prob. 8ECh. 3.1 - Prob. 9ECh. 3.1 - Prob. 10ECh. 3.2 - Prob. 1ACYPCh. 3.2 - Prob. 1BCYPCh. 3.2 - Prob. 1CCYPCh. 3.2 - Prob. 2ACYPCh. 3.2 - Prob. 2BCYPCh. 3.2 - Prob. 2CCYPCh. 3.2 - Prob. 3CYPCh. 3.2 - Prob. 4ACYPCh. 3.2 - Prob. 4BCYPCh. 3.2 - Prob. 1CYUCh. 3.2 - Prob. 2CYUCh. 3.2 - Prob. 3CYUCh. 3.2 - Prob. 4CYUCh. 3.2 - Prob. 5CYUCh. 3.2 - Prob. 6CYUCh. 3.2 - Prob. 7CYUCh. 3.2 - Prob. 8CYUCh. 3.2 - Prob. 9CYUCh. 3.2 - Prob. 10CYUCh. 3.2 - Prob. 11CYUCh. 3.2 - Prob. 12CYUCh. 3.2 - Prob. 13CYUCh. 3.2 - Prob. 14CYUCh. 3.2 - Prob. 15PPSCh. 3.2 - Prob. 16PPSCh. 3.2 - Prob. 17PPSCh. 3.2 - Prob. 18PPSCh. 3.2 - Prob. 19PPSCh. 3.2 - Prob. 20PPSCh. 3.2 - Prob. 21PPSCh. 3.2 - Prob. 22PPSCh. 3.2 - Prob. 23PPSCh. 3.2 - Prob. 24PPSCh. 3.2 - Prob. 25PPSCh. 3.2 - Prob. 26PPSCh. 3.2 - Prob. 27PPSCh. 3.2 - Prob. 28PPSCh. 3.2 - Prob. 29PPSCh. 3.2 - Prob. 30PPSCh. 3.2 - Prob. 31PPSCh. 3.2 - Prob. 32PPSCh. 3.2 - Prob. 33PPSCh. 3.2 - Prob. 34PPSCh. 3.2 - Prob. 35PPSCh. 3.2 - Prob. 36PPSCh. 3.2 - Prob. 37PPSCh. 3.2 - Prob. 38PPSCh. 3.2 - Prob. 39PPSCh. 3.2 - Prob. 40PPSCh. 3.2 - Prob. 41PPSCh. 3.2 - Prob. 42PPSCh. 3.2 - Prob. 43PPSCh. 3.2 - Prob. 44PPSCh. 3.2 - Prob. 45PPSCh. 3.2 - Prob. 46PPSCh. 3.2 - Prob. 47PPSCh. 3.2 - Prob. 48PPSCh. 3.2 - Prob. 49PPSCh. 3.2 - Prob. 50PPSCh. 3.2 - Prob. 51PPSCh. 3.2 - Prob. 52PPSCh. 3.2 - Prob. 53PPSCh. 3.2 - Prob. 54PPSCh. 3.2 - Prob. 55PPSCh. 3.2 - Prob. 56PPSCh. 3.2 - Prob. 57PPSCh. 3.2 - Prob. 58PPSCh. 3.2 - Prob. 59PPSCh. 3.2 - Prob. 60PPSCh. 3.2 - Prob. 61PPSCh. 3.2 - Prob. 62PPSCh. 3.2 - Prob. 63PPSCh. 3.2 - Prob. 64HPCh. 3.2 - Prob. 65HPCh. 3.2 - Prob. 66HPCh. 3.2 - Prob. 67HPCh. 3.2 - Prob. 68HPCh. 3.2 - Prob. 69HPCh. 3.2 - Prob. 70HPCh. 3.2 - Prob. 71HPCh. 3.2 - Prob. 72HPCh. 3.2 - Prob. 73STPCh. 3.2 - Prob. 74STPCh. 3.2 - Prob. 75STPCh. 3.2 - Prob. 76STPCh. 3.2 - Prob. 77STPCh. 3.2 - Prob. 78STPCh. 3.2 - Prob. 79STPCh. 3.2 - Prob. 80STPCh. 3.2 - Prob. 81SCh. 3.2 - Prob. 82SCh. 3.2 - Prob. 83SCh. 3.3 - Prob. 1ACYPCh. 3.3 - Prob. 1BCYPCh. 3.3 - Prob. 2ACYPCh. 3.3 - Prob. 2BCYPCh. 3.3 - Prob. 3CYPCh. 3.3 - Prob. 4ACYPCh. 3.3 - Prob. 4BCYPCh. 3.3 - Prob. 1CYUCh. 3.3 - Prob. 2CYUCh. 3.3 - Prob. 3CYUCh. 3.3 - Prob. 4CYUCh. 3.3 - Prob. 5CYUCh. 3.3 - Prob. 6CYUCh. 3.3 - Prob. 7PPSCh. 3.3 - Prob. 8PPSCh. 3.3 - Prob. 9PPSCh. 3.3 - Prob. 10PPSCh. 3.3 - Prob. 11PPSCh. 3.3 - Prob. 12PPSCh. 3.3 - Prob. 13PPSCh. 3.3 - Prob. 14PPSCh. 3.3 - Prob. 15PPSCh. 3.3 - Prob. 16PPSCh. 3.3 - Prob. 17PPSCh. 3.3 - Prob. 18PPSCh. 3.3 - Prob. 19PPSCh. 3.3 - Prob. 20PPSCh. 3.3 - Prob. 21PPSCh. 3.3 - Prob. 22PPSCh. 3.3 - Prob. 23PPSCh. 3.3 - Prob. 24PPSCh. 3.3 - Prob. 25PPSCh. 3.3 - Prob. 26PPSCh. 3.3 - Prob. 27PPSCh. 3.3 - Prob. 28PPSCh. 3.3 - Prob. 29PPSCh. 3.3 - Prob. 30PPSCh. 3.3 - Prob. 31PPSCh. 3.3 - Prob. 32PPSCh. 3.3 - Prob. 33PPSCh. 3.3 - Prob. 34PPSCh. 3.3 - Prob. 35PPSCh. 3.3 - Prob. 36PPSCh. 3.3 - Prob. 37PPSCh. 3.3 - Prob. 38PPSCh. 3.3 - Prob. 39PPSCh. 3.3 - Prob. 40PPSCh. 3.3 - Prob. 41PPSCh. 3.3 - Prob. 42PPSCh. 3.3 - Prob. 43PPSCh. 3.3 - Prob. 44PPSCh. 3.3 - Prob. 45HPCh. 3.3 - Prob. 46HPCh. 3.3 - Prob. 47HPCh. 3.3 - Prob. 48HPCh. 3.3 - Prob. 49HPCh. 3.3 - Prob. 50HPCh. 3.3 - Prob. 51HPCh. 3.3 - Prob. 52HPCh. 3.3 - Prob. 53HPCh. 3.3 - Prob. 54HPCh. 3.3 - Prob. 55STPCh. 3.3 - Prob. 56STPCh. 3.3 - Prob. 57STPCh. 3.3 - Prob. 58STPCh. 3.3 - Prob. 59STPCh. 3.3 - Prob. 60STPCh. 3.3 - Prob. 61STPCh. 3.3 - Prob. 62STPCh. 3.3 - Prob. 63SCh. 3.3 - Prob. 64SCh. 3.3 - Prob. 65SCh. 3.3 - Prob. 66SCh. 3.3 - Prob. 67SCh. 3.3 - Prob. 68SCh. 3.3 - Prob. 1ECh. 3.3 - Prob. 2ECh. 3.3 - Prob. 3ECh. 3.3 - Prob. 4ECh. 3.3 - Prob. 5ECh. 3.3 - Prob. 6ECh. 3.3 - Prob. 7ECh. 3.3 - Prob. 8ECh. 3.3 - Prob. 9ECh. 3.4 - Prob. 1ACYPCh. 3.4 - Prob. 1BCYPCh. 3.4 - Prob. 2ACYPCh. 3.4 - Prob. 2BCYPCh. 3.4 - Prob. 3CYPCh. 3.4 - Prob. 1CYUCh. 3.4 - Prob. 2CYUCh. 3.4 - Prob. 3CYUCh. 3.4 - Prob. 4CYUCh. 3.4 - Prob. 5CYUCh. 3.4 - Prob. 6CYUCh. 3.4 - Prob. 7CYUCh. 3.4 - Prob. 8PPSCh. 3.4 - Prob. 9PPSCh. 3.4 - Prob. 10PPSCh. 3.4 - Prob. 11PPSCh. 3.4 - Prob. 12PPSCh. 3.4 - Prob. 13PPSCh. 3.4 - Prob. 14PPSCh. 3.4 - Prob. 15PPSCh. 3.4 - Prob. 16PPSCh. 3.4 - Prob. 17PPSCh. 3.4 - Prob. 18PPSCh. 3.4 - Prob. 19PPSCh. 3.4 - Prob. 20PPSCh. 3.4 - Prob. 21PPSCh. 3.4 - Prob. 22PPSCh. 3.4 - Prob. 23PPSCh. 3.4 - Prob. 24PPSCh. 3.4 - Prob. 25PPSCh. 3.4 - Prob. 26PPSCh. 3.4 - Prob. 27PPSCh. 3.4 - Prob. 28PPSCh. 3.4 - Prob. 29PPSCh. 3.4 - Prob. 30HPCh. 3.4 - Prob. 31HPCh. 3.4 - Prob. 32HPCh. 3.4 - Prob. 33HPCh. 3.4 - Prob. 34HPCh. 3.4 - Prob. 35HPCh. 3.4 - Prob. 36HPCh. 3.4 - Prob. 37HPCh. 3.4 - Prob. 38STPCh. 3.4 - Prob. 39STPCh. 3.4 - Prob. 40STPCh. 3.4 - Prob. 41STPCh. 3.4 - Prob. 42STPCh. 3.4 - Prob. 43STPCh. 3.4 - Prob. 44STPCh. 3.4 - Prob. 45STPCh. 3.4 - Prob. 46STPCh. 3.4 - Prob. 47STPCh. 3.4 - Prob. 48STPCh. 3.4 - Prob. 49STPCh. 3.4 - Prob. 50SCh. 3.4 - Prob. 51SCh. 3.4 - Prob. 52SCh. 3.4 - Prob. 53SCh. 3.4 - Prob. 54SCh. 3.4 - Prob. 55SCh. 3.5 - Prob. 1ACYPCh. 3.5 - Prob. 1BCYPCh. 3.5 - Prob. 2ACYPCh. 3.5 - Prob. 2BCYPCh. 3.5 - Prob. 3CYPCh. 3.5 - Prob. 1CYUCh. 3.5 - Prob. 2CYUCh. 3.5 - Prob. 3CYUCh. 3.5 - Prob. 4CYUCh. 3.5 - Prob. 5CYUCh. 3.5 - Prob. 6CYUCh. 3.5 - Prob. 7CYUCh. 3.5 - Prob. 8PPSCh. 3.5 - Prob. 9PPSCh. 3.5 - Prob. 10PPSCh. 3.5 - Prob. 11PPSCh. 3.5 - Prob. 12PPSCh. 3.5 - Prob. 13PPSCh. 3.5 - Prob. 14PPSCh. 3.5 - Prob. 15PPSCh. 3.5 - Prob. 16PPSCh. 3.5 - Prob. 17PPSCh. 3.5 - Prob. 18PPSCh. 3.5 - Prob. 19PPSCh. 3.5 - Prob. 20PPSCh. 3.5 - Prob. 21PPSCh. 3.5 - Prob. 22PPSCh. 3.5 - Prob. 23PPSCh. 3.5 - Prob. 24HPCh. 3.5 - Prob. 25HPCh. 3.5 - Prob. 26HPCh. 3.5 - Prob. 27HPCh. 3.5 - Prob. 28HPCh. 3.5 - Prob. 29HPCh. 3.5 - Prob. 30HPCh. 3.5 - Prob. 31HPCh. 3.5 - Prob. 32HPCh. 3.5 - Prob. 33HPCh. 3.5 - Prob. 34STPCh. 3.5 - Prob. 35STPCh. 3.5 - Prob. 36STPCh. 3.5 - Prob. 37STPCh. 3.5 - Prob. 38STPCh. 3.5 - Prob. 39SCh. 3.5 - Prob. 40SCh. 3.5 - Prob. 41SCh. 3.5 - Prob. 42SCh. 3 - Prob. 1QCCh. 3 - Prob. 2QCCh. 3 - Prob. 3QCCh. 3 - Prob. 4QCCh. 3 - Prob. 5QCCh. 3 - Prob. 6QCCh. 3 - Prob. 7QCCh. 3 - Prob. 8QCCh. 3 - Prob. 9QCCh. 3 - Prob. 10QCCh. 3 - Prob. 11QCCh. 3 - Prob. 12QCCh. 3 - Prob. 13QCCh. 3 - Prob. 14QCCh. 3 - Prob. 1MCQCh. 3 - Prob. 2MCQCh. 3 - Prob. 3MCQCh. 3 - Prob. 4MCQCh. 3 - Prob. 5MCQCh. 3 - Prob. 6MCQCh. 3 - Prob. 7MCQCh. 3 - Prob. 8MCQCh. 3 - Prob. 9MCQCh. 3 - Prob. 10MCQCh. 3 - Prob. 11MCQCh. 3 - Prob. 12MCQCh. 3 - Prob. 13MCQCh. 3 - Prob. 14MCQCh. 3 - Prob. 15MCQCh. 3 - Prob. 16MCQCh. 3 - Prob. 17MCQCh. 3 - Prob. 18MCQCh. 3 - Prob. 19MCQCh. 3 - Prob. 20MCQCh. 3 - Prob. 21MCQCh. 3 - Prob. 22MCQCh. 3 - Prob. 1SGRCh. 3 - Prob. 2SGRCh. 3 - Prob. 3SGRCh. 3 - Prob. 4SGRCh. 3 - Prob. 5SGRCh. 3 - Prob. 6SGRCh. 3 - Prob. 7SGRCh. 3 - Prob. 8SGRCh. 3 - Prob. 9SGRCh. 3 - Prob. 10SGRCh. 3 - Prob. 11SGRCh. 3 - Prob. 12SGRCh. 3 - Prob. 13SGRCh. 3 - Prob. 14SGRCh. 3 - Prob. 15SGRCh. 3 - Prob. 16SGRCh. 3 - Prob. 17SGRCh. 3 - Prob. 18SGRCh. 3 - Prob. 19SGRCh. 3 - Prob. 20SGRCh. 3 - Prob. 21SGRCh. 3 - Prob. 22SGRCh. 3 - Prob. 23SGRCh. 3 - Prob. 24SGRCh. 3 - Prob. 25SGRCh. 3 - Prob. 26SGRCh. 3 - Prob. 27SGRCh. 3 - Prob. 28SGRCh. 3 - Prob. 29SGRCh. 3 - Prob. 30SGRCh. 3 - Prob. 1PTCh. 3 - Prob. 2PTCh. 3 - Prob. 3PTCh. 3 - Prob. 4PTCh. 3 - Prob. 5PTCh. 3 - Prob. 6PTCh. 3 - Prob. 7PTCh. 3 - Prob. 8PTCh. 3 - Prob. 9PTCh. 3 - Prob. 10PTCh. 3 - Prob. 11PTCh. 3 - Prob. 12PTCh. 3 - Prob. 13PTCh. 3 - Prob. 14PTCh. 3 - Prob. 15PTCh. 3 - Prob. 16PTCh. 3 - Prob. 17PTCh. 3 - Prob. 18PTCh. 3 - Prob. 19PTCh. 3 - Prob. 1ECh. 3 - Prob. 2ECh. 3 - Prob. 3ECh. 3 - Prob. 4ECh. 3 - Prob. 1STPCh. 3 - Prob. 2STPCh. 3 - Prob. 3STPCh. 3 - Prob. 4STPCh. 3 - Prob. 5STPCh. 3 - Prob. 6STPCh. 3 - Prob. 7STPCh. 3 - Prob. 8STPCh. 3 - Prob. 9STPCh. 3 - Prob. 10STPCh. 3 - Prob. 11STPCh. 3 - Prob. 12STPCh. 3 - Prob. 13STP
Additional Math Textbook Solutions
Find more solutions based on key concepts
Two dice are thrown. Let E be the event that the sum of the dice is odd, let F be the event that at least one o...
A First Course in Probability (10th Edition)
Views on Capital Punishment In carrying out a study of views on capital punishment, a student asked a question ...
Introductory Statistics
Identify f as being linear, quadratic, or neither. If f is quadratic, identify the leading coefficient a and ...
College Algebra with Modeling & Visualization (5th Edition)
the given equation
Pre-Algebra Student Edition
Fill in each blank so that the resulting statement is true. The quadratic function f(x)=a(xh)2+k,a0, is in ____...
Algebra and Trigonometry (6th Edition)
Assessment 1-1A Cookies are sold singly or in packages of 2 or 6. With this packaging, how many ways can you bu...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- 1. vector projection. Assume, ER1001 and you know the following: ||||=4, 7=-0.5.7. For each of the following, explicitly compute the value. འབ (a) (b) (c) (d) answer. Explicitly compute ||y7||. Explain your answer. Explicitly compute the cosine similarity of and y. Explain your Explicitly compute (x, y). Explain your answer. Find the projection of onto y and the projection of onto .arrow_forward2. Answer the following questions using vectors u and v. --0-0-0 = find the the cosine similarity and the angle between u and v. འརྒྱ (a) (b) find the scalar projection of u onto v. (c) find the projection of u onto v. (d) (e) (f) find the scalar projection of onto u. find the projection of u onto u. find the projection of u onto and the projection of onto . (Hint: find the inner product and verify the orthogonality)arrow_forwardPlease type out answerarrow_forward
- The function f(x) = log x is transformed to produce g(x) = log (x) – 3. Identify the type of transformation and describe the change. Please type out answerarrow_forwardEach graph below is the graph of a system of three linear equations in three unknowns of the form Ax = b. Determine whether each system has a solution and, if it does, the number of free variables. A. O free variables ✓ B. no solution C. no solution D. no solution E. 1 free variable F. 1 free variablearrow_forwardSolve the following systems of equations and show all work.y = x2 + 3y = x + 5 Please type out answerarrow_forward
- Solve the following system of equations. Show all work and solutions.y = 2x2 + 6x + 1y = −4x2 + 1 Please type out answerarrow_forwardDalia buys 20 collectible gems per month. Grace sells 10 gems from her collection of 120 each month. When will Dalia have more gems than Grace? Show your work. Dear Student If You Face any issue let me know i will solve your all doubt. I will provide solution again in more detail systematic and organized way. I would also like my last 3 questions credited to mearrow_forwardDalia buys 20 collectible gems per month. Grace sells 10 gems from her collection of 120 each month. When will Dalia have more gems than Grace? Show your work.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON
Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON
Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education
Basic Reflection Trigonometric Identity; Author: Anil kumar;https://www.youtube.com/watch?v=y-EGUD49fmw;License: Standard YouTube License, CC-BY