Chapter 3.4, Problem 27PPS

a.

To determine

To calculate:The number of small and large package to maximize the revenue.

Answer to Problem 27PPS

The number of small packages are 160 to have maximum revenue.

Explanation of Solution

Given information:

The small packages weigh 25 pounds and 3 cubic feet capacity each. Large packages weigh 50 pounds and have 5 cubic capacity each. 4200 pounds of cargo and capacity of 480 cubic feet is hold by train. $5 is charged for each small package and $8 is charged for each large package.

Calculation:

Consider the provided information thatsmall packages weigh 25 pounds and 3 cubic feet capacity each. Large packages weigh 50 pounds and have 5 cubic capacity each. 4200 pounds of cargo and capacity of 480 cubic feet is hold by train. $5 is charged for each small package and $8 is charged for each large package.

Let x denote the number of small package and y denote the number of large packages.

The constraints are,

  $\begin{array}{c}x\ge 0\\ y\ge 0\\ 25x+50y\le 4200\\ 3x+5y\le 480\end{array}$

Since, $5 is charged for each small package and $8 is charged for each large package.

The objective function or revenue function is,

  $f\left(x,y\right)=5x+8y$

Plot the above inequalities on coordinate plane.

  

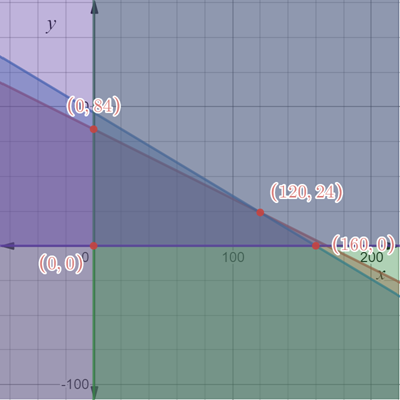
The shaded region represents the feasible. It is a quadrilateral with 4 corner points. The coordinates of feasible region are $\left(0,0\right),\left(0,84\right),\left(120,24\right)\text{ and }\left(160,0\right)$ .

Substitute the vertices of the feasible region to find the point at which maximum profit is there.

Substitute $\left(0,0\right)$ in the function $f\left(x,y\right)=5x+8y$ .

  $f\left(0,0\right)=0$

Substitute $\left(0,84\right)$ in the function $f\left(x,y\right)=5x+8y$ .

  $f\left(0,84\right)=672$

Substitute $\left(160,0\right)$ in the function $f\left(x,y\right)=5x+8y$ .

  $f\left(160,0\right)=800$

Substitute $\left(120,24\right)$ in the function $f\left(x,y\right)=5x+8y$ .

  $f\left(120,24\right)=792$

Thus, the maximum revenue is when 160 small packages are placed.

b.

To determine

To calculate:The maximum revenue per train car.

Answer to Problem 27PPS

The maximum revenue per train car is $800.

Explanation of Solution

Given information:

The small packages weigh 25 pounds and 3 cubic feet capacity each. Large packages weigh 50 pounds and have 5 cubic capacity each. 4200 pounds of cargo and capacity of 480 cubic feet is hold by train. $5 is charged for each small package and $8 is charged for each large package.

Calculation:

Consider the provided information thatsmall packages weigh 25 pounds and 3 cubic feet capacity each. Large packages weigh 50 pounds and have 5 cubic capacity each. 4200 pounds of cargo and capacity of 480 cubic feet is hold by train. $5 is charged for each small package and $8 is charged for each large package.

Let x denote the number of small package and y denote the number of large packages.

The constraints are,

  $\begin{array}{c}x\ge 0\\ y\ge 0\\ 25x+50y\le 4200\\ 3x+5y\le 480\end{array}$

Since, $5 is charged for each small package and $8 is charged for each large package.

The objective function or revenue function is,

  $f\left(x,y\right)=5x+8y$

Plot the above inequalities on coordinate plane.

  

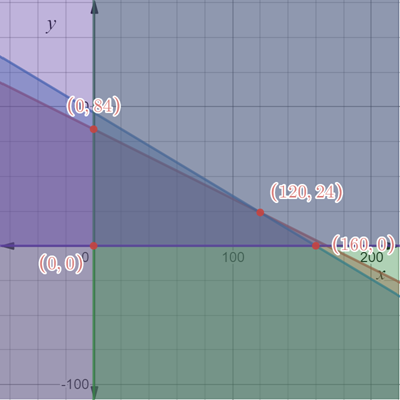
The shaded region represents the feasible. It is a quadrilateral with 4 corner points. The coordinates of feasible region are $\left(0,0\right),\left(0,84\right),\left(120,24\right)\text{ and }\left(160,0\right)$ .

Substitute the vertices of the feasible region to find the point at which maximum profit is there.

Substitute $\left(0,0\right)$ in the function $f\left(x,y\right)=5x+8y$ .

  $f\left(0,0\right)=0$

Substitute $\left(0,84\right)$ in the function $f\left(x,y\right)=5x+8y$ .

  $f\left(0,84\right)=672$

Substitute $\left(160,0\right)$ in the function $f\left(x,y\right)=5x+8y$ .

  $f\left(160,0\right)=800$

Substitute $\left(120,24\right)$ in the function $f\left(x,y\right)=5x+8y$ .

  $f\left(120,24\right)=792$

Thus, the maximum revenue is $800 which is when 160 small packages and 0 large packages are placed.

c.

To determine

To explain:The maximization of revenue per train car is benefitted for the company or not.

Answer to Problem 27PPS

The maximization of revenue per train car is notbenefitted for the company. As people cannot ship large packages from one destination to other.

Explanation of Solution

Given information:

The small packages weigh 25 pounds and 3 cubic feet capacity each. Large packages weigh 50 pounds and have 5 cubic capacity each. 4200 pounds of cargo and capacity of 480 cubic feet is hold by train. $5 is charged for each small package and $8 is charged for each large package.

Calculation:

Consider the provided information thatsmall packages weigh 25 pounds and 3 cubic feet capacity each. Large packages weigh 50 pounds and have 5 cubic capacity each. 4200 pounds of cargo and capacity of 480 cubic feet is hold by train. $5 is charged for each small package and $8 is charged for each large package.

Let x denote the number of small package and y denote the number of large packages.

The constraints are,

  $\begin{array}{c}x\ge 0\\ y\ge 0\\ 25x+50y\le 4200\\ 3x+5y\le 480\end{array}$

Since, $5 is charged for each small package and $8 is charged for each large package.

The objective function or revenue function is,

  $f\left(x,y\right)=5x+8y$

Plot the above inequalities on coordinate plane.

  

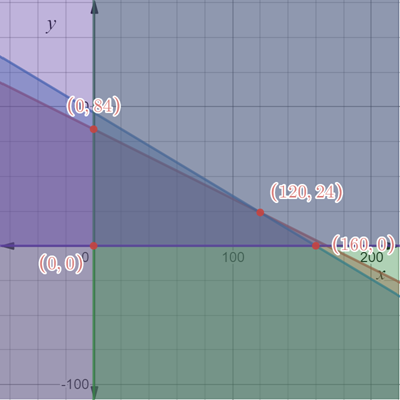
The shaded region represents the feasible. It is a quadrilateral with 4 corner points. The coordinates of feasible region are $\left(0,0\right),\left(0,84\right),\left(120,24\right)\text{ and }\left(160,0\right)$ .

Substitute the vertices of the feasible region to find the point at which maximum profit is there.

Substitute $\left(0,0\right)$ in the function $f\left(x,y\right)=5x+8y$ .

  $f\left(0,0\right)=0$

Substitute $\left(0,84\right)$ in the function $f\left(x,y\right)=5x+8y$ .

  $f\left(0,84\right)=672$

Substitute $\left(160,0\right)$ in the function $f\left(x,y\right)=5x+8y$ .

  $f\left(160,0\right)=800$

Substitute $\left(120,24\right)$ in the function $f\left(x,y\right)=5x+8y$ .

  $f\left(120,24\right)=792$

Thus, the maximum revenue is $800 which is when 160 small packages and 0 large packages are placed.

The maximization of revenue per train car is not benefitted for the company. As people cannot ship large packages from one destination to other.

As zero large packaged are placed in train for maximum revenue so people will look for carrier alternatives to transfer large packages from one place to another.