Fundamentals of Physics Extended
10th Edition
ISBN: 9781118230725
Author: David Halliday, Robert Resnick, Jearl Walker
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 31, Problem 93P
When the generator emf in Sample Problem 31.07 is a maximum, what is the voltage across (a) the generator, (b) the resistance, (c) the capacitance, and (d) the inductance? (e) By summing these with appropriate signs, verify that the loop rule is satisfied.
Sample Problem 31.06
In Fig. 31-7, let R = 200 Ω, C = 15.0 µF, L = 230 mH, fd = 60.0 Hz, and ℰm = 36.0 V. (These parameters are those used in the earlier sample problems.)
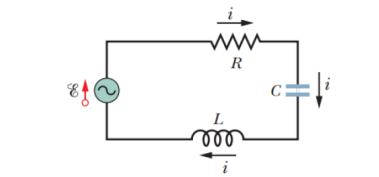
Figure 31-7 A single-loop circuit containing a resistor, a capacitor, and an inductor. A generator, represented by a sine wave in a circle, produces an alternating emf that establishes an alternating current; the directions of the emf and current are indicated here at only one instant.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
A series RCL circuit contains a 5.10-μF capacitor and a generator whose voltage is 11.0 V. At a resonant frequency of 1.30 kHz the power delivered to the circuit is 25.0 W. Find the values of (a) the inductance and (b) the resistance.
An ac generator with emf em sin vdt, where m e=25.0 V and vd 377 rad/s, is connected to a 4.15 mF capacitor. (a)What is the maximum value of the current? (b) When the current is a maximum, what is the emf of the generator? (c)When the emf of the generator is -12.5 V and increasing in magnitude, what is the current?
An RLC series circuit has a voltage source given by E(t)=40V, a resistor of 140 Ω, an inductor of 4H, and a capacitor of 0.01F. If the initial current is zero and the initial charge on the capacitor is 5C, determine the current in the circuit for t>0.
Chapter 31 Solutions
Fundamentals of Physics Extended
Ch. 31 - Figure 31-19 shows three oscillating LC circuits...Ch. 31 - Figure 31-20 shows graphs of capacitor voltage vc...Ch. 31 - Prob. 3QCh. 31 - What values of phase constant in Eq. 31-12 allow...Ch. 31 - Curve a in Fig. 31-21 gives the impedance Z of a...Ch. 31 - Prob. 6QCh. 31 - Prob. 7QCh. 31 - The values of the phase constant for four...Ch. 31 - Prob. 9QCh. 31 - Figure 31-24 shows three situations like those of...
Ch. 31 - Prob. 11QCh. 31 - Figure 31-25 shows the current i and driving emf ...Ch. 31 - Prob. 13QCh. 31 - An oscillating LC circuit consists of a 75.0 mH...Ch. 31 - The frequency of oscillation of a certain LC...Ch. 31 - In a certain oscillating LC circuit, the total...Ch. 31 - What is the capacitance of an oscillating LC...Ch. 31 - In an oscillating LC circuit, L = 1.10 mH and C =...Ch. 31 - A 0.50 kg body oscillates in SHM on a spring that,...Ch. 31 - SSM The energy in an oscillating LC circuit...Ch. 31 - A single loop consists of inductors L1, L2, . . ....Ch. 31 - ILW In an oscillating LC circuit with L = 50 mH...Ch. 31 - Prob. 10PCh. 31 - SSM WWW A variable capacitor with a range from 10...Ch. 31 - In an oscillating LC circuit, when 75.0 of the...Ch. 31 - In an oscillating LC circuit, L = 3.00 mH and C =...Ch. 31 - To construct an oscillating LC system, you can...Ch. 31 - ILW An oscillating LC circuit consisting of a 1.0...Ch. 31 - An inductor is connected across a capacitor whose...Ch. 31 - Prob. 17PCh. 31 - Prob. 18PCh. 31 - Using the loop rule, derive the differential...Ch. 31 - GO In an oscillating LC circuit in which C = 4.00...Ch. 31 - Prob. 21PCh. 31 - A series circuit containing inductance L1 and...Ch. 31 - GO In an oscillating LC circuit, L = 25.0 mH and C...Ch. 31 - Prob. 24PCh. 31 - Prob. 25PCh. 31 - GO In an oscillating series RLC circuit, find the...Ch. 31 - SSM In an oscillating series RLC circuit, show...Ch. 31 - A 1.50 F capacitor is connected as in Fig. 31-10...Ch. 31 - ILW A 50.0 mH inductor is connected as in Fig....Ch. 31 - A 50.0 resistor is connected as in Fig. 31-8 to...Ch. 31 - a At what frequency would a 6.0 mH inductor and a...Ch. 31 - GO An ac generator has emf = m sin dt, with m =...Ch. 31 - SSM An ac generator has emf = m sindt = /4, where...Ch. 31 - GO An ac generator with emf = m sin dt, where m =...Ch. 31 - ILW A coil of inductance 88 mH and unknown...Ch. 31 - An alternating source with a variable frequency, a...Ch. 31 - An electric motor has an effective resistance of...Ch. 31 - The current amplitude I versus driving angular...Ch. 31 - Remove the inductor from the circuit in Fig. 31-7...Ch. 31 - An alternating source drives a series RLC circuit...Ch. 31 - Prob. 41PCh. 31 - An alternating source with a variable frequency,...Ch. 31 - Prob. 43PCh. 31 - GO An ac generator with emf amplitude m = 220 V...Ch. 31 - GO ILW a In an RLC circuit, can the amplitude of...Ch. 31 - GO An alternating emf source with a variable...Ch. 31 - SSM WWW An RLC circuit such as that of Fig. 31-7...Ch. 31 - Prob. 48PCh. 31 - GO In Fig. 31-33, a generator with an adjustable...Ch. 31 - An alternating emf source with a variable...Ch. 31 - SSM The fractional half-width d of a resonance...Ch. 31 - An ac voltmeter with large impedance is connected...Ch. 31 - SSM An air conditioner connected to a 120 V rms ac...Ch. 31 - What is the maximum value of an ac voltage whose...Ch. 31 - What direct current will produce the same amount...Ch. 31 - Prob. 56PCh. 31 - Prob. 57PCh. 31 - For Fig. 31 -35, show that the average rate at...Ch. 31 - GO In Fig. 31-7, R = 15.0 , C = 4.70 F, and L =...Ch. 31 - Prob. 60PCh. 31 - SSM WWW Figure 31-36 shows an ac generator...Ch. 31 - Prob. 62PCh. 31 - SSM ILW A transformer has 500 primary turns and 10...Ch. 31 - Prob. 64PCh. 31 - An ac generator provides emf to a resistive load...Ch. 31 - In Fig. 31-35, let the rectangular box on the left...Ch. 31 - GO An ac generator produces emf = m sindt /4,...Ch. 31 - A series RLC circuit is driven by a generator at a...Ch. 31 - A generator of frequency 3000 Hz drives a series...Ch. 31 - A 45.0 mH inductor has a reactance of 1.30 k. a...Ch. 31 - An RLC circuit is driven by a generator with an...Ch. 31 - A series RLC circuit is driven in such a way that...Ch. 31 - A capacitor of capacitance 158 f and an inductor...Ch. 31 - An oscillating LC circuit has an inductance of...Ch. 31 - For a certain driven series RLC circuit, the...Ch. 31 - A L5D F capacitor has a capacitive re ac lance of...Ch. 31 - Prob. 77PCh. 31 - An electric motor connected to a 120 V, 60.0 Hz ac...Ch. 31 - SSM a In an oscillating LC circuit in terms of the...Ch. 31 - A series RLC circuit is driven by an alternating...Ch. 31 - SSM In a certain series RLC circuit being driven...Ch. 31 - A 1.50 mH inductor in an oscillating LC circuit...Ch. 31 - A generator with an adjustable frequency of...Ch. 31 - A series RLC circuit has a resonant frequency of...Ch. 31 - SSM An LC circuit oscillates at a frequency of...Ch. 31 - When under load and operating at an rms voltage of...Ch. 31 - The ac generator in Fig. 31-39 supplies 120 V at...Ch. 31 - In an oscillating LC circuit, L = 8.00 mH and C =...Ch. 31 - Prob. 89PCh. 31 - What capacitance would you connect across a 1.30...Ch. 31 - A series circuit with resistor inductor ...Ch. 31 - Prob. 92PCh. 31 - When the generator emf in Sample Problem 31.07 is...
Additional Science Textbook Solutions
Find more solutions based on key concepts
WHAT IF? In 2005, at least ten grizzly bears in the greater Yellowstone ecosystem were killed through contact w...
Campbell Biology in Focus (2nd Edition)
What are four functions of connective tissue?
Anatomy & Physiology (6th Edition)
Flask A contains yeast cells in glucose-minimal salts broth incubated at 30C with aeration. Flask B contains ye...
Microbiology: An Introduction
The structure of thyroxine, a thyroid hormone that helps to regulate metabolic rate, was determined in part by ...
Organic Chemistry
Carefully examine the common sedimentary rocks shown In Figure 2.13. Use these photos and the preceding discuss...
Applications and Investigations in Earth Science (9th Edition)
Your bore cells, muscle cells, and skin cells look different because a. different kinds of genes are present in...
Campbell Essential Biology (7th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- An RLC series circuit has a voltage source given by E(t)=20 V, a resistor of 150 Ω, an inductor of 6 H, and a capacitor of 0.05 F. If the initial current is zero and the initial charge on the capacitor is 4 C, determine the current in the circuit for t>0. I(t)=?arrow_forwardA simple electric circuit consisting of a resistor, a capacitor, and an inductor is depicted as shown below. The charge on the capacitor q(t) as a function of time can be computed as 9(1) = goe-Rt/(2L) Cos LC 2L where t = time, q0 = the initial charge, R = the resistance, L = inductance, and C = capacitance. Use MATLAB to generate a plot of this function from t= 0 to 0.8 with an increment of 0.001, given that q0= 10, R = 60, L = 9, and C = 0.00005. What is the maximum value of q= Blank 1? Express your answer to the nearest whole number. Blank 1 Add your answerarrow_forwardProblem 2: An AC voltage source is connected to an inductor with inductance 0.046 H. The voltage source provides a time-dependent voltage V across the inductor as described by V(t) = vo sin(wt), where vo = 260 V and w = 997 rad/s. Part (a) Find the current through the inductor, in amperes with its sign, at time t = 1.5 s. Numeric : A numeric value is expected and not an expression. i = Part (b) Calculate the instantaneous power, in watts with its sign, that the inductor is absorbing at time t = 1.5 s. Numeric : A numeric value is expected and not an expression. p(t) =arrow_forward
- Problem 1: An AC current source is connected to an inductor with an inductance L = 6 H. At time t= 0, the current through the inductor is io 0 A. The power supply provides a time-dependent current i through the inductor as described by the following equation : i()-10.cos(ot), where ω-500 rad/s. The current is measured in units of Amps ions sharing Part (a) Determine the current through the inductor, in amperes, at time t 5.5 sec. Δ tan() acos() sinh() cos(0) HOME sin cotan atan()a 4 5 6 acotansin cosh) tanhO cotanh ODegrees O Radians 0 BACKSPACE CLEAR Submit I give up! Hints: 0 for a 0% deduction. Hints remaining: 0 Feedback: 1% deduction per feedback. Part (b) Determine the voltage across the inductor, in volts, at time t 5.5 sec. A Part (c) Determine the magnitude of the maximum voltage, in volts, across the inductor.arrow_forwardAn L-R-C series circuit has inductance 42.0 mH, capacitanceC, and resistance R. Without the resistor, the angular frequencyof oscillation is 624 rad/s. With the resistor, the angular frequency is208 rad/s. Find the values of (a) C and (b) R.arrow_forwardAn inductor with inductance L is connected to an AC source. If the AC source provides a voltage VL(t) = V0cos(omegat), what is the current IL through the inductor as a function of time? Use the cosine function.arrow_forward
- At t = 0 a current of 3 amperes flows in an RLC circuit with resistance 4 ohms, inductance 0.5 henrys, and capacitance 0.001 farads. The initial charge on the capacitor is 9 coulomb. Assume that the impressed voltage, provided by an alternating current generator, is E(t) 10 cos(7t). Note: The phase-angle is between 0 and r. (a) Find the charge in the circuit at time t = 5. (b) Find the amplitude-phase form of the steady state current in the RLC flowing in the circuit, and evaluate it at t = 105. (c) Find the amplitude-phase form of the steady state current in the RLC flowing in the circuit if at t = 0 a current of 6 amperes flowed in the RLC circuit, and the initial charge on the capacitor is 18 coulomb. Evaluate the function you obtain at t = 105.arrow_forwardA series RLC circuit driven by a source with an amplitude of 120.0 V and a frequency of 50.0 Hz has an inductance of 767 mH, a resistance of 278 N, and a capacitance of 42.9 µF. (a) What are the maximum current and the phase angle between the current and the source emf in this circuit? Imax = A Pミ (b) What are the maximum potential difference across the inductor and the phase angle between this potential difference and the current in the circuit? VL, max V P = (c) What are the maximum potential difference across the resistor and the phase angle between this potential difference and the current in this circuit? VR, max P = (d) What are the maximum potential difference across the capacitor and the phase angle between this potential difference and the current in this circuit? Vc, max Varrow_forwardAt what frequency will the inductive reactance of a 0.0211 H inductor be equal to the capacitive reactance of a 75 μF capacitor?arrow_forward
- A series RLC circuit is driven in such a way that the maximum voltage across the inductor is 1.50 times the maximum voltage across the capacitor and 2.10 times the maximum voltage across the resistor. (a) What is o for the circuit? The resistance is 51.8 Q and the current amplitude is 150 mA. (b) What is the amplitude of the driving emf? (a) Number i Units (b) Number i Unitsarrow_forwardIn an RLC circuit assume that R =5.00 , L =60.0 mH, fd = 60.0 Hz, and m = 30.0 V. For what valuesof the capacitance would the average rate at which energy isdissipated in the resistance be (a) a maximum and (b) a minimum?What are (c) the maximum dissipation rate and the corresponding(d) phase angle and (e) power factor? What are (f) the minimum dissipation rate and the corresponding (g) phase angle and (h) power factor?arrow_forwardAn L-R-C series circuit has inductance 42.0 mH, capacitance C, and resistance R. Without the resistor, the angular frequency of oscillation is 624 rad/srad/s. With the resistor, the angular frequency is 208 rad/srad/s. Find the value of C and R. The value of R is NOT 69.888 ohms OR 8.736 ohmsarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
College Physics
Physics
ISBN:9781285737027
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning
Introduction To Alternating Current; Author: Tutorials Point (India) Ltd;https://www.youtube.com/watch?v=0m142qAZZpE;License: Standard YouTube License, CC-BY