Chapter 3, Problem 92P

Consider a 1-m wide inclined gate of negligible weight that separates water from another fluid. What would be the volume of the concrete block $\left(\text{SG}=2.4\right)$ immersed in water lo keep the gate at the position shown? Disregard any frictional effects.

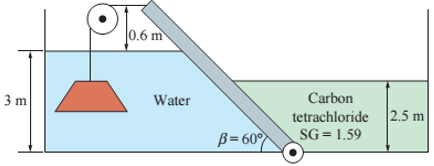
FIGURE P3−92

To determine

The volume of the concrete block.

Answer to Problem 92P

The volume of the concrete block is $0.0941{\text{m}}^3$.

Explanation of Solution

Given information:

Specific gravity of the concrete is $2.4$.

The below figure shows the free body diagram of the gate.

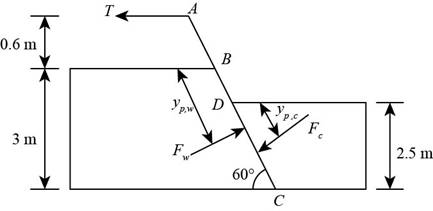
  Figure-(1)

Write the expression for the length of the portion BC.

   $l_{BC}=\frac{3}{\sin \theta }$    ...... (I)

Here, the angle from negative x axis is $\theta$.

Write the expression for the length of the height DC.

   $l_{DC}=\frac{2.5\text{m}}{\sin \theta }$    ...... (II)

Write the expression for the vertical depth of the centre of gravity of the gate in contact with water from the free surface.

   ${h^{\prime }}_w=\frac{h_w}{2}$    ...... (III)

Here, the vertical height of the gate in contact with water is $h_w$.

Write the expression for the pressure of water acting on the gate.

   $p_w={\rho }_{w}g{h^{\prime }}_w$    ...... (IV)

Here, the acceleration due to gravity is $g$ and the density of water is ${\rho }_w$.

Write the expression for the resultant force acting on the triangular gate.

   $F_w=p_w{A}_w$    ...... (V)

Here, the area is $A_w$.

Write the expression for the density of tetrachloride is ${\left(SG\right)}_c$.

Write the expression for the vertical depth of the centre of gravity of the gate in contact with the carbon tetrachloride from the free surface.

   ${h^{\prime }}_c=\frac{h_c}{2}$    ...... (VI)

Here, the height of the gate in contact with the carbon tetrachloride from the free surface is $h_c$.

Write the expression for the resultant force of carbon tetrachloride acting on the gate.

   $F_c=p_c{A}_c$    ...... (VII)

Here, the area of resisting pressure of carbon tetrachloride is $A_c$ and the pressure of carbon tetrachloride acting on the gate is $p_c$.

Write the expression for the pressure of the carbon tetrachloride acting on the gate.

   $p_c={\rho }_{c}g{h^{\prime }}_c$    ...... (VIII)

Here, the density of carbon tetrachloride is ${\rho }_c$.

Write the expression for the area of the resisting pressure of carbon tetrachloride.

   $A_c=l_{DC}w$    ...... (IX)

Here, the width of the gate is $w$.

Write the expression for the point of the application of force due to the water.

   $y_{p,w}=\frac{{h^{\prime }}_w}{\sin 60°}+\frac{l_{xx,C}}{\left(\frac{{h^{\prime }}_w}{\sin 60°}\right)A}$    ...... (X)

Here, the moment of inertia about x axis and point C is $I_{xx,C}$.

Write the expression for the moment of inertia about x axis and for portion BC.

   $l_{xx,C}=\frac{b{\left(l_{BC}\right)}^3}{12}$    ...... (XI)

Substitute $b{\left(l_{BC}\right)}^3/12$ in Equation (X).

   $y_{p,w}=\frac{{h^{\prime }}_w}{\sin 60°}+\frac{b{\left(l_{BC}\right)}^3/12}{\left(\frac{{h^{\prime }}_w}{\sin 60°}\right)b{l}_{BC}}$    ...... (XII)

Write the expression for the point of the application of force due to the carbon tetrachloride.

   $y_{p,c}=\frac{{h^{\prime }}_c}{\sin 120°}+\frac{l_{xx,C}}{\left(\frac{{h^{\prime }}_c}{\sin 120°}\right)A}$    ...... (XI)

Write the expression for the moment of inertia about x axis and for portion DC.

   $l_{xx,C}=\frac{b{\left(l_{DC}\right)}^3}{12}$    ...... (XIII)

Substitute $b{\left(l_{DC}\right)}^3/12$ in Equation (X).

   $y_{p,c}=\frac{{h^{\prime }}_c}{\sin 60°}+\frac{{\left(l_{DC}\right)}^3/12}{\left(\frac{{h^{\prime }}_c}{\sin 60°}\right)l_{DC}}$    ...... (XIV)

Write the expression for the moments about point C.

   $\begin{array}{l}Th=F_w\left(\frac{3}{\sin 60°}-y_{p,w}\right)+F_c\left(\frac{3}{\sin 60°}-y_{p,c}\right)\\ T=\frac{F_w\left(\frac{3}{\sin 60°}-y_{p,w}\right)-F_c\left(\frac{3}{\sin 60°}-y_{p,c}\right)}{h}\end{array}$    ...... (XV)

Here, the tension in the string is $T$.

Below figure shows the free body diagram of the block.

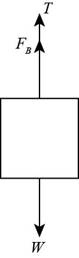
  Figure-(2)

Write the expression for the force balance in vertical direction.

   $T=W-F_B$    ...... (XVI)

Here, the weight of the concrete block is $W$ and the buoyancy force is $F_B$.

Write the expression for the weight of the block.

   $W={\rho }_{concrete}gV$    ...... (XVII)

Here the volume of the block is $V$.

Write the expression for the density of the concrete.

   ${\rho }_{concrete}={\left(SG\right)}_{concrete}{\rho }_w$    ...... (XVIII)

Here, the specific gravity of the concrete block is ${\left(SG\right)}_{concrete}$.

Substitute ${\left(SG\right)}_{concrete}{\rho }_w$ for ${\rho }_{concrete}$ in Equation (XVII).

   $W={\left(SG\right)}_{concrete}{\rho }_{w}gV$    ...... (XIX)

Write the expression for the buoyancy force.

   $F_B={\rho }_{w}gV$    ...... (XX)

Here, the displaced volume is $V$.

Write the expression for the area of the resisting pressure of water.

   $A_w=l_{BC}w$    ...... (XXI)

Write the expression for the density of the carbon tetrachloride.

   ${\rho }_c={\left(SG\right)}_c{\rho }_w$    ...... (XXII)

Here, the specific gravity of the carbon tetrachloride is ${\left(SG\right)}_c$.

Calculation:

Substitute $60°$ for $\theta$ in Equation (I).

   $\begin{array}{c}{l}_{BC}=\frac{3\text{m}}{\sin 60°}\\ =\frac{3\text{m}}{\sqrt{3}/2}\\ =2\sqrt{3}\text{m}\end{array}$

Substitute $60°$ for $\theta$ in Equation (II).

   $\begin{array}{c}{l}_{DC}=\frac{2.5\text{m}}{\sin 60°}\\ =\frac{2.5\text{m}}{\frac{\sqrt{3}}{2}}\\ =\frac{5}{\sqrt{3}}\text{m}\end{array}$

Substitute $3\text{m}$ for $h_w$ in Equation (III).

   $\begin{array}{c}{h^{\prime }}_w=\frac{3\text{m}}{2}\\ =1.5\text{m}\end{array}$

Substitute $1000\text{kg}/{\text{m}}^{\text{3}}$ for ${\rho }_w$, $9.81\text{m}/{\text{s}}^{\text{2}}$ for $g$ and $1.5\text{m}$ for ${h^{\prime }}_w$ in Equation (IV).

   $\begin{array}{c}{p}_w=1000\text{kg}/{\text{m}}^{\text{3}}\left(9.81\text{m}/{\text{s}}^{\text{2}}\right)\left(1.5\text{m}\right)\\ =9810\text{kg}/{\text{m}}^2\cdot {\text{s}}^{\text{2}}\left(1.5\text{m}\right)\\ =14715\text{kg}\cdot \text{m}/{\text{s}}^{\text{2}}\left(\frac{1}{{\text{m}}^2}\right)\\ =14715\text{N}/{\text{m}}^2\end{array}$

Substitute $2\sqrt{3}\text{m}$ for $l_{BC}$ and $1\text{m}$ for $w$ in Equation (XXI).

   $\begin{array}{c}{A}_w=\left(2\sqrt{3}\text{m}\right)\left(1\text{m}\right)\\ =2\sqrt{3}{\text{m}}^2\end{array}$

Substitute $2\sqrt{3}{\text{m}}^2$ for $A_w$ and $14715\text{N}/{\text{m}}^2$ for $p_w$ in Equation (V).

   $\begin{array}{c}{F}_w=\left(14715\text{N}/{\text{m}}^2\right)\left(2\sqrt{3}{\text{m}}^2\right)\\ =50974.2\text{N}/{\text{m}}^2\left({\text{m}}^2\right)\\ =50974.2\text{N}\end{array}$

Substitute $1.59$ for ${\left(SG\right)}_c$ and $1000\text{kg}/{\text{m}}^{\text{3}}$ for ${\rho }_w$ in Equation (XVIII).

   $\begin{array}{c}{\rho }_c=1.59\left(1000\text{kg}/{\text{m}}^{\text{3}}\right)\\ =1590\text{kg}/{\text{m}}^{\text{3}}\end{array}$

Substitute $2.5\text{m}$ for ${h^{\prime }}_c$ in Equation (VI).

   $\begin{array}{c}{h^{\prime }}_c=\frac{2.5\text{m}}{2}\\ =1.25\text{m}\end{array}$

Substitute $1590\text{kg}/{\text{m}}^{\text{3}}$ for ${\rho }_c$, $9.81\text{m}/{\text{s}}^{\text{2}}$ for $g$ and $1.25\text{m}$ for ${h^{\prime }}_c$ in Equation (VIII).

   $\begin{array}{c}{p}_c=\left(1590\text{kg}/{\text{m}}^{\text{3}}\right)\left(9.81\text{m}/{\text{s}}^{\text{2}}\right)\left(1.25\text{m}\right)\\ =15597.9\text{kg}/{\text{m}}^2\cdot {\text{s}}^{\text{2}}\left(1.25\text{m}\right)\\ =19497.37\text{kg}\cdot \text{m}/{\text{s}}^{\text{2}}\left(\frac{1}{{\text{m}}^2}\right)\\ =19497.375\text{N}/{\text{m}}^2\end{array}$

Substitute $\frac{5}{\sqrt{3}}\text{m}$ for $l_{DC}$ and $1\text{m}$ for $w$ in Equation (XIX).

   $\begin{array}{c}{A}_c=\left(\frac{5}{\sqrt{3}}\text{m}\right)\left(1\text{m}\right)\\ =\frac{5}{\sqrt{3}}{\text{m}}^2\end{array}$

Substitute $19497.375\text{N}/{\text{m}}^2$ for $p_c$ and $\frac{5}{\sqrt{3}}{\text{m}}^2$ for $A_c$ in Equation (VII).

   $\begin{array}{c}{F}_c=\left(19497.375\text{N}/{\text{m}}^2\right)\left(\frac{5}{\sqrt{3}}{\text{m}}^2\right)\\ =\frac{97486.875\text{N}}{1.732}\\ =56284.073\text{N}\end{array}$

Substitute $1.5\text{m}$ for ${h^{\prime }}_w$ and $2\sqrt{3}\text{m}$ for $l_{BC}$ in Equation (XII).

   $\begin{array}{c}{y}_{p,w}=\frac{1.5\text{m}}{\sin 60°}+\frac{{\left(2\sqrt{3}\text{m}\right)}^3/12}{\left(\frac{1.5\text{m}}{\sin 60°}\right)b\left(2\sqrt{3}\text{m}\right)}\\ =1.732\text{m}+0.577\text{m}\\ =2.309\text{m}\end{array}$

Substitute $1.25\text{m}$ for ${h^{\prime }}_c$ and $\frac{5}{\sqrt{3}}\text{m}$ for $l_{DC}$ and $2.4\sqrt{3}\text{m}$ for $l_{AC}$ in Equation (XIv).

   $\begin{array}{c}{y}_{p,c}=\frac{1.25\text{m}}{\sin 60°}+\frac{{\left(\frac{5}{\sqrt{3}}\text{m}\right)}^3/12}{\left(\frac{1.25\text{m}}{\sin 60°}\right)\left(\frac{5}{\sqrt{3}}\text{m}\right)}\\ =1.4433\text{m}+0.4806\text{m}\\ =1.924\text{m}\end{array}$

Substitute $50974.2\text{N}$ for $F_w$, $2.309\text{m}$ for $y_{p,w}$, $56284.07\text{N}$ for $F_c$, $1.924\text{m}$ for $y_{p,c}$ and $3.6\text{m}$ for $h$ in Equation (XV).

   $\begin{array}{c}T=\frac{\left[\begin{array}{l}50974.2\text{N}\left(\frac{3\text{m}}{\sin 60°}-2.309\text{m}\right)-\\ 56284.07\text{N}\left(\frac{3\text{m}}{\sin 60°}-1.924\text{m}\right)\end{array}\right]}{3.6\text{m}}\\ =\frac{\left[\begin{array}{l}50974.2\text{N}\left(1.155\text{m}\right)-\\ 56284.07\text{N}\left(1.540\text{m}\right)\end{array}\right]}{3.6\text{m}}\\ =\frac{4692.82\text{N}\cdot \text{m}}{3.6\text{m}}\\ =1303.56\text{N}\end{array}$

Substitute $2.4$ for ${\left(SG\right)}_{concrete}$, $1000\text{kg}/{\text{m}}^{\text{3}}$ for ${\rho }_w$ and $9.81\text{m}/{\text{s}}^{\text{2}}$ for $g$ in Equation (XIX).

   $\begin{array}{c}W=2.4\left(1000\text{kg}/{\text{m}}^{\text{3}}\right)\left(9.81\text{m}/{\text{s}}^{\text{2}}\right)V\\ W=\left(2400\text{kg}/{\text{m}}^{\text{3}}\right)\left(9.81\text{m}/{\text{s}}^{\text{2}}\right)V\\ W=23544\text{kg}/{\text{m}}^2\cdot {\text{s}}^{\text{2}}\left(V\right)\end{array}$    ...... (XXIII)

Substitute $1000\text{kg}/{\text{m}}^{\text{3}}$ for

   ${\rho }_w$ and $9.81\text{m}/{\text{s}}^{\text{2}}$ for $g$ in Equation (XX).

   $\begin{array}{c}{F}_B=1000\text{kg}/{\text{m}}^{\text{3}}\left(9.81\text{m}/{\text{s}}^{\text{2}}\right)V\\ F_B=9810\text{kg}/{\text{m}}^2\cdot {\text{s}}^{\text{2}}\left(V\right)\end{array}$    ...... (XXIV)

Substitute $9810\text{kg}/{\text{m}}^2\cdot {\text{s}}^{\text{2}}\left(V\right)$ for $F_B$, $23544\text{kg}/{\text{m}}^2\cdot {\text{s}}^{\text{2}}\left(V\right)$ for $W$ and $1303.56\text{N}$ for $T$ in Equation (XVI).

   $\begin{array}{l}1303.56\text{N}=23544\text{kg}/{\text{m}}^2\cdot {\text{s}}^{\text{2}}\left(V\right)-9810\text{kg}/{\text{m}}^2\cdot {\text{s}}^{\text{2}}\left(V\right)\\ 1303.56\text{N}=\left(23544\text{kg}/{\text{m}}^2\cdot {\text{s}}^{\text{2}}-9810\text{kg}/{\text{m}}^2\cdot {\text{s}}^{\text{2}}\right)\left(V\right)\\ V=\frac{1303.56\text{N}}{13734\text{kg}\cdot \text{m}/{\text{s}}^{\text{2}}\left(\frac{1}{{\text{m}}^3}\right)}\\ V=\frac{1303.56\text{N}\left(\frac{1\text{kg}\cdot \text{m}/{\text{s}}^{\text{2}}}{1\text{N}}\right)}{13734\text{kg}\cdot \text{m}/{\text{s}}^{\text{2}}\left(\frac{1}{{\text{m}}^3}\right)}\end{array}$

   $V=0.09491{\text{m}}^3$

Conclusion:

The volume of the concrete block is $0.0941{\text{m}}^3$.