INTRODUCTORY STATISTICS (LOOSELEAF)
3rd Edition
ISBN: 9780135163146
Author: Gould
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 3, Problem 43SE
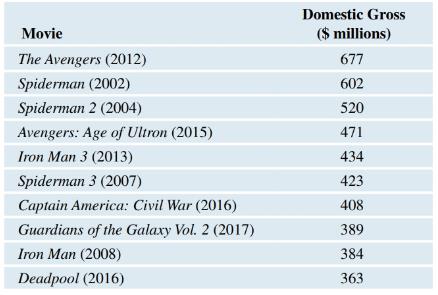
Marvel Movies (Example 11 and 13) The top ten movies based on Marvel comic book characters for the U.S. box office as of fall 2017 are shown in the following table, with domestic gross rounded to the nearest hundred million. (Source: ultimatemovieranking.com)
a. Sort the domestic gross income from smallest to largest. Find the
b. Using the sorted data, find Q1 and Q3. Then find the
c. Find the range of the data. Explain why the IQR is preferred over the range as a measure of variability.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
6. Show that
1{AU B} = max{1{A}, I{B}} = I{A} + I{B} - I{A} I{B};
I{AB} = min{I{A}, I{B}} = I{A} I{B};
I{A A B} = I{A} + I{B}-21{A} I {B} = (I{A} - I{B})².
Theorem 3.5 Suppose that P and Q are probability measures defined on the same
probability space (2, F), and that F is generated by a л-system A. If P(A) = Q(A)
for all A = A, then P = Q, i.e., P(A) = Q(A) for all A = F.
6. Show that, for any random variable, X, and a > 0,
Lo P(x
-00
P(x < x
Chapter 3 Solutions
INTRODUCTORY STATISTICS (LOOSELEAF)
Ch. 3 - Earnings A sociologist says, “Typically, men in...Ch. 3 - Houses A real estate agent claims that all things...Ch. 3 - Exercise Hours (Example 1) The histogram shows the...Ch. 3 - Sodium The following histogram shows the sodium...Ch. 3 - Tall Buildings The table shows the location and...Ch. 3 - Roller Coasters The table shows the names and...Ch. 3 - River Lengths (Example 2) The table shows the...Ch. 3 - Children of First Ladies This list represents the...Ch. 3 - 200-Meter Run The table show the gold medal...Ch. 3 - Olympic Swimming Times The table shows the...
Ch. 3 - Wedding Costs by Gender (Example 3) StatCrunch did...Ch. 3 - Wedding Costs by Experience StatCrunch did a...Ch. 3 - Surfing College students and surfers Rex Robinson...Ch. 3 - State College Tuition The tuition costs (in...Ch. 3 - Winter Temperatures San Jose and Denver (Example...Ch. 3 - Summer Temperatures L.A. and NYC The histograms...Ch. 3 - Weight Gain during Pregnancy (Example 5) The mean...Ch. 3 - Birth Length The mean birth length for U.S....Ch. 3 - Orange Juice Prices (Example 6) From Amazon.com,...Ch. 3 - Sibling Ages Four siblings are 2, 6, 9, and 10...Ch. 3 - Olympics In the most recent summer Olympics, do...Ch. 3 - Weights Suppose you have a data set with the...Ch. 3 - Home Prices (SC and TN) The prices (in $ thousand)...Ch. 3 - Home Prices (FL and GA) The prices (in $ thousand)...Ch. 3 - Drinkers The number of alcoholic drinks per week...Ch. 3 - Prob. 26SECh. 3 - Standard Deviation Is it possible for a standard...Ch. 3 - Standard Deviation Is it possible for a standard...Ch. 3 - Major League Baseball Runs (Example 7) The...Ch. 3 - Residential Energy Consumption (East) Data on...Ch. 3 - Pollution Index (Example 8) In 2017 a pollution...Ch. 3 - Pollution Index In 2017 a pollution index was...Ch. 3 - Heights and z-Scores The dotplot shows heights of...Ch. 3 - Heights Refer to the dotplot in the previous...Ch. 3 - Unusual IQs (Example 9) Wechsler IQ tests have a...Ch. 3 - Lengths of Pregnancy Distributions of gestation...Ch. 3 - Low-Birth-Weight Babies (Example 10) Babies born...Ch. 3 - Birth Lengths Babies born after 40 weeks gestation...Ch. 3 - Men’s Heights Assume that men’s heights have a...Ch. 3 - Women’s Heights Assume that women’s heights have a...Ch. 3 - Name two measures of the center of a distribution,...Ch. 3 - Name two measures of the variation of a...Ch. 3 - Marvel Movies (Example 11 and 13) The top ten...Ch. 3 - DC Movies The top seven movies based on DC comic...Ch. 3 - Top Seven Marvel Movies (Example 12) Use the data...Ch. 3 - Top Five DC Movies Use the data in Exercise 3.44,...Ch. 3 - Total Energy Consumption Data was collected on the...Ch. 3 - Industrial Energy Consumption Data was collected...Ch. 3 - Outliers a. In your own words, describe to someone...Ch. 3 - Center and Variation When you are comparing two...Ch. 3 - An Error A dieter recorded the number of calories...Ch. 3 - Baseball Strike In 1994, Major League Baseball...Ch. 3 - Home Prices Home prices in San Luis Obispo County...Ch. 3 - Youth Heights The National Longitudinal Survey...Ch. 3 - Senator Years in Office (Example 14) The following...Ch. 3 - MLB Player Ages The following histograms show the...Ch. 3 - Prob. 57SECh. 3 - Death Row: West The following table shows the...Ch. 3 - The Consumer Price Index (CPI) (Example 16)...Ch. 3 - Prob. 60SECh. 3 - Prob. 61SECh. 3 - Mean from a Histogram The histogram shows the...Ch. 3 - Poverty Rates The following boxplot shows the...Ch. 3 - Regional Population Density The figure shows the...Ch. 3 - Professional Sport Ticket Prices The following...Ch. 3 - BA Attainment The following boxplot shows the...Ch. 3 - Matching Boxplots and Histograms a. Report the...Ch. 3 - Matching Boxplots and Histograms Match each of the...Ch. 3 - Public Libraries Data at this text’s website show...Ch. 3 - Gas Taxes Data at this text’s website show the gas...Ch. 3 - Roller Coaster Heights (Example 17) The dotplot...Ch. 3 - Passing the Bar Exam The following dotplot shows...Ch. 3 - Exam Scores The five-number summary for a...Ch. 3 - Exam Scores The five-number summary for a...Ch. 3 - Prob. 75CRECh. 3 - Gas Taxes (West) The following table shows the gas...Ch. 3 - Final Exam Grades The data that follow are final...Ch. 3 - Speeding Tickets College students Diane Glover and...Ch. 3 - Heights The following graph shows the heights for...Ch. 3 - Marathon Times The following histogram of marathon...Ch. 3 - Prob. 81CRECh. 3 - Chain Restaurant Calories The New York Times...Ch. 3 - 12th-Grade Sleep The histogram shows hours of...Ch. 3 - For exercises 3.85 through 3.88, construct two...Ch. 3 - For exercises 3.85 through 3.88, construct two...Ch. 3 - For exercises 3.85 through 3.88, construct two...Ch. 3 - For exercises 3.85 through 3.88, construct two...Ch. 3 - Educational Attainment The tables below show the...Ch. 3 - Unemployment Rates The tables below show the 2015...Ch. 3 - Professional Sports Ticket Prices The following...Ch. 3 - Professional Sports Ticket Prices The following...Ch. 3 - Exam Scores An exam score has a mean of 80 and a...Ch. 3 - Boys’ Heights Three-year-old boys in the United...Ch. 3 - SAT and ACT Scores Quantitative SAT scores have a...Ch. 3 - Children’s Heights Mrs. Diaz has two children: a...Ch. 3 - Prob. 97CRECh. 3 - Students’ Ages Here are the ages of some students...Ch. 3 - Race Times The following graph shows the time it...Ch. 3 - Prob. 100CRECh. 3 - Prob. 101CRECh. 3 - Prob. 102CRE
Additional Math Textbook Solutions
Find more solutions based on key concepts
1. How much money is Joe earning when he’s 30?
Pathways To Math Literacy (looseleaf)
For Problems 23-28, write in simpler form, as in Example 4. logbFG
Finite Mathematics for Business, Economics, Life Sciences and Social Sciences
For Exercises 13–18, write the negation of the statement.
13. The cell phone is out of juice.
Math in Our World
1. How is a sample related to a population?
Elementary Statistics: Picturing the World (7th Edition)
Empirical versus Theoretical A Monopoly player claims that the probability of getting a 4 when rolling a six-si...
Introductory Statistics
Find all solutions of each equation in the interval .
Precalculus: A Unit Circle Approach (3rd Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.Similar questions
- 5. Suppose that X is an integer valued random variable, and let mЄ N. Show that 8 11118 P(narrow_forward食食假 6. Show that I(AUB) = max{1{A}, I{B}} = I{A} + I{B} - I{A} I{B}; I(AB)= min{I{A}, I{B}} = I{A} I{B}; I{A A B} = I{A} + I{B}-21{A} I{B} = (I{A} - I{B})². -arrow_forward11. Suppose that the events (An, n ≥ 1) are independent. Show that the inclusion- exclusion formula reduces to P(UAL)-1-(1-P(Ak)). k=1 k=1arrow_forward12. (a) Explain tail events and the tail o-field. Give an example.arrow_forwardLet A, A1, A2,... be measurable sets. Then P(A)=1- P(A); • P(Ø) = 0; P(A1 UA2) ≤ P(A1) + P(A2); A1 C A2 P(A1) P(A2); P(UA) + P(n=14) = 1. Exercise 3.1 Prove these relations. ☐arrow_forward17. Suppose that X1, X2,..., Xn are random variables, such that E|xk| < ∞ for all k, and set Yn = max1arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_iosRecommended textbooks for you
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw HillBig Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin HarcourtHolt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
The Shape of Data: Distributions: Crash Course Statistics #7; Author: CrashCourse;https://www.youtube.com/watch?v=bPFNxD3Yg6U;License: Standard YouTube License, CC-BY
Shape, Center, and Spread - Module 20.2 (Part 1); Author: Mrmathblog;https://www.youtube.com/watch?v=COaid7O_Gag;License: Standard YouTube License, CC-BY
Shape, Center and Spread; Author: Emily Murdock;https://www.youtube.com/watch?v=_YyW0DSCzpM;License: Standard Youtube License