Calculus Volume 1
17th Edition
ISBN: 9781938168024
Author: Strang, Gilbert
Publisher: OpenStax College
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 2.3, Problem 122E
In the following exercises, use the following graphs and the limits laws to evaluate each limit.
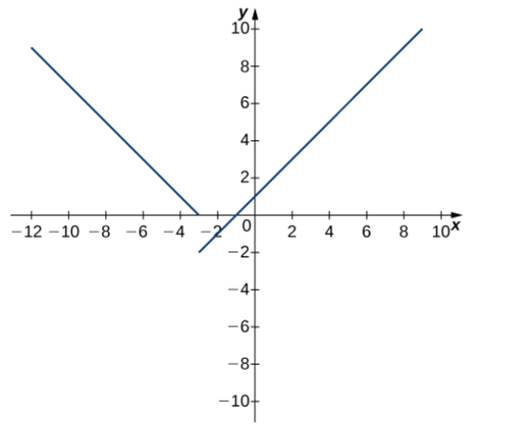
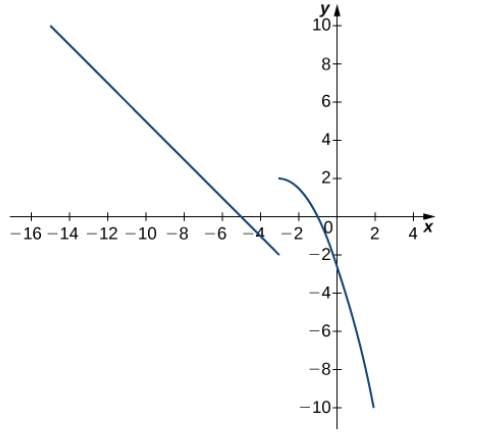
122.
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
Test the null hypothesis that the slope is zero (e.g., β=0).
Do College Students With Part-Time Jobs Sleep Less?
College students were surveyed about the number of hours they sleep each night.Group A = With part-time jobs | Group B = Without jobs
Group A: 6, 5, 7, 6, 5Group B: 8, 7, 9, 8, 7
Instructions:
State your hypothesis and perform a two-sample t-test with all formulas.
Create histograms for each group. Label axes and add titles.
Comment on the distribution shape (e.g., normal, skewed, etc.).Solve on pen and paper
H0: mean egg weight is the same in all three diets
HA: there is at least one difference among the means
Chapter 2 Solutions
Calculus Volume 1
Ch. 2.1 - For the following exercises, points P(l, 2) and...Ch. 2.1 - For the following exercises, points P(l, 2) and...Ch. 2.1 - For the following exercises, points P(l, 2) and...Ch. 2.1 - For the following exercises, points P(l, 1) and...Ch. 2.1 - For the following exercises, points P(l, 1) and...Ch. 2.1 - For the following exercises, points P(l, 1) and...Ch. 2.1 - For the following exercises, points P(4, 2) and...Ch. 2.1 - For the following exercises, points P(4, 2) and...Ch. 2.1 - For the following exercises, points P(4, 2) and...Ch. 2.1 - For the following exercises, points P(l.5, 0) and...
Ch. 2.1 - For the following exercises, points P( 1.5, 0) and...Ch. 2.1 - For the following exercises, points P( 1.5, 0) and...Ch. 2.1 - For the following exercises, points P(-1, -1) and...Ch. 2.1 - For the following exercises, points P(-1,-1) and...Ch. 2.1 - For the following exercises, points P(-1, - 1) and...Ch. 2.1 - For the following exercises, the position function...Ch. 2.1 - For the following exercises, the position function...Ch. 2.1 - For the following exercises, consider a stone...Ch. 2.1 - For the following exercises, consider a stone...Ch. 2.1 - For the following exercises, consider a rocket...Ch. 2.1 - For the following exercises, consider a rocket...Ch. 2.1 - For the following exercises, consider an athlete...Ch. 2.1 - For the following exercises, consider an athlete...Ch. 2.1 - For the following exercises, consider the...Ch. 2.1 - For the following exercises, consider the function...Ch. 2.1 - For the following exercises, consider the function...Ch. 2.1 - For the following exercises, consider the...Ch. 2.1 - For the following exercises, consider the function...Ch. 2.1 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - In the following exercises, consider the graph of...Ch. 2.2 - In the following exercises, consider the graph of...Ch. 2.2 - In the following exercises, consider the graph of...Ch. 2.2 - In the following exercises, consider the graph of...Ch. 2.2 - In the following exercises, use the following...Ch. 2.2 - In the following exercises, use the following...Ch. 2.2 - In the following exercises, use the following...Ch. 2.2 - In the following exercises, use the following...Ch. 2.2 - In the following exercises, use the following...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, sketch the graph of a...Ch. 2.2 - In the following exercises, sketch the graph of a...Ch. 2.2 - In the following exercises, sketch the graph of a...Ch. 2.2 - In the following exercises, sketch the graph of a...Ch. 2.2 - In the following exercises, sketch the graph of a...Ch. 2.2 - Shock waves arise in many physical applications,...Ch. 2.2 - A track coach uses a camera with a fast shutter to...Ch. 2.3 - Some of the geometric formulas we take for granted...Ch. 2.3 - Some of the geometric formulas we take for granted...Ch. 2.3 - Some of the geometric formulas we take for granted...Ch. 2.3 - Some of the geometric formulas we take for granted...Ch. 2.3 - Some of the geometric formulas we take for granted...Ch. 2.3 - In the following exercises, use the limit Laws to...Ch. 2.3 - In the following exercises, use the limit laws to...Ch. 2.3 - In the following exercises, use the limit laws to...Ch. 2.3 - In the following exercises, use the limit laws to...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - ]In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - [T] In the following exercises, use a calculator...Ch. 2.3 - [T] In the following exercises, use a calculator...Ch. 2.3 - [T] In the following exercises, use a calculator...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - yIn the following exercises, use the following...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - For the following problems, evaluate the limit...Ch. 2.3 - For the following problems, evaluate the limit...Ch. 2.3 - For the following problems, evaluate the limit...Ch. 2.3 - [T] In physics, the magnitude of an electric field...Ch. 2.3 - [T] The density of an object is given by its mass...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, decide if the...Ch. 2.4 - For the following exercises, decide if the...Ch. 2.4 - For the following exercises, decide if the...Ch. 2.4 - For the following exercises, decide if the...Ch. 2.4 - For the following exercises, decide if the...Ch. 2.4 - For the following exercises, decide if the...Ch. 2.4 - In the following exercises, find the value(s) of k...Ch. 2.4 - In the following exercises, find the value(s) of k...Ch. 2.4 - In the following exercises, find the value(s) of k...Ch. 2.4 - In the following exercises, find the value(s) of k...Ch. 2.4 - In the following exercises, find the value(s) of k...Ch. 2.4 - In the following exercises, use the Intermediate...Ch. 2.4 - In the following exercises, use the Intermediate...Ch. 2.4 - In the following exercises, use the Intermediate...Ch. 2.4 - In the following exercises, use the Intermediate...Ch. 2.4 - Consider the graph of the function y=f(x) shown in...Ch. 2.4 - Let f(x)={3x,x1x3,x1 . Sketch the graph of f. Is...Ch. 2.4 - Let f(x)=x41x21forx1,1 . a. Sketch the graph of f....Ch. 2.4 - Sketch the graph of the function y=f(x) with...Ch. 2.4 - Sketch the graph of the function y=f(x) with...Ch. 2.4 - In the following exercises, suppose y=f(x) is...Ch. 2.4 - In the following exercises, suppose y=f(x) is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - [T] The following problems consider the scalar...Ch. 2.4 - [T] The following problems consider the scalar...Ch. 2.4 - [T] The following problems consider the scalar...Ch. 2.4 - [T] After a certain distance D has passed, the...Ch. 2.4 - As the rocket travels away from Earth’s surface,...Ch. 2.4 - wqProve the following functions are continuous...Ch. 2.4 - Prove the following functions are continuous...Ch. 2.4 - Prove the following functions are continuous...Ch. 2.5 - In the following exercises, write the appropriate ...Ch. 2.5 - In the following exercises, write the appropriate ...Ch. 2.5 - In the following exercises, write the appropriate ...Ch. 2.5 - In the following exercises, write the appropriate ...Ch. 2.5 - The following graph of the function f satisfies...Ch. 2.5 - The following graph of the function f satisfies...Ch. 2.5 - The following graph of the function f satisfies...Ch. 2.5 - The following graph of the function f satisfies...Ch. 2.5 - The following graph of the function f satisfies...Ch. 2.5 - The following graph of the function f satisfies...Ch. 2.5 - [T] In the following exercises, use a graphing...Ch. 2.5 - [T] In the following exercises, use a graphing...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - An engineer is using a machine to cut a flat...Ch. 2.5 - Use the precise definition of limit to prove that...Ch. 2.5 - Using precise definitions of limits, prove that...Ch. 2.5 - Using precise definitions of limits, prove that...Ch. 2.5 - Using precise definitions of limits, prove that...Ch. 2.5 - Using the function from the previous exercise, use...Ch. 2.5 - limxa(f(x)g(x))=LMCh. 2.5 - limxa[cf(x)]=cL for any real constant c (Hint....Ch. 2.5 - ...Ch. 2 - wTrue or False. In the following exercises,...Ch. 2 - True or False. In the following exercises, justify...Ch. 2 - True or False. In the following exercises, justify...Ch. 2 - True or False. In the following exercises, justify...Ch. 2 - Using the graph, find each limit or explain why...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - wIn the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, use the squeeze...Ch. 2 - In the following exercises, use the squeeze...Ch. 2 - In the following exercises, use the squeeze...Ch. 2 - In the following exercises, determine the value of...Ch. 2 - In the following exercises, determine the value of...Ch. 2 - In the following exercises, use the precise...Ch. 2 - In the following exercises, use the precise...Ch. 2 - A ball is thrown into the air and the vertical...Ch. 2 - A particle moving along a line has a displacement...Ch. 2 - From the previous exercises, estimate the...
Additional Math Textbook Solutions
Find more solutions based on key concepts
Two fair dice are rolled. What is the conditional probability that at least one lands on 6 given that the dice ...
A First Course in Probability (10th Edition)
Exercises 1–6 give the positions s = f(t) of a body moving on a coordinate line, with s in meters and t in seco...
University Calculus: Early Transcendentals (4th Edition)
SAT Test. In Exercises 15–20, assume that random guesses are made for eight multiple choice questions on an SAT...
Elementary Statistics (13th Edition)
Show that 34=12 using each of the following models. a. Repeated-addition number line b. Rectangular array c. Ar...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Trigonometric substitutions Evaluate the following integrals. 17. 64x2dx
Calculus: Early Transcendentals (2nd Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- This is advanced mathematics question that need detailed solutionsarrow_forwardQuestion: Let F be a field. Prove that F contains a unique smallest subfield, called the prime subfield, which is isomorphic to either Q or Zp for some prime p. Instructions: • Begin by identifying the identity element 1 € F. • Use the closure under addition and inverses to build a subring. • • • Show that either the map ZF or Q →F is an embedding. Prove minimality and uniqueness. Discuss the characteristic of a field and link it to the structure of the prime subfield.arrow_forwardTopic: Group Theory | Abstract Algebra Question: Let G be a finite group of order 45. Prove that G has a normal subgroup of order 5 or order 9, and describe the number of Sylow subgroups for each. Instructions: • Use Sylow's Theorems (existence, conjugacy, and counting). • List divisors of 45 and compute possibilities for n for p = 3 and p = 5. Show that if n = 1, the subgroup is normal. Conclude about group structure using your analysis.arrow_forward
- Topic: Group Theory | Abstract Algebra Question: Let G be a finite group of order 45. Prove that G has a normal subgroup of order 5 or order 9, and describe the number of Sylow subgroups for each. Instructions: • Use Sylow's Theorems (existence, conjugacy, and counting). • List divisors of 45 and compute possibilities for n for p = 3 and p = 5. Show that if n = 1, the subgroup is normal. Conclude about group structure using your analysis.arrow_forwardTopic: Group Theory | Abstract Algebra Question: Let G be a finite group of order 45. Prove that G has a normal subgroup of order 5 or order 9, and describe the number of Sylow subgroups for each. Instructions: • Use Sylow's Theorems (existence, conjugacy, and counting). • List divisors of 45 and compute possibilities for n for p = 3 and p = 5. Show that if n = 1, the subgroup is normal. Conclude about group structure using your analysis.arrow_forwardComplete solution requiredarrow_forward
- Topic: Group Theory | Abstract Algebra Question: Let G be a finite group of order 45. Prove that G has a normal subgroup of order 5 or order 9, and describe the number of Sylow subgroups for each. Instructions: • Use Sylow's Theorems (existence, conjugacy, and counting). • List divisors of 45 and compute possibilities for n for p = 3 and p = 5. Show that if n = 1, the subgroup is normal. Conclude about group structure using your analysis.arrow_forwardTopic: Group Theory | Abstract Algebra Question: Let G be a finite group of order 45. Prove that G has a normal subgroup of order 5 or order 9, and describe the number of Sylow subgroups for each. Instructions: • Use Sylow's Theorems (existence, conjugacy, and counting). • List divisors of 45 and compute possibilities for n for p = 3 and p = 5. Show that if n = 1, the subgroup is normal. Conclude about group structure using your analysis.arrow_forwardDo with graph of the regionarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Discrete Mathematics and Its Applications ( 8th I...MathISBN:9781259676512Author:Kenneth H RosenPublisher:McGraw-Hill Education
Mathematics for Elementary Teachers with Activiti...MathISBN:9780134392790Author:Beckmann, SybillaPublisher:PEARSON
Thinking Mathematically (7th Edition)MathISBN:9780134683713Author:Robert F. BlitzerPublisher:PEARSON
Discrete Mathematics With ApplicationsMathISBN:9781337694193Author:EPP, Susanna S.Publisher:Cengage Learning,
Pathways To Math Literacy (looseleaf)MathISBN:9781259985607Author:David Sobecki Professor, Brian A. MercerPublisher:McGraw-Hill Education
Discrete Mathematics and Its Applications ( 8th I...
Math
ISBN:9781259676512
Author:Kenneth H Rosen
Publisher:McGraw-Hill Education
Mathematics for Elementary Teachers with Activiti...
Math
ISBN:9780134392790
Author:Beckmann, Sybilla
Publisher:PEARSON
Thinking Mathematically (7th Edition)
Math
ISBN:9780134683713
Author:Robert F. Blitzer
Publisher:PEARSON
Discrete Mathematics With Applications
Math
ISBN:9781337694193
Author:EPP, Susanna S.
Publisher:Cengage Learning,
Pathways To Math Literacy (looseleaf)
Math
ISBN:9781259985607
Author:David Sobecki Professor, Brian A. Mercer
Publisher:McGraw-Hill Education
Limits and Continuity; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=9brk313DjV8;License: Standard YouTube License, CC-BY