Calculus Volume 1
17th Edition
ISBN: 9781938168024
Author: Strang, Gilbert
Publisher: OpenStax College
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 2, Problem 229RE
In the following exercises, use the precise definition of limit to prove the limit.
229. 
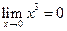
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Explain the key points and reasons for the establishment of 12.3.2(integral Test)
Use identity (1+x+x2+...+xn)*(1-x)=1-xn+1 to derive the result of 12.2.2. Please notice that identity doesn't work when x=1.
Explain the key points and reasons for the establishment of 11.3.2(integral Test)
Chapter 2 Solutions
Calculus Volume 1
Ch. 2.1 - For the following exercises, points P(l, 2) and...Ch. 2.1 - For the following exercises, points P(l, 2) and...Ch. 2.1 - For the following exercises, points P(l, 2) and...Ch. 2.1 - For the following exercises, points P(l, 1) and...Ch. 2.1 - For the following exercises, points P(l, 1) and...Ch. 2.1 - For the following exercises, points P(l, 1) and...Ch. 2.1 - For the following exercises, points P(4, 2) and...Ch. 2.1 - For the following exercises, points P(4, 2) and...Ch. 2.1 - For the following exercises, points P(4, 2) and...Ch. 2.1 - For the following exercises, points P(l.5, 0) and...
Ch. 2.1 - For the following exercises, points P( 1.5, 0) and...Ch. 2.1 - For the following exercises, points P( 1.5, 0) and...Ch. 2.1 - For the following exercises, points P(-1, -1) and...Ch. 2.1 - For the following exercises, points P(-1,-1) and...Ch. 2.1 - For the following exercises, points P(-1, - 1) and...Ch. 2.1 - For the following exercises, the position function...Ch. 2.1 - For the following exercises, the position function...Ch. 2.1 - For the following exercises, consider a stone...Ch. 2.1 - For the following exercises, consider a stone...Ch. 2.1 - For the following exercises, consider a rocket...Ch. 2.1 - For the following exercises, consider a rocket...Ch. 2.1 - For the following exercises, consider an athlete...Ch. 2.1 - For the following exercises, consider an athlete...Ch. 2.1 - For the following exercises, consider the...Ch. 2.1 - For the following exercises, consider the function...Ch. 2.1 - For the following exercises, consider the function...Ch. 2.1 - For the following exercises, consider the...Ch. 2.1 - For the following exercises, consider the function...Ch. 2.1 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - For the following exercises, consider the function...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - [T] In the following exercises, set up a table of...Ch. 2.2 - In the following exercises, consider the graph of...Ch. 2.2 - In the following exercises, consider the graph of...Ch. 2.2 - In the following exercises, consider the graph of...Ch. 2.2 - In the following exercises, consider the graph of...Ch. 2.2 - In the following exercises, use the following...Ch. 2.2 - In the following exercises, use the following...Ch. 2.2 - In the following exercises, use the following...Ch. 2.2 - In the following exercises, use the following...Ch. 2.2 - In the following exercises, use the following...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, use the graph of the...Ch. 2.2 - In the following exercises, sketch the graph of a...Ch. 2.2 - In the following exercises, sketch the graph of a...Ch. 2.2 - In the following exercises, sketch the graph of a...Ch. 2.2 - In the following exercises, sketch the graph of a...Ch. 2.2 - In the following exercises, sketch the graph of a...Ch. 2.2 - Shock waves arise in many physical applications,...Ch. 2.2 - A track coach uses a camera with a fast shutter to...Ch. 2.3 - Some of the geometric formulas we take for granted...Ch. 2.3 - Some of the geometric formulas we take for granted...Ch. 2.3 - Some of the geometric formulas we take for granted...Ch. 2.3 - Some of the geometric formulas we take for granted...Ch. 2.3 - Some of the geometric formulas we take for granted...Ch. 2.3 - In the following exercises, use the limit Laws to...Ch. 2.3 - In the following exercises, use the limit laws to...Ch. 2.3 - In the following exercises, use the limit laws to...Ch. 2.3 - In the following exercises, use the limit laws to...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - ]In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, use direct...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - In the following exercises, assume that...Ch. 2.3 - [T] In the following exercises, use a calculator...Ch. 2.3 - [T] In the following exercises, use a calculator...Ch. 2.3 - [T] In the following exercises, use a calculator...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - yIn the following exercises, use the following...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - In the following exercises, use the following...Ch. 2.3 - For the following problems, evaluate the limit...Ch. 2.3 - For the following problems, evaluate the limit...Ch. 2.3 - For the following problems, evaluate the limit...Ch. 2.3 - [T] In physics, the magnitude of an electric field...Ch. 2.3 - [T] The density of an object is given by its mass...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, determine the...Ch. 2.4 - For the following exercises, decide if the...Ch. 2.4 - For the following exercises, decide if the...Ch. 2.4 - For the following exercises, decide if the...Ch. 2.4 - For the following exercises, decide if the...Ch. 2.4 - For the following exercises, decide if the...Ch. 2.4 - For the following exercises, decide if the...Ch. 2.4 - In the following exercises, find the value(s) of k...Ch. 2.4 - In the following exercises, find the value(s) of k...Ch. 2.4 - In the following exercises, find the value(s) of k...Ch. 2.4 - In the following exercises, find the value(s) of k...Ch. 2.4 - In the following exercises, find the value(s) of k...Ch. 2.4 - In the following exercises, use the Intermediate...Ch. 2.4 - In the following exercises, use the Intermediate...Ch. 2.4 - In the following exercises, use the Intermediate...Ch. 2.4 - In the following exercises, use the Intermediate...Ch. 2.4 - Consider the graph of the function y=f(x) shown in...Ch. 2.4 - Let f(x)={3x,x1x3,x1 . Sketch the graph of f. Is...Ch. 2.4 - Let f(x)=x41x21forx1,1 . a. Sketch the graph of f....Ch. 2.4 - Sketch the graph of the function y=f(x) with...Ch. 2.4 - Sketch the graph of the function y=f(x) with...Ch. 2.4 - In the following exercises, suppose y=f(x) is...Ch. 2.4 - In the following exercises, suppose y=f(x) is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - Determine whether each of the given statements is...Ch. 2.4 - [T] The following problems consider the scalar...Ch. 2.4 - [T] The following problems consider the scalar...Ch. 2.4 - [T] The following problems consider the scalar...Ch. 2.4 - [T] After a certain distance D has passed, the...Ch. 2.4 - As the rocket travels away from Earth’s surface,...Ch. 2.4 - wqProve the following functions are continuous...Ch. 2.4 - Prove the following functions are continuous...Ch. 2.4 - Prove the following functions are continuous...Ch. 2.5 - In the following exercises, write the appropriate ...Ch. 2.5 - In the following exercises, write the appropriate ...Ch. 2.5 - In the following exercises, write the appropriate ...Ch. 2.5 - In the following exercises, write the appropriate ...Ch. 2.5 - The following graph of the function f satisfies...Ch. 2.5 - The following graph of the function f satisfies...Ch. 2.5 - The following graph of the function f satisfies...Ch. 2.5 - The following graph of the function f satisfies...Ch. 2.5 - The following graph of the function f satisfies...Ch. 2.5 - The following graph of the function f satisfies...Ch. 2.5 - [T] In the following exercises, use a graphing...Ch. 2.5 - [T] In the following exercises, use a graphing...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - In the following exercises, use the precise...Ch. 2.5 - An engineer is using a machine to cut a flat...Ch. 2.5 - Use the precise definition of limit to prove that...Ch. 2.5 - Using precise definitions of limits, prove that...Ch. 2.5 - Using precise definitions of limits, prove that...Ch. 2.5 - Using precise definitions of limits, prove that...Ch. 2.5 - Using the function from the previous exercise, use...Ch. 2.5 - limxa(f(x)g(x))=LMCh. 2.5 - limxa[cf(x)]=cL for any real constant c (Hint....Ch. 2.5 - ...Ch. 2 - wTrue or False. In the following exercises,...Ch. 2 - True or False. In the following exercises, justify...Ch. 2 - True or False. In the following exercises, justify...Ch. 2 - True or False. In the following exercises, justify...Ch. 2 - Using the graph, find each limit or explain why...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - wIn the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, evaluate the limit...Ch. 2 - In the following exercises, use the squeeze...Ch. 2 - In the following exercises, use the squeeze...Ch. 2 - In the following exercises, use the squeeze...Ch. 2 - In the following exercises, determine the value of...Ch. 2 - In the following exercises, determine the value of...Ch. 2 - In the following exercises, use the precise...Ch. 2 - In the following exercises, use the precise...Ch. 2 - A ball is thrown into the air and the vertical...Ch. 2 - A particle moving along a line has a displacement...Ch. 2 - From the previous exercises, estimate the...
Additional Math Textbook Solutions
Find more solutions based on key concepts
Version 2 of the Chain Rule Use Version 2 of the Chain Rule to calculate the derivatives of the following funct...
Calculus: Early Transcendentals (2nd Edition)
CHECK POINT 1 Write a word description of the set L = {a, b, c, d, e, f}.
Thinking Mathematically (6th Edition)
Assessment 71A Write each of the following as a sum in expanded place value form. a. 0.023 b. 206.06 c. 312.010...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
TRY IT YOURSELF 1
Find the mean of the points scored by the 51 winning teams listed on page 39.
Elementary Statistics: Picturing the World (7th Edition)
Evaluate the integrals in Exercises 1–34.
13.
University Calculus: Early Transcendentals (4th Edition)
Finding Critical Values. In Exercises 5–8, find the critical value z?/2 that corresponds to the given confidenc...
Elementary Statistics (13th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- To explain how to view "Infinite Series" from "Infinite Sequence"’s perspective, refer to 12.2.1arrow_forwardExplain the key points and reasons for the establishment of 12.2.5 and 12.2.6arrow_forwardPage < 1 of 2 - ZOOM + 1) a) Find a matrix P such that PT AP orthogonally diagonalizes the following matrix A. = [{² 1] A = b) Verify that PT AP gives the correct diagonal form. 2 01 -2 3 2) Given the following matrices A = -1 0 1] an and B = 0 1 -3 2 find the following matrices: a) (AB) b) (BA)T 3) Find the inverse of the following matrix A using Gauss-Jordan elimination or adjoint of the matrix and check the correctness of your answer (Hint: AA¯¹ = I). [1 1 1 A = 3 5 4 L3 6 5 4) Solve the following system of linear equations using any one of Cramer's Rule, Gaussian Elimination, Gauss-Jordan Elimination or Inverse Matrix methods and check the correctness of your answer. 4x-y-z=1 2x + 2y + 3z = 10 5x-2y-2z = -1 5) a) Describe the zero vector and the additive inverse of a vector in the vector space, M3,3. b) Determine if the following set S is a subspace of M3,3 with the standard operations. Show all appropriate supporting work.arrow_forward
- 13) Let U = {j, k, l, m, n, o, p} be the universal set. Let V = {m, o,p), W = {l,o, k}, and X = {j,k). List the elements of the following sets and the cardinal number of each set. a) W° and n(W) b) (VUW) and n((V U W)') c) VUWUX and n(V U W UX) d) vnWnX and n(V WnX)arrow_forward9) Use the Venn Diagram given below to determine the number elements in each of the following sets. a) n(A). b) n(A° UBC). U B oh a k gy ท W z r e t ་ Carrow_forward10) Find n(K) given that n(T) = 7,n(KT) = 5,n(KUT) = 13.arrow_forward
- 7) Use the Venn Diagram below to determine the sets A, B, and U. A = B = U = Blue Orange white Yellow Black Pink Purple green Grey brown Uarrow_forward8. For x>_1, the continuous function g is decreasing and positive. A portion of the graph of g is shown above. For n>_1, the nth term of the series summation from n=1 to infinity a_n is defined by a_n=g(n). If intergral 1 to infinity g(x)dx converges to 8, which of the following could be true? A) summation n=1 to infinity a_n = 6. B) summation n=1 to infinity a_n =8. C) summation n=1 to infinity a_n = 10. D) summation n=1 to infinity a_n diverges.arrow_forward8) Use the Venn diagram provided to shade An Bº. A B U Darrow_forward
- 5) Describe the difference between disjoint sets and overlapping sets.arrow_forward12) Suppose U = {a,b,c,d,e) and A = {a, b, c, e) and B = (c,d,e). Determine (An B).arrow_forward1) Use the roster method to list the elements of the set consisting of: a) All positive multiples of 3 that are less than 20. b) Nothing (An empty set).arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Discrete Mathematics and Its Applications ( 8th I...MathISBN:9781259676512Author:Kenneth H RosenPublisher:McGraw-Hill Education
Mathematics for Elementary Teachers with Activiti...MathISBN:9780134392790Author:Beckmann, SybillaPublisher:PEARSON
Thinking Mathematically (7th Edition)MathISBN:9780134683713Author:Robert F. BlitzerPublisher:PEARSON
Discrete Mathematics With ApplicationsMathISBN:9781337694193Author:EPP, Susanna S.Publisher:Cengage Learning,
Pathways To Math Literacy (looseleaf)MathISBN:9781259985607Author:David Sobecki Professor, Brian A. MercerPublisher:McGraw-Hill Education
Discrete Mathematics and Its Applications ( 8th I...
Math
ISBN:9781259676512
Author:Kenneth H Rosen
Publisher:McGraw-Hill Education
Mathematics for Elementary Teachers with Activiti...
Math
ISBN:9780134392790
Author:Beckmann, Sybilla
Publisher:PEARSON
Thinking Mathematically (7th Edition)
Math
ISBN:9780134683713
Author:Robert F. Blitzer
Publisher:PEARSON
Discrete Mathematics With Applications
Math
ISBN:9781337694193
Author:EPP, Susanna S.
Publisher:Cengage Learning,
Pathways To Math Literacy (looseleaf)
Math
ISBN:9781259985607
Author:David Sobecki Professor, Brian A. Mercer
Publisher:McGraw-Hill Education
Limits and Continuity; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=9brk313DjV8;License: Standard YouTube License, CC-BY