Algebra 2
1st Edition
ISBN: 9780078884825
Author: McGraw-Hill/Glencoe
Publisher: Glencoe/McGraw-Hill School Pub Co
expand_more
expand_more
format_list_bulleted
Videos
Question
Chapter 2.2, Problem 62STP
To determine
To state: the domain and range of relation. Then determine whether relation is a function, if it is determine if it is one-to-one, onto, both , or neither.
Expert Solution & Answer
Answer to Problem 62STP
Domain and Range are all real numbers.
The relation is a function.
The function is one-to-one and onto.
Explanation of Solution
Given information:
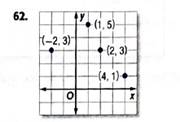
Formula used:
A function is a relation in which each element of the domain is paired with exactly one element in the range.
One-to −One function: Each element of the domain pairs exactly one unique element of the range.
Onto function: Each element of the range corresponds to an element of the domain.
Vertical−Test: If no vertical line intersects a graph in more than one point, the graph represents a function whereas if vertical line intersects a graph more than one point ,the graph does not represent a function.
Calculation:
According to the question ,
Every real number is the x -coordinate of points showed in graph and every real number is
y -coordinate of points showed in graph.
So, the domain and range are both all real numbers.
The graph passes the vertical test. Therefore, it is a function.
The graph shows the following coordinates:
Every x -value is paired with exactly one unique y -value, and every y -value corresponds to an x -value.
Thus, the function is both one-to-one and onto.
Chapter 2 Solutions
Algebra 2
Ch. 2.1 - Prob. 1ACYPCh. 2.1 - Prob. 1BCYPCh. 2.1 - Prob. 2CYPCh. 2.1 - Prob. 3CYPCh. 2.1 - Prob. 4ACYPCh. 2.1 - Prob. 4BCYPCh. 2.1 - Prob. 1CYUCh. 2.1 - Prob. 2CYUCh. 2.1 - Prob. 3CYUCh. 2.1 - Prob. 4CYU
Ch. 2.1 - Prob. 5CYUCh. 2.1 - Prob. 6CYUCh. 2.1 - Prob. 7CYUCh. 2.1 - Prob. 8CYUCh. 2.1 - Prob. 9CYUCh. 2.1 - Prob. 10CYUCh. 2.1 - Prob. 11PPSCh. 2.1 - Prob. 12PPSCh. 2.1 - Prob. 13PPSCh. 2.1 - Prob. 14PPSCh. 2.1 - Prob. 15PPSCh. 2.1 - Prob. 16PPSCh. 2.1 - Prob. 17PPSCh. 2.1 - Prob. 18PPSCh. 2.1 - Prob. 19PPSCh. 2.1 - Prob. 20PPSCh. 2.1 - Prob. 21PPSCh. 2.1 - Prob. 22PPSCh. 2.1 - Prob. 23PPSCh. 2.1 - Prob. 24PPSCh. 2.1 - Prob. 25PPSCh. 2.1 - Prob. 26PPSCh. 2.1 - Prob. 27PPSCh. 2.1 - Prob. 28PPSCh. 2.1 - Prob. 29PPSCh. 2.1 - Prob. 30PPSCh. 2.1 - Prob. 31PPSCh. 2.1 - Prob. 32PPSCh. 2.1 - Prob. 33PPSCh. 2.1 - Prob. 34PPSCh. 2.1 - Prob. 35HPCh. 2.1 - Prob. 36HPCh. 2.1 - Prob. 37HPCh. 2.1 - Prob. 38HPCh. 2.1 - Prob. 39HPCh. 2.1 - Prob. 40HPCh. 2.1 - Prob. 41HPCh. 2.1 - Prob. 42HPCh. 2.1 - Prob. 43HPCh. 2.1 - Prob. 44HPCh. 2.1 - Prob. 45STPCh. 2.1 - Prob. 46STPCh. 2.1 - Prob. 47STPCh. 2.1 - Prob. 48STPCh. 2.1 - Prob. 49STPCh. 2.1 - Prob. 50STPCh. 2.1 - Prob. 51STPCh. 2.1 - Prob. 52STPCh. 2.1 - Prob. 53STPCh. 2.1 - Prob. 54STPCh. 2.1 - Prob. 55STPCh. 2.1 - Prob. 56SCh. 2.1 - Prob. 57SCh. 2.1 - Prob. 58SCh. 2.1 - Prob. 59SCh. 2.1 - Prob. 60SCh. 2.1 - Prob. 61SCh. 2.1 - Prob. 62SCh. 2.1 - Prob. 63SCh. 2.1 - Prob. 64SCh. 2.1 - Prob. 1ECh. 2.1 - Prob. 2ECh. 2.1 - Prob. 3ECh. 2.1 - Prob. 4ECh. 2.1 - Prob. 5ECh. 2.2 - Prob. 1ACYPCh. 2.2 - Prob. 1BCYPCh. 2.2 - Prob. 2ACYPCh. 2.2 - Prob. 2BCYPCh. 2.2 - Prob. 3ACYPCh. 2.2 - Prob. 3BCYPCh. 2.2 - Prob. 4CYPCh. 2.2 - Prob. 1CYUCh. 2.2 - Prob. 2CYUCh. 2.2 - Prob. 3CYUCh. 2.2 - Prob. 4CYUCh. 2.2 - Prob. 5CYUCh. 2.2 - Prob. 6CYUCh. 2.2 - Prob. 7CYUCh. 2.2 - Prob. 8CYUCh. 2.2 - Prob. 9CYUCh. 2.2 - Prob. 10CYUCh. 2.2 - Prob. 11CYUCh. 2.2 - Prob. 12CYUCh. 2.2 - Prob. 13CYUCh. 2.2 - Prob. 14CYUCh. 2.2 - Prob. 15CYUCh. 2.2 - Prob. 16PPSCh. 2.2 - Prob. 17PPSCh. 2.2 - Prob. 18PPSCh. 2.2 - Prob. 19PPSCh. 2.2 - Prob. 20PPSCh. 2.2 - Prob. 21PPSCh. 2.2 - Prob. 22PPSCh. 2.2 - Prob. 23PPSCh. 2.2 - Prob. 24PPSCh. 2.2 - Prob. 25PPSCh. 2.2 - Prob. 26PPSCh. 2.2 - Prob. 27PPSCh. 2.2 - Prob. 28PPSCh. 2.2 - Prob. 29PPSCh. 2.2 - Prob. 30PPSCh. 2.2 - Prob. 31PPSCh. 2.2 - Prob. 32PPSCh. 2.2 - Prob. 33PPSCh. 2.2 - Prob. 34PPSCh. 2.2 - Prob. 35PPSCh. 2.2 - Prob. 36PPSCh. 2.2 - Prob. 37PPSCh. 2.2 - Prob. 38PPSCh. 2.2 - Prob. 39PPSCh. 2.2 - Prob. 40PPSCh. 2.2 - Prob. 41PPSCh. 2.2 - Prob. 42PPSCh. 2.2 - Prob. 43PPSCh. 2.2 - Prob. 44PPSCh. 2.2 - Prob. 45PPSCh. 2.2 - Prob. 46PPSCh. 2.2 - Prob. 47PPSCh. 2.2 - Prob. 48PPSCh. 2.2 - Prob. 49PPSCh. 2.2 - Prob. 50PPSCh. 2.2 - Prob. 51PPSCh. 2.2 - Prob. 52HPCh. 2.2 - Prob. 53HPCh. 2.2 - Prob. 54HPCh. 2.2 - Prob. 55HPCh. 2.2 - Prob. 56HPCh. 2.2 - Prob. 57HPCh. 2.2 - Prob. 58HPCh. 2.2 - Prob. 59HPCh. 2.2 - Prob. 60HPCh. 2.2 - Prob. 61STPCh. 2.2 - Prob. 62STPCh. 2.2 - Prob. 63STPCh. 2.2 - Prob. 64STPCh. 2.2 - Prob. 65STPCh. 2.2 - Prob. 66STPCh. 2.2 - Prob. 67STPCh. 2.2 - Prob. 68STPCh. 2.2 - Prob. 69SCh. 2.2 - Prob. 70SCh. 2.2 - Prob. 71SCh. 2.2 - Prob. 72SCh. 2.2 - Prob. 73SCh. 2.2 - Prob. 74SCh. 2.2 - Prob. 75SCh. 2.2 - Prob. 76SCh. 2.2 - Prob. 1ECh. 2.2 - Prob. 2ECh. 2.2 - Prob. 3ECh. 2.2 - Prob. 4ECh. 2.2 - Prob. 5ECh. 2.2 - Prob. 6ECh. 2.3 - Prob. 1CYPCh. 2.3 - Prob. 2CYPCh. 2.3 - Prob. 3ACYPCh. 2.3 - Prob. 3BCYPCh. 2.3 - Prob. 4ACYPCh. 2.3 - Prob. 4BCYPCh. 2.3 - Prob. 1CYUCh. 2.3 - Prob. 2CYUCh. 2.3 - Prob. 3CYUCh. 2.3 - Prob. 4CYUCh. 2.3 - Prob. 5CYUCh. 2.3 - Prob. 6CYUCh. 2.3 - Prob. 7CYUCh. 2.3 - Prob. 8CYUCh. 2.3 - Prob. 9PPSCh. 2.3 - Prob. 10PPSCh. 2.3 - Prob. 11PPSCh. 2.3 - Prob. 12PPSCh. 2.3 - Prob. 13PPSCh. 2.3 - Prob. 14PPSCh. 2.3 - Prob. 15PPSCh. 2.3 - Prob. 16PPSCh. 2.3 - Prob. 17PPSCh. 2.3 - Prob. 18PPSCh. 2.3 - Prob. 19PPSCh. 2.3 - Prob. 20PPSCh. 2.3 - Prob. 21PPSCh. 2.3 - Prob. 22PPSCh. 2.3 - Prob. 23PPSCh. 2.3 - Prob. 24PPSCh. 2.3 - Prob. 25PPSCh. 2.3 - Prob. 26PPSCh. 2.3 - Prob. 27PPSCh. 2.3 - Prob. 28PPSCh. 2.3 - Prob. 29PPSCh. 2.3 - Prob. 30PPSCh. 2.3 - Prob. 31PPSCh. 2.3 - Prob. 32PPSCh. 2.3 - Prob. 33PPSCh. 2.3 - Prob. 34PPSCh. 2.3 - Prob. 35PPSCh. 2.3 - Prob. 36HPCh. 2.3 - Prob. 37HPCh. 2.3 - Prob. 38HPCh. 2.3 - Prob. 39HPCh. 2.3 - Prob. 40HPCh. 2.3 - Prob. 41HPCh. 2.3 - Prob. 42HPCh. 2.3 - Prob. 43HPCh. 2.3 - Prob. 44HPCh. 2.3 - Prob. 45STPCh. 2.3 - Prob. 46STPCh. 2.3 - Prob. 47STPCh. 2.3 - Prob. 48STPCh. 2.3 - Prob. 49STPCh. 2.3 - Prob. 50STPCh. 2.3 - Prob. 51STPCh. 2.3 - Prob. 52STPCh. 2.3 - Prob. 53STPCh. 2.3 - Prob. 54STPCh. 2.3 - Prob. 55SCh. 2.3 - Prob. 56SCh. 2.3 - Prob. 57SCh. 2.4 - Prob. 1ACYPCh. 2.4 - Prob. 1BCYPCh. 2.4 - Prob. 2ACYPCh. 2.4 - Prob. 2BCYPCh. 2.4 - Prob. 3CYPCh. 2.4 - Prob. 4CYPCh. 2.4 - Prob. 1CYUCh. 2.4 - Prob. 2CYUCh. 2.4 - Prob. 3CYUCh. 2.4 - Prob. 4CYUCh. 2.4 - Prob. 5CYUCh. 2.4 - Prob. 6CYUCh. 2.4 - Prob. 7CYUCh. 2.4 - Prob. 8PPSCh. 2.4 - Prob. 9PPSCh. 2.4 - Prob. 10PPSCh. 2.4 - Prob. 11PPSCh. 2.4 - Prob. 12PPSCh. 2.4 - Prob. 13PPSCh. 2.4 - Prob. 14PPSCh. 2.4 - Prob. 15PPSCh. 2.4 - Prob. 16PPSCh. 2.4 - Prob. 17PPSCh. 2.4 - Prob. 18PPSCh. 2.4 - Prob. 19PPSCh. 2.4 - Prob. 20PPSCh. 2.4 - Prob. 21PPSCh. 2.4 - Prob. 22PPSCh. 2.4 - Prob. 23PPSCh. 2.4 - Prob. 24PPSCh. 2.4 - Prob. 25PPSCh. 2.4 - Prob. 26PPSCh. 2.4 - Prob. 27PPSCh. 2.4 - Prob. 28PPSCh. 2.4 - Prob. 29PPSCh. 2.4 - Prob. 30PPSCh. 2.4 - Prob. 31PPSCh. 2.4 - Prob. 32PPSCh. 2.4 - Prob. 33PPSCh. 2.4 - Prob. 34PPSCh. 2.4 - Prob. 35PPSCh. 2.4 - Prob. 36PPSCh. 2.4 - Prob. 37HPCh. 2.4 - Prob. 38HPCh. 2.4 - Prob. 39HPCh. 2.4 - Prob. 40HPCh. 2.4 - Prob. 41HPCh. 2.4 - Prob. 42HPCh. 2.4 - Prob. 43HPCh. 2.4 - Prob. 44HPCh. 2.4 - Prob. 45HPCh. 2.4 - Prob. 46HPCh. 2.4 - Prob. 47STPCh. 2.4 - Prob. 48STPCh. 2.4 - Prob. 49STPCh. 2.4 - Prob. 50STPCh. 2.4 - Prob. 51STPCh. 2.4 - Prob. 52STPCh. 2.4 - Prob. 53STPCh. 2.4 - Prob. 54STPCh. 2.4 - Prob. 55STPCh. 2.4 - Prob. 56STPCh. 2.4 - Prob. 57STPCh. 2.4 - Prob. 58STPCh. 2.4 - Prob. 59STPCh. 2.4 - Prob. 60SCh. 2.4 - Prob. 61SCh. 2.4 - Prob. 62SCh. 2.4 - Prob. 63SCh. 2.4 - Prob. 64SCh. 2.4 - Prob. 65SCh. 2.4 - Prob. 1ECh. 2.4 - Prob. 2ECh. 2.5 - Prob. 1CYPCh. 2.5 - Prob. 2CYPCh. 2.5 - Prob. 1CYUCh. 2.5 - Prob. 2CYUCh. 2.5 - Prob. 3PPSCh. 2.5 - Prob. 4PPSCh. 2.5 - Prob. 5PPSCh. 2.5 - Prob. 6PPSCh. 2.5 - Prob. 7PPSCh. 2.5 - Prob. 8PPSCh. 2.5 - Prob. 9PPSCh. 2.5 - Prob. 10PPSCh. 2.5 - Prob. 11PPSCh. 2.5 - Prob. 12HPCh. 2.5 - Prob. 13HPCh. 2.5 - Prob. 14HPCh. 2.5 - Prob. 15HPCh. 2.5 - Prob. 16HPCh. 2.5 - Prob. 17HPCh. 2.5 - Prob. 18HPCh. 2.5 - Prob. 19HPCh. 2.5 - Prob. 20HPCh. 2.5 - Prob. 21STPCh. 2.5 - Prob. 22STPCh. 2.5 - Prob. 23STPCh. 2.5 - Prob. 24STPCh. 2.5 - Prob. 25STPCh. 2.5 - Prob. 26STPCh. 2.5 - Prob. 27STPCh. 2.5 - Prob. 28STPCh. 2.5 - Prob. 29STPCh. 2.5 - Prob. 30SCh. 2.5 - Prob. 31SCh. 2.5 - Prob. 32SCh. 2.5 - Prob. 1ECh. 2.5 - Prob. 2ECh. 2.5 - Prob. 3ECh. 2.5 - Prob. 4ECh. 2.6 - Prob. 1CYPCh. 2.6 - Prob. 2ACYPCh. 2.6 - Prob. 2BCYPCh. 2.6 - Prob. 3CYPCh. 2.6 - Prob. 4ACYPCh. 2.6 - Prob. 4BCYPCh. 2.6 - Prob. 1CYUCh. 2.6 - Prob. 2CYUCh. 2.6 - Prob. 3CYUCh. 2.6 - Prob. 4CYUCh. 2.6 - Prob. 5CYUCh. 2.6 - Prob. 6CYUCh. 2.6 - Prob. 7CYUCh. 2.6 - Prob. 8CYUCh. 2.6 - Prob. 9CYUCh. 2.6 - Prob. 10CYUCh. 2.6 - Prob. 11CYUCh. 2.6 - Prob. 12PPSCh. 2.6 - Prob. 13PPSCh. 2.6 - Prob. 14PPSCh. 2.6 - Prob. 15PPSCh. 2.6 - Prob. 16PPSCh. 2.6 - Prob. 17PPSCh. 2.6 - Prob. 18PPSCh. 2.6 - Prob. 19PPSCh. 2.6 - Prob. 20PPSCh. 2.6 - Prob. 21PPSCh. 2.6 - Prob. 22PPSCh. 2.6 - Prob. 23PPSCh. 2.6 - Prob. 24PPSCh. 2.6 - Prob. 25PPSCh. 2.6 - Prob. 26PPSCh. 2.6 - Prob. 27PPSCh. 2.6 - Prob. 28PPSCh. 2.6 - Prob. 29PPSCh. 2.6 - Prob. 30PPSCh. 2.6 - Prob. 31PPSCh. 2.6 - Prob. 32PPSCh. 2.6 - Prob. 33PPSCh. 2.6 - Prob. 34PPSCh. 2.6 - Prob. 35PPSCh. 2.6 - Prob. 36PPSCh. 2.6 - Prob. 37PPSCh. 2.6 - Prob. 38PPSCh. 2.6 - Prob. 39PPSCh. 2.6 - Prob. 40HPCh. 2.6 - Prob. 41HPCh. 2.6 - Prob. 42HPCh. 2.6 - Prob. 43HPCh. 2.6 - Prob. 44HPCh. 2.6 - Prob. 45HPCh. 2.6 - Prob. 46HPCh. 2.6 - Prob. 47HPCh. 2.6 - Prob. 48HPCh. 2.6 - Prob. 49STPCh. 2.6 - Prob. 50STPCh. 2.6 - Prob. 51STPCh. 2.6 - Prob. 52STPCh. 2.6 - Prob. 53STPCh. 2.6 - Prob. 54STPCh. 2.6 - Prob. 55STPCh. 2.6 - Prob. 56STPCh. 2.6 - Prob. 57SCh. 2.6 - Prob. 58SCh. 2.6 - Prob. 59SCh. 2.7 - Prob. 1ACYPCh. 2.7 - Prob. 1BCYPCh. 2.7 - Prob. 2ACYPCh. 2.7 - Prob. 2BCYPCh. 2.7 - Prob. 3ACYPCh. 2.7 - Prob. 3BCYPCh. 2.7 - Prob. 4ACYPCh. 2.7 - Prob. 4BCYPCh. 2.7 - Prob. 5CYPCh. 2.7 - Prob. 1CYUCh. 2.7 - Prob. 2CYUCh. 2.7 - Prob. 3CYUCh. 2.7 - Prob. 4CYUCh. 2.7 - Prob. 5CYUCh. 2.7 - Prob. 6CYUCh. 2.7 - Prob. 7CYUCh. 2.7 - Prob. 8CYUCh. 2.7 - Prob. 9CYUCh. 2.7 - Prob. 10PPSCh. 2.7 - Prob. 11PPSCh. 2.7 - Prob. 12PPSCh. 2.7 - Prob. 13PPSCh. 2.7 - Prob. 14PPSCh. 2.7 - Prob. 15PPSCh. 2.7 - Prob. 16PPSCh. 2.7 - Prob. 17PPSCh. 2.7 - Prob. 18PPSCh. 2.7 - Prob. 19PPSCh. 2.7 - Prob. 20PPSCh. 2.7 - Prob. 21PPSCh. 2.7 - Prob. 22PPSCh. 2.7 - Prob. 23PPSCh. 2.7 - Prob. 24PPSCh. 2.7 - Prob. 25PPSCh. 2.7 - Prob. 26PPSCh. 2.7 - Prob. 27PPSCh. 2.7 - Prob. 28PPSCh. 2.7 - Prob. 29PPSCh. 2.7 - Prob. 30PPSCh. 2.7 - Prob. 31PPSCh. 2.7 - Prob. 32PPSCh. 2.7 - Prob. 33PPSCh. 2.7 - Prob. 34PPSCh. 2.7 - Prob. 35PPSCh. 2.7 - Prob. 36PPSCh. 2.7 - Prob. 37PPSCh. 2.7 - Prob. 38PPSCh. 2.7 - Prob. 39PPSCh. 2.7 - Prob. 40PPSCh. 2.7 - Prob. 41PPSCh. 2.7 - Prob. 42PPSCh. 2.7 - Prob. 43HPCh. 2.7 - Prob. 44HPCh. 2.7 - Prob. 45HPCh. 2.7 - Prob. 46HPCh. 2.7 - Prob. 47HPCh. 2.7 - Prob. 48HPCh. 2.7 - Prob. 49HPCh. 2.7 - Prob. 50HPCh. 2.7 - Prob. 51HPCh. 2.7 - Prob. 52STPCh. 2.7 - Prob. 53STPCh. 2.7 - Prob. 54STPCh. 2.7 - Prob. 55STPCh. 2.7 - Prob. 56STPCh. 2.7 - Prob. 57STPCh. 2.7 - Prob. 58STPCh. 2.7 - Prob. 59STPCh. 2.7 - Prob. 60STPCh. 2.7 - Prob. 61STPCh. 2.7 - Prob. 62STPCh. 2.7 - Prob. 63SCh. 2.7 - Prob. 64SCh. 2.7 - Prob. 65SCh. 2.8 - Prob. 1ACYPCh. 2.8 - Prob. 1BCYPCh. 2.8 - Prob. 2CYPCh. 2.8 - Prob. 3ACYPCh. 2.8 - Prob. 3BCYPCh. 2.8 - Prob. 1CYUCh. 2.8 - Prob. 2CYUCh. 2.8 - Prob. 3CYUCh. 2.8 - Prob. 4CYUCh. 2.8 - Prob. 5CYUCh. 2.8 - Prob. 6CYUCh. 2.8 - Prob. 7CYUCh. 2.8 - Prob. 8PPSCh. 2.8 - Prob. 9PPSCh. 2.8 - Prob. 10PPSCh. 2.8 - Prob. 11PPSCh. 2.8 - Prob. 12PPSCh. 2.8 - Prob. 13PPSCh. 2.8 - Prob. 14PPSCh. 2.8 - Prob. 15PPSCh. 2.8 - Prob. 16PPSCh. 2.8 - Prob. 17PPSCh. 2.8 - Prob. 18PPSCh. 2.8 - Prob. 19PPSCh. 2.8 - Prob. 20PPSCh. 2.8 - Prob. 21PPSCh. 2.8 - Prob. 22PPSCh. 2.8 - Prob. 23PPSCh. 2.8 - Prob. 24PPSCh. 2.8 - Prob. 25PPSCh. 2.8 - Prob. 26PPSCh. 2.8 - Prob. 27PPSCh. 2.8 - Prob. 28PPSCh. 2.8 - Prob. 29PPSCh. 2.8 - Prob. 30PPSCh. 2.8 - Prob. 31PPSCh. 2.8 - Prob. 32PPSCh. 2.8 - Prob. 33HPCh. 2.8 - Prob. 34HPCh. 2.8 - Prob. 35HPCh. 2.8 - Prob. 36HPCh. 2.8 - Prob. 37HPCh. 2.8 - Prob. 38HPCh. 2.8 - Prob. 39HPCh. 2.8 - Prob. 40HPCh. 2.8 - Prob. 41HPCh. 2.8 - Prob. 42STPCh. 2.8 - Prob. 43STPCh. 2.8 - Prob. 44STPCh. 2.8 - Prob. 45STPCh. 2.8 - Prob. 46STPCh. 2.8 - Prob. 47STPCh. 2.8 - Prob. 48STPCh. 2.8 - Prob. 49STPCh. 2.8 - Prob. 50STPCh. 2.8 - Prob. 51STPCh. 2.8 - Prob. 52STPCh. 2.8 - Prob. 53STPCh. 2.8 - Prob. 54STPCh. 2.8 - Prob. 55SCh. 2.8 - Prob. 56SCh. 2.8 - Prob. 57SCh. 2 - Prob. 1QCCh. 2 - Prob. 2QCCh. 2 - Prob. 3QCCh. 2 - Prob. 4QCCh. 2 - Prob. 5QCCh. 2 - Prob. 6QCCh. 2 - Prob. 7QCCh. 2 - Prob. 8QCCh. 2 - Prob. 9QCCh. 2 - Prob. 10QCCh. 2 - Prob. 11QCCh. 2 - Prob. 12QCCh. 2 - Prob. 13QCCh. 2 - Prob. 14QCCh. 2 - Prob. 15QCCh. 2 - Prob. 16QCCh. 2 - Prob. 1MCQCh. 2 - Prob. 2MCQCh. 2 - Prob. 3MCQCh. 2 - Prob. 4MCQCh. 2 - Prob. 5MCQCh. 2 - Prob. 6MCQCh. 2 - Prob. 7MCQCh. 2 - Prob. 8MCQCh. 2 - Prob. 9MCQCh. 2 - Prob. 10MCQCh. 2 - Prob. 11MCQCh. 2 - Prob. 12MCQCh. 2 - Prob. 13MCQCh. 2 - Prob. 14MCQCh. 2 - Prob. 15MCQCh. 2 - Prob. 16MCQCh. 2 - Prob. 17MCQCh. 2 - Prob. 18MCQCh. 2 - Prob. 19MCQCh. 2 - Prob. 20MCQCh. 2 - Prob. 21MCQCh. 2 - Prob. 22MCQCh. 2 - Prob. 23MCQCh. 2 - Prob. 24MCQCh. 2 - Prob. 25MCQCh. 2 - Prob. 1SGRCh. 2 - Prob. 2SGRCh. 2 - Prob. 3SGRCh. 2 - Prob. 4SGRCh. 2 - Prob. 5SGRCh. 2 - Prob. 6SGRCh. 2 - Prob. 7SGRCh. 2 - Prob. 8SGRCh. 2 - Prob. 9SGRCh. 2 - Prob. 10SGRCh. 2 - Prob. 11SGRCh. 2 - Prob. 12SGRCh. 2 - Prob. 13SGRCh. 2 - Prob. 14SGRCh. 2 - Prob. 15SGRCh. 2 - Prob. 16SGRCh. 2 - Prob. 17SGRCh. 2 - Prob. 18SGRCh. 2 - Prob. 19SGRCh. 2 - Prob. 20SGRCh. 2 - Prob. 21SGRCh. 2 - Prob. 22SGRCh. 2 - Prob. 23SGRCh. 2 - Prob. 24SGRCh. 2 - Prob. 25SGRCh. 2 - Prob. 26SGRCh. 2 - Prob. 27SGRCh. 2 - Prob. 28SGRCh. 2 - Prob. 29SGRCh. 2 - Prob. 30SGRCh. 2 - Prob. 31SGRCh. 2 - Prob. 32SGRCh. 2 - Prob. 33SGRCh. 2 - Prob. 34SGRCh. 2 - Prob. 35SGRCh. 2 - Prob. 36SGRCh. 2 - Prob. 37SGRCh. 2 - Prob. 38SGRCh. 2 - Prob. 39SGRCh. 2 - Prob. 40SGRCh. 2 - Prob. 41SGRCh. 2 - Prob. 42SGRCh. 2 - Prob. 43SGRCh. 2 - Prob. 44SGRCh. 2 - Prob. 45SGRCh. 2 - Prob. 46SGRCh. 2 - Prob. 47SGRCh. 2 - Prob. 48SGRCh. 2 - Prob. 49SGRCh. 2 - Prob. 50SGRCh. 2 - Prob. 51SGRCh. 2 - Prob. 52SGRCh. 2 - Prob. 53SGRCh. 2 - Prob. 54SGRCh. 2 - Prob. 55SGRCh. 2 - Prob. 56SGRCh. 2 - Prob. 57SGRCh. 2 - Prob. 58SGRCh. 2 - Prob. 59SGRCh. 2 - Prob. 60SGRCh. 2 - Prob. 61SGRCh. 2 - Prob. 62SGRCh. 2 - Prob. 1PTCh. 2 - Prob. 2PTCh. 2 - Prob. 3PTCh. 2 - Prob. 4PTCh. 2 - Prob. 5PTCh. 2 - Prob. 6PTCh. 2 - Prob. 7PTCh. 2 - Prob. 8PTCh. 2 - Prob. 9PTCh. 2 - Prob. 10PTCh. 2 - Prob. 11PTCh. 2 - Prob. 12PTCh. 2 - Prob. 13PTCh. 2 - Prob. 14PTCh. 2 - Prob. 15PTCh. 2 - Prob. 16PTCh. 2 - Prob. 17PTCh. 2 - Prob. 18PTCh. 2 - Prob. 19PTCh. 2 - Prob. 20PTCh. 2 - Prob. 1ECh. 2 - Prob. 2ECh. 2 - Prob. 1STPCh. 2 - Prob. 2STPCh. 2 - Prob. 3STPCh. 2 - Prob. 4STPCh. 2 - Prob. 5STPCh. 2 - Prob. 6STPCh. 2 - Prob. 7STPCh. 2 - Prob. 8STPCh. 2 - Prob. 9STPCh. 2 - Prob. 10STPCh. 2 - Prob. 11STPCh. 2 - Prob. 12STP
Additional Math Textbook Solutions
Find more solutions based on key concepts
Explain the meaning of the term “statistically significant difference” in statistics terminology.
Intro Stats, Books a la Carte Edition (5th Edition)
The given statement as true of false.
Pre-Algebra Student Edition
Integrals involving tan x and sec x Evaluate the following integrals. 35. tan34xdx
Calculus: Early Transcendentals (2nd Edition)
In Exercises 1–6, find the average rate of change of the function over the given interval or intervals.
3.
University Calculus: Early Transcendentals (4th Edition)
CHECK POINT 1 Find a counterexample to show that the statement The product of two two-digit numbers is a three-...
Thinking Mathematically (6th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- > > > we are hiring Salesforce Admin Location: Remote Key Responsibilities: Administer Salesforce Sales & Revenue Cloud (CPQ & Billing) Configure workflows, validation rules & dashboards Automate processes using Flows & Process Builder Collaborate with Sales, Finance & Marketing teams Manage user roles & security Apply: Hr@forcecraver.comarrow_forwardAnswer this questionarrow_forward1. vector projection. Assume, ER1001 and you know the following: ||||=4, 7=-0.5.7. For each of the following, explicitly compute the value. འབ (a) (b) (c) (d) answer. Explicitly compute ||y7||. Explain your answer. Explicitly compute the cosine similarity of and y. Explain your Explicitly compute (x, y). Explain your answer. Find the projection of onto y and the projection of onto .arrow_forward
- 2. Answer the following questions using vectors u and v. --0-0-0 = find the the cosine similarity and the angle between u and v. འརྒྱ (a) (b) find the scalar projection of u onto v. (c) find the projection of u onto v. (d) (e) (f) find the scalar projection of onto u. find the projection of u onto u. find the projection of u onto and the projection of onto . (Hint: find the inner product and verify the orthogonality)arrow_forwardPlease type out answerarrow_forwardPlease type out answerarrow_forward
- Please type out answerarrow_forwardUsing f(x) = log x, what is the x-intercept of g(x) = log (x + 4)? Explain your reasoning. Please type out answerarrow_forwardThe function f(x) = log x is transformed to produce g(x) = log (x) – 3. Identify the type of transformation and describe the change. Please type out answerarrow_forward
- Each graph below is the graph of a system of three linear equations in three unknowns of the form Ax = b. Determine whether each system has a solution and, if it does, the number of free variables. A. O free variables ✓ B. no solution C. no solution D. no solution E. 1 free variable F. 1 free variablearrow_forwardSolve the following systems of equations and show all work.y = x2 + 3y = x + 5 Please type out answerarrow_forwardSolve the following system of equations. Show all work and solutions.y = 2x2 + 6x + 1y = −4x2 + 1 Please type out answerarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON
Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON
Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education
What is a Relation? | Don't Memorise; Author: Don't Memorise;https://www.youtube.com/watch?v=hV1_wvsdJCE;License: Standard YouTube License, CC-BY
RELATIONS-DOMAIN, RANGE AND CO-DOMAIN (RELATIONS AND FUNCTIONS CBSE/ ISC MATHS); Author: Neha Agrawal Mathematically Inclined;https://www.youtube.com/watch?v=u4IQh46VoU4;License: Standard YouTube License, CC-BY