
Concept explainers
(a)
Interpretation:
The closest
Concept introduction:
A unit cell of the crystal is the three-dimensional arrangement of the atoms present in the crystal. The unit cell is the smallest and simplest unit of the crystal which on repetition forms an entire crystal. Unit cell can be a cubic unit cell or hexagonal unit cell. The classification of a unit cell depends on the lattice site occupied by the atoms.
Answer to Problem 22.35E
The closest
Explanation of Solution
The structure of a face-centered cubic lattice is shown below.
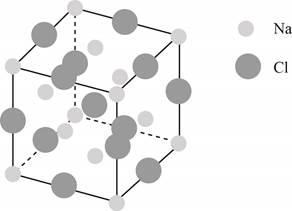
Figure 1
The plane of face-centered cubic lattice that has
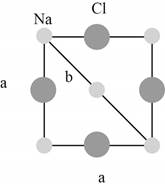
Figure 2
The closest
The lattice parameter
The Pythagoras theorem is shown below.
Where,
•
•
•
The hypotenuse of the triangle shown in Figure (2) is
The base and perpendicular of the triangle shown in Figure (2) are
Substitute the value of hypotenuse, base, and perpendicular in the equation (1).
Substitute the value of
Therefore, the closest
The closest
(b)
Interpretation:
The closest
Concept introduction:
A unit cell of the crystal is the three-dimensional arrangement of the atoms present in the crystal. The unit cell is the smallest and simplest unit of the crystal which on repetition forms an entire crystal. Unit cell can be a cubic unit cell or hexagonal unit cell. The classification of a unit cell depends on the lattice site occupied by the atoms.
Answer to Problem 22.35E
The closest
Explanation of Solution
The structure of a face-centered cubic lattice is shown below.
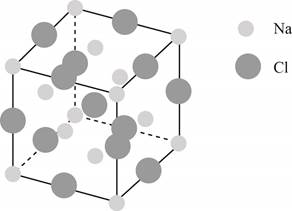
Figure 1
The plane of face-centered cubic lattice that has
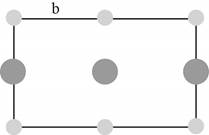
Figure 3
The lattice parameter
The relation between the length of the edge of a cube
The diagonal of the triangle shown in Figure (3) is
Substitute the value of
Therefore, the closest
The closest
(c)
Interpretation:
The closest
Concept introduction:
A unit cell of the crystal is the three-dimensional arrangement of the atoms present in the crystal. The unit cell is the smallest and simplest unit of the crystal which on repetition forms an entire crystal. Unit cell can be a cubic unit cell or hexagonal unit cell. The classification of a unit cell depends on the lattice site occupied by the atoms.
Answer to Problem 22.35E
The closest
Explanation of Solution
The structure of a face-centered cubic lattice is shown below.
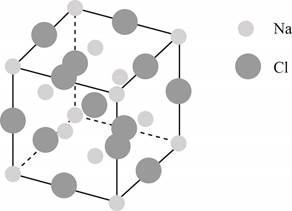
Figure 1
The plane of face-centered cubic lattice that has
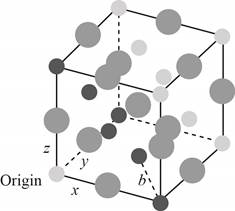
Figure 4
The side of the trigonal plane is same as the diagonal of the of the cube.
The lattice parameter
The relation between the length of the edge of a cube
The diagonal of the triangle shown in Figure (4) is
Substitute the value of
Therefore, the closest
The closest
Want to see more full solutions like this?
Chapter 22 Solutions
Physical Chemistry
- How many hydrogen atoms are connected to the indicated carbon atom?arrow_forwardIdentify the compound with the longest carbon - nitrogen bond. O CH3CH2CH=NH O CH3CH2NH2 CH3CH2C=N CH3CH=NCH 3 The length of all the carbon-nitrogen bonds are the samearrow_forwardIdentify any polar covalent bonds in epichlorohydrin with S+ and 8- symbols in the appropriate locations. Choose the correct answer below. Η H's+ 6Η Η Η Η Η Ηδ Η Ο Ο HH +Η Η +Η Η Η -8+ CIarrow_forward
- H H:O::::H H H HH H::O:D:D:H HH HH H:O:D:D:H .. HH H:O:D:D:H H H Select the correct Lewis dot structure for the following compound: CH3CH2OHarrow_forwardRank the following compounds in order of decreasing boiling point. ннннн -С-С-Н . н-с- ННННН H ΗΤΗ НННН TTTĪ н-с-с-с-с-о-н НННН НН C' Н н-с-с-с-с-н НН || Ш НННН H-C-C-C-C-N-H ННННН IVarrow_forwardRank the following compounds in order of decreasing dipole moment. |>||>||| ||>|||>| |>|||>|| |||>||>| O ||>>||| H F H F H c=c || H c=c F F IIIarrow_forward
- choose the description that best describes the geometry for the following charged species ch3-arrow_forwardWhy isn't the ketone in this compound converted to an acetal or hemiacetal by the alcohol and acid?arrow_forwardWhat is the approximate bond angle around the nitrogen atom? HNH H Harrow_forward
- OH 1. NaOCH2CH3 Q 2. CH3CH2Br (1 equiv) H3O+ Select to Draw 1. NaOCH2 CH3 2. CH3Br (1 equiv) heat Select to Edit Select to Drawarrow_forwardComplete and balance the following half-reaction in acidic solution. Be sure to include the proper phases for all species within the reaction. S₂O₃²⁻(aq) → S₄O₆²⁻(aq)arrow_forwardQ Select to Edit NH3 (CH3)2CHCI (1 equiv) AICI 3 Select to Draw cat. H2SO4 SO3 (1 equiv) HO SOCl2 pyridine Select to Edit >arrow_forward
 Principles of Modern ChemistryChemistryISBN:9781305079113Author:David W. Oxtoby, H. Pat Gillis, Laurie J. ButlerPublisher:Cengage Learning
Principles of Modern ChemistryChemistryISBN:9781305079113Author:David W. Oxtoby, H. Pat Gillis, Laurie J. ButlerPublisher:Cengage Learning Physical ChemistryChemistryISBN:9781133958437Author:Ball, David W. (david Warren), BAER, TomasPublisher:Wadsworth Cengage Learning,
Physical ChemistryChemistryISBN:9781133958437Author:Ball, David W. (david Warren), BAER, TomasPublisher:Wadsworth Cengage Learning, Chemistry: Principles and PracticeChemistryISBN:9780534420123Author:Daniel L. Reger, Scott R. Goode, David W. Ball, Edward MercerPublisher:Cengage Learning
Chemistry: Principles and PracticeChemistryISBN:9780534420123Author:Daniel L. Reger, Scott R. Goode, David W. Ball, Edward MercerPublisher:Cengage Learning General Chemistry - Standalone book (MindTap Cour...ChemistryISBN:9781305580343Author:Steven D. Gammon, Ebbing, Darrell Ebbing, Steven D., Darrell; Gammon, Darrell Ebbing; Steven D. Gammon, Darrell D.; Gammon, Ebbing; Steven D. Gammon; DarrellPublisher:Cengage Learning
General Chemistry - Standalone book (MindTap Cour...ChemistryISBN:9781305580343Author:Steven D. Gammon, Ebbing, Darrell Ebbing, Steven D., Darrell; Gammon, Darrell Ebbing; Steven D. Gammon, Darrell D.; Gammon, Ebbing; Steven D. Gammon; DarrellPublisher:Cengage Learning Chemistry: Principles and ReactionsChemistryISBN:9781305079373Author:William L. Masterton, Cecile N. HurleyPublisher:Cengage Learning
Chemistry: Principles and ReactionsChemistryISBN:9781305079373Author:William L. Masterton, Cecile N. HurleyPublisher:Cengage Learning Chemistry & Chemical ReactivityChemistryISBN:9781337399074Author:John C. Kotz, Paul M. Treichel, John Townsend, David TreichelPublisher:Cengage Learning
Chemistry & Chemical ReactivityChemistryISBN:9781337399074Author:John C. Kotz, Paul M. Treichel, John Townsend, David TreichelPublisher:Cengage Learning





