EBK PRECALCULUS: MATHEMATICS FOR CALCUL

6th Edition

ISBN: 9781133715047

Author: Watson

Publisher: YUZU

See similar textbooks

Concept explainers

Minimization

In mathematics, traditional optimization problems are typically expressed in terms of minimization. When we talk about minimizing or maximizing a function, we refer to the maximum and minimum possible values of that function. This can be expressed in ter…

Maxima and Minima

The extreme points of a function are the maximum and the minimum points of the function. A maximum is attained when the function takes the maximum value and a minimum is attained when the function takes the minimum value.

Derivatives

A derivative means a change. Geometrically it can be represented as a line with some steepness. Imagine climbing a mountain which is very steep and 500 meters high. Is it easier to climb? Definitely not! Suppose walking on the road for 500 meters. Which …

Concavity

In calculus, concavity is a descriptor of mathematics that tells about the shape of the graph. It is the parameter that helps to estimate the maximum and minimum value of any of the functions and the concave nature using the graphical method. We use the …

Videos

Textbook Question

Chapter 2, Problem 32P

Area of a Kite A kite frame is to be made from six pieces of wood. The four pieces that form its border have been cut to the lengths indicated in the figure. Let x be as shown in the figure

(a) Show that the area of the kite is given by the function

A ( x ) = x ( 25 − x 2 + 144 − x 2 )

(b) How long should each of the two crosspieces be to maximize the area of the kite?

(a)

Expert Solution

To determine

To show: The area function of the kite is A(x)=x(25−x2+144−x2) .

Explanation of Solution

The kite contains four triangles with finite lengths is shown below,

EBK PRECALCULUS: MATHEMATICS FOR CALCUL, Chapter 2, Problem 32P , additional homework tip 1

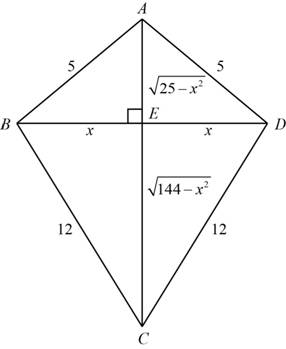
Figure (1)

In ΔAEB ,

Use the Pythagoras theorem to find the length AE,

AB2=AE2+BE2 [∵Hyptheses2=base2+perpendicular2](5)2=AE2+x2AE2=25−x2

In ΔDEC

Use the Pythagoras theorem to find the length CE,

DC2=EC2+DE2 [∵Hyptheses2=base2+perpendicular2](12)2=EC2+x2AE2=144−x2

The formula to calculate the area of triangle is,

Area of triangle = 12×base×Height

The formula to calculate area of kite,

Area of kite = Area of ΔABD+Area of ΔBCD= 12×2x×25−x2+ 12×2x×144−x2A(x)=x(25−x2+144−x2) square units

Thus, the area function of the kite is A(x)=x(25−x2+144−x2) .

(b)

Expert Solution

To determine

To find: The length of the cross pieces of the kite to maximize the area of the kite.

Answer to Problem 32P

The length of the two cross pieces are 9.952units and the 11.3757units of each of the cross pieces.

Explanation of Solution

Given:

The given kite frame to be made from six pieces of wood is shown below,

EBK PRECALCULUS: MATHEMATICS FOR CALCUL, Chapter 2, Problem 32P , additional homework tip 2

Figure (1)

From the part (a), the area function is A(x)=x(25−x2+144−x2) .

To find the lengths of the cross pieces of the kite which maximize the area of the kite, it needs to draw the graph of the function A(x)=x(25−x2+144−x2) .

The function contains the variable x is length of the horizontal cross piece.

The local maximum value of the function is the maximum finite value where the value of the function at the any number is greater than to the original function.

The condition for local minimum is,

f(a)≥f(x)

The graph of the function is shown below.

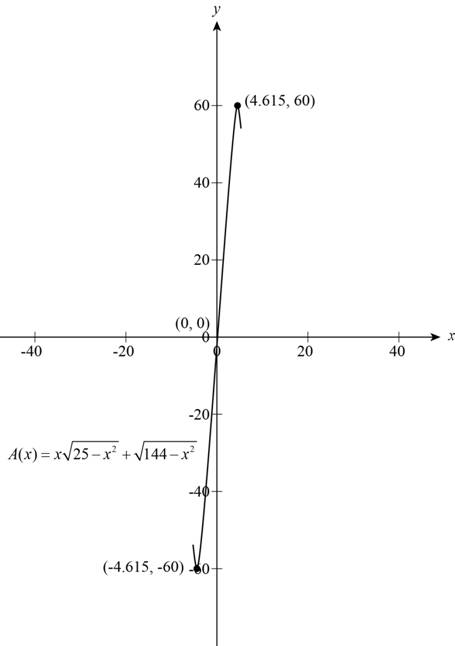
EBK PRECALCULUS: MATHEMATICS FOR CALCUL, Chapter 2, Problem 32P , additional homework tip 3

Figure (2)

From the graph of the function shown as Figure (2) the greatest peak of the graph at the point (4.615,60) .

Then, the maximum area of the kite is 60mi2 at x=4.615 .

The length of the side BD in the figure (1) is,

Substitute 4.979 for x in the value of BD=2x .

BD=2x=2⋅4.979=9.958

The length of the side AC in the figure (1) is 25−x2+144−x2 .

Now, substitute 4.979 for x in the length of the side AC.

AC=25−x2+144−x2=25−(4.979)2+144−(4.979)2=11.3757

So, the original value of x is 4.979miles .

Thus, the value of one cross piece is 9.952miles and the other crosspiece is 11.3757miles .

Chapter 2, Problem 31P

Chapter 3.1, Problem 1E

Chapter 2 Solutions

EBK PRECALCULUS: MATHEMATICS FOR CALCUL

Ch. 2.1 - Prob. 1E Ch. 2.1 - For a function f, the set of all possible inputs...Ch. 2.1 - (a) Which of the following functions have 5 in...Ch. 2.1 - A function is given algebraically by the formula...Ch. 2.1 - Prob. 5E Ch. 2.1 - Prob. 6E Ch. 2.1 - Prob. 7E Ch. 2.1 - Prob. 8E Ch. 2.1 - Prob. 9E Ch. 2.1 - Prob. 10E

Ch. 2.1 - Prob. 11E Ch. 2.1 - Prob. 12E Ch. 2.1 - Prob. 13E Ch. 2.1 - Prob. 14E Ch. 2.1 - Prob. 15E Ch. 2.1 - Prob. 16E Ch. 2.1 - Prob. 17E Ch. 2.1 - Prob. 18E Ch. 2.1 - Prob. 19E Ch. 2.1 - Prob. 20E Ch. 2.1 - Prob. 21E Ch. 2.1 - Prob. 22E Ch. 2.1 - Prob. 23E Ch. 2.1 - Prob. 24E Ch. 2.1 - Prob. 25E Ch. 2.1 - Prob. 26E Ch. 2.1 - Prob. 27E Ch. 2.1 - Prob. 28E Ch. 2.1 - Prob. 29E Ch. 2.1 - Prob. 30E Ch. 2.1 - Prob. 31E Ch. 2.1 - Prob. 32E Ch. 2.1 - Prob. 33E Ch. 2.1 - Prob. 34E Ch. 2.1 - Prob. 35E Ch. 2.1 - Prob. 36E Ch. 2.1 - Prob. 37E Ch. 2.1 - Prob. 38E Ch. 2.1 - Prob. 39E Ch. 2.1 - Prob. 40E Ch. 2.1 - Prob. 41E Ch. 2.1 - Prob. 42E Ch. 2.1 - Prob. 43E Ch. 2.1 - Prob. 44E Ch. 2.1 - Prob. 45E Ch. 2.1 - Prob. 46E Ch. 2.1 - Prob. 47E Ch. 2.1 - Prob. 48E Ch. 2.1 - Prob. 49E Ch. 2.1 - Prob. 50E Ch. 2.1 - Prob. 51E Ch. 2.1 - Prob. 52E Ch. 2.1 - Prob. 53E Ch. 2.1 - Prob. 54E Ch. 2.1 - Prob. 55E Ch. 2.1 - Prob. 56E Ch. 2.1 - Prob. 57E Ch. 2.1 - Prob. 58E Ch. 2.1 - Prob. 59E Ch. 2.1 - Prob. 60E Ch. 2.1 - Prob. 61E Ch. 2.1 - Prob. 62E Ch. 2.1 - Prob. 63E Ch. 2.1 - Prob. 64E Ch. 2.1 - Prob. 65E Ch. 2.1 - Prob. 66E Ch. 2.1 - Prob. 67E Ch. 2.1 - Prob. 68E Ch. 2.1 - Prob. 69E Ch. 2.1 - Prob. 70E Ch. 2.1 - Prob. 71E Ch. 2.1 - Prob. 72E Ch. 2.1 - Prob. 73E Ch. 2.1 - Prob. 74E Ch. 2.1 - Relativity According to the Theory of Relativity,...Ch. 2.1 - Income Tax In a certain country, income tax T is...Ch. 2.1 - Internet Purchases An Internet bookstore charges...Ch. 2.1 - Cost of a Hotel Stay A hotel chain charges 75 each...Ch. 2.1 - Speeding Tickets In a certain state the maximum...Ch. 2.1 - Height of Grass A home owner mows the lawn every...Ch. 2.1 - Prob. 81E Ch. 2.1 - Prob. 82E Ch. 2.1 - Prob. 83E Ch. 2.1 - Prob. 84E Ch. 2.1 - Prob. 85E Ch. 2.2 - Prob. 1E Ch. 2.2 - Prob. 2E Ch. 2.2 - Prob. 3E Ch. 2.2 - Match the function with its graph. (a) f(x) = x2...Ch. 2.2 - Prob. 5E Ch. 2.2 - Prob. 6E Ch. 2.2 - Prob. 7E Ch. 2.2 - Prob. 8E Ch. 2.2 - Prob. 9E Ch. 2.2 - Prob. 10E Ch. 2.2 - Prob. 11E Ch. 2.2 - Prob. 12E Ch. 2.2 - Prob. 13E Ch. 2.2 - Prob. 14E Ch. 2.2 - Prob. 15E Ch. 2.2 - Prob. 16E Ch. 2.2 - Prob. 17E Ch. 2.2 - Prob. 18E Ch. 2.2 - Prob. 19E Ch. 2.2 - Prob. 20E Ch. 2.2 - Prob. 21E Ch. 2.2 - Prob. 22E Ch. 2.2 - Prob. 23E Ch. 2.2 - Prob. 24E Ch. 2.2 - Prob. 25E Ch. 2.2 - Prob. 26E Ch. 2.2 - Prob. 27E Ch. 2.2 - Prob. 28E Ch. 2.2 - Prob. 29E Ch. 2.2 - Prob. 30E Ch. 2.2 - Prob. 31E Ch. 2.2 - Prob. 32E Ch. 2.2 - Prob. 33E Ch. 2.2 - Prob. 34E Ch. 2.2 - Prob. 35E Ch. 2.2 - Prob. 36E Ch. 2.2 - Prob. 37E Ch. 2.2 - Prob. 38E Ch. 2.2 - Prob. 39E Ch. 2.2 - Prob. 40E Ch. 2.2 - Prob. 41E Ch. 2.2 - Prob. 42E Ch. 2.2 - Prob. 43E Ch. 2.2 - Prob. 44E Ch. 2.2 - Prob. 45E Ch. 2.2 - Prob. 46E Ch. 2.2 - Prob. 47E Ch. 2.2 - Prob. 48E Ch. 2.2 - Prob. 49E Ch. 2.2 - Prob. 50E Ch. 2.2 - Prob. 51E Ch. 2.2 - Prob. 52E Ch. 2.2 - Prob. 53E Ch. 2.2 - Prob. 54E Ch. 2.2 - Prob. 55E Ch. 2.2 - Prob. 56E Ch. 2.2 - Prob. 57E Ch. 2.2 - Prob. 58E Ch. 2.2 - Prob. 59E Ch. 2.2 - Prob. 60E Ch. 2.2 - Prob. 61E Ch. 2.2 - Prob. 62E Ch. 2.2 - Prob. 63E Ch. 2.2 - Prob. 64E Ch. 2.2 - Prob. 65E Ch. 2.2 - Prob. 66E Ch. 2.2 - Prob. 67E Ch. 2.2 - Prob. 68E Ch. 2.2 - Prob. 69E Ch. 2.2 - Prob. 70E Ch. 2.2 - Prob. 71E Ch. 2.2 - Prob. 72E Ch. 2.2 - Prob. 73E Ch. 2.2 - Prob. 74E Ch. 2.2 - Prob. 75E Ch. 2.2 - Prob. 76E Ch. 2.2 - Prob. 77E Ch. 2.2 - Prob. 78E Ch. 2.2 - Weather Balloon As a weather balloon is inflated,...Ch. 2.2 - Power from a Wind Turbine The power produced by a...Ch. 2.2 - Utility Rates Westside Energy charges its electric...Ch. 2.2 - Taxicab Function A taxi company charges 2.00 for...Ch. 2.2 - Prob. 83E Ch. 2.2 - Prob. 84E Ch. 2.2 - Prob. 85E Ch. 2.2 - Prob. 86E Ch. 2.2 - Prob. 87E Ch. 2.3 - Prob. 1E Ch. 2.3 - Prob. 2E Ch. 2.3 - Prob. 3E Ch. 2.3 - Prob. 4E Ch. 2.3 - Prob. 5E Ch. 2.3 - Prob. 6E Ch. 2.3 - Prob. 7E Ch. 2.3 - Prob. 8E Ch. 2.3 - Prob. 9E Ch. 2.3 - Prob. 10E Ch. 2.3 - Prob. 11E Ch. 2.3 - Prob. 12E Ch. 2.3 - Prob. 13E Ch. 2.3 - Prob. 14E Ch. 2.3 - Prob. 15E Ch. 2.3 - Prob. 16E Ch. 2.3 - Prob. 17E Ch. 2.3 - Prob. 18E Ch. 2.3 - Prob. 19E Ch. 2.3 - Prob. 20E Ch. 2.3 - Prob. 21E Ch. 2.3 - Prob. 22E Ch. 2.3 - Prob. 23E Ch. 2.3 - Prob. 24E Ch. 2.3 - Prob. 25E Ch. 2.3 - Prob. 26E Ch. 2.3 - Prob. 27E Ch. 2.3 - Prob. 28E Ch. 2.3 - Prob. 29E Ch. 2.3 - Prob. 30E Ch. 2.3 - Prob. 31E Ch. 2.3 - Prob. 32E Ch. 2.3 - Prob. 33E Ch. 2.3 - Prob. 34E Ch. 2.3 - Prob. 35E Ch. 2.3 - Prob. 36E Ch. 2.3 - Prob. 37E Ch. 2.3 - Prob. 38E Ch. 2.3 - Prob. 39E Ch. 2.3 - Prob. 40E Ch. 2.3 - Prob. 41E Ch. 2.3 - Prob. 42E Ch. 2.3 - Prob. 43E Ch. 2.3 - Earthquake The graph shows the vertical...Ch. 2.3 - Prob. 45E Ch. 2.3 - Prob. 46E Ch. 2.3 - Prob. 47E Ch. 2.3 - Prob. 48E Ch. 2.3 - Hurdle Race Three runners compete in a 100-meter...Ch. 2.3 - Gravity Near the Moon We can use Newtons Law of...Ch. 2.3 - Prob. 51E Ch. 2.3 - Migrating Fish A fish swims at a speed v relative...Ch. 2.3 - Prob. 53E Ch. 2.3 - Prob. 54E Ch. 2.3 - Coughing When a foreign object that is lodged in...Ch. 2.3 - Prob. 56E Ch. 2.3 - Prob. 57E Ch. 2.3 - Prob. 58E Ch. 2.4 - If you travel 100 miles in two hours, then your...Ch. 2.4 - The average rate of change of a function f between...Ch. 2.4 - The average rate of change of the function f(x) =...Ch. 2.4 - (a) The average rate of change of a function f...Ch. 2.4 - Prob. 5E Ch. 2.4 - Prob. 6E Ch. 2.4 - Prob. 7E Ch. 2.4 - Prob. 8E Ch. 2.4 - Prob. 9E Ch. 2.4 - Prob. 10E Ch. 2.4 - Prob. 11E Ch. 2.4 - Prob. 12E Ch. 2.4 - Prob. 13E Ch. 2.4 - Prob. 14E Ch. 2.4 - Prob. 15E Ch. 2.4 - Prob. 16E Ch. 2.4 - Prob. 17E Ch. 2.4 - Prob. 18E Ch. 2.4 - Prob. 19E Ch. 2.4 - Prob. 20E Ch. 2.4 - Prob. 21E Ch. 2.4 - Prob. 22E Ch. 2.4 - Changing Water Levels The graph shows the depth of...Ch. 2.4 - Population Growth and Decline The graph shows the...Ch. 2.4 - Population Growth and Decline The table gives the...Ch. 2.4 - Running Speed A man is running around a circular...Ch. 2.4 - Prob. 27E Ch. 2.4 - Book Collection Between 1980 and 2000 a rare book...Ch. 2.4 - Cooling Soup When a bowl of hot soup is left in a...Ch. 2.4 - Farms in the United States The graph gives the...Ch. 2.4 - Prob. 31E Ch. 2.4 - Prob. 32E Ch. 2.4 - Prob. 33E Ch. 2.5 - Fill in the blank with the appropriate direction...Ch. 2.5 - Prob. 2E Ch. 2.5 - Prob. 3E Ch. 2.5 - Prob. 4E Ch. 2.5 - Prob. 5E Ch. 2.5 - Prob. 6E Ch. 2.5 - Prob. 7E Ch. 2.5 - Prob. 8E Ch. 2.5 - Prob. 9E Ch. 2.5 - Prob. 10E Ch. 2.5 - Prob. 11E Ch. 2.5 - Prob. 12E Ch. 2.5 - Prob. 13E Ch. 2.5 - Prob. 14E Ch. 2.5 - Prob. 15E Ch. 2.5 - Prob. 16E Ch. 2.5 - Prob. 17E Ch. 2.5 - Prob. 18E Ch. 2.5 - Prob. 19E Ch. 2.5 - Prob. 20E Ch. 2.5 - Prob. 21E Ch. 2.5 - Prob. 22E Ch. 2.5 - Prob. 23E Ch. 2.5 - Prob. 24E Ch. 2.5 - Prob. 25E Ch. 2.5 - Prob. 26E Ch. 2.5 - Prob. 27E Ch. 2.5 - Prob. 28E Ch. 2.5 - Prob. 29E Ch. 2.5 - Prob. 30E Ch. 2.5 - Prob. 31E Ch. 2.5 - Prob. 32E Ch. 2.5 - Prob. 33E Ch. 2.5 - Prob. 34E Ch. 2.5 - Prob. 35E Ch. 2.5 - Prob. 36E Ch. 2.5 - Prob. 37E Ch. 2.5 - Prob. 38E Ch. 2.5 - Prob. 39E Ch. 2.5 - Prob. 40E Ch. 2.5 - Prob. 41E Ch. 2.5 - Prob. 42E Ch. 2.5 - Prob. 43E Ch. 2.5 - Prob. 44E Ch. 2.5 - Prob. 45E Ch. 2.5 - Prob. 46E Ch. 2.5 - Prob. 47E Ch. 2.5 - Prob. 48E Ch. 2.5 - Prob. 49E Ch. 2.5 - Prob. 50E Ch. 2.5 - Prob. 51E Ch. 2.5 - Prob. 52E Ch. 2.5 - Prob. 53E Ch. 2.5 - Prob. 54E Ch. 2.5 - Prob. 55E Ch. 2.5 - Prob. 56E Ch. 2.5 - Prob. 57E Ch. 2.5 - Prob. 58E Ch. 2.5 - Prob. 59E Ch. 2.5 - Prob. 60E Ch. 2.5 - Prob. 61E Ch. 2.5 - Prob. 62E Ch. 2.5 - Prob. 63E Ch. 2.5 - Prob. 64E Ch. 2.5 - Prob. 65E Ch. 2.5 - Prob. 66E Ch. 2.5 - Prob. 67E Ch. 2.5 - Prob. 68E Ch. 2.5 - Prob. 69E Ch. 2.5 - Prob. 70E Ch. 2.5 - Prob. 71E Ch. 2.5 - Prob. 72E Ch. 2.5 - Prob. 73E Ch. 2.5 - Prob. 74E Ch. 2.5 - Prob. 75E Ch. 2.5 - Prob. 76E Ch. 2.5 - Prob. 77E Ch. 2.5 - Prob. 78E Ch. 2.5 - Prob. 79E Ch. 2.5 - Prob. 80E Ch. 2.5 - Prob. 81E Ch. 2.5 - Prob. 82E Ch. 2.5 - Prob. 83E Ch. 2.5 - Prob. 84E Ch. 2.5 - Prob. 85E Ch. 2.5 - Prob. 86E Ch. 2.5 - Prob. 87E Ch. 2.5 - Prob. 88E Ch. 2.5 - Prob. 89E Ch. 2.5 - Prob. 90E Ch. 2.5 - Prob. 91E Ch. 2.5 - Prob. 92E Ch. 2.5 - Prob. 93E Ch. 2.6 - From the graphs of f and g in the figure, we find...Ch. 2.6 - By definition, (f g)(x) = _____. So if g(2) = 5...Ch. 2.6 - If the rule of the function f is add one and the...Ch. 2.6 - We can express the functions in Exercise 3...Ch. 2.6 - Prob. 5E Ch. 2.6 - Prob. 6E Ch. 2.6 - Prob. 7E Ch. 2.6 - Prob. 8E Ch. 2.6 - Prob. 9E Ch. 2.6 - Prob. 10E Ch. 2.6 - Prob. 11E Ch. 2.6 - Prob. 12E Ch. 2.6 - Prob. 13E Ch. 2.6 - Prob. 14E Ch. 2.6 - Prob. 15E Ch. 2.6 - Prob. 16E Ch. 2.6 - Prob. 17E Ch. 2.6 - Prob. 18E Ch. 2.6 - Prob. 19E Ch. 2.6 - Prob. 20E Ch. 2.6 - Prob. 21E Ch. 2.6 - Prob. 22E Ch. 2.6 - Prob. 23E Ch. 2.6 - Prob. 24E Ch. 2.6 - Prob. 25E Ch. 2.6 - Prob. 26E Ch. 2.6 - Prob. 27E Ch. 2.6 - Prob. 28E Ch. 2.6 - Prob. 29E Ch. 2.6 - Prob. 30E Ch. 2.6 - Prob. 31E Ch. 2.6 - Prob. 32E Ch. 2.6 - Prob. 33E Ch. 2.6 - Prob. 34E Ch. 2.6 - Prob. 35E Ch. 2.6 - Prob. 36E Ch. 2.6 - Prob. 37E Ch. 2.6 - Prob. 38E Ch. 2.6 - Prob. 39E Ch. 2.6 - Prob. 40E Ch. 2.6 - Prob. 41E Ch. 2.6 - Prob. 42E Ch. 2.6 - Prob. 43E Ch. 2.6 - Prob. 44E Ch. 2.6 - Prob. 45E Ch. 2.6 - Prob. 46E Ch. 2.6 - Prob. 47E Ch. 2.6 - Prob. 48E Ch. 2.6 - Prob. 49E Ch. 2.6 - Prob. 50E Ch. 2.6 - Prob. 51E Ch. 2.6 - Prob. 52E Ch. 2.6 - Prob. 53E Ch. 2.6 - Prob. 54E Ch. 2.6 - Prob. 55E Ch. 2.6 - Prob. 56E Ch. 2.6 - Prob. 57E Ch. 2.6 - Prob. 58E Ch. 2.6 - Prob. 59E Ch. 2.6 - Revenue, Cost, and Profit A print shop makes...Ch. 2.6 - Area of a Ripple A stone is dropped in a lake,...Ch. 2.6 - Inflating a Balloon A spherical balloon is being...Ch. 2.6 - Area of a Balloon A spherical weather balloon is...Ch. 2.6 - Multiple Discounts You have a 50 coupon from the...Ch. 2.6 - Multiple Discounts An appliance dealer advertises...Ch. 2.6 - Airplane Trajectory An airplane is flying at a...Ch. 2.6 - Prob. 67E Ch. 2.6 - Prob. 68E Ch. 2.6 - Prob. 69E Ch. 2.6 - Prob. 70E Ch. 2.7 - A function f is one-to-one if different inputs...Ch. 2.7 - (a) For a function to have an inverse, it must be...Ch. 2.7 - A function f has the following verbal description:...Ch. 2.7 - True or false? (a) If f has an inverse, then f1(x)...Ch. 2.7 - Prob. 5E Ch. 2.7 - Prob. 6E Ch. 2.7 - Prob. 7E Ch. 2.7 - Prob. 8E Ch. 2.7 - Prob. 9E Ch. 2.7 - Prob. 10E Ch. 2.7 - Prob. 11E Ch. 2.7 - Prob. 12E Ch. 2.7 - Prob. 13E Ch. 2.7 - Prob. 14E Ch. 2.7 - Prob. 15E Ch. 2.7 - Prob. 16E Ch. 2.7 - Prob. 17E Ch. 2.7 - Prob. 18E Ch. 2.7 - Prob. 19E Ch. 2.7 - Prob. 20E Ch. 2.7 - Prob. 21E Ch. 2.7 - Prob. 22E Ch. 2.7 - Prob. 23E Ch. 2.7 - Prob. 24E Ch. 2.7 - Prob. 25E Ch. 2.7 - Prob. 26E Ch. 2.7 - Prob. 27E Ch. 2.7 - Prob. 28E Ch. 2.7 - Prob. 29E Ch. 2.7 - Prob. 30E Ch. 2.7 - Prob. 31E Ch. 2.7 - Prob. 32E Ch. 2.7 - Prob. 33E Ch. 2.7 - Prob. 34E Ch. 2.7 - Prob. 35E Ch. 2.7 - Prob. 36E Ch. 2.7 - Prob. 37E Ch. 2.7 - Prob. 38E Ch. 2.7 - Prob. 39E Ch. 2.7 - Prob. 40E Ch. 2.7 - Prob. 41E Ch. 2.7 - Prob. 42E Ch. 2.7 - Prob. 43E Ch. 2.7 - Prob. 44E Ch. 2.7 - Prob. 45E Ch. 2.7 - Prob. 46E Ch. 2.7 - Prob. 47E Ch. 2.7 - Prob. 48E Ch. 2.7 - Prob. 49E Ch. 2.7 - Prob. 50E Ch. 2.7 - Prob. 51E Ch. 2.7 - Prob. 52E Ch. 2.7 - Prob. 53E Ch. 2.7 - Prob. 54E Ch. 2.7 - Prob. 55E Ch. 2.7 - Prob. 56E Ch. 2.7 - Prob. 57E Ch. 2.7 - Prob. 58E Ch. 2.7 - Prob. 59E Ch. 2.7 - Prob. 60E Ch. 2.7 - Prob. 61E Ch. 2.7 - Prob. 62E Ch. 2.7 - Prob. 63E Ch. 2.7 - Prob. 64E Ch. 2.7 - Prob. 65E Ch. 2.7 - Prob. 66E Ch. 2.7 - Prob. 67E Ch. 2.7 - Prob. 68E Ch. 2.7 - Prob. 69E Ch. 2.7 - Prob. 70E Ch. 2.7 - Prob. 71E Ch. 2.7 - Prob. 72E Ch. 2.7 - Prob. 73E Ch. 2.7 - Prob. 74E Ch. 2.7 - Prob. 75E Ch. 2.7 - Prob. 76E Ch. 2.7 - Prob. 77E Ch. 2.7 - Prob. 78E Ch. 2.7 - Prob. 79E Ch. 2.7 - Prob. 80E Ch. 2.7 - Fee for Service For his services, a private...Ch. 2.7 - Prob. 82E Ch. 2.7 - Prob. 83E Ch. 2.7 - Prob. 84E Ch. 2.7 - Prob. 85E Ch. 2.7 - Prob. 86E Ch. 2.7 - Income Tax In a certain country the tax on incomes...Ch. 2.7 - Prob. 88E Ch. 2.7 - Prob. 89E Ch. 2.7 - Prob. 90E Ch. 2.7 - Prob. 91E Ch. 2.7 - Prob. 92E Ch. 2.7 - Prob. 93E Ch. 2 - Prob. 1RCC Ch. 2 - Prob. 2RCC Ch. 2 - Prob. 3RCC Ch. 2 - Prob. 4RCC Ch. 2 - Prob. 5RCC Ch. 2 - Prob. 6RCC Ch. 2 - Prob. 7RCC Ch. 2 - Prob. 8RCC Ch. 2 - Prob. 9RCC Ch. 2 - Prob. 10RCC Ch. 2 - Prob. 11RCC Ch. 2 - Prob. 12RCC Ch. 2 - Prob. 1RE Ch. 2 - Prob. 2RE Ch. 2 - Prob. 3RE Ch. 2 - Prob. 4RE Ch. 2 - Prob. 5RE Ch. 2 - Prob. 6RE Ch. 2 - Prob. 7RE Ch. 2 - Prob. 8RE Ch. 2 - Prob. 9RE Ch. 2 - Prob. 10RE Ch. 2 - Prob. 11RE Ch. 2 - Prob. 12RE Ch. 2 - Prob. 13RE Ch. 2 - Prob. 14RE Ch. 2 - Prob. 15RE Ch. 2 - Prob. 16RE Ch. 2 - Prob. 17RE Ch. 2 - Prob. 18RE Ch. 2 - Prob. 19RE Ch. 2 - Prob. 20RE Ch. 2 - Prob. 21RE Ch. 2 - Prob. 22RE Ch. 2 - Prob. 23RE Ch. 2 - Prob. 24RE Ch. 2 - Prob. 25RE Ch. 2 - Prob. 26RE Ch. 2 - Prob. 27RE Ch. 2 - Prob. 28RE Ch. 2 - Prob. 29RE Ch. 2 - Prob. 30RE Ch. 2 - Prob. 31RE Ch. 2 - Prob. 32RE Ch. 2 - Prob. 33RE Ch. 2 - Prob. 34RE Ch. 2 - Prob. 35RE Ch. 2 - Prob. 36RE Ch. 2 - Prob. 37RE Ch. 2 - Prob. 38RE Ch. 2 - Prob. 39RE Ch. 2 - Prob. 40RE Ch. 2 - Prob. 41RE Ch. 2 - Prob. 42RE Ch. 2 - Prob. 43RE Ch. 2 - Prob. 44RE Ch. 2 - Prob. 45RE Ch. 2 - Prob. 46RE Ch. 2 - Prob. 47RE Ch. 2 - Prob. 48RE Ch. 2 - Prob. 49RE Ch. 2 - Prob. 50RE Ch. 2 - Prob. 51RE Ch. 2 - Prob. 52RE Ch. 2 - Prob. 53RE Ch. 2 - Prob. 54RE Ch. 2 - Prob. 55RE Ch. 2 - Prob. 56RE Ch. 2 - Prob. 57RE Ch. 2 - Prob. 58RE Ch. 2 - Prob. 59RE Ch. 2 - Prob. 60RE Ch. 2 - Prob. 61RE Ch. 2 - Prob. 62RE Ch. 2 - Prob. 63RE Ch. 2 - Prob. 64RE Ch. 2 - Prob. 65RE Ch. 2 - Prob. 66RE Ch. 2 - Prob. 67RE Ch. 2 - Prob. 68RE Ch. 2 - Prob. 69RE Ch. 2 - Prob. 70RE Ch. 2 - Prob. 71RE Ch. 2 - Prob. 72RE Ch. 2 - Prob. 73RE Ch. 2 - Prob. 74RE Ch. 2 - Prob. 75RE Ch. 2 - Prob. 76RE Ch. 2 - Prob. 77RE Ch. 2 - Prob. 78RE Ch. 2 - Prob. 79RE Ch. 2 - Prob. 80RE Ch. 2 - Prob. 81RE Ch. 2 - Prob. 82RE Ch. 2 - Prob. 83RE Ch. 2 - Prob. 84RE Ch. 2 - Prob. 85RE Ch. 2 - Prob. 86RE Ch. 2 - Prob. 87RE Ch. 2 - Prob. 88RE Ch. 2 - Prob. 89RE Ch. 2 - Prob. 90RE Ch. 2 - Prob. 91RE Ch. 2 - Prob. 92RE Ch. 2 - Prob. 1T Ch. 2 - Prob. 2T Ch. 2 - A function f has the following verbal description:...Ch. 2 - A school fund-raising group sells chocolate bars...Ch. 2 - Prob. 5T Ch. 2 - Prob. 6T Ch. 2 - Prob. 7T Ch. 2 - Prob. 8T Ch. 2 - Prob. 9T Ch. 2 - Prob. 10T Ch. 2 - Prob. 11T Ch. 2 - Prob. 12T Ch. 2 - Prob. 1P Ch. 2 - Prob. 2P Ch. 2 - Prob. 3P Ch. 2 - Prob. 4P Ch. 2 - Prob. 5P Ch. 2 - Prob. 6P Ch. 2 - Prob. 7P Ch. 2 - Prob. 8P Ch. 2 - Prob. 9P Ch. 2 - Prob. 10P Ch. 2 - Prob. 11P Ch. 2 - Length A woman 5 ft tall is standing near a street...Ch. 2 - Distance Two ships leave port at the same time....Ch. 2 - Prob. 14P Ch. 2 - Area An isosceles triangle has a perimeter of 8...Ch. 2 - Prob. 16P Ch. 2 - Area A rectangle is inscribed in a semicircle of...Ch. 2 - Height The volume of a cone is 100 in3. Find a...Ch. 2 - Prob. 19P Ch. 2 - Minimizing a Sum Find two positive numbers whose...Ch. 2 - Prob. 21P Ch. 2 - Dividing a Pen A rancher with 750 ft of fencing...Ch. 2 - Fencing a Garden Plot A property owner wants to...Ch. 2 - Maximizing Area A wire 10 cm long is cut into two...Ch. 2 - Light from a Window A Norman window has the shape...Ch. 2 - Volume of a Box A box with an open top is to be...Ch. 2 - Area of a Box An open box with a square base is to...Ch. 2 - Inscribed Rectangle Find the dimensions that give...Ch. 2 - Minimizing Costs A rancher wants to build a...Ch. 2 - Minimizing Time A man stands at a point A on the...Ch. 2 - Bird Flight A bird is released from point A on an...Ch. 2 - Area of a Kite A kite frame is to be made from six...

Knowledge Booster

$Calculus$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.