
Concept explainers
a.
To plot: The
Given information:
The atmospheric concentration of carbon dioxide (in parts per million) recorded by the observatory in years between 1960 and 2015.
| x | y |
| 1960 | 317 |
| 1970 | 326 |
| 1980 | 339 |
| 1990 | 354 |
| 2000 | 369 |
| 2005 | 380 |
| 2010 | 390 |
| 2015 | 401 |
Plot:
Take the year as x coordinate and concentration as y coordinate, and then draw the scatter plot as shown below:
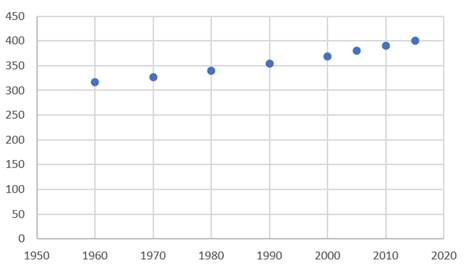
Interpretation:
From the scatter plot it seems that the linear regression will best fit the scenario.
b.
To find: If a linear or a quadratic model fit more appropriate.
A linear model seems more appropriate for the scatter plot.
Given information:
The atmospheric concentration of carbon dioxide (in parts per million) recorded by the observatory in years between 1960 and 2015.
| x | y |
| 1960 | 317 |
| 1970 | 326 |
| 1980 | 339 |
| 1990 | 354 |
| 2000 | 369 |
| 2005 | 380 |
| 2010 | 390 |
| 2015 | 401 |
Calculation:
Observe that the points do not fall nearly along a well-known curve. But, they do seem to fall near a line with upward slope.
Since the curve drawn seems to be a straight line, a linear model seems more appropriate for the scatter plot.
c.
To find: Find the regression equation for the appropriate model, and superimpose the graph of the regression equation on the scatter plot.
The regression can line by written as
Given information:
The atmospheric concentration of carbon dioxide (in parts per million) recorded by the observatory in years between 1960 and 2015.
| x | y |
| 1960 | 317 |
| 1970 | 326 |
| 1980 | 339 |
| 1990 | 354 |
| 2000 | 369 |
| 2005 | 380 |
| 2010 | 390 |
| 2015 | 401 |
Calculation:
Prepare the table to find the regression coefficients as follows;
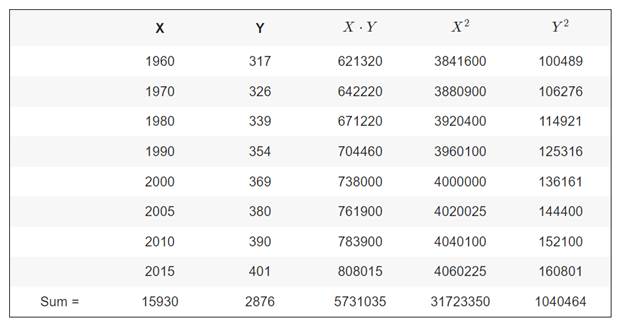
Use the table to perform the calculations as shown below.
Then,
Therefore,
So, the regression can line by written as
Now, plot the regression line as shown below.
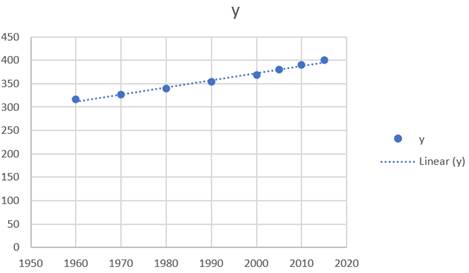
The graph of the regression line best fit the data. That means, the graph shows that the model appears to work well.
d.
To find: The approximate concentration level of carbon dioxide in the year 2025.
The concentration of carbon dioxide in the year 2025 will be
Given information:
The atmospheric concentration of carbon dioxide (in parts per million) recorded by the observatory in years between 1960 and 2015.
| x | y |
| 1960 | 317 |
| 1970 | 326 |
| 1980 | 339 |
| 1990 | 354 |
| 2000 | 369 |
| 2005 | 380 |
| 2010 | 390 |
| 2015 | 401 |
Calculation:
The model for the given situation is
Substitute
Conclusion:
The concentration of carbon dioxide in the year 2025 will be
e.
To explain: The circumstances that might caused the predication in part (d) of the mark and tell whether the true value will be higher or lower than the predicted value.
Given information:
The atmospheric concentration of carbon dioxide (in parts per million) recorded by the observatory in years between 1960 and 2015.
| x | y |
| 1960 | 317 |
| 1970 | 326 |
| 1980 | 339 |
| 1990 | 354 |
| 2000 | 369 |
| 2005 | 380 |
| 2010 | 390 |
| 2015 | 401 |
Explanation:
If the model is predicted to be an upward quadratic, then the prediction in part (d) would have been off the mark.
Since an upward quadratic function gives a higher value than a linear function with upward slope, the true value would have been lower than the predicated value.
Chapter 1 Solutions
Precalculus: Graphical, Numerical, Algebraic Common Core 10th Edition
- Use a graphing calculator to find where the curves intersect and to find the area between the curves. y=ex, y=-x²-4x a. The left point of intersection is (Type integers or decimals rounded to the nearest thousandth as needed. Type an ordered pair.)arrow_forwardFind the area between the curves. x= -5, x=3, y=2x² +9, y=0 The area between the curves is (Round to the nearest whole number as needed.)arrow_forwardcan you solve these questions with step by step with clear explaination pleasearrow_forward
- Find the area between the following curves. x=-1, x=3, y=x-1, and y=0 The area between the curves is (Simplify your answer.)arrow_forwardFind the area between the curves. x= − 2, x= 3, y=5x, y=x? - 6 6 The area between the curves is (Simplify your answer.) ...arrow_forwardplease question 9arrow_forward
- Use the definite integral to find the area between the x-axis and f(x) over the indicated interval. Check first to see if the graph crosses the x-axis in the given interval. 3. f(x) = 4x; [-5,3]arrow_forwardUse the definite integral to find the area between the x-axis and f(x) over the indicated interval. Check first to see if the graph crosses the x-axis in the given interval. f(x)=3e-4; [3,3]arrow_forwardA small company of science writers found that its rate of profit (in thousands of dollars) after t years of operation is given by P'(t) = (7t + 14) (t² + 4t+7) * (a) Find the total profit in the first four years. (b) Find the profit in the sixth year of operation. (c) What is happening to the annual profit over the long run?arrow_forward
- Calculus III May I have an expert explained how the terms were simplified into 6(3-x)^2? Thank you,arrow_forwardCalculus III May I have an expert explain how the integrand was simplified into the final for form to be integrated with respect to x? Thank you,arrow_forwardCalculus lll May I please have the semicolon statement in the box defined with explanation? Thank you,arrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





