VECTOR MECH. FOR EGR: STATS & DYNAM (LL
12th Edition
ISBN: 9781260663778
Author: BEER
Publisher: MCG
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 16.2, Problem 16.132P
A driver starts his car with the door on the passenger’s side wide open (θ = 0). The 100-lb door has a centroidal radius of gyration
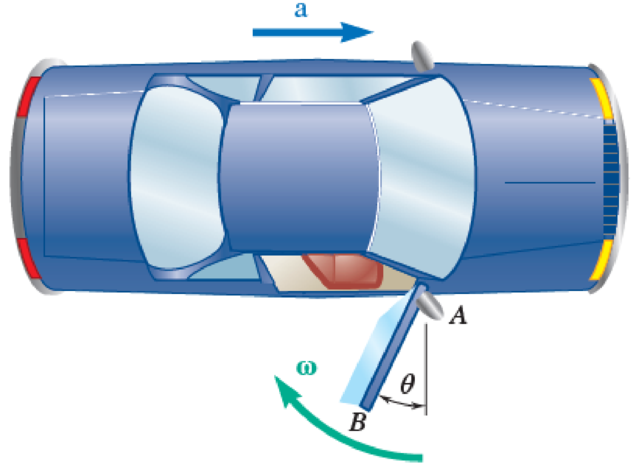
Fig. P16.132
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
In the helicopter shown; a vertical tail propeller is used to pre-
vent rotation of the cab as the speed of the main blades is
changed. Assuming that the tail propeller is not operating
determine the final angular velocity of the cab after the speed
of the main blades has been changed from I80 to 240 rpm. (The
speed of the main blades is measured relative to the cab, and
the cab has a centroidal moment of inertia of 650 lb.ft.s2.
Each of the four main blades is assumed to be a slender rod 14 ft weighing 55 lb.)
1. The gear shown has a mass of 10 kg and is mounted at
an angle of 10° to the rotating shaft which has a negligible
mass. (x, y, z) axes are aligned with the principal
directions of the gear and the moments of inertia with
respect to these axes have been measured as Izz =
0.1 kg-m², Ixx = Iyy = Izz/2. If the shaft is rotating with
a constant angular velocity of w = 30 rad/s, determine
the reaction forces at the two bearings at the instant
shown. Neglect gravity.
[ans. 71.6 N at A and 26.5 N at B, both vertical]
N
ACC
Xx
(
10°
10°
G
B
A 5.32-kg disk A of radius 0.445 m initially rotating counter-clockwise at 436 rev/min is engaged with a
6.72-kg disk B of radius 0.275 m initially rotating clockwise at 528 rev/min, where the moment of inertia of
a disk is given as I = ½ mi?. Determine their combined angular speed (in rpm) and direction of rotation
after the meshing of the two disks. Remember to show clearly the equations that you use!!'
Chapter 16 Solutions
VECTOR MECH. FOR EGR: STATS & DYNAM (LL
Ch. 16.1 - Two pendulums, A and B, with the masses and...Ch. 16.1 - Two pendulums, A and B, with the masses and...Ch. 16.1 - Two solid cylinders, A and B, have the same mass m...Ch. 16.1 - Prob. 16.1FBPCh. 16.1 - Prob. 16.2FBPCh. 16.1 - Prob. 16.3FBPCh. 16.1 - The 400-lb crate shown is lowered by means of two...Ch. 16.1 - A 60-lb uniform thin panel is placed in a truck...Ch. 16.1 - A 60-lb uniform thin panel is placed in a truck...Ch. 16.1 - A loading car is at rest on a track forming an...
Ch. 16.1 - A 2100-lb rear-wheel-drive tractor carries a 900...Ch. 16.1 - A uniform rod BC of mass 4 kg is connected to a...Ch. 16.1 - A 2000-kg truck is being used to lift a 400-kg...Ch. 16.1 - The support bracket shown is used to transport a...Ch. 16.1 - Prob. 16.8PCh. 16.1 - A 20-kg cabinet is mounted on casters that allow...Ch. 16.1 - Solve Prob. 16.9, assuming that the casters are...Ch. 16.1 - Prob. 16.11PCh. 16.1 - Prob. 16.12PCh. 16.1 - The retractable shelf shown is supported by two...Ch. 16.1 - Bars AB and BE, each with a mass of 4 kg, are...Ch. 16.1 - At the instant shown, the tensions in the vertical...Ch. 16.1 - Three bars, each of mass 3 kg, are welded together...Ch. 16.1 - Members ACE and DCB are each 600 mm long and are...Ch. 16.1 - A prototype rotating bicycle rack is designed to...Ch. 16.1 - The control rod AC is guided by two pins that...Ch. 16.1 - The coefficients of friction between the 30-lb...Ch. 16.1 - Prob. 16.21PCh. 16.1 - Prob. 16.22PCh. 16.1 - For a rigid body in translation, show that the...Ch. 16.1 - For a rigid body in centroidal rotation, show that...Ch. 16.1 - It takes 10 min for a 2.4-Mg flywheel to coast to...Ch. 16.1 - The rotor of an electric motor has an angular...Ch. 16.1 - The 10-in.-radius brake drum is attached to a...Ch. 16.1 - The 10-in.-radius brake drum is attached to a...Ch. 16.1 - The 100-mm-radius brake drum is attached to a...Ch. 16.1 - The 180-mm-radius disk is at rest when it is...Ch. 16.1 - Solve Prob. 16.30, assuming that the direction of...Ch. 16.1 - In order to determine the mass moment of inertia...Ch. 16.1 - The flywheel shown has a radius of 20 in., a...Ch. 16.1 - Each of the double pulleys shown has a mass moment...Ch. 16.1 - Two disks A and B, of mass mA = 2 kg and mB = 4...Ch. 16.1 - Two disks A and B, of mass mA = 2 kg and mB = 4...Ch. 16.1 - Gear A weighs 1 lb and has a radius of gyration of...Ch. 16.1 - The 25-lb double pulley shown is at rest and in...Ch. 16.1 - A belt of negligible mass passes between cylinders...Ch. 16.1 - Prob. 16.40PCh. 16.1 - Disk A has a mass of 6 kg and an initial angular...Ch. 16.1 - Prob. 16.42PCh. 16.1 - Disk A has a mass mA = 4 kg, a radius rA = 300 mm,...Ch. 16.1 - Disk B is at rest when it is brought into contact...Ch. 16.1 - Prob. 16.45PCh. 16.1 - Prob. 16.46PCh. 16.1 - For a rigid body in plane motion, show that the...Ch. 16.1 - A uniform slender rod AB rests on a frictionless...Ch. 16.1 - Prob. 16.49PCh. 16.1 - Prob. 16.50PCh. 16.1 - Prob. 16.51PCh. 16.1 - A 250-lb satellite has a radius of gyration of 24...Ch. 16.1 - A rectangular plate of mass 5 kg is suspended from...Ch. 16.1 - Prob. 16.54PCh. 16.1 - A drum with a 200-mm radius is attached to a disk...Ch. 16.1 - A drum with a 200-mm radius is attached to a disk...Ch. 16.1 - The 12-lb uniform disk shown has a radius of r =...Ch. 16.1 - Prob. 16.58PCh. 16.1 - Prob. 16.59PCh. 16.1 - 16.60 and 16.61The 400-lb crate shown is lowered...Ch. 16.1 - Prob. 16.61PCh. 16.1 - Two uniform cylinders, each of weight W = 14 lb...Ch. 16.1 - Prob. 16.63PCh. 16.1 - Prob. 16.64PCh. 16.1 - A uniform slender bar AB with a mass m is...Ch. 16.1 - Prob. 16.66PCh. 16.1 - 16.66 through 16.68A thin plate of the shape...Ch. 16.1 - 16.66 through 16.68A thin plate of the shape...Ch. 16.1 - A sphere of radius r and mass m is projected along...Ch. 16.1 - Solve Prob. 16.69, assuming that the sphere is...Ch. 16.1 - A bowler projects an 8-in.-diameter ball weighing...Ch. 16.1 - Prob. 16.72PCh. 16.1 - A uniform sphere of radius r and mass m is placed...Ch. 16.1 - A sphere of radius r and mass m has a linear...Ch. 16.2 - A cord is attached to a spool when a force P is...Ch. 16.2 - Prob. 16.5CQCh. 16.2 - Prob. 16.6CQCh. 16.2 - Prob. 16.7CQCh. 16.2 - Prob. 16.5FBPCh. 16.2 - Two identical 4-lb slender rods AB and BC are...Ch. 16.2 - Prob. 16.7FBPCh. 16.2 - Prob. 16.8FBPCh. 16.2 - Show that the couple I of Fig. 16.15 can be...Ch. 16.2 - A uniform slender rod of length L = 900 mm and...Ch. 16.2 - A crate of mass 80 kg is held in the position...Ch. 16.2 - A uniform slender rod of length L = 36 in. and...Ch. 16.2 - In Prob. 16.78, determine (a) the distance h for...Ch. 16.2 - An athlete performs a leg extension on a machine...Ch. 16.2 - Prob. 16.81PCh. 16.2 - A turbine disk weighing 50 lb rotates at a...Ch. 16.2 - The 80-lb tailgate of a car is supported by the...Ch. 16.2 - A uniform rod of length L and mass m is supported...Ch. 16.2 - Three stage lights are mounted on a pipe fixture...Ch. 16.2 - An adapted launcher uses a torsional spring about...Ch. 16.2 - A 4-kg slender rod is welded to the edge of a 3-kg...Ch. 16.2 - Prob. 16.88PCh. 16.2 - The object ABC consists of two slender rods welded...Ch. 16.2 - A 3.5-kg slender rod AB and a 2-kg slender rod BC...Ch. 16.2 - A 9-kg uniform disk is attached to the 5-kg...Ch. 16.2 - Derive the equation MC=IC for the rolling disk of...Ch. 16.2 - Prob. 16.93PCh. 16.2 - Prob. 16.94PCh. 16.2 - Prob. 16.95PCh. 16.2 - Prob. 16.96PCh. 16.2 - A 40-kg flywheel of radius R = 0.5 m is rigidly...Ch. 16.2 - Prob. 16.98PCh. 16.2 - 16.98 through 16.101A drum of 80-mm radius is...Ch. 16.2 - 16.98 through 16.101A drum of 80-mm radius is...Ch. 16.2 - 16.98 through 16.101A drum of 80-mm radius is...Ch. 16.2 - 16.102 through 16.105A drum of 4-in. radius is...Ch. 16.2 - 16.102 through 16.105A drum of 4-in. radius is...Ch. 16.2 - 16.102 through 16.105A drum of 4-in. radius is...Ch. 16.2 - 16.102 through 16.105A drum of 4-in. radius is...Ch. 16.2 - 16.106 and 16.107A 12-in.-radius cylinder of...Ch. 16.2 - 16.106 and 16.107A 12-in.-radius cylinder of...Ch. 16.2 - Gear C has a mass of 5 kg and a centroidal radius...Ch. 16.2 - Two uniform disks A and B, each with a mass of 2...Ch. 16.2 - A single-axis personal transport device starts...Ch. 16.2 - A hemisphere of weight W and radius r is released...Ch. 16.2 - A hemisphere of weight W and radius r is released...Ch. 16.2 - The center of gravity G of a 1.5-kg unbalanced...Ch. 16.2 - A small clamp of mass mB is attached at B to a...Ch. 16.2 - Prob. 16.115PCh. 16.2 - A 4-lb bar is attached to a 10-lb uniform cylinder...Ch. 16.2 - The uniform rod AB with a mass m and a length of...Ch. 16.2 - Prob. 16.118PCh. 16.2 - Prob. 16.119PCh. 16.2 - Prob. 16.120PCh. 16.2 - End A of the 6-kg uniform rod AB rests on the...Ch. 16.2 - End A of the 6-kg uniform rod AB rests on the...Ch. 16.2 - End A of the 8-kg uniform rod AB is attached to a...Ch. 16.2 - The 4-kg uniform rod ABD is attached to the crank...Ch. 16.2 - The 3-lb uniform rod BD is connected to crank AB...Ch. 16.2 - The 3-lb uniform rod BD is connected to crank AB...Ch. 16.2 - The test rig shown was developed to perform...Ch. 16.2 - Solve Prob. 16.127 for = 90. 16.127The test rig...Ch. 16.2 - The 4-kg uniform slender bar BD is attached to bar...Ch. 16.2 - The motion of the uniform slender rod of length L...Ch. 16.2 - At the instant shown, the 20-ft-long, uniform...Ch. 16.2 - A driver starts his car with the door on the...Ch. 16.2 - Prob. 16.133PCh. 16.2 - The hatchback of a car is positioned as shown to...Ch. 16.2 - The 6-kg rod BC connects a 10-kg disk centered at...Ch. 16.2 - Prob. 16.136PCh. 16.2 - In the engine system shown, l = 250 mm and b = 100...Ch. 16.2 - Solve Prob. 16.137 when = 90. 16.137In the engine...Ch. 16.2 - The 4-lb uniform slender rod AB, the 8-lb uniform...Ch. 16.2 - The 4-lb uniform slender rod AB, the 8-lb uniform...Ch. 16.2 - Two rotating rods in the vertical plane are...Ch. 16.2 - Two rotating rods in the vertical plane are...Ch. 16.2 - Two disks, each with a mass m and a radius r, are...Ch. 16.2 - A uniform slender bar AB of mass m is suspended as...Ch. 16.2 - A uniform rod AB, of mass 15 kg and length 1 m, is...Ch. 16.2 - The uniform slender 2-kg bar BD is attached to the...Ch. 16.2 - Prob. 16.147PCh. 16.2 - Prob. 16.148PCh. 16.2 - Prob. 16.149PCh. 16.2 - Prob. 16.150PCh. 16.2 - (a) Determine the magnitude and the location of...Ch. 16.2 - Prob. 16.152PCh. 16 - A cyclist is riding a bicycle at a speed of 20 mph...Ch. 16 - The forklift truck shown weighs 3200 lb and is...Ch. 16 - The total mass of the Baja car and driver,...Ch. 16 - Identical cylinders of mass m and radius r are...Ch. 16 - Prob. 16.157RPCh. 16 - The uniform rod AB of weight W is released from...Ch. 16 - Prob. 16.159RPCh. 16 - Prob. 16.160RPCh. 16 - A cylinder with a circular hole is rolling without...Ch. 16 - Two 3-kg uniform bars are connected to form the...Ch. 16 - A crate of mass 80 kg is held in the position...Ch. 16 - The Geneva mechanism shown is used to provide an...
Additional Engineering Textbook Solutions
Find more solutions based on key concepts
CONCEPT QUESTIONS
15.CQ3 The ball rolls without slipping on the fixed surface as shown. What is the direction ...
Vector Mechanics for Engineers: Statics and Dynamics
The triple jump is a track-and-field event in which an athlete gets a running start and tries to leap as far as...
Vector Mechanics For Engineers
A biological fluid moves at a flow rate of m=0.02kg/s through a coiled, thin-walled, 5-mm-diameter tube submerg...
Fundamentals of Heat and Mass Transfer
What is the importance of modeling in engineering? How are the mathematical models for engineering processes pr...
HEAT+MASS TRANSFER:FUND.+APPL.
Find the change in length of side AB.
Mechanics of Materials, 7th Edition
23.23 A highly oxidized and uneven round bar is being turned on a lathe. Would you recommend a small or a large...
Manufacturing Engineering & Technology
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- 3. An individual does leg curl exercise to strengthen his hamstring muscles. The 3 kg load is located 36 cm from the axis of rotation (the knee joint). The leg weight is 5 kg and the center of mass of the leg is located 20 cm from the knee joint. The radius of gyration about the center of mass of the leg is 14 cm. At the instant shown, the leg is in a horizontal position and is moving counterclockwise with an angular acceleration of a = +4 rad/s² and an angular velocity of w = +3 rad/s² The hamstring muscle inserts 3 cm from the knee joint and is oriented at an angle of 60° with respect to the leg. Treat the 3 kg load as a point mass. Calculate the following: (a) the magnitude of Fm required to cause this motion. (b) the magnitudes of both the tangential and centripetal accelerations of the load. Knee pivot Hamstring muscle Perpendicular distance to pivot Weight force (load) Fm 36 cm 60 knees 5 kg 3 cm 3 kg Answers: a) 890.46 N; b) tangential: 1.44 m/s²; centripetal: 3.24 m/s²arrow_forward4. Each of the gears A and B has a mass of 2.4 kg and a radius of gyration of 60 mm, while gear C has a mass of 12 kg and a radius of gyration of 150 mm. A couple M of constant magnitude 10 Nm is applied to gear C. Determine (a) the number of revolutions of gear C required for its angular velocity to increase from 100 to 450 rpm, (b) the corresponding tangential force acting on gear A. В S0 mm, S0 mm 200 mm. Marrow_forwardProblem (1) Gears A and B each have a mass of 4 kg and a radius of gyration of 75 mm about their centers, while gear C has a mass of 15 kg and a radius of gyration of 180 mm about its center. A couple moment M = (0.20) N-m is applied to gear C. Determine the number of revolutions gears A and B experience if gear C increases its angular velocity from 25 rpm to 500 rpm. B 80 mm S0 mm 200 mmarrow_forward
- L A collar with a mass of 2 kg is rigidly d attached at a distance d = 300 mm from the end of a uniform slender rod AB. The rod has a mass of 5 kg and is of length L = 400 mm. Knowing that the rod is released from rest in the position shown, Using the conservation of Energy principle, determine the angular velocity of the rod after it has rotated through 60°. Neglect the moment of inertia of the A В collar C.arrow_forwardThe steel roll shown has a mass of 1230 kg, a centroidal radius of gyration of 150 mm, and is lifted by two cables looped around its shaft. TA TB A B TA TB A В 100 mm Knowing that for each cable TA= 3100 N and Tg= 3300 N, determine the acceleration of its mass center. m/s2 1. The acceleration of the mass center isarrow_forwardA turbine rotor is found to be out of balance to the extent of 1.5 kg at 0.45 m radius in the plane AA and 2kg at 0.6 m radius in the plane BB, the relative angular positions being given in the end view. It is desired to balance these masses by a mass in each of the planes XX and YY at radii of 0.525m and 0.45m respectively. Determine the magnitude and positions of these masses and show their positions in an end view. (Answer: X, 1.42kg, 209.27 degrees from A; y, 2.12KG, 329.1 degrees from A)arrow_forward
- A 1.6-kg tube AB can slide freely on rod DE which in turn can rotate freely in a horizontal plane. Initially the assembly is rotating with an angular velocity of magnitude w = 5 rad/s and the tube is held in position by a cord. The moment of inertia of the rod and bracket about the vertical axis of rotation is 0.30 kg.m2 and the centroidal moment of inertia of the tube about a vertical axis is 0.0025 kg.m2If the cord suddenly breaks, determine (a) the angular velocity of the assembly after the tube has moved to end E, (b) the energy lost during the plastic impact at E.arrow_forwardA rotating shaft carries four unbalanced masses 20 kg, 16 kg, 18 kg and 14 kg at radii 55 mm, 65 mm, 75 mm and 65 mm respectively. The 2nd, 3rd and 4th masses revolve in planes 80 mm, 160 mm and 280 mm respectively measured from the plane of the first mass and are angularly located at 65°, 135° and 270° respectively measured clockwise from the first mass.The shaft is dynamically balanced by two masses, both located at 55 mm radii and revolving in planes mid-way between those of 1st and 2nd masses and midway between those of 3rd and 4th masses. Determine, balancing mass by drawing couple polygon and their respective angular position graphically.arrow_forwardThe 100-kg projectile shown has a radius of gyration of 100 mm about its axis of symmetry Gx and a radius of gyration of 250 mm about the transverse axis Gy. Its angular velocity v can be resolved into two components; one component, directed along Gx, measures the rate of spin of the projectile, while the other component, directed along GD, measures its rate of precession. Knowing that θ= 6° and that the angular momentum of the projectile about its mass center G is determine (a) the rate of spin, (b) ) the rate of precession.arrow_forward
- A shaft with 3 meters span between two bearings carries two masses of 120 g and 100 g acting at the extremities of the arms 40 mm and 50 mm long respectively. The planes in which these masses rotate are 1.5 m and 2.5 m respectively from the left end bearing supporting the shaft. The angle between the arms is 120°. The speed of rotation of the shaft is 200 r.p.m. If the masses are balanced by two counter-masses rotating with the shaft acting at radii of 0.3 m and placed at 0.5 m from each bearing centers, estimate the magnitude of the two balance masses and their orientation with respect to the X-axis, i.e. mass of 120 g.arrow_forwardLocate mass A at 35 mm from support X. 4.4. Locate mass B at 96.5 mm from support X. 4.5. Locate mass C at 122.7 mm from support X. 4.6. Position mass A at zero degrees and D at 90o from A. 4.7. Rotate the shaft by hand and release it. As the shaft is released masses should remain in any angular position. 4.8. Change the angular position of masses B and D till the shaft is statically balanced. 4.9. Rotate the shaft with the motor at high speed and notice the reaction of the system. High vibration and noise indicate that the system is out of balance. 4.10. Change the position of the mass D till the system is dynamically balanced.arrow_forwardThe rotor of an electric motor has an angular velocity of 3600 rpm when the load and power are cut off. The 50-kg rotor then coasts to rest after 5000 revolutions. Knowing that the kinetic friction of the rotor produces a couple of magnitude 4 N - m, determine the centroidal radius of gyration of the rotor (I = mk ). (Answer: k = 188.1mm)arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY
Mechanical SPRING DESIGN Strategy and Restrictions in Under 15 Minutes!; Author: Less Boring Lectures;https://www.youtube.com/watch?v=dsWQrzfQt3s;License: Standard Youtube License