
(a)
To find: The range of the first eighteen winter Olympic games.
(a)
Answer to Problem 11E
The range is
Explanation of Solution
Given:
The number of nations:
Concept used:
Calculation:
According to the given in Ascending order is:
To calculate the range of a set of data, subtract the lowest value from highest value.
Hence, the range is
(b)
To find: the appropriate class intervals of first eighteen winter Olympic games.
(b)
Answer to Problem 11E
Class interval is
Explanation of Solution
Given:
The number of nations:
Concept used:
Calculation:
According to the given in Ascending order is:
Class interval can be found by:
Hence, class interval is
(c)
To find: the class limit of a first eighteen winter Olympic games.
(c)
Answer to Problem 11E
Class limits are
Explanation of Solution
Given:
The number of nations:
Concept used:
The class limit are lower limit and upper limit.
Intervals of the class is always same.
Calculation:
According to the given in Ascending order is:
The class limit are lower limit and upper limit of the class size since, the class size is
Hence, the class limits are
(d)
To find: The class marks of first eighteen winter Olympic games.
(d)
Answer to Problem 11E
Class marks are
Explanation of Solution
Given:
The number of nations:
Concept used:
Class marks are the mid value or mid-point of every interval.
Calculation:
According to the given in Ascending order is:
Class marks are the mid value or mid-point of every interval.
Since, class limits are:
For the first interval the class marks:
Similarly, it can be used to find the class mark respective class intervals or add
Hence, class marks are
(e)
To construct: the frequency distribution table for the first eighteen winter Olympic games.
(e)
Answer to Problem 11E
From the frequency distribution tables the frequency can be calculated and total frequency is
Explanation of Solution
Given:
The number of nations:
Concept used:
The frequency distribution table consist of frequency and tally marks which can easily measure the range class limits class size by looking at their respective blocks.
Calculation:
According to the given in Ascending order is:
(f)
To graph: the histogram from the first eighteen winter Olympic games.
(f)
Answer to Problem 11E
From histogram its more cleared to mention the maximum and minimum frequency through which range can be solved.
Explanation of Solution
Given:
The number of nations:
Concept used:
The histogram chart can be draw through Microsoft excel and this histogram chart can give so many information regarding the data.
The histogram is most commonly used graph to show frequency distributions
The purpose of histogram is to graphically summarize the distribution of a univariate data set.
Calculation:
According to the given in Ascending order is:
The histogram chart:
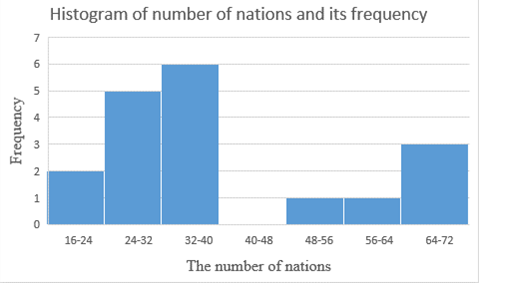
Hence, from histogram its moreclear to mention the maximum and minimum frequency through which range can be solved.
Chapter 14 Solutions
Advanced Mathematical Concepts: Precalculus with Applications, Student Edition
Additional Math Textbook Solutions
Algebra and Trigonometry (6th Edition)
A First Course in Probability (10th Edition)
Pre-Algebra Student Edition
Thinking Mathematically (6th Edition)
Basic Business Statistics, Student Value Edition
- nd ave a ction and ave an 48. The domain of f y=f'(x) x 1 2 (= x<0 x<0 = f(x) possible. Group Activity In Exercises 49 and 50, do the following. (a) Find the absolute extrema of f and where they occur. (b) Find any points of inflection. (c) Sketch a possible graph of f. 49. f is continuous on [0,3] and satisfies the following. X 0 1 2 3 f 0 2 0 -2 f' 3 0 does not exist -3 f" 0 -1 does not exist 0 ve tes where X 0 < x <1 1< x <2 2arrow_forwardNumerically estimate the value of limx→2+x3−83x−9, rounded correctly to one decimal place. In the provided table below, you must enter your answers rounded exactly to the correct number of decimals, based on the Numerical Conventions for MATH1044 (see lecture notes 1.3 Actions page 3). If there are more rows provided in the table than you need, enter NA for those output values in the table that should not be used. x→2+ x3−83x−9 2.1 2.01 2.001 2.0001 2.00001 2.000001arrow_forwardFind the general solution of the given differential equation. (1+x)dy/dx - xy = x +x2arrow_forwardEstimate the instantaneous rate of change of the function f(x) = 2x² - 3x − 4 at x = -2 using the average rate of change over successively smaller intervals.arrow_forwardGiven the graph of f(x) below. Determine the average rate of change of f(x) from x = 1 to x = 6. Give your answer as a simplified fraction if necessary. For example, if you found that msec = 1, you would enter 1. 3' −2] 3 -5 -6 2 3 4 5 6 7 Ꮖarrow_forwardGiven the graph of f(x) below. Determine the average rate of change of f(x) from x = -2 to x = 2. Give your answer as a simplified fraction if necessary. For example, if you found that msec = , you would enter 3 2 2 3 X 23arrow_forwardA function is defined on the interval (-π/2,π/2) by this multipart rule: if -π/2 < x < 0 f(x) = a if x=0 31-tan x +31-cot x if 0 < x < π/2 Here, a and b are constants. Find a and b so that the function f(x) is continuous at x=0. a= b= 3arrow_forwardUse the definition of continuity and the properties of limits to show that the function is continuous at the given number a. f(x) = (x + 4x4) 5, a = -1 lim f(x) X--1 = lim x+4x X--1 lim X-1 4 x+4x 5 ))" 5 )) by the power law by the sum law lim (x) + lim X--1 4 4x X-1 -(0,00+( Find f(-1). f(-1)=243 lim (x) + -1 +4 35 4 ([ ) lim (x4) 5 x-1 Thus, by the definition of continuity, f is continuous at a = -1. by the multiple constant law by the direct substitution propertyarrow_forward1. Compute Lo F⚫dr, where and C is defined by F(x, y) = (x² + y)i + (y − x)j r(t) = (12t)i + (1 − 4t + 4t²)j from the point (1, 1) to the origin.arrow_forward2. Consider the vector force: F(x, y, z) = 2xye²i + (x²e² + y)j + (x²ye² — z)k. (A) [80%] Show that F satisfies the conditions for a conservative vector field, and find a potential function (x, y, z) for F. Remark: To find o, you must use the method explained in the lecture. (B) [20%] Use the Fundamental Theorem for Line Integrals to compute the work done by F on an object moves along any path from (0,1,2) to (2, 1, -8).arrow_forwardhelp pleasearrow_forwardIn each of Problems 1 through 4, draw a direction field for the given differential equation. Based on the direction field, determine the behavior of y as t → ∞. If this behavior depends on the initial value of y at t = 0, describe the dependency.1. y′ = 3 − 2yarrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





