
(a)
The equation for the area A of the
(a)
Answer to Problem 43PPS
The equation is
Explanation of Solution
Given information:
The area A of a segment of a circle with a radius r and a central

Formula used:
Calculation:
Draw a perpendicular from the center to the chord to get two congruent
Let the height of the triangle be h and the length of the chord, which is a base of the triangle be l.
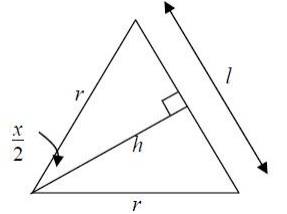
Use trigonometry to find l and h in terms of r and x.
The area of the triangle is determined as below,
Lastly, find the area of the segment.
Conclusion:
The equation is
(b)
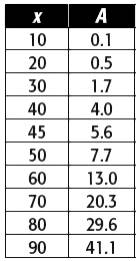
The table ten values of A for x ranging from 10 to 90
(b)
Answer to Problem 43PPS
The value is
Explanation of Solution
Given information:
Calculate and record in a table ten values of A for x-values ranging from 10 to 90 if r is 12 inches. Round to the nearest tenth
Formula used:
Calculation:
If r = 12, then the new formula is:
Enter this formula into Y1 of your calculator. The select the table
Conclusion:
The value is
(c)
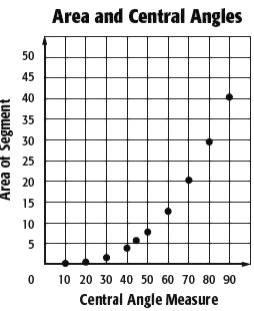
To sketch: The graph based on the x-values in the horizontal axis.
(c)
Answer to Problem 43PPS
The graph gets plotted against x and y values and hence the value get increases continuously.
Explanation of Solution
Given information:
Graph the data from your table with the x-values on the horizontal axis and the A-values on the vertical axis
Formula used:
The central angle measure is plotted against x axis and area of segment is plotted against y axis.
Calculation:
One other option would be to enter 10 through 90 by 10 in L1 and enter the formula for L2, replacing x with L1. Then, you can select STATPLOT L1, L2.
Conclusion:
The graph gets plotted against x and y values and hence the value get increases continuously.
(d)
The value of A.
(d)
Answer to Problem 43PPS
The area of the segment is about 15.0 when x is 63°
Explanation of Solution
Given information:
Use your graph to predict the value of A when x is 63. Then use the formula you generated in part a to calculate the value of A when x is 63. How do the values compare?
Formula used:
Calculation:
From the graph, it looks like the area would be about 15.5 when x is 63°.
Therefore, the area of the segment is about 15.0 when x is 63°
Conclusion:
The area of the segment is about 15.0 when x is 63°
Chapter 11 Solutions
Geometry, Student Edition
Additional Math Textbook Solutions
Elementary Statistics: Picturing the World (7th Edition)
Introductory Statistics
Calculus: Early Transcendentals (2nd Edition)
College Algebra with Modeling & Visualization (5th Edition)
Thinking Mathematically (6th Edition)
- Name: Date: Bell: Unit 11: Volume & Surface Area Homework 2: Area of Sectors Directions: Find the area of each shaded sector. Round to the hundredths place. 1. GH 11 in 2. KL 20 ft H F 64 G L 119 M K 3. BA 6.5 cm 4. YZ 14.2 m B 23 X 87° Y Z 5. KL = 27.1 mm J 32 L X:360-32.1 K A-3 360 7. BD 18 cm E 136 B X=32.8 127.0 (271) A: 069.13 Amm² 19=2102.13 A-136 360.16912 A:300cm² A=96.13 6. PQ = 2.8 in P R 311° 8. WZ 5.3 km V = Z 108 W D 9. HK = 25 ft G H KO 26 X 10. SR 26 m = S 73 T R Gina Wilson (All Things Algebarrow_forward538 Chapter 13 12. Given: Points E(-4, 1), F(2, 3), G(4, 9), and H(-2, 7) a. Show that EFGH is a rhombus. b. Use slopes to verify that the diagonals are perpendicular. 13. Given: Points R(-4, 5), S(-1, 9), T(7, 3) and U(4, -1) a. Show that RSTU is a rectangle. b. Use the distance formula to verify that the diagonals are congruent. 14. Given: Points N(-1, -5), O(0, 0), P(3, 2), and 2(8, 1) a. Show that NOPQ is an isosceles trapezoid. b. Show that the diagonals are congruent. Decide what special type of quadrilateral HIJK is. Then prove that your answer is correct. 15. H(0, 0) 16. H(0, 1) 17. H(7, 5) 18. H(-3, -3) I(5, 0) I(2,-3) 1(8, 3) I(-5, -6) J(7, 9) K(1, 9) J(-2, -1) K(-4, 3) J(0, -1) K(-1, 1) J(4, -5) K(6,-2) 19. Point N(3, - 4) lies on the circle x² + y² = 25. What is the slope of the (Hint: Recall Theorem 9-1.) - line that is tangent to the circle at N? 20. Point P(6, 7) lies on the circle (x + 2)² + (y − 1)² = 100. What is the slope of the line that is tangent to the circle at…arrow_forwardCan you cut the 12 glass triangles from a sheet of glass that is 4 feet by 8 feet? If so, how can it be done?arrow_forward
- Can you cut 12 glass triangles from a sheet of glass that is 4 feet by 8 feet? If so, draw a diagram of how it can be done.arrow_forwardIn triangle with sides of lengths a, b and c the angle a lays opposite to a. Prove the following inequality sin a 2√bc C α b a Warrow_forwardFind the values of x, y, and z. Round to the nearest tenth, if necessary. 8, 23arrow_forward
- 11 In the Pharlemina's Favorite quilt pattern below, vega-pxe-frame describe a motion that will take part (a) green to part (b) blue. Part (a) Part (b)arrow_forward5. 156 m/WXY = 59° 63 E 7. B E 101 C mFE = 6. 68° 8. C 17arrow_forward1/6/25, 3:55 PM Question: 14 Similar right triangles EFG and HIJ are shown. re of 120 √65 adjacent E hypotenuse adjaca H hypotenuse Item Bank | DnA Er:nollesup .es/prist Sisupe ed 12um jerit out i al F 4 G I oppe J 18009 90 ODPO ysma brs & eaus ps sd jon yem What is the value of tan J? ed on yem O broppo 4 ○ A. √65 Qx oppoEF Adj art saused taupe ed for yem 4 ○ B. √65 29 asipnisht riod 916 zelprisht rad √65 4 O ○ C. 4 √65 O D. VIS 9 OD elimiz 916 aelonsider saused supsarrow_forward
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning

