Glencoe Algebra 1, Student Edition, 9780079039897, 0079039898, 2018
18th Edition
ISBN: 9780079039897
Author: Carter
Publisher: McGraw Hill
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Question
Chapter 1, Problem 74SGR
To determine
To identify: The whether the graph provided below is linear or not. Also compute and interpret the intercepts, symmetry, positive, negative, increasing, decreasing and the x -coordinate of any relative extrema and end the end behavior of the graph.
Expert Solution & Answer
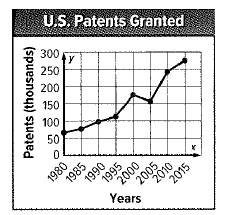
Answer to Problem 74SGR
The graph is non-linear. The y -intercept
Explanation of Solution
Given information:
The graphical representation of number of U.S. Patents granted from the year 1980 to 2015.
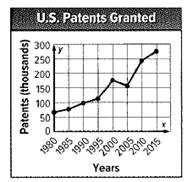
Formula used:
The graph of a function is said to be linear is it is straight line. If it is a curve it is non-linear.
The x- intercepts are the points on x -axis where the graph of the equation intersects the x -axis.
The y- intercepts are the points on y -axis where the graph of the equation intersects the y -axis.
When the graph of the function lies above the x -axis the function is said to be positive otherwise negative.
When the graph of the function goes up it is an increasing function when viewed from left to right otherwise function is decreasing.
The function has relative high and low values of function. A function has relative minimum when no other near by point has a lesser y -coordinate. A function has relative maximum when no other near by point has a greater y -coordinate.
End behavior of the graph represents the values of the function at positive and negative extremes in domain of the function.
Calculation:
Consider the graphical representation of number of U.S. Patents granted from the year 1980 to 2015.
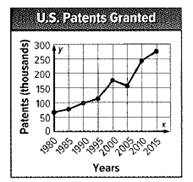
The x -axis denote the years and y -axis denote the number of patents in thousands.
Recall that the graph of a function is said to be linear is it is straight line. If it is a curve it is non-linear.
Observe that graph is not a straight line so it is a non-linear graph.
Also the graph of the function is not divided by any line into mirror images, so it is not symmetric also.
Recall that the x- intercepts are the points on x -axis where the graph of the equation intersects the x -axis.
Observe that the graph does not intersect the x -axis so there are no x -intercepts.
The y- intercepts are the points on y -axis where the graph of the equation intersects the y -axis.
Observe that the graph intersects the y -axis at the point
Recall that when the graph of the function lies above the x -axis the function is said to be positive otherwise negative.
Observe that graph of the function always lie above the x -axisso the function is said to be positive that is number of patents is always a positive number.
Recall that when the graph of the function goes up it is an increasing function when viewed from left to right otherwise function is decreasing.
When value of x lie between 1980 and 2000 also between 2005 and 2015 the function is increasing, the number of patents increase between the years 1980 and 2000 also between 2005 and 2015. The function decreasing when x lie between 2000 and 2005 that is number of patents decreased during that year.
Recall that the function has relative high and low values of function. A function has relative minimum when no other near by point has a lesser y -coordinate. A function has relative maximum when no other near by point has a greater y -coordinate.
At
Recall that the end behavior of the graph represents the values of the function at positive and negative extremes in domain of the function.
The graph describes the number U.S patents only till the year 2015. So end behavior of the graph is predicted by the nature of graph between the year 2010 and 2015. It is observed that the graph is increasing between the years so end behavior is number of patents increased between the years 2010 and 2015.
Chapter 1 Solutions
Glencoe Algebra 1, Student Edition, 9780079039897, 0079039898, 2018
Ch. 1.1 - Prob. 1AGPCh. 1.1 - Prob. 1BGPCh. 1.1 - Prob. 2AGPCh. 1.1 - Prob. 2BGPCh. 1.1 - Prob. 1CYUCh. 1.1 - Prob. 2CYUCh. 1.1 - Prob. 3CYUCh. 1.1 - Prob. 4CYUCh. 1.1 - Prob. 5CYUCh. 1.1 - Prob. 6CYU
Ch. 1.1 - Prob. 7CYUCh. 1.1 - Prob. 8CYUCh. 1.1 - Prob. 9CYUCh. 1.1 - Prob. 10CYUCh. 1.1 - Prob. 11PPSCh. 1.1 - Prob. 12PPSCh. 1.1 - Prob. 13PPSCh. 1.1 - Prob. 14PPSCh. 1.1 - Prob. 15PPSCh. 1.1 - Prob. 16PPSCh. 1.1 - Prob. 17PPSCh. 1.1 - Prob. 18PPSCh. 1.1 - Prob. 19PPSCh. 1.1 - Prob. 20PPSCh. 1.1 - Prob. 21PPSCh. 1.1 - Prob. 22PPSCh. 1.1 - Prob. 23PPSCh. 1.1 - Prob. 24PPSCh. 1.1 - Prob. 25PPSCh. 1.1 - Prob. 26PPSCh. 1.1 - Prob. 27PPSCh. 1.1 - Prob. 28PPSCh. 1.1 - Prob. 29PPSCh. 1.1 - Prob. 30PPSCh. 1.1 - Prob. 31PPSCh. 1.1 - Prob. 32PPSCh. 1.1 - Prob. 33PPSCh. 1.1 - Prob. 34PPSCh. 1.1 - Prob. 35PPSCh. 1.1 - Prob. 36PPSCh. 1.1 - Prob. 37PPSCh. 1.1 - Prob. 38HPCh. 1.1 - Prob. 39HPCh. 1.1 - Prob. 40HPCh. 1.1 - Prob. 41HPCh. 1.1 - Prob. 42HPCh. 1.1 - Prob. 43PFACh. 1.1 - Prob. 44PFACh. 1.1 - Prob. 45PFACh. 1.1 - Prob. 46PFACh. 1.1 - Prob. 47PFACh. 1.1 - Prob. 48PFACh. 1.1 - Prob. 49PFACh. 1.2 - Prob. 1AGPCh. 1.2 - Prob. 1BGPCh. 1.2 - Prob. 1CGPCh. 1.2 - Prob. 2AGPCh. 1.2 - Prob. 2BGPCh. 1.2 - Prob. 3AGPCh. 1.2 - Prob. 3BGPCh. 1.2 - Prob. 3CGPCh. 1.2 - Prob. 4AGPCh. 1.2 - Prob. 4BGPCh. 1.2 - Prob. 5AGPCh. 1.2 - Prob. 1CYUCh. 1.2 - Prob. 2CYUCh. 1.2 - Prob. 3CYUCh. 1.2 - Prob. 4CYUCh. 1.2 - Prob. 5CYUCh. 1.2 - Prob. 6CYUCh. 1.2 - Prob. 7CYUCh. 1.2 - Prob. 8CYUCh. 1.2 - Prob. 9CYUCh. 1.2 - Prob. 10CYUCh. 1.2 - Prob. 11CYUCh. 1.2 - Prob. 12CYUCh. 1.2 - Prob. 13CYUCh. 1.2 - Prob. 14CYUCh. 1.2 - Prob. 15PPSCh. 1.2 - Prob. 16PPSCh. 1.2 - Prob. 17PPSCh. 1.2 - Prob. 18PPSCh. 1.2 - Prob. 19PPSCh. 1.2 - Prob. 20PPSCh. 1.2 - Prob. 21PPSCh. 1.2 - Prob. 22PPSCh. 1.2 - Prob. 23PPSCh. 1.2 - Prob. 24PPSCh. 1.2 - Prob. 25PPSCh. 1.2 - Prob. 26PPSCh. 1.2 - Prob. 27PPSCh. 1.2 - Prob. 28PPSCh. 1.2 - Prob. 29PPSCh. 1.2 - Prob. 30PPSCh. 1.2 - Prob. 31PPSCh. 1.2 - Prob. 32PPSCh. 1.2 - Prob. 33PPSCh. 1.2 - Prob. 34PPSCh. 1.2 - Prob. 35PPSCh. 1.2 - Prob. 36PPSCh. 1.2 - Prob. 37PPSCh. 1.2 - Prob. 38PPSCh. 1.2 - Prob. 39PPSCh. 1.2 - Prob. 40PPSCh. 1.2 - Prob. 41PPSCh. 1.2 - Prob. 42PPSCh. 1.2 - Prob. 43PPSCh. 1.2 - Prob. 44PPSCh. 1.2 - Prob. 45PPSCh. 1.2 - Prob. 46PPSCh. 1.2 - Prob. 47PPSCh. 1.2 - Prob. 48PPSCh. 1.2 - Prob. 49PPSCh. 1.2 - Prob. 50PPSCh. 1.2 - Prob. 51PPSCh. 1.2 - Prob. 52PPSCh. 1.2 - Prob. 53PPSCh. 1.2 - Prob. 54PPSCh. 1.2 - Prob. 55PPSCh. 1.2 - Prob. 56PPSCh. 1.2 - Prob. 57PPSCh. 1.2 - Prob. 58PPSCh. 1.2 - Prob. 59HPCh. 1.2 - Prob. 60HPCh. 1.2 - Prob. 61HPCh. 1.2 - Prob. 62HPCh. 1.2 - Prob. 63HPCh. 1.2 - Prob. 64HPCh. 1.2 - Prob. 65PFACh. 1.2 - Prob. 66PFACh. 1.2 - Prob. 67PFACh. 1.2 - Prob. 68PFACh. 1.2 - Prob. 69PFACh. 1.2 - Prob. 70PFACh. 1.2 - Prob. 71PFACh. 1.3 - Prob. 1AGPCh. 1.3 - Prob. 1BGPCh. 1.3 - Prob. 2GPCh. 1.3 - Prob. 3AGPCh. 1.3 - Prob. 3BGPCh. 1.3 - Prob. 1CYUCh. 1.3 - Prob. 2CYUCh. 1.3 - Prob. 3CYUCh. 1.3 - Prob. 4CYUCh. 1.3 - Prob. 5CYUCh. 1.3 - Prob. 6CYUCh. 1.3 - Prob. 7CYUCh. 1.3 - Prob. 8CYUCh. 1.3 - Prob. 9PPSCh. 1.3 - Prob. 10PPSCh. 1.3 - Prob. 11PPSCh. 1.3 - Prob. 12PPSCh. 1.3 - Prob. 13PPSCh. 1.3 - Prob. 14PPSCh. 1.3 - Prob. 15PPSCh. 1.3 - Prob. 16PPSCh. 1.3 - Prob. 17PPSCh. 1.3 - Prob. 18PPSCh. 1.3 - Prob. 19PPSCh. 1.3 - Prob. 20PPSCh. 1.3 - Prob. 21PPSCh. 1.3 - Prob. 22PPSCh. 1.3 - Prob. 23PPSCh. 1.3 - Prob. 24PPSCh. 1.3 - Prob. 25PPSCh. 1.3 - Prob. 26PPSCh. 1.3 - Prob. 27PPSCh. 1.3 - Prob. 28PPSCh. 1.3 - Prob. 29PPSCh. 1.3 - Prob. 30PPSCh. 1.3 - Prob. 31PPSCh. 1.3 - Prob. 32PPSCh. 1.3 - Prob. 33PPSCh. 1.3 - Prob. 34PPSCh. 1.3 - Prob. 35PPSCh. 1.3 - Prob. 36PPSCh. 1.3 - Prob. 37PPSCh. 1.3 - Prob. 38PPSCh. 1.3 - Prob. 39PPSCh. 1.3 - Prob. 40PPSCh. 1.3 - Prob. 41PPSCh. 1.3 - Prob. 42PPSCh. 1.3 - Prob. 43PPSCh. 1.3 - Prob. 44PPSCh. 1.3 - Prob. 45PPSCh. 1.3 - Prob. 46PPSCh. 1.3 - Prob. 47PPSCh. 1.3 - Prob. 48PPSCh. 1.3 - Prob. 49PPSCh. 1.3 - Prob. 50PPSCh. 1.3 - Prob. 51PPSCh. 1.3 - Prob. 52PPSCh. 1.3 - Prob. 53PPSCh. 1.3 - Prob. 54HPCh. 1.3 - Prob. 55HPCh. 1.3 - Prob. 56HPCh. 1.3 - Prob. 57HPCh. 1.3 - Prob. 58HPCh. 1.3 - Prob. 59HPCh. 1.3 - Prob. 60HPCh. 1.3 - Prob. 61PFACh. 1.3 - Prob. 62PFACh. 1.3 - Prob. 63PFACh. 1.3 - Prob. 64PFACh. 1.3 - Prob. 65PFACh. 1.3 - Prob. 66PFACh. 1.3 - Prob. 67PFACh. 1.4 - Prob. 1GPCh. 1.4 - Prob. 2AGPCh. 1.4 - Prob. 2BGPCh. 1.4 - Prob. 2CGPCh. 1.4 - Prob. 2DGPCh. 1.4 - Prob. 3AGPCh. 1.4 - Prob. 3BGPCh. 1.4 - Prob. 3CGPCh. 1.4 - Prob. 3DGPCh. 1.4 - Prob. 4AGPCh. 1.4 - Prob. 4BGPCh. 1.4 - Prob. 4CGPCh. 1.4 - Prob. 4DGPCh. 1.4 - Prob. 5GPCh. 1.4 - Prob. 1CYUCh. 1.4 - Prob. 2CYUCh. 1.4 - Prob. 3CYUCh. 1.4 - Prob. 4CYUCh. 1.4 - Prob. 5CYUCh. 1.4 - Prob. 6CYUCh. 1.4 - Prob. 7CYUCh. 1.4 - Prob. 8CYUCh. 1.4 - Prob. 9CYUCh. 1.4 - Prob. 10CYUCh. 1.4 - Prob. 11PPSCh. 1.4 - Prob. 12PPSCh. 1.4 - Prob. 13PPSCh. 1.4 - Prob. 14PPSCh. 1.4 - Prob. 15PPSCh. 1.4 - Prob. 16PPSCh. 1.4 - Prob. 17PPSCh. 1.4 - Prob. 18PPSCh. 1.4 - Prob. 19PPSCh. 1.4 - Prob. 20PPSCh. 1.4 - Prob. 21PPSCh. 1.4 - Prob. 22PPSCh. 1.4 - Prob. 23PPSCh. 1.4 - Prob. 24PPSCh. 1.4 - Prob. 25PPSCh. 1.4 - Prob. 26PPSCh. 1.4 - Prob. 27PPSCh. 1.4 - Prob. 28PPSCh. 1.4 - Prob. 29PPSCh. 1.4 - Prob. 30PPSCh. 1.4 - Prob. 31PPSCh. 1.4 - Prob. 32PPSCh. 1.4 - Prob. 33PPSCh. 1.4 - Prob. 34PPSCh. 1.4 - Prob. 35PPSCh. 1.4 - Prob. 36PPSCh. 1.4 - Prob. 37PPSCh. 1.4 - Prob. 38PPSCh. 1.4 - Prob. 39PPSCh. 1.4 - Prob. 40PPSCh. 1.4 - Prob. 41PPSCh. 1.4 - Prob. 42PPSCh. 1.4 - Prob. 43PPSCh. 1.4 - Prob. 44PPSCh. 1.4 - Prob. 45PPSCh. 1.4 - Prob. 46PPSCh. 1.4 - Prob. 47PPSCh. 1.4 - Prob. 48PPSCh. 1.4 - Prob. 49PPSCh. 1.4 - Prob. 50PPSCh. 1.4 - Prob. 51PPSCh. 1.4 - Prob. 52PPSCh. 1.4 - Prob. 53PPSCh. 1.4 - Prob. 54PPSCh. 1.4 - Prob. 55PPSCh. 1.4 - Prob. 56HPCh. 1.4 - Prob. 57HPCh. 1.4 - Prob. 58HPCh. 1.4 - Prob. 59HPCh. 1.4 - Prob. 60PFACh. 1.4 - Prob. 61PFACh. 1.4 - Prob. 62PFACh. 1.4 - Prob. 63PFACh. 1.4 - Prob. 64PFACh. 1.4 - Prob. 65PFACh. 1.4 - Prob. 66PFACh. 1.5 - Prob. 1AGPCh. 1.5 - Prob. 1BGPCh. 1.5 - Prob. 1CGPCh. 1.5 - Prob. 2AGPCh. 1.5 - Prob. 2BGPCh. 1.5 - Prob. 3AGPCh. 1.5 - Prob. 3BGPCh. 1.5 - Prob. 3CGPCh. 1.5 - Prob. 4AGPCh. 1.5 - Prob. 4BGPCh. 1.5 - Prob. 4CGPCh. 1.5 - Prob. 1CYUCh. 1.5 - Prob. 2CYUCh. 1.5 - Prob. 3CYUCh. 1.5 - Prob. 4CYUCh. 1.5 - Prob. 5PPSCh. 1.5 - Prob. 6PPSCh. 1.5 - Prob. 7PPSCh. 1.5 - Prob. 8PPSCh. 1.5 - Prob. 9PPSCh. 1.5 - Prob. 10PPSCh. 1.5 - Prob. 11PPSCh. 1.5 - Prob. 12PPSCh. 1.5 - Prob. 13PPSCh. 1.5 - Prob. 14PPSCh. 1.5 - Prob. 15PPSCh. 1.5 - Prob. 16PPSCh. 1.5 - Prob. 17PPSCh. 1.5 - Prob. 18PPSCh. 1.5 - Prob. 19PPSCh. 1.5 - Prob. 20PPSCh. 1.5 - Prob. 21PPSCh. 1.5 - Prob. 22PPSCh. 1.5 - Prob. 23PPSCh. 1.5 - Prob. 24PPSCh. 1.5 - Prob. 25PPSCh. 1.5 - Prob. 26PPSCh. 1.5 - Prob. 27HPCh. 1.5 - Prob. 28HPCh. 1.5 - Prob. 29PFACh. 1.5 - Prob. 30PFACh. 1.5 - Prob. 31PFACh. 1.5 - Prob. 32PFACh. 1.5 - Prob. 33PFACh. 1.5 - Prob. 34PFACh. 1.5 - Prob. 35PFACh. 1.6 - Prob. 1AGPCh. 1.6 - Prob. 1BGPCh. 1.6 - Prob. 2AGPCh. 1.6 - Prob. 2BGPCh. 1.6 - Prob. 3AGPCh. 1.6 - Prob. 3BGPCh. 1.6 - Prob. 1CYUCh. 1.6 - Prob. 2CYUCh. 1.6 - Prob. 3CYUCh. 1.6 - Prob. 4CYUCh. 1.6 - Prob. 5CYUCh. 1.6 - Prob. 6CYUCh. 1.6 - Prob. 7CYUCh. 1.6 - Prob. 8CYUCh. 1.6 - Prob. 9PPSCh. 1.6 - Prob. 10PPSCh. 1.6 - Prob. 11PPSCh. 1.6 - Prob. 12PPSCh. 1.6 - Prob. 13PPSCh. 1.6 - Prob. 14PPSCh. 1.6 - Prob. 15PPSCh. 1.6 - Prob. 16PPSCh. 1.6 - Prob. 17PPSCh. 1.6 - Prob. 18PPSCh. 1.6 - Prob. 19PPSCh. 1.6 - Prob. 20PPSCh. 1.6 - Prob. 21PPSCh. 1.6 - Prob. 22PPSCh. 1.6 - Prob. 23PPSCh. 1.6 - Prob. 24PPSCh. 1.6 - Prob. 25PPSCh. 1.6 - Prob. 26PPSCh. 1.6 - Prob. 27PPSCh. 1.6 - Prob. 28PPSCh. 1.6 - Prob. 29PPSCh. 1.6 - Prob. 30PPSCh. 1.6 - Prob. 31PPSCh. 1.6 - Prob. 32PPSCh. 1.6 - Prob. 33PPSCh. 1.6 - Prob. 34PPSCh. 1.6 - Prob. 35PPSCh. 1.6 - Prob. 36PPSCh. 1.6 - Prob. 37PPSCh. 1.6 - Prob. 38HPCh. 1.6 - Prob. 39HPCh. 1.6 - Prob. 40HPCh. 1.6 - Prob. 41HPCh. 1.6 - Prob. 42HPCh. 1.6 - Prob. 43PFACh. 1.6 - Prob. 44PFACh. 1.6 - Prob. 45PFACh. 1.6 - Prob. 46PFACh. 1.6 - Prob. 47PFACh. 1.7 - Prob. 1GPCh. 1.7 - Prob. 2GPCh. 1.7 - Prob. 3AGPCh. 1.7 - Prob. 3BGPCh. 1.7 - Prob. 4AGPCh. 1.7 - Prob. 4BGPCh. 1.7 - Prob. 4CGPCh. 1.7 - Prob. 4DGPCh. 1.7 - Prob. 5AGPCh. 1.7 - Prob. 5BGPCh. 1.7 - Prob. 5CGPCh. 1.7 - Prob. 5DGPCh. 1.7 - Prob. 1CYUCh. 1.7 - Prob. 2CYUCh. 1.7 - Prob. 3CYUCh. 1.7 - Prob. 4CYUCh. 1.7 - Prob. 5CYUCh. 1.7 - Prob. 6CYUCh. 1.7 - Prob. 7CYUCh. 1.7 - Prob. 8CYUCh. 1.7 - Prob. 9CYUCh. 1.7 - Prob. 10CYUCh. 1.7 - Prob. 11CYUCh. 1.7 - Prob. 12CYUCh. 1.7 - Prob. 13CYUCh. 1.7 - Prob. 14CYUCh. 1.7 - Prob. 15CYUCh. 1.7 - Prob. 16CYUCh. 1.7 - Prob. 17CYUCh. 1.7 - Prob. 18CYUCh. 1.7 - Prob. 19CYUCh. 1.7 - Prob. 20PPSCh. 1.7 - Prob. 21PPSCh. 1.7 - Prob. 22PPSCh. 1.7 - Prob. 23PPSCh. 1.7 - Prob. 24PPSCh. 1.7 - Prob. 25PPSCh. 1.7 - Prob. 26PPSCh. 1.7 - Prob. 27PPSCh. 1.7 - Prob. 28PPSCh. 1.7 - Prob. 29PPSCh. 1.7 - Prob. 30PPSCh. 1.7 - Prob. 31PPSCh. 1.7 - Prob. 32PPSCh. 1.7 - Prob. 33PPSCh. 1.7 - Prob. 34PPSCh. 1.7 - Prob. 35PPSCh. 1.7 - Prob. 36PPSCh. 1.7 - Prob. 37PPSCh. 1.7 - Prob. 38PPSCh. 1.7 - Prob. 39PPSCh. 1.7 - Prob. 40PPSCh. 1.7 - Prob. 41PPSCh. 1.7 - Prob. 42PPSCh. 1.7 - Prob. 43PPSCh. 1.7 - Prob. 44PPSCh. 1.7 - Prob. 45PPSCh. 1.7 - Prob. 46PPSCh. 1.7 - Prob. 47PPSCh. 1.7 - Prob. 48PPSCh. 1.7 - Prob. 49HPCh. 1.7 - Prob. 50HPCh. 1.7 - Prob. 51HPCh. 1.7 - Prob. 52HPCh. 1.7 - Prob. 53HPCh. 1.7 - Prob. 54HPCh. 1.7 - Prob. 55HPCh. 1.7 - Prob. 56PFACh. 1.7 - Prob. 57PFACh. 1.7 - Prob. 58PFACh. 1.7 - Prob. 59PFACh. 1.7 - Prob. 60PFACh. 1.7 - Prob. 61PFACh. 1.8 - Prob. 1GPCh. 1.8 - Prob. 2GPCh. 1.8 - Prob. 3GPCh. 1.8 - Prob. 4GPCh. 1.8 - Prob. 1CYUCh. 1.8 - Prob. 2CYUCh. 1.8 - Prob. 3CYUCh. 1.8 - Prob. 4CYUCh. 1.8 - Prob. 5PPSCh. 1.8 - Prob. 6PPSCh. 1.8 - Prob. 7PPSCh. 1.8 - Prob. 8PPSCh. 1.8 - Prob. 9PPSCh. 1.8 - Prob. 10PPSCh. 1.8 - Prob. 11PPSCh. 1.8 - Prob. 12PPSCh. 1.8 - Prob. 13PPSCh. 1.8 - Prob. 14PPSCh. 1.8 - Prob. 15PPSCh. 1.8 - Prob. 16PPSCh. 1.8 - Prob. 17PPSCh. 1.8 - Prob. 18PPSCh. 1.8 - Prob. 19PPSCh. 1.8 - Prob. 20HPCh. 1.8 - Prob. 21HPCh. 1.8 - Prob. 22HPCh. 1.8 - Prob. 23HPCh. 1.8 - Prob. 24HPCh. 1.8 - Prob. 25PFACh. 1.8 - Prob. 26PFACh. 1.8 - Prob. 27PFACh. 1.8 - Prob. 28PFACh. 1.8 - Prob. 29PFACh. 1.8 - Prob. 30PFACh. 1 - Prob. 1MCQCh. 1 - Prob. 2MCQCh. 1 - Prob. 3MCQCh. 1 - Prob. 4MCQCh. 1 - Prob. 5MCQCh. 1 - Prob. 6MCQCh. 1 - Prob. 7MCQCh. 1 - Prob. 8MCQCh. 1 - Prob. 9MCQCh. 1 - Prob. 10MCQCh. 1 - Prob. 11MCQCh. 1 - Prob. 12MCQCh. 1 - Prob. 13MCQCh. 1 - Prob. 14MCQCh. 1 - Prob. 15MCQCh. 1 - Prob. 16MCQCh. 1 - Prob. 17MCQCh. 1 - Prob. 18MCQCh. 1 - Prob. 19MCQCh. 1 - Prob. 20MCQCh. 1 - Prob. 21MCQCh. 1 - Prob. 22MCQCh. 1 - Prob. 23MCQCh. 1 - Prob. 24MCQCh. 1 - Prob. 25MCQCh. 1 - Prob. 1SGRCh. 1 - Prob. 2SGRCh. 1 - Prob. 3SGRCh. 1 - Prob. 4SGRCh. 1 - Prob. 5SGRCh. 1 - Prob. 6SGRCh. 1 - Prob. 7SGRCh. 1 - Prob. 8SGRCh. 1 - Prob. 9SGRCh. 1 - Prob. 10SGRCh. 1 - Prob. 11SGRCh. 1 - Prob. 12SGRCh. 1 - Prob. 13SGRCh. 1 - Prob. 14SGRCh. 1 - Prob. 15SGRCh. 1 - Prob. 16SGRCh. 1 - Prob. 17SGRCh. 1 - Prob. 18SGRCh. 1 - Prob. 19SGRCh. 1 - Prob. 20SGRCh. 1 - Prob. 21SGRCh. 1 - Prob. 22SGRCh. 1 - Prob. 23SGRCh. 1 - Prob. 24SGRCh. 1 - Prob. 25SGRCh. 1 - Prob. 26SGRCh. 1 - Prob. 27SGRCh. 1 - Prob. 28SGRCh. 1 - Prob. 29SGRCh. 1 - Prob. 30SGRCh. 1 - Prob. 31SGRCh. 1 - Prob. 32SGRCh. 1 - Prob. 33SGRCh. 1 - Prob. 34SGRCh. 1 - Prob. 35SGRCh. 1 - Prob. 36SGRCh. 1 - Prob. 37SGRCh. 1 - Prob. 38SGRCh. 1 - Prob. 39SGRCh. 1 - Prob. 40SGRCh. 1 - Prob. 41SGRCh. 1 - Prob. 42SGRCh. 1 - Prob. 43SGRCh. 1 - Prob. 44SGRCh. 1 - Prob. 45SGRCh. 1 - Prob. 46SGRCh. 1 - Prob. 47SGRCh. 1 - Prob. 48SGRCh. 1 - Prob. 49SGRCh. 1 - Prob. 50SGRCh. 1 - Prob. 51SGRCh. 1 - Prob. 52SGRCh. 1 - Prob. 53SGRCh. 1 - Prob. 54SGRCh. 1 - Prob. 55SGRCh. 1 - Prob. 56SGRCh. 1 - Prob. 57SGRCh. 1 - Prob. 58SGRCh. 1 - Prob. 59SGRCh. 1 - Prob. 60SGRCh. 1 - Prob. 61SGRCh. 1 - Prob. 62SGRCh. 1 - Prob. 63SGRCh. 1 - Prob. 64SGRCh. 1 - Prob. 65SGRCh. 1 - Prob. 66SGRCh. 1 - Prob. 67SGRCh. 1 - Prob. 68SGRCh. 1 - Prob. 69SGRCh. 1 - Prob. 70SGRCh. 1 - Prob. 71SGRCh. 1 - Prob. 72SGRCh. 1 - Prob. 73SGRCh. 1 - Prob. 74SGRCh. 1 - Prob. 75SGRCh. 1 - Prob. 1PTCh. 1 - Prob. 2PTCh. 1 - Prob. 3PTCh. 1 - Prob. 4PTCh. 1 - Prob. 5PTCh. 1 - Prob. 6PTCh. 1 - Prob. 7PTCh. 1 - Prob. 8PTCh. 1 - Prob. 9PTCh. 1 - Prob. 10PTCh. 1 - Prob. 11PTCh. 1 - Prob. 12PTCh. 1 - Prob. 13PTCh. 1 - Prob. 14PTCh. 1 - Prob. 15PTCh. 1 - Prob. 16PTCh. 1 - Prob. 17PTCh. 1 - Prob. 18PTCh. 1 - Prob. 19PTCh. 1 - Prob. 20PTCh. 1 - Prob. 21PTCh. 1 - Prob. 1PFACh. 1 - Prob. 2PFACh. 1 - Prob. 3PFACh. 1 - Prob. 4PFACh. 1 - Prob. 5PFACh. 1 - Prob. 6PFACh. 1 - Prob. 7PFACh. 1 - Prob. 8PFACh. 1 - Prob. 9PFACh. 1 - Prob. 10PFACh. 1 - Prob. 11PFACh. 1 - Prob. 12PFACh. 1 - Prob. 13PFACh. 1 - Prob. 14PFACh. 1 - Prob. 15PFA
Additional Math Textbook Solutions
Find more solutions based on key concepts
Find the point-slope form of the line passing through the given points. Use the first point as (x1, .y1). Plot ...
College Algebra with Modeling & Visualization (5th Edition)
x vs. Two symbols are used for the mean: and x. a. Which represents a parameter, and which a statistic? b. In...
Introductory Statistics
The null hypothesis, alternative hypothesis, test statistic, P-value and state the conclusion. To test: Whether...
Elementary Statistics
Comparison tests Use the Comparison Test or Limit Comparison Test to determine whether the following series con...
Calculus: Early Transcendentals (2nd Edition)
Fill in each blank so that the resulting statement is true.
1. A combination of numbers, variables, and opera...
College Algebra (7th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- Kate, Luke, Mary and Nancy are sharing a cake. The cake had previously been divided into four slices (s1, s2, s3 and s4). The following table shows the values of the slices in the eyes of each player. What is fair share to nancy? S1 S2 S3 S4 Kate $4.00 $6.00 $6.00 $4.00 Luke $5.30 $5.00 $5.25 $5.45 Mary $4.25 $4.50 $3.50 $3.75 Nancy $6.00 $4.00 $4.00 $6.00arrow_forwardKate, Luke, Mary and Nancy are sharing a cake. The cake had previously been divided into four slices (s1, s2, s3 and s4). The following table shows the values of the slices in the eyes of each player. S1 S2 S3 S4 Kate $4.00 $6.00 $6.00 $4.00 Luke $5.30 $5.00 $5.25 $5.45 Mary $4.25 $4.50 $3.50 $3.75 Nancy $6.00 $4.00 $4.00 $6.00 how much is the cak worth to maryarrow_forwardKate, Luke, Mary and Nancy are sharing a cake. The cake had previously been divided into four slices (s1, s2, s3 and s4). The following table shows the values of the slices in the eyes of each player. What is the threshold of fair share for Luke? S1 S2 S3 S4 Kate $4.00 $6.00 $6.00 $4.00 Luke $5.30 $5.00 $5.25 $5.45 Mary $4.25 $4.50 $3.50 $3.75 Nancy $6.00 $4.00 $4.00 $6.00arrow_forward
- 2. A microwave manufacturing firm has determined that their profit function is P(x)=-0.0014x+0.3x²+6x-355 , where is the number of microwaves sold annually. a. Graph the profit function using a calculator. b. Determine a reasonable viewing window for the function. c. Approximate all of the zeros of the function using the CALC menu of your calculator. d. What must be the range of microwaves sold in order for the firm to profit?arrow_forwardA clothing manufacturer's profitability can be modeled by p (x)=-x4 + 40x² - 144, where .x is the number of items sold in thousands and p (x) is the company's profit in thousands of dollars. a. Sketch the function on your calculator and describe the end behavior. b. Determine the zeros of the function. c. Between what two values should the company sell in order to be profitable? d. Explain why only two of the zeros are considered in part c.arrow_forwardCCSS REASONING The number of subscribers using pagers in the United States can be modeled by f(x) = 0.015x4 -0.44x³ +3.46x² - 2.7x+9.68 where x is the number of years after 1990 and f(x) is the number of subscribers in millions. a. Graph the function. b. Describe the end behavior of the graph. c. What does the end behavior suggest about the number of pager subscribers? d. Will this trend continue indefinitely? Explain your reasoning.arrow_forward
- Can you help me solve this?arrow_forwardName Assume there is the following simplified grade book: Homework Labs | Final Exam | Project Avery 95 98 90 100 Blake 90 96 Carlos 83 79 Dax 55 30 228 92 95 79 90 65 60 Assume that the weights used to compute the final grades are homework 0.3, labs 0.2, the final 0.35, and the project 0.15. | Write an explicit formula to compute Avery's final grade using a single inner product. Write an explicit formula to compute everyone's final grade simultane- ously using a single matrix-vector product.arrow_forward1. Explicitly compute by hand (with work shown) the following Frobenius inner products 00 4.56 3.12 (a) ((º º º). (156 (b) 10.9 -1 0 2)), Fro 5')) Froarrow_forward
- 3. Let 4 0 0 00 0 0 1.2 0 00 0 0 0 -10.1 0 0 0 D = 0 0 0 00 0 0 0 0 05 0 0 0 0 0 0 2.8 Either explicitly compute D-¹ or explain why it doesn't exist.arrow_forward4. [9 points] Assume that B, C, E are all 3 x 3 matrices such that BC == -64 -1 0 3 4 4 4 -2 2 CB=-1-2 4 BE -2 1 3 EC = 1 3 2 -7, 1 6 -6 2-5 -7 -2 Explicitly compute the following by hand. (I.e., write out the entries of the 3 × 3 matrix.) (a) [3 points] B(E+C) (b) [3 points] (E+B)C (c) [3 points] ETBTarrow_forward6. Consider the matrices G = 0 (3) -3\ -3 2 and H = -1 2 0 5 0 5 5 noting that H(:, 3) = 2H(:,1) + H(:, 2). Is G invertible? Explain your answer. Is H invertible? Explain your answer. Use co-factor expansion to find the determinant of H. (Hint: expand the 2nd or 3rd row)arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON
Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON
Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education
Finding Local Maxima and Minima by Differentiation; Author: Professor Dave Explains;https://www.youtube.com/watch?v=pvLj1s7SOtk;License: Standard YouTube License, CC-BY