Chapter 9.2, Problem 11P

For the beam and loading shown, (a) express the magnitude and location of the maximum deflection in terms of w₀, L, E, and I. (b) Calculate the value of the maximum deflection, assuming that beam AB is a W18 × 50 rolled shape and that w₀= 4.5 kips/ft, L = 18 ft, and E = 29 ×10⁶ psi.

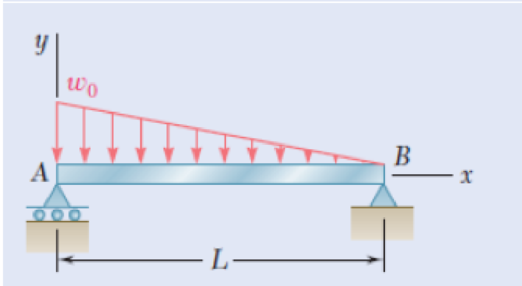
Fig. P9.11

To determine

The magnitude and location of the maximum deflection in terms of $w_{0,}$ $L,$ $E,$ and I.

Answer to Problem 11P

The location of the maximum deflection $x_m$ is $\underset{\_}{0.481L}$.

The magnitude and location of the maximum deflection in terms of $w_{0,}$ $L,$ $E,$ and I is $\underset{\_}{0.00652\frac{w_0{L}^4}{EI}\downarrow }$.

Explanation of Solution

Given that:

The length (L) of the beam is $18\text{ft}$.

The load $w_0$ is $4.5\text{kips}/\text{ft}$.

The young’s modulus E is $29\times {10}^6\text{psi}$.

Calculation:

Sketch the free body diagram of beam as shown in Figure 1.

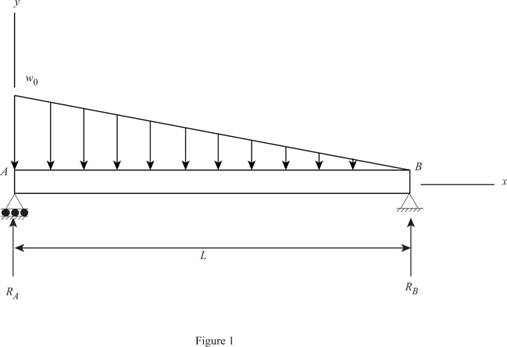
Find the reactions of the beam.

$R_A+R_B=\frac{1}{2}\times w_o\times L$

Take the moment at B.

$\begin{array}{c}{R}_A\times L-\left(\frac{1}{2}\times w_o\times L\right)\left(\frac{2}{3}\times L\right)=0\\ R_A\times L-\frac{w_o{L}^2}{3}=0\\ R_A=\frac{w_o{L}^2}{3}\times \frac{1}{L}\\ =\frac{w_{o}L}{3}\end{array}$

Find the reaction at B.

$\begin{array}{c}{R}_A+R_B=\frac{1}{2}\times w_o\times L\\ \frac{w_{o}L}{3}+R_B=\frac{1}{2}\times w_o\times L\\ R_B=\frac{w_{o}L}{2}-\frac{w_{o}L}{3}\\ =\frac{w_{o}L}{6}\end{array}$

Take the section 1-1 at x distance from A as shown in Figure 2.

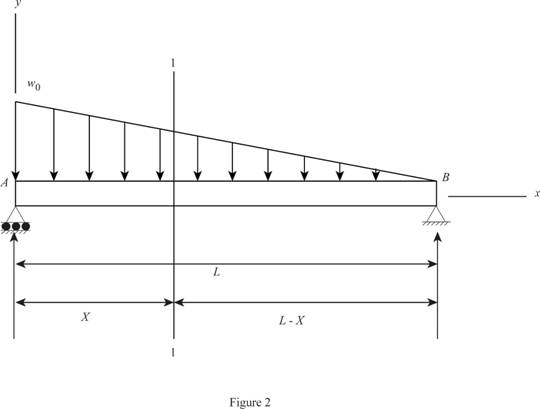
Consider a section $x-x$ at a distance x from a.

Sketch the section x-x as shown in Figure 3.

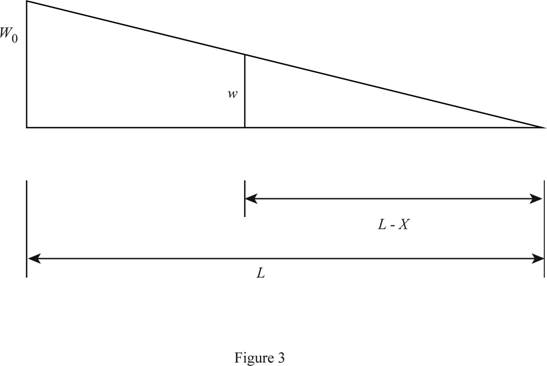
Calculate the intensity of loading w at the section x using similar triangle method as shown below:

$\begin{array}{l}\frac{w}{L-x}=\frac{w_0}{L}\\ w=\frac{w_0}{L}\left(L-x\right)\end{array}$

Find the shear force using the expression as follows:

$\begin{array}{c}\frac{dV}{dx}=-w\\ =-\frac{w_o\left(L-x\right)}{L}\end{array}$

Find the shear force using integration:

$\begin{array}{c}\frac{dV}{dx}=\frac{-w_o\left(L-x\right)}{L}\\ V=-\frac{w_o}{L}\left(Lx-\frac{x^2}{2}\right)+C_V\\ =\frac{dM}{dx}\end{array}$

Find the moment using the relation as follows:

$\begin{array}{l}\frac{dM}{dx}=-\frac{w_o}{L}\left(Lx-\frac{x^2}{2}\right)+C_V\\ M=-\frac{w_o}{L}\left(\frac{L{x}^2}{2}-\frac{x^3}{6}\right)+C_{V}x+C_M\end{array}$ (1)

Apply the boundary conditions:

When $x=0$, the moment $M=0$, substitute in Equation (1):

$\begin{array}{l}M=-\frac{w_o}{L}\left(\frac{L{x}^2}{2}-\frac{x^3}{6}\right)+C_{V}x+C_M\\ 0=-\frac{w_o}{L}\left(0-0\right)+0+C_M\\ C_M=0\end{array}$

When $x=L$, the moment $M=0$.

Substitute 0 for $C_M$ and boundary condition in Equation (1):

$\begin{array}{c}M=-\frac{w_o}{L}\left(\frac{L{x}^2}{2}-\frac{x^3}{6}\right)+C_{V}x+C_M\\ 0=-\frac{w_o}{L}\left(\frac{L{\left(L\right)}^2}{2}-\frac{L^3}{6}\right)+C_{V}L+0\\ =-\frac{w_o}{L}\left(\frac{L^3}{2}-\frac{L^3}{6}\right)+C_{V}L\\ =-\frac{w_o}{L}\left(\frac{2L^3}{6}\right)+C_{V}L\end{array}$

$\begin{array}{c}{C}_{V}L=\frac{w_o}{L}\left(\frac{L^3}{3}\right)\\ C_V=\frac{w_o}{L}\left(\frac{L^3}{3}\right)\left(\frac{1}{L}\right)\\ =\frac{w_{o}L}{3}\end{array}$

Write the moment Equation:

$\begin{array}{c}EI\frac{d^{2}y}{d{x}^2}=M\\ =-\frac{w_o}{L}\left(\frac{L{x}^2}{2}-\frac{x^3}{6}\right)+C_{V}x+C_M\end{array}$

Substitute $\frac{w_{o}L}{3}$ for $C_V$ and 0 for $C_M$.

$\begin{array}{c}EI\frac{d^{2}y}{d{x}^2}=-\frac{w_o}{L}\left(\frac{L{x}^2}{2}-\frac{x^3}{6}\right)+\left(\frac{w_{0}L}{3}\right)x+0\\ =-\frac{w_o}{L}\left(\frac{L{x}^2}{2}-\frac{x^3}{6}\right)+\left(\frac{w_{0}L}{3}\right)x\times \left(\frac{L}{L}\right)\\ =-\frac{w_o}{L}\left(\frac{L{x}^2}{2}-\frac{x^3}{6}-\frac{L^{2}x}{3}\right)\\ =\frac{w_o}{L}\left(\frac{L^{2}x}{3}-\frac{L{x}^2}{2}+\frac{x^3}{6}\right)\end{array}$ (2)

Integrate the Equation (2).

$\begin{array}{c}EI\frac{d^{2}y}{d{x}^2}=\frac{w_o}{L}\left(\frac{L^{2}x}{3}-\frac{L{x}^2}{2}+\frac{x^3}{6}\right)\\ EI\frac{dy}{dx}=\frac{w_o}{L}\left(\frac{L^2{x}^2}{6}-\frac{L{x}^3}{6}+\frac{x^4}{24}\right)+C_1\end{array}$ (3)

Integrate the Equation (3).

$\begin{array}{l}EI\frac{dy}{dx}=\frac{w_o}{L}\left(\frac{L^2{x}^2}{6}-\frac{L{x}^3}{6}+\frac{x^4}{24}\right)+C_1\\ EIy=\frac{w_o}{L}\left(\frac{L^2{x}^3}{18}-\frac{L{x}^4}{24}+\frac{x^5}{120}\right)+C_{1}x+C_2\end{array}$ (4)

$EIy=\frac{w_o}{L}\left(\frac{L^2{x}^3}{18}-\frac{L{x}^4}{24}+\frac{x^5}{120}\right)+C_{1}x+C_2$

Apply the boundary condition in $C_2$ as follows:

At $x=0$, $y=0$

Find the $C_2$ value as shown follows:

Substitute 0 for x and 0 for $y$ in Equation (4).

$\begin{array}{c}EIy=\frac{w_o}{L}\left(\frac{L^2{\left(0\right)}^3}{18}-\frac{L{\left(0\right)}^4}{24}+\frac{{\left(0\right)}^5}{120}\right)+C_1\left(0\right)+C_2\\ =\frac{w_o}{L}\left(0-0+0\right)+0+C_2\\ C_2=0\end{array}$

Apply the boundary condition in $C_1$ as follows:

At $x=L$, $y=0$

Find the $C_1$ value as shown follows:

Substitute 0 for x and 0 for $y$ in Equation (4).

$\begin{array}{l}EI\left(0\right)=\frac{w_o}{L}\left(\frac{L^2{\left(L\right)}^3}{18}-\frac{L{\left(L\right)}^4}{24}+\frac{{\left(L\right)}^5}{120}\right)+C_1\left(L\right)+C_2\\ 0=\frac{w_o}{L}\left(\frac{L^5}{18}-\frac{L^5}{24}+\frac{{\left(L\right)}^5}{120}\right)+C_1\left(L\right)+0\\ -C_1\left(L\right)=\frac{w_o}{L}\left(\frac{L^5}{45}\right)\\ -C_1\left(L\right)=\frac{w_o{L}^4}{45}\end{array}$

$C_1=-\frac{w_o{L}^3}{45}$

Substitute $-\frac{w_o{L}^3}{45}$ for $C_1$ and 0 for $C_2$ in Equation (4).

$\begin{array}{c}EIy=\frac{w_o}{L}\left(\frac{L^2{x}^3}{18}-\frac{L{x}^4}{24}+\frac{x^5}{120}\right)-\frac{w_o{L}^3}{45}x+0\\ y=\frac{w_o}{EIL}\left(\frac{1}{18}{L}^2{x}^3-\frac{1}{24}L{x}^4+\frac{1}{120}{x}^5-\frac{1}{45}{L}^{4}x\right)\end{array}$ (5)

Differentiate with respect to x in Equation (5).

$\frac{dy}{dx}=\frac{w_o}{EIL}\left(\frac{1}{6}{L}^2{x}^2-\frac{1}{6}L{x}^3+\frac{1}{24}{x}^4-\frac{1}{45}{L}^4\right)$ (6)

To find the location of maximum deflection:

$\frac{dy}{dx}=0$

$f=\frac{1}{6}{L}^2{x}_m^2-\frac{1}{6}L{x}_m^3+\frac{1}{24}{x}_m^4-\frac{1}{45}{L}^4$

Consider the function $z=\frac{x_m}{L}$.

$f\left(z\right)=\frac{1}{6}{z}^2-\frac{1}{6}{z}^3+\frac{1}{24}{z}^4-\frac{1}{45}$ (7)

Differentiate with respect to z in Equation (7).

$\begin{array}{l}f\left(z\right)=\frac{1}{6}{z}^2-\frac{1}{6}{z}^3+\frac{1}{24}{z}^4-\frac{1}{45}\\ \frac{df}{dz}=\frac{1}{3}z-\frac{z^2}{2}+\frac{1}{6}{z}^3\end{array}$

Find the value z using Newton-Raphson method as follows:

$z=z_0-\frac{f\left(z_0\right)}{\frac{df}{dz}}$ (8)

Show the calculated values of $f\left(z\right)$, $\frac{df}{dz}$, and z values as shown in Table 1.

$z_0$	$f\left(z\right)$	$\frac{df}{dz}$	$z$
0.22	-0.01583	0.050908	0.53100
0.24	-0.01479	0.053504	0.51639
0.26	-0.01369	0.055796	0.50544
0.28	-0.01256	0.057792	0.49730
0.3	-0.01138	0.0595	0.49134
0.32	-0.01018	0.060928	0.48708
0.34	-0.00895	0.062084	0.48415
0.36	-0.0077	0.062976	0.48224
0.38	-0.00643	0.063612	0.48111
0.4	-0.00516	0.064	0.48056
0.42	-0.00387	0.064148	0.48039
0.44	-0.00259	0.064064	0.48045
0.46	-0.00131	0.063756	0.48059
0.48	-4.2E-05	0.063232	0.4807
0.5000	0.0012	0.0625	0.4806
0.52	0.002456	0.061568	0.48010

Refer to table: 1.

The value of $x_m$ is $0.481L$.

Find the value of $y_m$ using the relation as follows:

Substitute $0.481L$ for $x_m$ in Equation (5).

$\begin{array}{c}{y}_m=\frac{w_o}{EIL}\left(\frac{1}{18}{L}^2{\left(0.481L\right)}^3-\frac{1}{24}L{\left(0.481L\right)}^4+\frac{1}{120}{\left(0.481L\right)}^5-\frac{1}{45}{L}^4\left(0.481L\right)\right)\\ =\frac{w_o{L}^4}{EIL}\left(\frac{1}{18}{\left(0.4807\right)}^3-\frac{1}{24}{\left(0.4807\right)}^4+\frac{1}{120}{\left(0.4807\right)}^5-\frac{1}{45}\left(0.4807\right)\right)\\ =\frac{w_o{L}^4}{EIL}\left(0.00617-0.00222+0.00021388-0.01068\right)\\ =-0.00652\frac{w_o{L}^4}{EIL}\end{array}$

Therefore, he magnitude of the maximum deflection in terms of $w_{0,}$ $L,$ $E,$ and I is $\underset{\_}{0.00652\frac{w_0{L}^4}{EI}\downarrow }$.

Therefore, the location of maximum deflection is $\underset{\_}{0.481L}$.

To determine

The value of maximum deflection.

Answer to Problem 11P

The value of maximum deflection is $\underset{\_}{0.229\text{in}\text{.}}$

Explanation of Solution

Calculation:

Convert $\text{kips}/\text{ft}$ to $\text{lb}/\text{in}\text{.}$

$\begin{array}{c}{w}_0=4.5\text{kips}/\text{ft}\\ =\frac{4500}{12}\\ =375\text{lb}/\text{in}\text{.}\end{array}$

The rolled shape section $W18\times 50$,

The value of $I$ is $800{\text{in}}^{\text{4}}$.

Find the maximum deflection using the relation:

$y_m=-0.00652\frac{w_0{L}^4}{EIL}$ (9).

Substitute $375\text{lb}/\text{in}\text{.}$ for $w_0$, $18\text{ft}$ for L, and $800{\text{in}}^{\text{4}}$ for I in Equation (9).

$\begin{array}{c}{y}_m=-0.00652\frac{375\times 18\text{ft}\left(\frac{12\text{in}}{1\text{ft}}\right)}{29\times {10}^6\left(800\right)}\\ =-0.00652\frac{375\times {\left(216\right)}^4}{29\times {10}^6\left(800\right)}\\ =-0.229\text{in}\text{.}\end{array}$

Thus, the value of maximum deflection is $\underset{\_}{0.229\text{in}.\downarrow }$