
Concept explainers
-10 Derive the equations of the deflection curve for beam AB with sliding support at A and roller support at B, supporting a distributed load of maximum intensity q0acting on the right-hand half of the beam (see figure). Also, determine deflection
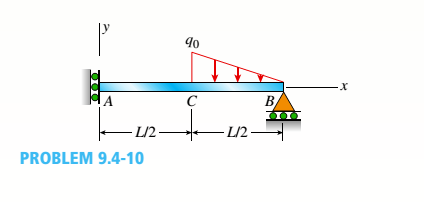
To derive: the equations of deflection curve for beam. Also, find deflection
Answer to Problem 9.4.10P
Equations of deflection curve:
With guiding support at A,
With roller support at B,
Deflection
Angle of rotation:
Deflection
Explanation of Solution
Given:
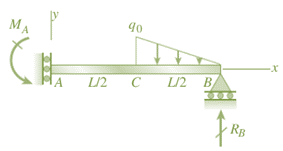
Load equation:
Upon integration
Upon integration
Boundary condition 1:
Therefore,
Boundary condition 2:
Therefore,
Upon integration
Boundary condition 3:
Therefore,
Load equation:
Upon integration
Upon integration
Boundary condition 4:
Therefore,
Boundary condition 5:
Therefore,
Upon integration
Boundary condition 6
Therefore,
Upon integration
Boundary condition 7:
Therefore,
B.C 8:
Thus, we have,
At
And
At
And
At
Want to see more full solutions like this?
Chapter 9 Solutions
Mechanics of Materials (MindTap Course List)
- (read image)arrow_forwardQu 2 Schematically plot attractive, repulsive, and net energies versus interatomic separation for two atoms or ions. Note on this plot the equilibrium separation (distance) ro and the bonding energy Eo. Qu 3 How many atoms (or molecules) are in one mole of the substance? Qu 4 Mole, in the context of this book, is taken in units of gram-mole. On this basis, how many atoms are there in a pound-mole of a substance? Qu 5 The atomic radii of Mg* and F ions are 0.072 and 0.133 nm, respectively. Calculate the force of attraction between these two ions at their equilibrium interionic separation (i.e., when the ions just touch one another). What is the force of repulsion at this same separation distance?show all work step by step problems formulaarrow_forwardQu 4 Silver has FCC crystal structure at room temperature, and a lattice constant, a, of 0.407 nm. Draw a reduced sphere silver unit cell in the grids provided below, clearly label the lattice dimensions. Within the unit cell you drew, shade the (1 0 0) plane. How many atoms are contained within the (1 0 0) plane? Calculate the area of (1 0 0) plane in [nm?]. Express your answer in [nm?] to three significant figures. Calculate the planar density of the (1 0 0) plane in [atoms/nm?]. Express the answer in atoms/nm to three significant figures. show all work step by steparrow_forward
- Can I get help on this question?arrow_forwardDuring some actual expansion and compression processes in piston–cylinder devices, the gases have been observed to satisfy the relationship PVn = C, where n and C are constants. Calculate the work done when a gas expands from 350 kPa and 0.03 m3 to a final volume of 0.2 m3 for the case of n = 1.5. The work done in this case is kJ.arrow_forwardCarbon dioxide contained in a piston–cylinder device is compressed from 0.3 to 0.1 m3. During the process, the pressure and volume are related by P = aV–2, where a = 6 kPa·m6. Calculate the work done on carbon dioxide during this process. The work done on carbon dioxide during this process is kJ.arrow_forward
- The volume of 1 kg of helium in a piston–cylinder device is initially 5 m3. Now helium is compressed to 3 m3 while its pressure is maintained constant at 130 kPa. Determine the initial and final temperatures of helium as well as the work required to compress it, in kJ. The gas constant of helium is R = 2.0769 kJ/kg·K. The initial temperature of helium is K. The final temperature of helium is K. The work required to compress helium is kJ.arrow_forwardA piston-cylinder device initially contains 0.4 kg of nitrogen gas at 160 kPa and 140°C. Nitrogen is now expanded isothermally to a pressure of 80 kPa. Determine the boundary work done during this process. The properties of nitrogen are R= 0.2968 kJ/kg-K and k= 1.4. N₂ 160 kPa 140°C The boundary work done during this process is KJ.arrow_forward! Required information An abrasive cutoff wheel has a diameter of 5 in, is 1/16 in thick, and has a 3/4-in bore. The wheel weighs 4.80 oz and runs at 11,700 rev/min. The wheel material is isotropic, with a Poisson's ratio of 0.20, and has an ultimate strength of 12 kpsi. Choose the correct equation from the following options: Multiple Choice о σmax= (314) (4r2 — r²) - о σmax = p² (3+) (4r² + r²) 16 σmax = (314) (4r² + r²) σmax = (314) (4² - r²)arrow_forward
 Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
