
Interpretation:
To show
To show the Jacobian matrix for the linearization has the form
Here, A and B are
By considering trace and determinant of matrix
To show that depending on the sizes of g and T, the determinant of matrix
By using computer, show that Hopf bifurcation can be supercritical.
Concept Introduction:
To find fixed point of the system, put
Jacobian matrix is used to check the stability of the fixed points, and it is given as:
The system which is settling down to equilibrium by exponentially damping and its decay rate depends on a control parameter
Answer to Problem 17E
Solution:
It is shown that the Jacobian matrix for the linearization has the form
It is shown that all eigenvalues of the matrix
It is shown that values of g and T determine whether the determinant of matrix
It is shown that the system goes through supercritical Hopf bifurcation.
Explanation of Solution
a)
The system equations are:
Assuming that
Here, the condition of
In above equation, L.H.S. and R.H.S. are zero and positive respectively when
Thus, the curves intersect and have unique symmetric solution.
b)
The Jacobian matrix is:
To prove determinant law,
Expanding along last column,
Again expanding along bottom row,
Therefore,
This result is used for calculation of eigenvalues of the
c)
Using determinant is product of the eigenvalues and trace is sum of eigenvalues,
But
Here,
From the above result,
The trace
Thus,
d)
For the matrix
The determinant
And trace
From the above results, the determinant is positive if
Trace can negative or positive depending upon values of b and T.
From the above conclusions of eigenvalues, pitchfork or Hopf bifurcation can occur at the point
By adjusting parameter g, if initially trace is negative, and by varying parameter g, its value is shifting towards positive value, then the pitchfork bifurcation occurs at
By adjusting parameter T, if the determinant is positive, by changing the parameter T, the trace of the matrix changes its sign, then the Hopf bifurcation occurs at a
e)
The plots of
For T being very small, the system is nearly settled down to fixed point

Increasing the value of T, the stable limit cycles just appear.
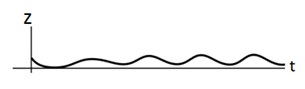
Further increasing the value of T, the size of the limit cycle increases.
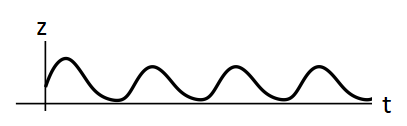
Therefore, the system goes through supercritical Hopf bifurcation.
Want to see more full solutions like this?
Chapter 8 Solutions
Nonlinear Dynamics and Chaos
- Let M = M₁U M₂ UM3 and K M₁ = {(x1, x2) ER²: 2 ≤ x ≤ 8, 2≤ x ≤8}, M₂ = {(x1, x2)™ € R² : 4 ≤ x₁ ≤ 6, 0 ≤ x2 ≤ 10}, M3 = {(x1, x2) Є R²: 0 ≤ x₁ ≤ 10, 4≤ x ≤ 6}, ¯ = cone {(1, 2), (1,3)†} ≤ R². (a) Determine the set E(M,K) of efficient points of M with respect to K. (b) Determine the set P(M, K) of properly efficient points of M with respect to K.arrow_forward5.17 An aluminum curtain wall panel 12 feet high is attached to large concrete columns (top and bottom) when the temperature is 65°F. No provision is made for differen- tial thermal movement vertically. Because of insulation between them, the sun heats up the wall panel to 120°F but the column to only 80°F. Determine the consequent compressive stress in the curtain wall. CONCRETE COLUMNS CONNECTIONS Stress= ALUMINUM WALL PANEL 12'-0"arrow_forward6.2 יך 4" 2" 2" Find the centroid of the following cross-sections and planes. X= Y=arrow_forward
- Find the directional derivative of the function at P in direction Varrow_forward6.4 49 Find the centroid of the following cross-sections and planes. X=_ Y= C15 XAO (CENTERED) KW14x90arrow_forward5.18 The steel rails of a continuous, straight railroad track are each 60 feet long and are laid with spaces be- tween their ends of 0.25 inch at 70°F. a. At what temperature will the rails touch end to end? b. What compressive stress will be produced in the rails if the temperature rises to 150°F? T= Stress= L= 60' 25 @T=70°Farrow_forward
- Strength of Materials Problems 5.16 A long concrete bearing wall has vertical expansion joints placed every 40 feet. Determine the required width of the gap in a joint if it is wide open at 20°F and just barely closed at 80°F. Assume α = 6 × 10-6/°F. Width= CONCRETE BEARING WALL EXPANSION JOINT 40' 40' 40' 293arrow_forwardCan you show me a step by step explanation please.arrow_forward9.7 Given the equations 0.5x₁-x2=-9.5 1.02x₁ - 2x2 = -18.8 (a) Solve graphically. (b) Compute the determinant. (c) On the basis of (a) and (b), what would you expect regarding the system's condition? (d) Solve by the elimination of unknowns. (e) Solve again, but with a modified slightly to 0.52. Interpret your results.arrow_forward
- 12.42 The steady-state distribution of temperature on a heated plate can be modeled by the Laplace equation, 0= FT T + 200°C 25°C 25°C T22 0°C T₁ T21 200°C FIGURE P12.42 75°C 75°C 00°C If the plate is represented by a series of nodes (Fig. P12.42), cen- tered finite-divided differences can be substituted for the second derivatives, which results in a system of linear algebraic equations. Use the Gauss-Seidel method to solve for the temperatures of the nodes in Fig. P12.42.arrow_forward9.22 Develop, debug, and test a program in either a high-level language or a macro language of your choice to solve a system of equations with Gauss-Jordan elimination without partial pivoting. Base the program on the pseudocode from Fig. 9.10. Test the program using the same system as in Prob. 9.18. Compute the total number of flops in your algorithm to verify Eq. 9.37. FIGURE 9.10 Pseudocode to implement the Gauss-Jordan algorithm with- out partial pivoting. SUB GaussJordan(aug, m, n, x) DOFOR k = 1, m d = aug(k, k) DOFOR j = 1, n aug(k, j) = aug(k, j)/d END DO DOFOR 1 = 1, m IF 1 % K THEN d = aug(i, k) DOFOR j = k, n aug(1, j) END DO aug(1, j) - d*aug(k, j) END IF END DO END DO DOFOR k = 1, m x(k) = aug(k, n) END DO END GaussJordanarrow_forward11.9 Recall from Prob. 10.8, that the following system of equations is designed to determine concentrations (the e's in g/m³) in a series of coupled reactors as a function of amount of mass input to each reactor (the right-hand sides are in g/day): 15c3cc33300 -3c18c26c3 = 1200 -4c₁₂+12c3 = 2400 Solve this problem with the Gauss-Seidel method to & = 5%.arrow_forward
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,






