
Concept explainers
(a)
To find: The model predict about the aphids.
(a)
Answer to Problem 12E
In the absence of ladybugs we expect the aphid population to stabilize at 10,000
Explanation of Solution
Given information:
The modelled populations of aphids and ladybugs with a Lotka- Volterra system.
Suppose we modify those equations as follows:
Formula used:
Substitution method is used.
Calculation:
If
So,
Or
Since,
The aphid population to increase to 10,000 for these values of A
Since,
The aphid population to decrease to 10,000 for these values of A
Therefore,in the absence of ladybugs we expect the aphid population to stabilize at 10,000
Conclusion:
In the absence of ladybugs we expect the aphid population to stabilize at 10,000
(b)
To find:The equilibrium solutions.
(b)
Answer to Problem 12E
The equilibrium solution is
(i) L= 0, A= 0 (ii) L= 0, A=10,000 (iii) A=5000, L= 100
Explanation of Solution
Given information:
The populations of aphids and ladybugs with a Lotka- Volterra system.
Suppose the equations as follows:
Formula used:
Calculation:
A and L are constant
The second equation is true if L = 0 or A = 0.5/0.0001 = 5000.
If L = 0 in the first equation, then either A = 0 or A = 1100001 = 10,000.
If A = 5000, then we have that 0 = 5000[2(1 - 0.0001*5000) - 0.01 L]
So the equilibrium solution is
(i) L= 0, A= 0 (ii) L= 0, A=10,000 (iii) A=5000, L= 100
Conclusion:
The equilibrium solution is
(i) L= 0, A= 0 (ii) L= 0, A=10,000 (iii) A=5000, L= 100
(c)
To find: The expression for
(c)
Answer to Problem 12E
The value is
Explanation of Solution
Given information:
The populations of aphids and ladybugs with a Lotka- Volterra system.
Suppose equations as follows:
Formula used:
Calculation:
Conclusion:
The value is
(d)
To find:The phase trajectories being in common.
(d)
Answer to Problem 12E
The entire phase trajectories spiral tightly around the equilibrium solution (5000, 100)
Explanation of Solution
Given information:
The populations of aphids and ladybugs with a Lotka- Volterra system.
Suppose the equations as follows:
Formula used:
The graph is plotted against x axis and y axis.
Calculation:
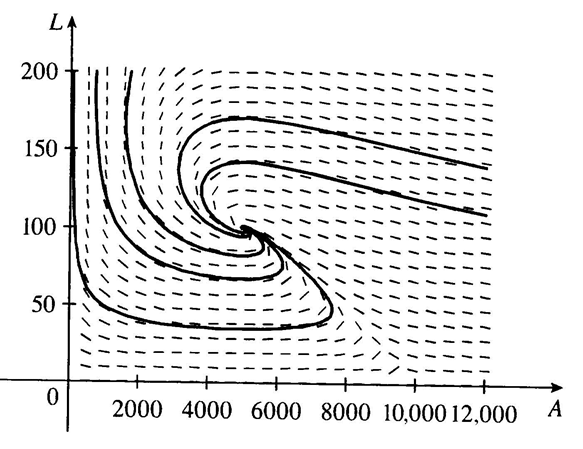
The entire phase trajectories spiral tightly around the equilibrium solution (5000, 100)
Conclusion:
The entire phase trajectories spiral tightly around the equilibrium solution (5000, 100)
(e)
To explain: the population value get changed.
(e)
Answer to Problem 12E
The value get increases and decreases based on the values.
Explanation of Solution
Given information:
The modelled populations of aphids and ladybugs with a Lotka- Volterra system.
Suppose the equation equations as follows:
Formula used:
The graph is plotted against x axis and y axis.
Calculation:
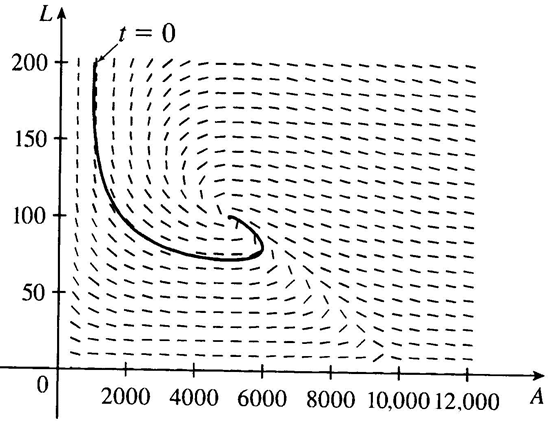
Conclusion:
The value get increases and decreases based on the values.
(f)
To explain: The graph being related to each other.
(f)
Answer to Problem 12E
The ladybug population starts to increase and quickly stabilizes at 100, while the aphid population stabilizes at 5000
Explanation of Solution
Given information:
The populations of aphids and ladybugs with a Lotka- Volterra system.
Suppose the equations as follows:
Formula used:
Substitution method is used.
Calculation:
At t = 0, the ladybug population decreases rapidly and the aphid population decreases slightly before beginning to increase. As the aphid population continues to increase, the ladybug population reaches a minimum at about (5000, 75). The ladybug population starts to increase and quickly stabilizes at 100, while the aphid population stabilizes at 5000.
Conclusion:
The ladybug population starts to increase and quickly stabilizes at 100, while the aphid population stabilizes at 5000
(f)
To explain: The graph being related to each other.
(f)
Answer to Problem 12E
The graph of A peaks just after the graph of L has a minimum.
Explanation of Solution
Given information:
Use pan (e) to make rough sketches of the aphid and ladybug populations as functions of t. How are the graphs related to each other?
Formula used:
The graph is plotted against x axis and y axis.
Calculation:
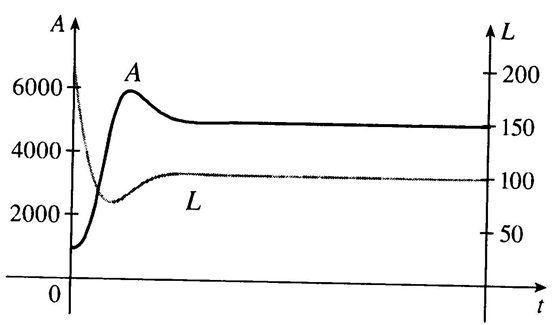
The graph of A peaks just after the graph of L has a minimum.
Conclusion:
The graph of A peaks just after the graph of L has a minimum.
Chapter 7 Solutions
Single Variable Calculus: Concepts and Contexts, Enhanced Edition
- For each graph in Figure 16, determine whether f (1) is larger or smaller than the slope of the secant line between x = 1 and x = 1 + h for h > 0. Explain your reasoningarrow_forwardPoints z1 and z2 are shown on the graph.z1 is at (4 real,6 imaginary), z2 is at (-5 real, 2 imaginary)Part A: Identify the points in standard form and find the distance between them.Part B: Give the complex conjugate of z2 and explain how to find it geometrically.Part C: Find z2 − z1 geometrically and explain your steps.arrow_forwardA polar curve is represented by the equation r1 = 7 + 4cos θ.Part A: What type of limaçon is this curve? Justify your answer using the constants in the equation.Part B: Is the curve symmetrical to the polar axis or the line θ = pi/2 Justify your answer algebraically.Part C: What are the two main differences between the graphs of r1 = 7 + 4cos θ and r2 = 4 + 4cos θ?arrow_forward
- A curve, described by x2 + y2 + 8x = 0, has a point A at (−4, 4) on the curve.Part A: What are the polar coordinates of A? Give an exact answer.Part B: What is the polar form of the equation? What type of polar curve is this?Part C: What is the directed distance when Ø = 5pi/6 Give an exact answer.arrow_forwardNew folder 10. Find the area enclosed by the loop of the curve (1- t², t-t³)arrow_forward1. Graph and find the corresponding Cartesian equation for: t X== y = t +1 2 te(-∞, ∞) 42,369 I APR 27 F5 3 MacBook Air stv A Aa T 4 DIIarrow_forward
- Middle School GP... Echo home (1) Addition and su... Google Docs Netflix Netflix New folder 9. Find the area enclosed by x = sin²t, y = cost and the y-axis.arrow_forward2. Graph and find the corresponding Cartesian equation for: (4 cos 0,9 sin 0) θ ε [0, 2π) 42,369 I APR 27 3 MacBook Air 2 tv A Aaarrow_forward30 Page< 3. Find the equation of the tangent line for x = 1+12, y = 1-3 at t = 2 42,369 APR A 27 M . tv NA 1 TAGN 2 Aa 7 MacBook Air #8arrow_forward
- Evaluate the following integrals as they are writtenarrow_forwardCalculus lll May I please have the blank lines completed, and final statement defined as a result? Thank you for the support!arrow_forward3. Consider the polynomial equation 6-iz+7z² - iz³ +z = 0 for which the roots are 3i, -2i, -i, and i. (a) Verify the relations between this roots and the coefficients of the polynomial. (b) Find the annulus region in which the roots lie.arrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





