
Calculate the current response
Answer to Problem 57P
The current response
Explanation of Solution
Given data:
Refer to Figure 7.123 in the textbook.
The value of inductance
The value of inductance
Formula used:
Write the general expression to find the current response for the RL circuit.
Here,
Write the expression to calculate the time constant for the RL circuit.
Here,
L is the inductance of the inductor.
Write the general expression for the unit step function.
Calculation:
For
The given Figure 7.123 is redrawn as shown in Figure 1. At this condition, the inductor reaches steady state and acts like a short circuit to DC.

In Figure 1, apply Kirchhoff’s current law at node
Simplify the equation as follows,
Now find the initial inductor current
Substitute
Now find the initial inductor current
Substitute
For
Figure 2 shows the modified circuit diagram when the switch is kept in closed position.
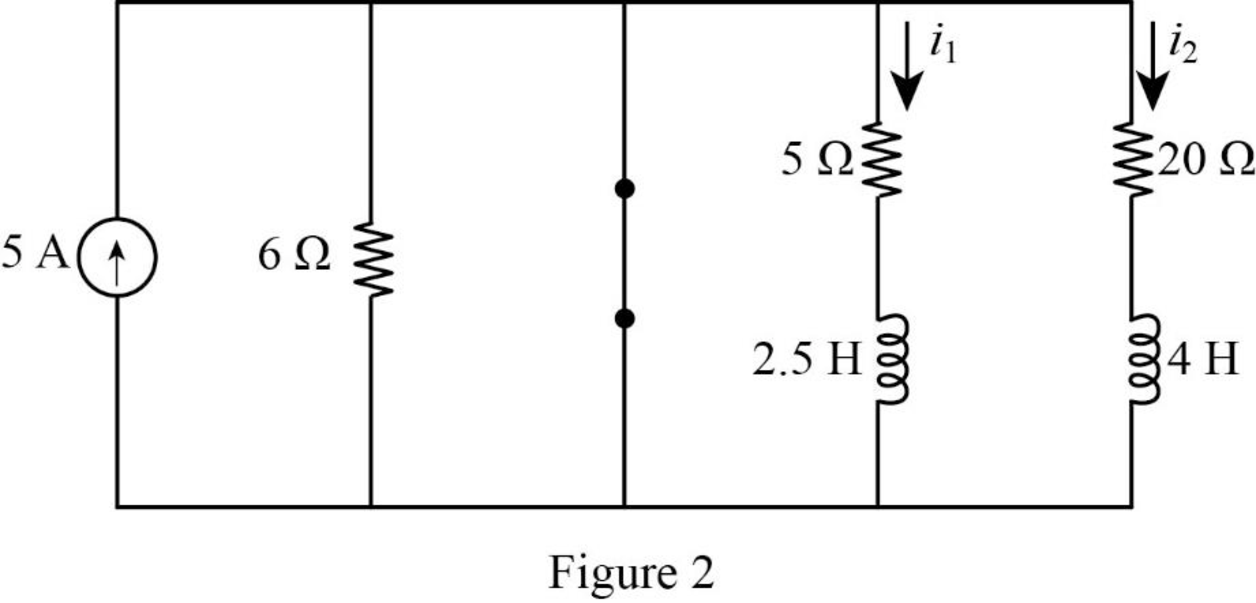
Consider the value of resistances
In Figure 2, the switch is closed so that the energies in the inductance
The current response
The time constant in equation (6) can be rewritten as like in equation (2) as follows.
Substitute
Substitute the units
Substitute
Apply the unit step function in equation (3) to equation (9).
The current response
The time constant in equation (10) can be rewritten as like in equation (2) as follows.
Substitute
Substitute the units
Substitute
Apply the unit step function in equation (3) to equation (13).
Convert the unit A to mA.
Conclusion:
Thus, the current response
Want to see more full solutions like this?
Chapter 7 Solutions
EBK FUNDAMENTALS OF ELECTRIC CIRCUITS
- Don't use ai to answer I will report you answerarrow_forwardThe values of the elements in the circuit given in the figure are given below. Find the maximum average power that can be transferred to the ZL load. Vg=5cos(10000t) VoltR=38 kilo ohmC=35 nano faradL=150 milli henryarrow_forwardPrelab Information 1. Laboratory Preliminary Discussion Second-order RC Circuit Analysis The second-order RC circuit shown in figure 1 below represents all voltages and impedances as functions of the complex variable, s. Note, of course, that the impedances associated with Rs, R₁, and R2 are constant independent of frequency, so the 's' notation is omitted. Again, one of the advantages of s-domain analysis is that we can apply all of the circuit analysis techniques learned for AC and DC circuits. To generate the s-domain expression for the output voltage, Vout(s) = Vc2(s), for the circuit shown in figure 1, we can apply voltage division in the s-domain as shown in equation 1 below. Equation 1 will be used in the prelab computations to find an expression for the output voltage, vc2(t), in the time domain. Note also that when we collect frequency response data for the circuit it will be operating at AC steady-state conditions for each frequency tested. Note that under AC steady-state…arrow_forward
- Don't use ai to answer I will report you answerarrow_forwardThe power values of the loads in the circuit given in the figure are given below. Accordingly, which of the following is the RMS value of the Vs voltage amplitude? Load 1 (L1): the power factor is 1 and draws 13 kW of power,Load 2 (L2): draws 1 kVA at a forward power factor of 0.6,Load 3 (L3): draws 4 kW of average power and gives 3 kVAR of reactive power.arrow_forwardThe values of the elements in the circuit given in the figure are given below. Find the average power value on the R2 resistor. (Hint: First find the current of the R2 resistor with the loop current method. Four mutual inductance effect expressions should be added to each of the two loop equations.) Vs=238 voltsR1=13 ohmsR2=15 ohmsarrow_forward
- Don't use ai to answer I will report you answerarrow_forwardA transformer bank is composed of three single-phase transformers of10kVA, 20kV/200V; copper losses are 100 W and core lossesthey are 50 W. The bank is connected in Delta on the high voltage side and in star on the sidethe low-voltage side. A. What are the values of voltages B.What are the values of currents C. losses in the core and losses in copper. D. nominal power of the transformer bank.Solve by one of the experts, not using artificial intelligencearrow_forwardA 50 kVA, 13800/208 V transformer connected in Dy has an Rcc of 1% and aXcc of 7% per unit. A. What is the voltage regulation at full load and fp 0.8 in delay using the values of theimpedance (Ω).b. What is the voltage regulation under the same conditions using the system per unit Solve by one of the experts, not using artificial inteliggencearrow_forward
 Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON
Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning
Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education
Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education
Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON
Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,
Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,





