Chapter 6.1, Problem 64E

Solution

To prove: The diagonal is trisected by the lines connecting the midpoints of the opposing sides of a parallelogram at one of its vertices.

Explanation:

The following figure has been obtained according to the problem statement:

  

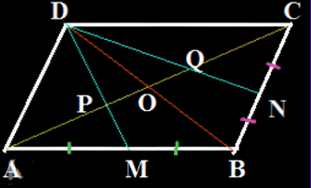
The requirement is to prove $AP=PQ=QC$ .

Consider the $\Delta ADB$ where $AO$ and $DM$ are median. So,

  $\overrightarrow{AP}=\frac{2}{3}\overrightarrow{AO}=\frac{2}{3}\cdot \frac{1}{2}\overrightarrow{AC}=\frac{1}{3}\overrightarrow{AC}$

Similarly, consider the $\Delta CDB$ where $CO$ and $DN$ are median. So,

  $\overrightarrow{CQ}=\frac{2}{3}\overrightarrow{CO}=\frac{2}{3}\cdot \frac{1}{2}\overrightarrow{CA}=\frac{1}{3}\overrightarrow{CA}$

Thus, it can be concluded that,

  $AP=CQ=\frac{1}{3}AC$

It is understood that $PQ=AC-AP-CQ$ . So,

  $\begin{array}{l}PQ=AC-\frac{1}{3}AC-\frac{1}{3}AC\\ PQ=\frac{1}{3}AC\end{array}$

Thus,

  $AP=PQ=QC=\frac{1}{3}AC$

Conclusion:

Hence, it is proved that the diagonal is trisected by the lines connecting the midpoints of the opposing sides of a parallelogram at one of its vertices.