Chapter 5.3, Problem 90P

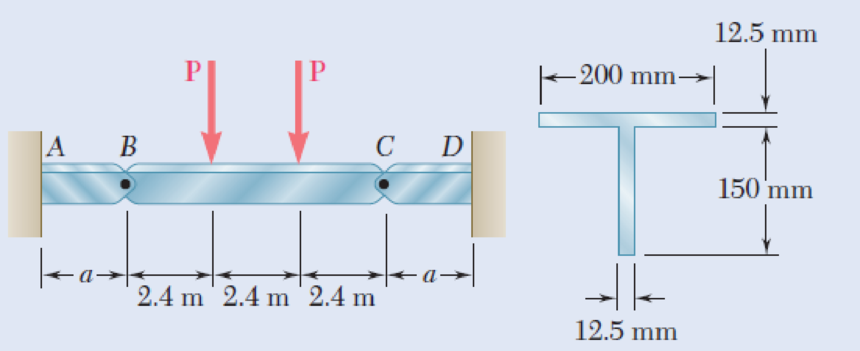
Beams AB, BC, and CD have the cross section shown and are pin-connected at B and C. Knowing that the allowable normal stress is +110 MPa in tension and –150 MPa in compression, determine (a) the largest permissible value of P if beam BC is not to be overstressed, (b) the corresponding maximum distance a for which the cantilever beams AB and CD are not overstressed.

Fig. P5.90

To determine

The largest permissible value of P for the condition that the beam BC is not overstressed.

Answer to Problem 90P

The largest permissible value of P is $\underset{\_}{4.01\text{kN}}$ if the beam BC is not overstressed.

Explanation of Solution

Given information:

The allowable normal stress of the material in tension is ${\left({\sigma }_{all}\right)}_T=+110\text{MPa}$.

The allowable normal stress of the material in compression is ${\left({\sigma }_{all}\right)}_C=-150\text{MPa}$

Calculation:

Show the free-body diagram of the section BC as in Figure 1.

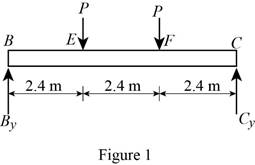
Determine the vertical reaction at point C by taking moment about point B.

$\begin{array}{l}{\displaystyle \sum M_B=0}\\ -P\left(2.4\right)-P\left(4.8\right)+C_y\left(7.2\right)=0\\ -2.4P-4.8P+7.2C_y=0\\ C_y=P\end{array}$

Determine the vertical reaction at point B by resolving the vertical component of forces.

$\begin{array}{l}{\displaystyle \sum F_y=0}\\ B_y-P-P+C_y=0\\ B_y-2P+P=0\\ B_y=P\end{array}$

Show the free-body diagram of the section AB as in Figure 2.

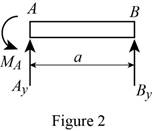
Determine the vertical reaction at point A by resolving the vertical component of forces.

$\begin{array}{l}{\displaystyle \sum F_y=0}\\ A_y-B_y=0\\ A_y-P=0\\ A_y=P\end{array}$

Determine the moment at point A by taking moment about the point A.

$\begin{array}{l}{\displaystyle \sum M_A=0}\\ -B_y\left(a\right)+M_A=0\\ -Pa+M_A=0\\ M_A=Pa\end{array}$

Show the free-body diagram of the section CD as in Figure 3.

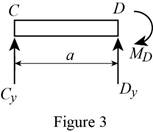
Determine the vertical reaction at point D by resolving the vertical component of forces.

$\begin{array}{l}{\displaystyle \sum F_y=0}\\ D_y-C_y=0\\ D_y-P=0\\ D_y=P\end{array}$

Determine the moment at point D by taking moment about the point D.

$\begin{array}{l}{\displaystyle \sum M_D=0}\\ C_y\left(a\right)-M_D=0\\ Pa-M_D=0\\ M_D=Pa\end{array}$

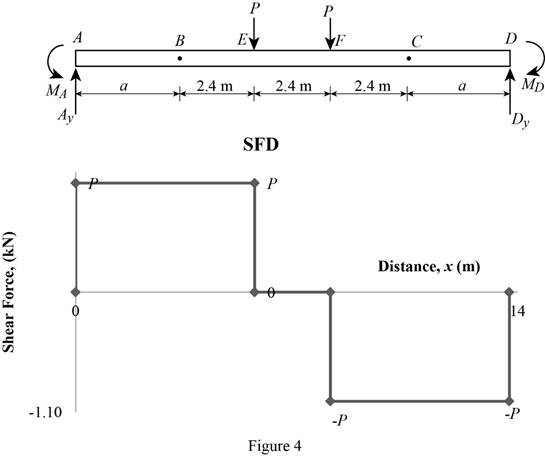
Shear force:

Show the calculation of shear force as follows;

$V_A=P$

$\begin{array}{c}{V}_E-V_A=0\\ V_E^{Left}=V_A\\ =P\end{array}$

$\begin{array}{c}{V}_E^{Right}=P-P\\ =0\end{array}$

$\begin{array}{c}{V}_F-V_E=0\\ V_F^{Left}=V_E\\ =0\end{array}$

$\begin{array}{c}{V}_F^{Right}=0-P\\ =-P\end{array}$

$\begin{array}{c}{V}_D-V_F=0\\ V_D=V_F\\ =-P\end{array}$

Show the calculated shear force values as in Table 1.

Location (x) m	Shear force (V) kN
A	P
E (Left)	P
E (Right)	0
F (Left)	0
F (Right)	–P
D	–P

Plot the shear force diagram as in Figure 4.

Bending moment:

Show the calculation of the bending moment as follows;

$M_A=-Pa$

$M_B=0$

$\begin{array}{c}{M}_E-M_B=P\times 2.4\\ M_E=2.4P+M_B\\ =2.4P+0\\ =2.4P\end{array}$

$\begin{array}{c}{M}_F-M_E=0\\ M_F=M_E\\ =2.4P\end{array}$

$M_C=0$

$M_D=-Pa$

Show the calculated bending moment values as in Table 2.

Location (x) m	Bending moment (M) kN-m
A	­Pa
B	0
E	2.4P
F	2.4P
C	0
D	–Pa

Plot the bending moment diagram as in Figure 5.

Show the free-body diagram of the T-section as in Figure 6.

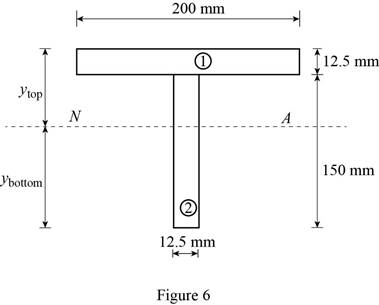
Determine the centroid in y-axis $\left(\overline{y}\right)$ using the equation.

$\overline{y}=\frac{A_1{y}_1+A_2{y}_2}{A_1+A_2}$

Here, the area of the section 1 is $A_1$, the depth of the section 1 from the bottom is $y_1$, the area of the section 2 is $A_2$, and depth of the section 2 from the bottom is $y_2$.

Refer to Figure 4;

$\begin{array}{l}{A}_1=\left(200\times 12.5\right){\text{mm}}^2;y_1=156.25\text{mm}\\ A_2=\left(12.5\times 150\right){\text{mm}}^2;y_2=75\text{mm}\end{array}$

Substitute $\left(200\times 12.5\right){\text{mm}}^2$ for $A_1$, 156.25 mm for $y_1$, $\left(12.5\times 150\right){\text{mm}}^2$ for $A_2$, and 75 mm for $y_2$.

$\begin{array}{c}\overline{y}=\frac{\left(200\times 12.5\times 156.25\right)+\left(12.5\times 150\times 75\right)}{\left(200\times 12.5\right)+\left(12.5\times 150\right)}\\ =121.43\text{mm}\end{array}$

Determine the moment of inertia (I) using the equation.

$I=\frac{b_1{d}_1^3}{12}+A_1{\left(y_1-\overline{y}\right)}^2+\frac{b_2{d}_2^3}{12}+A_2{\left(y_2-\overline{y}\right)}^2$

Here, the depth of the section 1 is $d_1$, the width of the section 1 is $b_1$, the depth of the section 2 is $d_2$, and the width of the section 2 is $b_2$.

Substitute 12.5 mm for $d_1$, 200 mm for $b_1$, $\left(200\times 12.5\right){\text{mm}}^2$ for $A_1$, 156.25 mm for $y_1$, 121.43 mm for $\overline{y}$, 150 mm for $d_2$, 12.5 mm for $b_2$, $\left(12.5\times 150\right){\text{mm}}^2$ for $A_2$, and 75 mm for $y_2$.

$\begin{array}{c}I=\frac{200\times {12.5}^3}{12}+200\times 12.5{\left(156.25-121.43\right)}^2+\frac{12.5\times {150}^3}{12}+12.5\times 150{\left(75-121.43\right)}^2\\ =32,552.08+3,031,081+3,515,625+4,042,021.69\\ =10.621\times {10}^6{\text{mm}}^4\times {\left(\frac{\text{1}\text{m}}{\text{1000}\text{mm}}\right)}^4\\ =10.621\times {10}^{-6}{\text{m}}^4\end{array}$

Refer to Figure 4;

$\begin{array}{l}{y}_{bottom}=-121.43\text{mm}\\ y_{top}=41.07\text{mm}\end{array}$

Tension at Points B and D:

Refer to Figure 5;

Determine the moment at points B and D using the relation.

$M_B\text{and}{M}_D=-\frac{{\left({\sigma }_{all}\right)}_{T}I}{y_{top}}$

Substitute 110 MPa for ${\left({\sigma }_{all}\right)}_T$, $10.621\times {10}^{-6}{\text{m}}^4$ for I, and 41.07 mm for $y_{top}$.

$\begin{array}{c}{M}_B\text{and}{M}_D=-\frac{110\text{MPa}\times \frac{{10}^3{\text{kN/m}}^2}{1\text{MPa}}\times 10.621\times {10}^{-6}}{41.07\text{mm}\times \frac{\text{1}\text{m}}{\text{1000}\text{mm}}}\\ =-28.45\text{kN-m}\end{array}$

Compression at Points B and C:

Refer to Figure 5;

Determine the moment at points B and D using the relation.

$M_B\text{and}{M}_D=-\frac{{\left({\sigma }_{all}\right)}_{C}I}{y_{bottom}}$

Substitute –150 MPa for ${\left({\sigma }_{all}\right)}_C$, $10.621\times {10}^{-6}{\text{m}}^4$ for I, and –121.43 mm for $y_{bottom}$.

$\begin{array}{c}{M}_B\text{and}{M}_D=-\frac{-150\text{MPa}\times \frac{{10}^3{\text{kN/m}}^2}{1\text{MPa}}\times 10.621\times {10}^{-6}}{-121.43\text{mm}\times \frac{\text{1}\text{m}}{\text{1000}\text{mm}}}\\ =-13.12\text{kN-m}\end{array}$

Tension at maximum bending moment:

Refer to Figure 5;

Determine the maximum moment using the relation.

$M_{\max }=-\frac{{\left({\sigma }_{all}\right)}_{T}I}{y_{bottom}}$

Substitute 110 MPa for ${\left({\sigma }_{all}\right)}_T$, $10.621\times {10}^{-6}{\text{m}}^4$ for I, and –121.43 mm for $y_{bottom}$.

$\begin{array}{c}{M}_{\max }=-\frac{110\text{MPa}\times \frac{{10}^3{\text{kN/m}}^2}{1\text{MPa}}\times 10.621\times {10}^{-6}}{-121.43\text{mm}\times \frac{\text{1}\text{m}}{\text{1000}\text{mm}}}\\ =9.62\text{kN-m}\end{array}$

Compression at maximum bending moment:

Refer to Figure 5;

Determine the maximum moment using the relation.

$M_{\max }=-\frac{{\left({\sigma }_{all}\right)}_{C}I}{y_{top}}$

Substitute –150 MPa for ${\left({\sigma }_{all}\right)}_C$, $10.621\times {10}^{-6}{\text{m}}^4$ for I, and 41.07 mm for $y_{top}$.

$\begin{array}{c}{M}_{\max }=-\frac{-150\text{MPa}\times \frac{{10}^3{\text{kN/m}}^2}{1\text{MPa}}\times 10.621\times {10}^{-6}}{41.07\text{mm}\times \frac{\text{1}\text{m}}{\text{1000}\text{mm}}}\\ =38.79\text{kN-m}\end{array}$

Refer to the calculated distribution loads; the smallest value controls the design.

Refer to Figure 5;

Equate the maximum bending moment calculated and the maximum bending moment in the tension side.

$\begin{array}{l}2.4P=9.62\text{kN-m}\\ P=4.01\text{kN}\end{array}$

Therefore, the largest permissible value of P for the condition that the beam BC is not overstressed is $\underset{\_}{4.01\text{kN}}$.

To determine

The maximum distance a for the condition that the beams AB and CD are not overstressed.

Answer to Problem 90P

The maximum distance a for the condition that the beams AB and CD are not overstressed is $\underset{\_}{3.27\text{m}}$.

Explanation of Solution

Refer to Part (a), Figure 4;

The maximum bending moment in the beams AB and CD occurs at the ends A and D.

The calculated maximum bending moment at the points A and D is as follows:

$M_A\text{and}{M}_D=-Pa$

The maximum allowable compression moment at the points A and D is as follows:

$M_B\text{and}{M}_D=-13.12\text{kN-m}$

Equate the values;

$-Pa=-13.12\text{kN-m}$

Refer to the answer of the part (a); $P=4.01\text{kN}$.

Substitute 4.01 kN for P.

$\begin{array}{l}-4.01a=-13.12\text{kN-m}\\ a=3.27\text{m}\end{array}$

Therefore, the maximum distance a for the condition that the beams AB and CD are not overstressed is $\underset{\_}{3.27\text{m}}$.