
Concept explainers
A solid steel bar has a square cross section of side b and is supported as shown. Knowing that for steel ρ = 7860 kg/m3, determine the dimension b for which the maximum normal stress due to bending is (a) 10 MPa, (b) 50 MPa.
Fig. P5.33
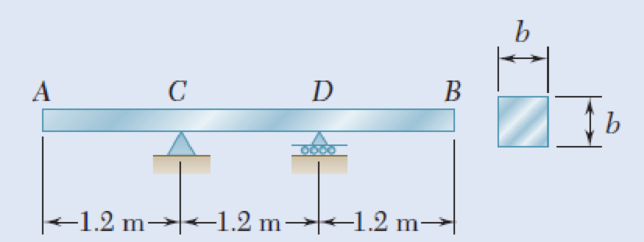
(a)
the dimension b.
Answer to Problem 33P
The dimension b of the square cross section is
Explanation of Solution
Given information:
The maximum normal stress due to bending is 10 MPa.
Determine the weight density
Here, the mass density of the bar is
Consider the acceleration due to gravity as
Substitute
Determine the dead load (W) of the solid steel bar using the relation.
Here, the cross sectional area of the steel bar is A, the length of the beam is L, and the dimension of the bar is d.
Convert the mass density into weight density as follows;
Substitute
Determine the reactions of the beam.
Show the free-body diagram of the beam as in Figure 1.
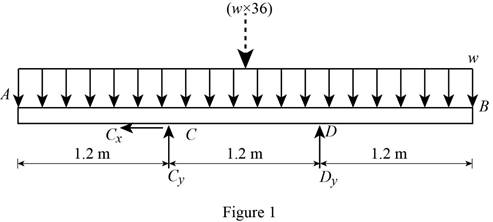
Determine the vertical reaction at point C by taking moment at point D.
Show the free-body diagram of the sections as in Figure 2.
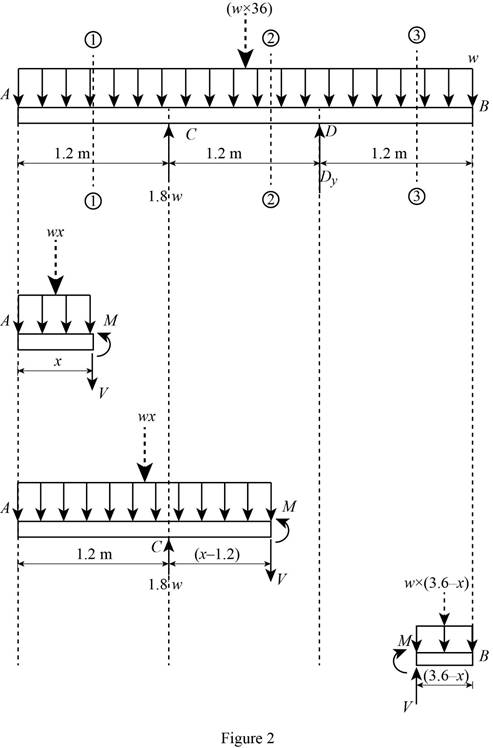
Region AC (Section 1-1):
Determine the bending moment at the section by taking moment about the section.
Region CD (Section 2-2):
Determine the bending moment at the section by taking moment about the section.
Region DB (Section 3-3):
Determine the bending moment at the section by taking moment about the section.
Bending moment values:
Show the calculated bending moment values as in Table 1.
| Location (x) m | Bending moment (M) N-m |
| A (0 m) | 0 |
| C (1-1) (1.2 m) | –0.72w |
| C (2-2) (1.2 m) | –0.72w |
| Mid-point (1.8 m) | –0.54w |
| D (2-2) (2.4 m) | –0.72w |
| D (3-3) (2.4 m) | –0.72w |
| B (3.6 m) | 0 |
Plot the bending moment diagram as in Figure 3.
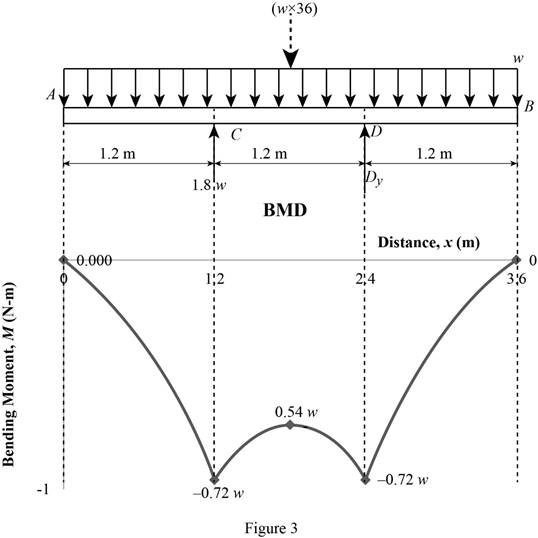
Refer to Figure 3;
The maximum bending moment is
Determine the section modulus (S) of the square section using the equation.
Determine the maximum normal stress
Substitute
Substitute 10 MPa for
Therefore, the dimension b of the square cross section is
(b)
the dimension b.
Answer to Problem 33P
The dimension b of the square cross section is
Explanation of Solution
Given information:
The maximum normal stress due to bending is 50 MPa.
Determine the weight density
Here, the mass density of the bar is
Consider the acceleration due to gravity as
Substitute
Determine the dead load (W) of the solid steel bar using the relation.
Here, the cross sectional area of the steel bar is A, the length of the beam is L, and the dimension of the bar is d.
Convert the mass density into weight density as follows;
Substitute
Determine the reactions of the beam.
Show the free-body diagram of the beam as in Figure 4.
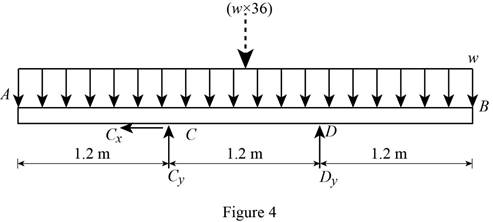
Determine the vertical reaction at point C by taking moment at point D.
Show the free-body diagram of the sections as in Figure 5.
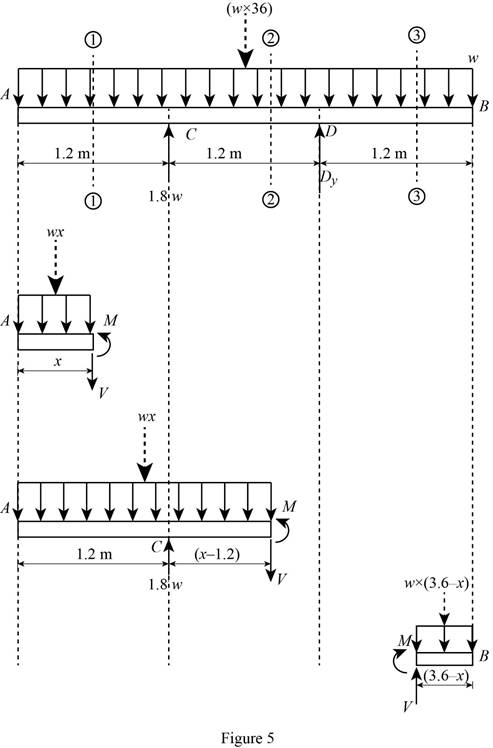
Region AC (Section 1-1):
Determine the bending moment at the section by taking moment about the section.
Region CD (Section 2-2):
Determine the bending moment at the section by taking moment about the section.
Region DB (Section 3-3):
Determine the bending moment at the section by taking moment about the section.
Bending moment values:
Show the calculated bending moment values as in Table 2.
| Location (x) m | Bending moment (M) N-m |
| A (0 m) | 0 |
| C (1-1) (1.2 m) | –0.72w |
| C (2-2) (1.2 m) | –0.72w |
| Mid-point (1.8 m) | –0.54w |
| D (2-2) (2.4 m) | –0.72w |
| D (3-3) (2.4 m) | –0.72w |
| B (3.6 m) | 0 |
Plot the bending moment diagram as in Figure 6.
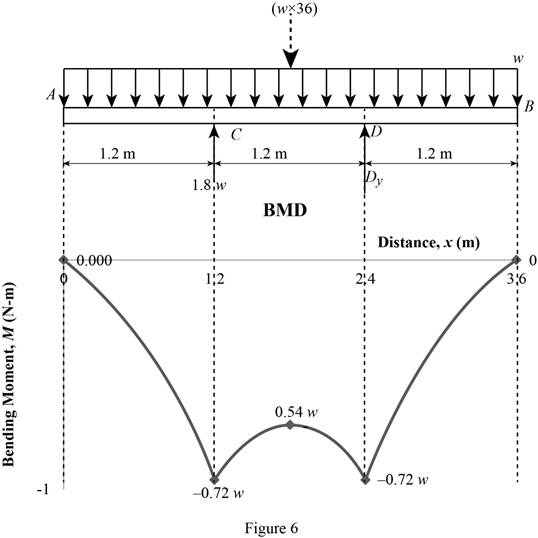
Refer to the Figure 6;
The maximum bending moment is
Determine the section modulus (S) of the square section using the equation.
Determine the maximum normal stress
Substitute
Substitute 50 MPa for
Therefore, the dimension b of the square cross section is
Want to see more full solutions like this?
Chapter 5 Solutions
Mechanics of Materials, 7th Edition
- Need helparrow_forwardY F1 α В X F2 You and your friends are planning to move the log. The log. needs to be moved straight in the x-axis direction and it takes a combined force of 2.9 kN. You (F1) are able to exert 610 N at a = 32°. What magnitude (F2) and direction (B) do you needs your friends to pull? Your friends had to pull at: magnitude in Newton, F2 = direction in degrees, ẞ = N degarrow_forwardProblem 1 8 in. in. PROBLEM 15.109 Knowing that at the instant shown crank BC has a constant angular velocity of 45 rpm clockwise, determine the acceleration (a) of Point A, (b) of Point D. 8 in. Answer: convert rpm to rad/sec first. (a). -51.2j in/s²; (b). 176.6 i + 50.8 j in/s²arrow_forward
- Problem 4 The semicircular disk has a radius of 0.4 m. At one instant, when 0-60°, it is rotating counterclockwise at 0-4 rad/s, which is increasing in the same direction at 1 rad/s². Find the velocity and acceleration of point B at this instant. (Suggestion: Set up relative velocity and relative acceleration that way you would for a no-slip disk; remember what I told you to memorize on the first day of class.) (Answer: B = −2.98î - 0.8ĵ m/s, ãB = 2.45î - 5.74ĵ m/s²) B 0.4 m y Xarrow_forwardA C C 2r A 2r B B (a) (b) Problem 3 Refer to (b) of the figure shown above. The disk OA is now rolling with no slip at a constant angular velocity of w. Find the angular velocity and angular acceleration of link AB and BC. (Partial Answers: WBC = 2wk, AB = w²k)arrow_forwardProblem 2 Refer to (a) of the figure shown below, where the disk OA rotates at a constant angular velocity of w. Find the angular velocity and angular acceleration of link AB and link BC. (Partial Answers: WBC = wk, AB = w²k) A 2r C B (a) A 2r B (b)arrow_forward
- Example Two rotating rods are connected by slider block P. The rod attached at A rotates with a constant clockwise angular velocity WA. For the given data, determine for the position shown (a) the angular velocity of the rod attached at B, (b) the relative velocity of slider block P with respect to the rod on which it slides. b = 8 in., w₁ = 6 rad/s. Given: b = 8 in., WA = 6 rad/s CW constant Find: (a). WBE (b). Vp/Frame E 60° 20° Barrow_forwardY F1 α В X F2 You and your friends are planning to move the log. The log. needs to be moved straight in the x-axis direction and it takes a combined force of 2.9 kN. You (F1) are able to exert 610 N at a = 32°. What magnitude (F2) and direction (B) do you needs your friends to pull? Your friends had to pull at: magnitude in Newton, F2 = direction in degrees, ẞ = N degarrow_forward100 As a spring is heated, its spring constant decreases. Suppose the spring is heated and then cooled so that the spring constant at time t is k(t) = t sin + N/m. If the mass-spring system has mass m = 2 kg and a damping constant b = 1 N-sec/m with initial conditions x(0) = 6 m and x'(0) = -5 m/sec and it is subjected to the harmonic external force f (t) = 100 cos 3t N. Find at least the first four nonzero terms in a power series expansion about t = 0, i.e. Maclaurin series expansion, for the displacement: • Analytically (hand calculations) Creating Simulink Model Plot solutions for first two, three and four non-zero terms as well as the Simulink solution on the same graph for the first 15 sec. The graph must be fully formatted by code.arrow_forward
- Two springs and two masses are attached in a straight vertical line as shown in Figure Q3. The system is set in motion by holding the mass m₂ at its equilibrium position and pushing the mass m₁ downwards of its equilibrium position a distance 2 m and then releasing both masses. if m₁ = m² = 1 kg, k₁ = 3 N/m and k₂ = 2 N/m. (y₁ = 0) www k₁ = 3 Jm₁ = 1 k2=2 www (Net change in spring length =32-31) (y₂ = 0) m₂ = 1 32 32 System in static equilibrium System in motion Figure Q3 - Coupled mass-spring system Determine the equations of motion y₁ (t) and y₂(t) for the two masses m₁ and m₂ respectively: Analytically (hand calculations) Using MATLAB Numerical Functions (ode45) Creating Simulink Model Produce an animation of the system for all solutions for the first minute.arrow_forwardTwo large tanks, each holding 100 L of liquid, are interconnected by pipes, with the liquid flowing from tank A into tank B at a rate of 3 L/min and from B into A at a rate of 1 L/min (see Figure Q1). The liquid inside each tank is kept well stirred. A brine solution with a concentration of 0.2 kg/L of salt flows into tank A at a rate of 6 L/min. The diluted solution flows out of the system from tank A at 4 L/min and from tank B at 2 L/min. If, initially, tank A contains pure water and tank B contains 20 kg of salt. A 6 L/min 0.2 kg/L x(t) 100 L 4 L/min x(0) = 0 kg 3 L/min 1 L/min B y(t) 100 L y(0) = 20 kg 2 L/min Figure Q1 - Mixing problem for interconnected tanks Determine the mass of salt in each tank at time t≥ 0: Analytically (hand calculations) Using MATLAB Numerical Functions (ode45) Creating Simulink Model Plot all solutions on the same graph for the first 15 min. The graph must be fully formatted by code.arrow_forward5. Estimate the friction pressure gradient in a 10.15 cm bore unheated horizontal pipe for the following conditions: Fluid-propylene Pressure 8.175 bar Temperature-7°C Mass flow of liquid-2.42 kg/s. Density of liquid-530 kg/m³ Mass flow of vapour-0.605 kg/s. Density of vapour-1.48 kg/m³arrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





