Chapter 4.2, Problem 11E

(a) Sketch the graph of a function that has a local maximum at 2 and is differentiable at 2.

(b) Sketch the graph of a function that has a local maximum at 2 and is continuous but not differentiable at 2.

(c) Sketch the graph of a function that has a local maximum at 2 and is not continuous at 2.

To determine

To sketch: The graph of a function thatsatisfies the conditions that the graph has local maximum at 2 and is differentiable at 2.

Explanation of Solution

Let the x be represented in the x-axis and the value of the function $f\left(x\right)$ be represented in the y-axis.

Since an absolute maximum occurs at the point 2, choose the greatest point on x-axis. That is, consider the point on (2, 2).

The graph of a differentiable function must have a tangent at each point in its domain. it should be a smooth curve and cannot contain any break or cusps.

Since the graph is differentiable at 2, the derivative of $f^{\prime }\left(2\right)$ exists.

Draw the graph of the function f in such a way that it satisfies the given conditions as shown below in Figure 1.

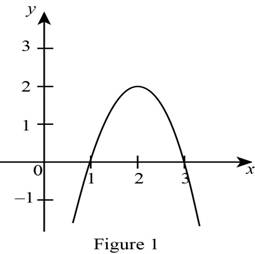
From Figure 1, it is observed that the absolute maximum occurs at x = 2.

Also observe that the graph of the function is continuous and differentiable at x = 2.

To determine

To sketch: The graph of a function that satisfies the conditions that the graph has local maximum at 2 and it is continuous but not differentiable at 2.

Explanation of Solution

Let the x be represented in the x-axis and the value of the function $f\left(x\right)$ be represented in the y-axis.

Since the local maximum occurs at the point 2, choose the point 2 at any open interval of a domain. That is, consider the point on (2, 2).

Since the graph is not differentiable at 2 the derivative of $f^{\prime }\left(2\right)$ does not exists.

Draw the graph of the function f in such a way that it satisfies the given conditions as shown below in Figure 2.

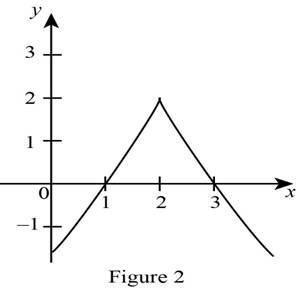
From Figure 2 observe that the local maximum occurs at x = 2. And the curve is continuous.

Also, observe that the graph not differentiable at x = 2.

To determine

To sketch: The graph of a function that satisfies the conditions that the graph has local maximum at 2 and it is not continuous at 2.

Explanation of Solution

Let the x be represented in the x-axis and the value of the function $f\left(x\right)$ be represented in the y-axis.

Since local maximum occurs at the point 2, choose the point 2 on the open interval of a domain. That is, consider the point on (2, 2).

A function is discontinuous at x = 2 if the graph of the function has break at that point.

Draw the graph of the function f in such a way that it satisfies the given conditions as shown below in Figure 3.

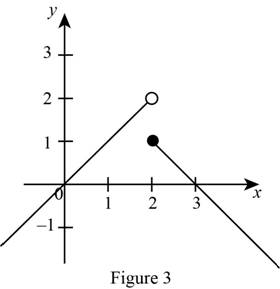
From Figure 3 observe that the local maximum occurs at x = 2 and the function is not continuous at x = 2.