Chapter 4, Problem 5P

The Carbondale Hospital is considering the purchase of a new ambulance. The decision will rest partly on the anticipated mileage to be driven next year. The miles driven during the past 5 years are as follows:

a) Forecast the mileage for next year (6th year) using a 2-year moving average.

b) Find the MAD based on the 2-year moving average. (Hint: You will have only 3 years of matched data.)

c) Use a weighted 2-year moving average with weights of .4 and .6 to forecast next year’s mileage. (The weight of .6 is for the most recent year.) What MAD results from using this approach to forecasting? (Hint: You will have only 3 years of matched data.)

d) Compute the forecast for year 6 using exponential smoothing, an initial forecast for year 1 of 3,000 miles, and α = .5.

Summary Introduction

To determine: Using 2-year moving average, forecast the mileage for the 6^th year.

Introduction: Forecasting is used to predict future changes or demand patterns. It involves different approaches and varies with different time periods. A sequence of data points in successive order is known as a time series. Time series forecasting is the prediction based on past events which are at a uniform time interval.

Answer to Problem 5P

The forecasted mileage for the 6^th year is 3,750.

Explanation of Solution

Given information:

Year	Mileage
1	3,000
2	4,000
3	3,400
4	3,800
5	3,700

Formula to forecast the mileage for the 6^th year:

$\text{Moving}\text{average}=\frac{{\displaystyle \sum \text{demand}\text{in}\text{previous}n\text{periods}}}{n}$

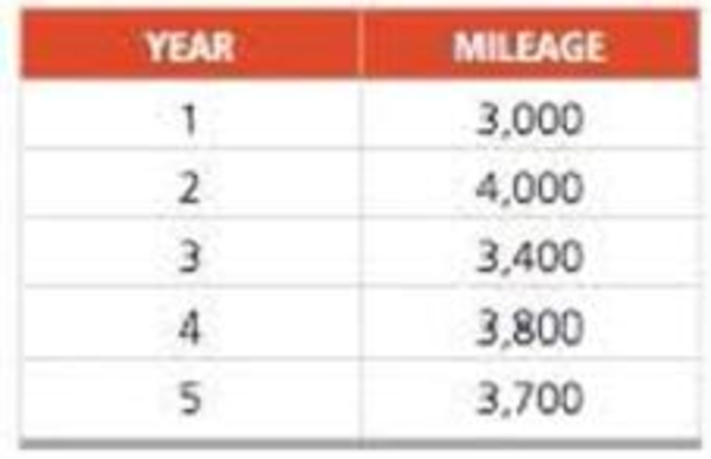
Year	Mileage	Moving Average
1	3,000
2	4,000
3	3,400	3,500
4	3,800	3,700
5	3,700	3,600
6		3,750

Excel worksheet:

Calculation of the mileage forecast for the 6^th year:

The mileage forecast for the 6^th year is obtained by summing up the mileage for the 4^th and 5^thyears and dividing the sum by the $n^{\text{th}}$ period; n=2.

$\begin{array}{c}\text{Moving}\text{average}=\frac{3,800+3,700}{2}\\ =3,750\end{array}$

The forecast for the 6^th year is 3,750.

Hence, using 2-year moving average, the mileage forecast for the 6^th year is 3,750

Summary Introduction

To determine: The computation of the Mean Average Deviation (MAD) using 2-year moving average.

Answer to Problem 5P

Mean Average Deviation (MAD) using 2-year moving average is 100

Explanation of Solution

Given information:

Year	Mileage
1	3,000
2	4,000
3	3,400
4	3,800
5	3,700

Formula to compute MAD:

$\text{MAD}=\frac{{\displaystyle \sum \left|\text{Actual-Forecast}\right|}}{n}$

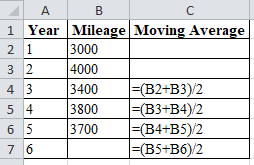
Year	Mileage	Moving Average	Error	Absolute Error
1	3,000
2	4,000
3	3,400	3,500	-100	100
4	3,800	3,700	100	100
5	3,700	3,600	100	100
			Total	300
			MAD	100

Excel worksheet:

$\text{Absolute}\text{Error}=\left|\text{Actual}-\text{Forecast}\right|$

Calculation of the absolute error for year 3:

Moving average:

$\begin{array}{c}\text{Moving}\text{average}=\frac{\text{3,000}+\text{4,000}}{\text{2}}\\ =\text{3,500}\end{array}$

Error:

$\begin{array}{c}\text{Error}=\text{Actual}-\text{Forecast}\\ =\text{3,400}-\text{3,500}\\ =-\text{100}\end{array}$

Absolute error:

$\begin{array}{c}\text{Absolute}\text{error}=\left|\text{Error}\right|\\ =\left|-\text{100}\right|\\ =\text{100}\end{array}$

To calculate the forecast for year 3, divide the summation of the values of year 1&2 by period n i.e. 2. The corresponding value is 3500 which is the forecast for the year 3.

Absolute Error for year 3 is the modulus of the difference between 3400 and 3500, which corresponds to 100. Therefore absolute error for year 3 is 100.

Calculation of the absolute error for year 4:

Moving average:

$\begin{array}{c}\text{Moving}\text{average}=\frac{\text{4,000}+\text{3,400}}{\text{2}}\\ =\text{3,700}\end{array}$

Error:

$\begin{array}{c}\text{Error}=\text{Actual}-\text{Forecast}\\ =\text{3,800}-\text{3,700}\\ =\text{100}\end{array}$

Absolute error:

$\begin{array}{c}\text{Absolute}\text{error}=\left|\text{Error}\right|\\ =\left|\text{100}\right|\\ =\text{100}\end{array}$

To calculate the forecast for year 4, divide the summation of the values of year 2&3 by period n i.e. 2. The corresponding value is 3700 which is the forecast for the year 4.

Absolute Error for year 4 is the modulus of the difference between 3800 & 3700 which corresponds to 100. Therefore Absolute error for Year 4 is 100.

Calculation of the absolute error for year 5:

Moving average:

$\begin{array}{c}\text{Moving}\text{average}=\frac{\text{3,400+3,800}}{\text{2}}\\ =\text{3,600}\end{array}$

Error:

$\begin{array}{c}\text{Error}=\text{Actual}-\text{Forecast}\\ =\text{3,700}-\text{3,600}\\ =\text{100}\end{array}$

Absolute error:

$\begin{array}{c}\text{Absolute}\text{error}=\left|\text{Error}\right|\\ =\left|\text{100}\right|\\ =\text{100}\end{array}$

To calculate the forecast for year 5, divide the summation of the values of year 3&4 by period n i.e. 2. The corresponding value is 3600 which is the forecast for the year 5.

Absolute Error for year 5 is the modulus of the difference between 3,700 & 3,600 which corresponds to 100. Therefore Absolute error for Year 5 is 100.

Calculation of the Mean Absolute Deviation:

Mean Absolute Deviation is obtained by dividing the summation of absolute values by the number of years. Absolute error is obtained by taking modulus for the difference between Actual and forecasted values.

$\begin{array}{c}\text{MAD}=\frac{{\displaystyle \sum \left|\text{Actual}-\text{Forecast}\right|}}{n}\\ =\frac{\text{100}+\text{100}+\text{100}}{\text{3}}\\ =\frac{\text{300}}{\text{3}}\\ =\text{100}\end{array}$

Upon substitution of summation value of absolute error for 3 years i.e. 300 is divided by number of years i.e. 3 yields MAD of 100.

Hence, the computed Mean Average Deviation (MAD) using 2-year moving average is 100

Summary Introduction

To determine: The mileage forecast for year 6 and also compute Mean Absolute Deviation (MAD) using weighted 2-year moving average with weights 0.4 and 0.6

Answer to Problem 5P

The forecast for year 6 and the Mean Absolute Deviation (MAD) using weighted moving averages are 3,741 & 139.67 respectively.

Explanation of Solution

Given information:

Year	Mileage
1	3,000
2	4,000
3	3,400
4	3,800
5	3,700

Formula to compute MAD:

$\begin{array}{c}\text{Weighted}\text{moving}\text{average}=\frac{{\displaystyle \sum \left(\left(\text{Weight}\text{for}\text{period}\text{n}\right)\left(\text{Demand}\text{in}\text{period}\text{n}\right)\right)}}{{\displaystyle \sum \text{Weights}}}\\ \text{MAD}=\frac{{\displaystyle \sum \left|\text{Actual-Forecast}\right|}}{n}\end{array}$

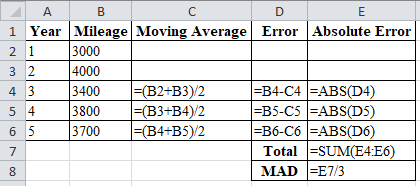
Year	Mileage	Weighted Moving Average	Error	Absolute Error
1	3,000
2	4,000
3	3,400	3,601	-201	201
4	3,800	3,641	159	159
5	3,700	3,641	59	59
6		3,741
			Total	419
			MAD	139.67

Excel worksheet:

Calculation of the weighted moving average for year 3:

$\begin{array}{c}\text{Weighted}\text{moving}\text{average}=\frac{\left(\text{0}\text{.6}\times \text{4,000}\right)+\left(\text{0}\text{.4}\times \text{3,000}\right)}{\text{0}\text{.6}+\text{0}\text{.4}}\\ =\text{3,601}\end{array}$

To calculate the forecast for year 3, multiply the weights with the mileage of recent year, i.e. multiply weight 0.6 with 4,000 and 0.4 with 3,000. Divide the summation of the multiplied values with the summation of the weights i.e. (0.6+0.4).

The corresponding result is 3,601 obtained is the forecasted value for year 3. Therefore, forecasted Weighted Moving Average for year 3 is 3,601.

Calculation of the weighted moving average for year 4:

$\begin{array}{c}\text{Weighted}\text{moving}\text{average}=\frac{\left(\text{0}\text{.6}\times \text{3,400}\right)+\left(\text{0}\text{.4}\times \text{4,000}\right)}{\text{0}\text{.6}+\text{0}\text{.4}}\\ =\text{3,641}\end{array}$

To calculate the forecast for year 4, multiply the weights with the mileage of recent year, i.e. multiply weight 0.6 with 3,400 and 0.4 with 4,000. Divide the summation of the multiplied values with the summation of the weights i.e. (0.6+0.4).

The corresponding result is 3641 obtained is the forecasted value for year 4. Therefore, forecasted Weighted Moving Average for year 3 is 3,641.

Calculation of the weighted moving average for year 5:

$\begin{array}{c}\text{Weighted}\text{moving}\text{average}=\frac{\left(\text{0}\text{.6}\times \text{3,800}\right)+\left(\text{0}\text{.4}\times \text{3,400}\right)}{\text{0}\text{.6}+\text{0}\text{.4}}\\ =\text{3,641}\end{array}$

To calculate the forecast for year 5, multiply the weights with the mileage of recent year, i.e. multiply weight 0.6 with 3,800 and 0.4 with 3,400. Divide the summation of the multiplied values with the summation of the weights i.e. (0.6+0.4).

The corresponding result is 3641 obtained is the forecasted value for year 5. Therefore, forecasted Weighted Moving Average for year 3 is 3,641.

Calculation of the weighted moving average for year 6:

$\begin{array}{c}\text{Weighted}\text{moving}\text{average}=\frac{\left(\text{0}\text{.6}\times \text{3,700}\right)+\left(\text{0}\text{.4}\times \text{3,800}\right)}{\text{0}\text{.6}+\text{0}\text{.4}}\\ =\text{3,741}\end{array}$

To calculate the forecast for year 6, multiply the weights with the mileage of recent year, i.e. multiply weight 0.6 with 3,700 and 0.4 with 3,800. Divide the summation of the multiplied values with the summation of the weights i.e. $\left(0.6+0.4\right)$.

The corresponding result is 3,741 obtained is the forecasted value for year 6. Therefore, forecasted Weighted Moving Average for year 6 is 3,741.

Absolute Error:

Absolute error is the modulus of the difference between the values of actual and forecasted demand.

$\text{Absolute}\text{Error}=\left|\text{Actual}-\text{Forecast}\right|$

Calculation of the absolute error for year 3:

$\begin{array}{c}\text{Error}=\text{Actual}-\text{Forecast}\\ =\text{3,400}-\text{3,601}\\ =-20\text{1}\end{array}$

$\begin{array}{c}\text{Absolute}\text{error}=\left|\text{Error}\right|\\ =\left|-\text{201}\right|\\ =201\end{array}$

Absolute Error for year 3 is the modulus of the difference between 3400 and 3601, which corresponds to 201. Therefore absolute error for year 3is 201;

Calculation of the absolute error for year 4:

$\begin{array}{c}\text{Error}=\text{Actual}-\text{Forecast}\\ =\text{3,800}-\text{3,641}\\ =159\end{array}$

$\begin{array}{c}\text{Absolute}\text{error}=\left|\text{Error}\right|\\ =\left|\text{159}\right|\\ =\text{159}\end{array}$

Absolute Error for year 4 is the modulus of the difference between 3800 and 3641, which corresponds to 159. The absolute error for year 4 is 159.

Calculation of the absolute error for year 5:

$\begin{array}{c}\text{Error}=\text{Actual}-\text{Forecast}\\ =\text{3,700}-\text{3,641}\\ =59\end{array}$

$\begin{array}{c}\text{Absolute}\text{error}=\left|\text{Error}\right|\\ =\left|59\right|\\ =59\end{array}$

Absolute Error for year 5 is the modulus of the difference between 3,700 and 3,641, which corresponds to 59. The absolute error for year 5 is 59.

Calculation of the Mean Absolute Deviation:

$\begin{array}{c}\text{MAD}=\frac{{\displaystyle \sum \left|\text{Actual}-\text{Forecast}\right|}}{n}\\ =\frac{\text{201}+\text{159}+\text{59}}{\text{3}}\\ =\frac{419}{\text{3}}\\ =\text{139}\text{.67}\end{array}$

Upon substitution of summation value of absolute error for 13 years i.e. 419 is divided by number of years i.e. 3 yields MAD of 139.67. Therefore, Mean Absolute Deviation =139.67

Hence, using weighted 2-year moving average with weights 0.4 and 0.6, the mileage forecast for year 6 and the computed Mean Absolute Deviation (MAD)are 3,741and  139.67,respectively.

Summary Introduction

To determine: Using exponential smoothing technique, forecast the mileage for year 6.

Answer to Problem 5P

The mileage forecast for year 6 is 3,662.5

Explanation of Solution

Given information:

Year	Mileage
1	3,000
2	4,000
3	3,400
4	3,800
5	3,700

Formula to calculate the demand forecast:

${\text{F}}_{\text{t}}={\text{F}}_{\text{t-1}}+\text{α}\left({\text{A}}_{\text{t-1}}-{\text{F}}_{\text{t-1}}\right)$

Where,

$\begin{array}{c}{\text{F}}_{\text{t}}=\text{new}\text{forecast}\\ {\text{F}}_{\text{t-1}}=\text{Previous}\text{period's}\text{forecast}\\ \text{α}=\text{smoothing}\text{constant}\\ {\text{A}}_{\text{t-1}}=\text{Previous}\text{period}\text{actual}\text{Demand}\end{array}$

Year	Mileage	Forecast
1	3,000	3,000
2	4,000	3,000
3	3,400	3,500
4	3,800	3,450
5	3,700	3,625
6		3,662.5

Excel worksheet:

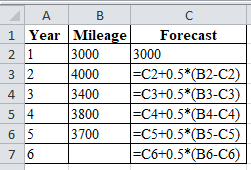
Calculation of the forecast for year 2:

$\begin{array}{c}{\text{F}}_2={\text{F}}_1+\text{α}\left({\text{A}}_1-{\text{F}}_1\right)\\ =3,000+0.5\left(3,000-3,000\right)\\ =3,000\end{array}$

To calculate forecast for the year 2, substitute the value of forecast of year 1, smoothing constant and difference of actual and forecasted mileage in the above formula. The result of forecast for year 2 is 3,000.

Calculation of the forecast for year 3:

$\begin{array}{c}{\text{F}}_3={\text{F}}_2+\text{α}\left({\text{A}}_2-{\text{F}}_2\right)\\ =3,000+0.5\left(4,000-3,000\right)\\ =3,500\end{array}$

To calculate forecast for the year 3, substitute the value of forecast of year 2, smoothing constant and difference of actual and forecasted mileage in the above formula. The result of forecast for year 3 is 3500.

Calculation of the forecast for year 4:

$\begin{array}{c}{\text{F}}_4={\text{F}}_3+\text{α}\left({\text{A}}_3-{\text{F}}_3\right)\\ =3,500+0.5\left(3,400-3,500\right)\\ =3,450\end{array}$

To calculate forecast for the year 4, substitute the value of forecast of year 3, smoothing constant and difference of actual and forecasted mileage in the above formula. The result of forecast for year 2 is 3,450.

Calculation of the forecast for year 5:

$\begin{array}{c}{\text{F}}_5={\text{F}}_4+\text{α}\left({\text{A}}_4-{\text{F}}_4\right)\\ =3,450+0.5\left(3,800-3,450\right)\\ =3,625\end{array}$

To calculate forecast for the year 5, substitute the value of forecast of year 4, smoothing constant and difference of actual and forecasted mileage in the above formula. The result of forecast for year 5 is 3,625.

Calculation of the forecast for year 6:

$\begin{array}{c}{\text{F}}_6={\text{F}}_5+\text{α}\left({\text{A}}_5-{\text{F}}_5\right)\\ =3,625+0.5\left(3,700-3,625\right)\\ =3662.5\end{array}$

To calculate forecast for the year 6, substitute the value of forecast of year 5, smoothing constant and difference of actual and forecasted mileage in the above formula. The result of forecast for year 6 is 3,662.5.

Hence, using exponential smoothing technique, the forecast for year 6 is 3,662.5.