Chapter 2.9, Problem 74P

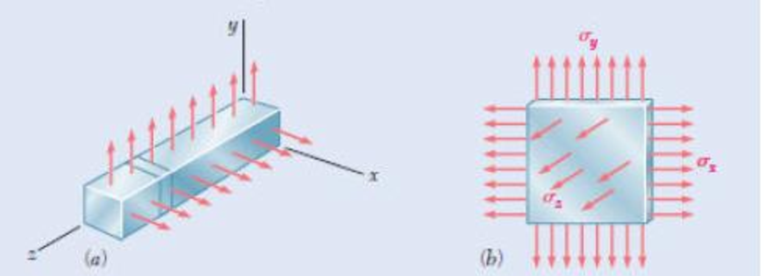
In many situations physical constraints prevent strain from occurring in a given direction. For example, ∈_z = 0 in the case shown, where longitudinal movement of the long prism is prevented at every point. Plane sections perpendicular to the longitudinal axis remain plane and the same distance apart. Show that for this situation, which is known as plane strain, we can express ϭ_z, ∈_x and ∈_y. as follows:

$\begin{array}{l}{\sigma }_z=\nu \left({\sigma }_x+{\sigma }_y\right)\\ {\in }_x=\frac{1}{E}\left[\left(1-{\nu }^2\right){\sigma }_x-\left(1+\nu \right){\sigma }_y\right]\\ {\in }_y=\frac{1}{E}\left[\left(1-{\nu }^2\right){\sigma }_y-\left(1+\nu \right){\sigma }_x\right]\end{array}$